The closure constraint for the hyperbolic tetrahedron as a Bianchi identity

Abstract

The closure constraint is a central piece of the mathematics of loop quantum gravity. It encodes the gauge invariance of the spin network states of quantum geometry and provides them with a geometrical interpretation: each decorated vertex of a spin network is dual to a quantized polyhedron in ${\mathbb R}^{3}$. For instance, a 4-valent vertex is interpreted as a tetrahedron determined by the four normal vectors of its faces. We develop a framework where the closure constraint is re-interpreted as a Bianchi identity, with the normals defined as holonomies around the polyhedron faces of a connection (constructed from the spinning geometry interpretation of twisted geometries). This allows us to define closure constraints for hyperbolic tetrahedra (living in the 3-hyperboloid of unit future-oriented spacelike vectors in ${\mathbb R}^{3,1}$) in terms of normals living all in $\mathrm {SU}(2)$ or in $\mathrm {SB}(2,{\mathbb C})$. The latter fits perfectly with the classical phase space developed for q-deformed loop quantum gravity supposed to account for a non-vanishing cosmological constant $\Lambda >0$. This allows the interpretation of q-deformed twisted geometries as actual discrete hyperbolic triangulations for 4d quantum gravity.

Appendices

Appendix A: Holonomy around a finite hyperbolic triangle

1.1 1. Triad and holonomies in spherical coordinates

We parametrize the hyperboloid, $t^2-x^2-y^2-z^2=\kappa ^2$, $t>0$ in terms of spherical coordinates:

$$\begin{aligned} \left\{ \begin{array}{lcl} t&{}=&{}\kappa \cosh \eta \\ x&{}=&{}\kappa \sinh \eta \sin \theta \cos \phi \\ y&{}=&{}\kappa \sinh \eta \sin \theta \sin \phi \\ z&{}=&{}\kappa \sinh \eta \cos \theta \\ \end{array} \right. \,, \end{aligned}$$

(A1)

with the boost parameter $\eta \in {\mathbb R}_{+}$ and the angles $\theta \in \,[0,\pi ]$ and $\phi \in \,[0,2\pi ]$ The metric on the hyperboloid induced by the flat 4d metric is the standard homogeneous hyperbolic metric $\mathrm {d}s^2=\kappa ^2\mathrm {d}\eta ^2+\kappa ^2\sinh ^2\eta \,(\mathrm {d}\theta ^2+\sin ^2\theta \mathrm {d}\phi ^2)$:

$$\begin{aligned} q_{ab}=\kappa ^2\,\left( \begin{array}{ccc}1&{}&{}\\ {} &{}\sinh ^2\eta &{}\\ &{}&{}\sinh ^2\eta \sin ^2\theta \end{array} \right) \,. \,, \end{aligned}$$

(A2)

This gives a diagonal triad field $e^i_{a}$ such that $q_{ab}=e^i_{a}e^i_{b}$:

$$\begin{aligned} e^i_{a}=(\vec {e}_{\eta },\vec {e}_{\theta },\vec {e}_{\phi }) =\kappa \,\left( \begin{array}{ccc}1&{}&{}\\ {} &{}\sinh \eta &{}\\ {} &{}\quad &{}\quad \sinh \eta \sin \theta \end{array} \right) \,, \end{aligned}$$

(A3)

where we use the vectorial notation for the coordinates on the tangent space. We compute the corresponding spin-connection $\Gamma ^i_{a}$ compatible with the triad, which is given by the usual formula:

$$\begin{aligned} \partial _{[a}e^i_{b]}-\epsilon ^i{}_{jk}\Gamma ^j_{[a}e^k_{b]}=0\,, \qquad \Gamma ^i_{a} = \frac{1}{2}\epsilon ^{ijk}e^b_{k}\,\big ( \partial _{[b}e^j_{a]}+e^c_{j}e^l_{a}\partial _{b}e^l_{c} \big )\,, \end{aligned}$$

(A4)

where $e^a_{i}$ is the inverse triad, $e^a_{i}e^i_{b}=\delta ^a_{b}$. This gives the spin-connection:

$$\begin{aligned} \Gamma ^i_{a}=(\vec {\Gamma }_{\eta },\vec {\Gamma }_{\theta },\vec {\Gamma }_{\phi }) =\, \left( \begin{array}{ccc}0&{}\quad 0&{}\quad \cos \theta \\ 0&{}\quad 0&{}\quad -\sin \theta \cosh \eta \\ 0&{}\quad -\cosh \eta &{}\quad 0\end{array} \right) \,, \end{aligned}$$

(A5)

Finally we get the Ashtekar–Barbero connection A by adding the triad to the spin-connection:

$$\begin{aligned} A^i_{a} =\Gamma ^i_{a}+\beta \,\frac{e^i_{a}}{\kappa } =(\vec {A}_{\eta },\vec {A}_{\theta },\vec {A}_{\phi }) =\, \left( \begin{array}{ccc}\beta &{}\quad 0&{}\quad \cos \theta \\ 0&{}\quad \beta \sinh \eta &{}\quad -\sin \theta \cosh \eta \\ 0&{}\quad -\cosh \eta &{}\quad \beta \sinh \eta \sin \theta \end{array} \right) \,. \end{aligned}$$

(A6)

Now we would like to compute the holonomy around a hyperbolic triangle. We first choose a first vertex, A, of the triangle as the origin of our coordinate system. And using the invariance of the triad and connection under rotation, we place the whole triangle in the equatorial plane at $z=0$, by fixing the angle $\theta =\frac{\pi }{2}$, and further put the point B on the x-axis (at $\phi =0$).

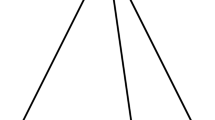
The subtlety about spherical coordinates is the conical-like defect at the origin. To address this issue, we need to regularize our holonomies. As illustrated on Fig. 13, we introduce two shifts of the point A along the geodesics (AB) and (AC). The holonomy around the hyperbolic triangle is then defined as the holonomy along the loop $(A'BCA'')$ in the limit where we send the shifted points $A'$ and $A''$ back to A.

Fig. 13

The hyperbolic triangle. a Each angle of the hyperbolic triangle is noted with respect to the corresponding vertex, while the length along each edge is given by the boost parameter $\eta$. b We regularize the holonomy around the triangle by introducing the shifted points $A'$ and $A''$ along the geodesics (AB) and (AC) in order to avoid the conical singularity at the origin A of the spherical coordinate system

The holonomies h of the Ashtekar–Barbero connection A along the geodesics starting at the origin are simply computed by integration:

$$\begin{aligned} h_{AB} = e^{i\int _{0}^{\eta _{AB}} \mathrm {d}\eta \, \vec {A}_{\eta }\cdot \frac{\vec {\sigma }}{2}} =e^{i\beta \eta _{AB}\frac{\sigma _{1}}{2}} =\left( \begin{array}{cc}\cos \frac{\beta \eta _{AB}}{2}&{} i\sin \frac{\beta \eta _{AB}}{2}\\ i\sin \frac{\beta \eta _{AB}}{2}&{}\cos \frac{\beta \eta _{AB}}{2}\end{array} \right) \,\equiv L^x_{\beta \eta _{AB}} \,. \end{aligned}$$

(A7)

where we introduce the notation $L^x$ for the Lorentz transformations generated by the Pauli matrix $\sigma _{1}$ for arbitrary complex exponentiation parameter. The holonomies of the spin-connection $\Gamma$ corresponds to the value $\beta =0$ and are trivial along those geodesics as expected (since $\vec {\Gamma }_{\eta }=0$):

$$\begin{aligned} g_{AB}=h_{AB}^{(\beta =0)}=\mathbb {I}\,. \end{aligned}$$

(A8)

Similarly it is easy to compute the holonomies along the infinitesimal arc $(A'A'')$ (in the equatorial plane):

$$\begin{aligned} h_{A'A''}=e^{i\int _{0}^{\hat{a}} \mathrm {d}\phi \,\vec {A_{\phi }}\cdot \frac{\vec {\sigma }}{2}} \,\,\underset{A',A''\rightarrow A}{=}\, e^{i\,\hat{a}\frac{\sigma _{3}}{2}} \,\equiv R^z_{\hat{a}}\,, \end{aligned}$$

(A9)

where we introduce the notation $R^z$ for $\mathrm {SU}(2)$ transformations generated by $\sigma _{3}$. Here, we point out that we chose to associate the Pauli matrices to the tangent directions on the internal space as $\vec {\sigma }=(\sigma _{1},-\sigma _{3},\sigma _{2})$ in order to keep the intuition of the z-direction as normal to the triangle.

In order to compute the holonomy $h_{BC}$ along the edge (BC), we change coordinate system and choose new spherical coordinates centered on the origin C. Since the hyperboloid is homogeneous, the new triad will be equal to the initial triad by a simple $\mathrm {SU}(2)$-gauge transformation. Similarly, the new holonomies of the spin-connection and of the Ashtekar–Barbero connections will be obtained from the initial holonomies by the same gauge transformation. Let us look at the holonomies of the spin-connection, writing g’s for the initial holonomies in the coordinate system with A as origin and $\tilde{g}$ for the new holonomies with C as origin.

To make everything work without singularity, we need to introduce shifts $C'$ and $C''$ of the vertex C along the edges of the triangle. The original holonomies are:

$$\begin{aligned} \left\{ \begin{array}{ccl} g_{A'B}&{}=&{}\mathbb {I}\\ g_{BC'}&{}=&{}R^z_{\pi -\hat{b}-\hat{c}}\\ g_{C'C''}&{}=&{}\mathbb {I}\\ g_{C''A''}&{}=&{}\mathbb {I}\\ g_{A''A'}&{}=&{}R^z_{-\hat{a}} \end{array} \right. \,, \end{aligned}$$

(A10)

so that the overall holonomy around the triangle $g_{ABC}=g_{A''A'}g_{C''A''}g_{C'C''}g_{BC'}g_{A'B}=R^z_{\varphi }$ reproduces the deficit angle $\varphi \equiv \pi -\hat{a}-\hat{b}-\hat{c}$ as already known [32]. The new holonomies using C as origin should result from those by a $\mathrm {SU}(2)$ gauge-transformation $k_{v}$ for all points v:

$$\begin{aligned} \left\{ \begin{array}{ccccl} \tilde{g}_{A'B}&{}=&{}k_{B}^{-1}g_{A'B}k_{A'}&{}=&{} R^z_{\pi -\hat{a}-\hat{b}}\\ \tilde{g}_{BC'}&{}=&{}k_{C'}^{-1}g_{BC'}k_{B}&{}=&{}\mathbb {I}\\ \tilde{g}_{C'C''}&{}=&{}k_{C''}^{-1}g_{C'C''}k_{C'}&{}=&{}R^z_{-\hat{c}}\\ \tilde{g}_{C''A''}&{}=&{}k_{A''}^{-1}g_{C''A''}k_{C''}&{}=&{}\mathbb {I}\\ \tilde{g}_{A''A'}&{}=&{}k_{A'}^{-1}g_{A''A'}k_{A''}&{}=&{}\mathbb {I}\\ \end{array} \right. \,, \end{aligned}$$

(A11)

This first implies that all the k’s are also rotations around the z-axis. Setting $k_{A'}=R^z_{\psi }$, we easily solve the system to:

$$\begin{aligned} k_{B}=R^z_{\psi +\hat{a}+\hat{b}-\pi }\,,\quad k_{C'}=R^z_{\psi +\hat{a}-\hat{c}}\,,\quad k_{C''}=k_{A''}=R^z_{\psi +\hat{a}}\,. \end{aligned}$$

(A12)

One way to determine the angle $\psi$ is to work out the explicit coordinate change. By a simple look at the triangle geometry, as shown on Fig. 14, we easily get $\psi =\pi -\hat{a}$, giving the gauge transformation:

$$\begin{aligned} k_{B}=R^z_{\hat{b}}\,,\quad k_{C'}=R^z_{\pi -\hat{c}}\,,\quad k_{C''}=k_{A''}=R^z_{\pi }\,. \end{aligned}$$

(A13)

Fig. 14

Illustration of the rotation of the coordinates when we choose C as the reference point in our coordinates

Applying this gauge transformation to the holonomies of the Ashtekar–Barbero connection, we get:

$$\begin{aligned} \tilde{h}_{BC} =k_{C'}^{-1} h _{BC} k_{B} \quad \Longrightarrow \quad h_{BC}=k_{C'} \tilde{h}_{BC} k_{B}^{-1} = R^z_{\pi -\hat{c}}\,L^x_{-\beta \eta _{BC}}\,R^z_{-\hat{b}}.\quad \end{aligned}$$

(A14)

Putting all the pieces together, we finally get the holonomy around the hyperbolic triangle:

$$\begin{aligned} h_{ABC}=h_{A''A'}h_{CA''}h_{BC}h_{A'B} = R^z_{-\hat{a}}\,L^x_{-\beta \eta _{AC}}\,R^z_{\pi -\hat{c}}\,L^x_{-\beta \eta _{BC}}\,R^z_{-\hat{b}}\,L^x_{\beta \eta _{AB}}.\qquad \end{aligned}$$

(A15)

Using that $L^x_{-\eta }=R^z_{-\pi }L^x_{+\eta }R^z_{+\pi }$, it is convenient to re-organize this expression in a more symmetrical fashion to clarify its geometrical interpretation:

$$\begin{aligned}&h_{ABC}= R^z_{\varphi }\, \Big (\big (R^z_{\pi -\hat{c}}R^z_{\pi -\hat{b}}\big )^{-1}\,L^x_{\beta \eta _{AC}}\,\big (R^z_{\pi -\hat{c}}R^z_{\pi -\hat{b}}\big )\Big )\nonumber \\&\quad \times \Big (\big (R^z_{\pi -\hat{b}}\big )^{-1}\,L^x_{\beta \eta _{BC}}\,\big (R^z_{\pi -\hat{b}}\big )\Big ) \,L^x_{\beta \eta _{AB}}\,. \end{aligned}$$

(A16)

The first $\mathrm {SU}(2)$ group element $R^z_{\varphi }$ is the rotation around the normal direction to the triangle with angle given by the deficit angle $\varphi =\pi -\hat{a}-\hat{b}-\hat{c}$. This is exactly the holonomy $g_{ABC}$ of the spin-connection $\Gamma ^{i}_{a}$ around the triangle. Then the three following terms are the pure boosts along the edges of the hyperbolic triangle, respectively (CA), (BC) and finally (AB). Moving back to an arbitrary hyperbolic triangle (not necessarily in the equatorial plane), this structure directly generalizes to a rather simple formula:

$$\begin{aligned} h_{ABC}\, =\, R B_{CA}^{\mathrm {i}\beta } B_{BC}^{\mathrm {i}\beta } B_{AB}^{\mathrm {i}\beta }\,. \end{aligned}$$

(A17)

The pure boost $B_{AB}\in \mathrm {SH}_{2}({\mathbb C})$ maps the vertex A to the vertex B, and so on around the triangle. The rotation $R\in \mathrm {SU}(2)$ is the holonomy of the spin-connection around the triangle. It enters the “almost-closure” relation of boosts around the triangle:

$$\begin{aligned} R=B_{AB}B_{BC}B_{CA}=B_{BA}B_{CB}B_{AC}\,, \end{aligned}$$

(A18)

where the second equality holds by taking the inverse and self-adjoint. Finally the complex exponentiation for a pure boost B must be understood as a quick hand notation for:

$$\begin{aligned} B^{\alpha }=\left( \exp \left( \frac{\eta }{2} \hat{u} \cdot \overrightarrow{\sigma }\right) \right) ^{\alpha } = \exp \left( \frac{\alpha \eta }{2} \hat{u} \cdot \overrightarrow{\sigma }\right) \,,\quad \forall \eta \in {\mathbb R}\,,\,\,\alpha \in {\mathbb C}\,,\,\,\hat{u}\in {\mathcal S}^{2},\quad \end{aligned}$$

(A19)

which is strictly defined only for boosts, though $\alpha$ can be complex.

The case $\beta =0$ clearly reduces the Ashtekar–Barbero holonomies to those of the original spin-connection. The cases $\beta =\pm i$ correspond to the self-dual and anti-self-dual Lorentz connection, which are flat, and give as expected $h_{ABC}^{(\beta =i)}=h_{ABC}^{(\beta =-i)}=\mathbb {I}$.

1.2 2. Triad and holonomies in the Cartesian coordinate system

We could use the Cartesian coordinates (x, y, z) instead of spherical coordinates to parametrize the hyperboloid. In that case, the induced 3d metric is not diagonal anymore:

$$\begin{aligned} q= & {} \frac{1}{\kappa ^{2}+\vec {x}^{2}}\,\left( \begin{array}{ccc}\kappa ^{2}+y^{2}+z^{2} &{} -xy&{} -xz \\ -xy &{} \kappa ^{2}+x^{2}+z^{2} &{} -yz \\ -xz &{} -yz &{} \kappa ^{2}+x^{2}+y^{2}\end{array} \right) \,\,,\nonumber \\ q_{ab}= & {} \delta _{ab}-\frac{1}{\kappa ^{2}+\vec {x}^{2}}x_{a}x_{b}\,. \end{aligned}$$

(A20)

The triad $e^{i}_{a}$ can be expressed as a cross-product:

$$\begin{aligned} e^{i}_{a}=\frac{1}{\sqrt{\kappa ^{2}+\vec {x}^{2}}}\,\big (\kappa \delta ^{i}_{a}-\epsilon ^{iab}x_{b}\big )\,,\quad q_{ab}=e^{i}_{a}e^{i}_{b}\,. \end{aligned}$$

(A21)

The inverse triad is similarly computed:

$$\begin{aligned} e^{a}_{i}=\frac{\kappa }{\sqrt{\kappa ^{2}+\vec {x}^{2}}}\,\big ( \delta ^{a}_{i}+\frac{x_{a}x_{i}}{\kappa ^{2}}-\epsilon ^{iab}\frac{x_{b}}{\kappa } \big )\,. \end{aligned}$$

(A22)

This allows to compute the spin-connection:

$$\begin{aligned} \Gamma ^{i}_{a}=\frac{1}{\kappa }\Big (\delta ^{i}_{a}-\frac{x_{i}x_{a}}{\kappa ^{2}+\vec {x}^{2}}\Big )\,. \end{aligned}$$

(A23)

From here, we can easily compute holonomies of the Ashtekar–Barbero connection $A^{i}_{a}=\Gamma ^{i}_{a}+\beta e^{i}_{a}/\kappa$ along geodesics starting at the origin and see that they go along the direction of the geodesic itself.

Appendix B: About the reconstruction of the tetrahedra: the hyperbolic bidual

In order to reconstruct the hyperbolic tetrahedron from the $\mathrm {SB}(2,{\mathbb C})$-normals to its triangles, we try to adapt the bi-dual tetrahedron construction of the flat case where a dual tetrahedron is constructed with the dual vertices defined as the normals of the initial tetrahedron. In that case, we know that taking the dual twice leads back to the initial tetrahedron up to a global re-scaling (by the tetrahedron volume).

So let us start with a hyperbolic tetrahedron. We place one of its vertices at the hyperboloid origin. The other three vertices are defined by three pure boosts (in $\mathrm {SH}_{2}({\mathbb C})$), or equivalently by three group elements in $\mathrm {SB}(2,{\mathbb C})\sim \mathrm {SL}(2,{\mathbb C})\mathrm {SU}(2)$. Following our definitions, we choose a complex value of the Immirzi parameter $\beta$ and compute the $\mathrm {SL}(2,{\mathbb C})$ holonomies $\Lambda _{i}$ of the Ashtekar–Barbero connection around the four hyperbolic triangles. These holonomies satisfy a $\mathrm {SL}(2,{\mathbb C})$ closure constraint, $\Lambda _{D}\Lambda _{C}\Lambda _{B}\Lambda _{A}=\mathbb {I}$. Finally taking their Iwasawa decompositions leads to the $\mathrm {SB}(2,{\mathbb C})$-normals to the hyperbolic triangles, which also satisfy a $\mathrm {SB}(2,{\mathbb C})$ closure relation:

$$\begin{aligned} L_D L_C L_B L_A = \mathbb {I}\,. \end{aligned}$$

(B1)

We can now use these four new $\mathrm {SB}(2,\mathbb {C})$ group elements as the Lorentz boosts defining the vertices of a new tetrahedron, as $L_{A}L_{A}^{\dagger }$ and so on. Unfortunately, this definition would not behave properly under 3d rotations. Indeed, due to the non-abelian nature $\mathrm {SB}(2,{\mathbb C})$ and the twisting induced by the Iwasawa decomposition, we know that the four $\mathrm {SB}(2,{\mathbb C})$-normals don’t transform with the same $\mathrm {SU}(2)$ transformation under rotations and the correct transformation law was earlier given in (54):

$$\begin{aligned} \left| \begin{array}{lcl} L_{D}&{}\rightarrow &{}k L_{D}k_{D}^{-1}\\ L_{C}&{}\rightarrow &{}k_{D} L_{C}k_{C}^{-1}\\ L_{B}&{}\rightarrow &{}k_{C} L_{B}k_{B}^{-1}\\ L_{A}&{}\rightarrow &{}k_{B} L_{A}k^{-1}\\ \end{array} \right. \qquad \left| \begin{array}{lcl} H_{D}&{}\rightarrow &{}k_{D} H_{D}k^{-1}\\ H_{C}&{}\rightarrow &{}k_{C} H_{C}k_{D}^{-1}\\ H_{B}&{}\rightarrow &{}k_{B} H_{B}k_{C}^{-1}\\ H_{A}&{}\rightarrow &{}k H_{A}k_{B}^{-1}\\ \end{array} \right. \,, \end{aligned}$$

where we recall that the L’s and H’s were both derived from the $\mathrm {SL}(2,{\mathbb C})$-holonomies as:

$$\begin{aligned} \left\{ \begin{array}{ll} \Lambda _D = L_D H_D \\ \left( H_D\right) \Lambda _C \left( H_D\right) ^{-1} = L_C H_C \\ \left( H_C H_D\right) \Lambda _B \left( H_C H_D\right) ^{-1} = L_B H_B \\ \left( H_B H_C H_D\right) \Lambda _A \left( H_B H_C H_D\right) ^{-1} = L_A H_A \end{array}\right. \end{aligned}$$

So under a 3d rotation, the dual vertex $L_{D}L_{D}^{\dagger }$ would get conjugated by the $\mathrm {SU}(2)$ group element k while the dual vertex $L_{C}L_{C}^\dagger$ would get conjugated by $k_{D}$. The way out is to use the H’s to compensate the twist in the action of rotations. This actually amounts to coming back to the original $\mathrm {SL}(2,{\mathbb C})$ holonomies. Indeed, these follow simple homogeneous law under rotation:

$$\begin{aligned} \Lambda _{i}\,\longrightarrow \, k\Lambda _{i}k^{-1}\,. \end{aligned}$$

(B2)

In the end, our dual hyperbolic tetrahedron has its four dual vertices defined as:

$$\begin{aligned} \left| \begin{array}{l} \Lambda _{D}\Lambda _{D}^{\dagger }=L_{D}L_{D}^{\dagger }\\ \Lambda _{C}\Lambda _{C}^{\dagger }=H_{D}^{-1}\,(L_{C}L_{C}^{\dagger })\,H_{D}\\ \Lambda _{B}\Lambda _{B}^{\dagger }=(H_{C}H_{D})^{-1}\,(L_{B}L_{B}^{\dagger })\,(H_{C}H_{D})\\ \Lambda _{A}\Lambda _{A}^{\dagger }=(H_{B}H_{C}H_{D})^{-1}\,(L_{A}L_{A}^{\dagger })\,(H_{B}H_{C}H_{D}). \end{array} \right. \end{aligned}$$

(B3)

Finally, we can always translate back the first vertex to the hyperboloid origin¹², considering the translated dual vertices defined as $\mathbb {I}$, $\Lambda _{D}^{-1}\Lambda _{C}\Lambda _{C}^{\dagger }\Lambda _{D}^{-1\dagger }$, $\Lambda _{D}^{-1}\Lambda _{B}\Lambda _{B}^{\dagger }\Lambda _{D}^{-1}$ and $\Lambda _{D}^{-1}\Lambda _{A}\Lambda _{A}^{\dagger }\Lambda _{D}^{-1}$. Let us point out that we could also decide to translate using the Borel group element $L_{D}$ instead of the full Lorentz transformation $\Lambda _{D}$. This stills translates the first vertex back to the origin and is more consistent with the logic of distinguishing the 3d rotations from 3d translations on the hyperboloid by selecting a proper section of the coset $\mathrm {SL}(2,{\mathbb C})/\mathrm {SU}(2)$ and choosing the Borel group $\mathrm {SB}(2,{\mathbb C})$ as defining the hyperbolic translations. The resulting translated dual tetrahedra only differ by an overall rotation (by $H_{D}$), so we should keep in mind that we might recover the initial tetrahedron only up to a global rotation through this bi-dual procedure.

We are now ready to take the dual of the dual tetrahedron. We give ourself the freedom to choose a different Immirzi parameter $\tilde{\beta }$ for computing the $\mathrm {SL}(2,{\mathbb C})$-holonomies around the dual triangles and defining the $\mathrm {SB}(2,{\mathbb C})$-normals to the dual tetrahedron. In this scheme, we can potentially adjust the new Immirzi parameter $\tilde{\beta }$ in function of the initial value $\beta$ in order to recover the initial hyperbolic tetrahedron.

We have worked out preliminary numerical simulations focusing on the simplified case of a square tetrahedron: the three edges meeting at the root vertex are orthogonal to each other and furthermore of equal length. Such a tetrahedron is defined entirely by the value of a single edge length. Indeed, once the length of the three edges meeting at the right angles is given, the length of the remaining three edges is fixed.

As displayed on Fig. 15, we first checked that the bidual tetrahedron of such a square hyperbolic tetrahedron is again a square tetrahedron. We plotted the six lengths and checked that indeed they group into two lengths: the length of the edges meeting at the root vertex and the length of the opposite edges. This by itself is not enough to ensure that the angles at the root vertex are right again. Therefore, we further plotted the two lengths against each other and compared to the curve expected for a square tetrahedron and the match seems perfect. This provides a numerical check that the bidual of a square tetrahedron is again a square tetrahedron.

Fig. 15

Numerical analysis of the bidual of the tetrahedron in order to check that it is indeed also square. The original tetrahedron is a square tetrahedron of edge length 0.2. The dual is constructed with Immirzi parameter $\cosh (1)+\mathrm {i}\sinh (1)$ and the bidual with Immirzi parameter $\cosh (\eta )+\mathrm {i}\sinh (\eta )$. This illustrates the fact that the bidual is still square and therefore the reconstruction procedure, in this peculiar case, can be tested using only one edge. a We plot the lengths of the edges of the bidual of a square tetrahedron with edge length 0.2 at the square. The original Immirzi parameter is taken to be $\cosh (1)+\mathrm {i}\sinh (1)$ for the construction of the dual and the bidual is constructed using an Immirzi parameter of the form $\cosh (\eta )+\mathrm {i}\sinh (\eta )$. The six lengths do indeed group into two different lengths: the length of the edges meeting at the square angle and the length of the opposite edges. b We plot the two lengths of the edges of the bidual of a square tetrahedron with edge length 0.2 at the square against each other. The original Immirzi parameter is taken to be $\cosh (1)+\mathrm {i}\sinh (1)$ for the construction of the dual and the bidual is constructed using an Immirzi parameter of the form $\cosh (\eta )+\mathrm {i}\sinh (\eta )$. We also plot the expected relation between the two for a square tetrahedron and find that the curves match perfectly. It seems therefore that the bidual is indeed square

Fig. 16

Numerical analysis of the dual of the tetrahedron in order to understand its shape. The original tetrahedron is a square tetrahedron of edge length 0.2. The dual is constructed with Immirzi parameter $\cosh (\eta )+\mathrm {i}\sinh (\eta )$. Though the dual is not square, it seems to retain some symmetry in the grouping of the edges lengths. a We plot the lengths of the edges of the dual of a square tetrahedron with edge length 0.2 at the square. The Immirzi parameter is taken to be $\cosh (\eta )+\mathrm {i}\sinh (\eta )$ for the construction. The six lengths do indeed group into two different lengths as for the bidual. b We plot the two lengths of the edges of the dual of a square tetrahedron with edge length 0.2 at the square against each other. The Immirzi parameter is taken to be $\cosh (\eta )+\mathrm {i}\sinh (\eta )$. We also plot the expected relation between the two for a square tetrahedron and find that the curves do not match at all. It seems therefore that the dual is not square

We also checked the shape of the dual of a square tetrahedron (Fig. 16). In the flat space case, the dual tetrahedron would be square too, but this is actually not the case in the hyperbolic case. The six lengths are indeed still grouped into two groups, reflecting some preservation of the symmetry, but these two lengths do not have the correct relations between themselves and therefore the dual is not a square tetrahedron. In this context, it is easy to proceed to check whether the bidual tetrahedron will match or not with the initial square tetrahedron: we fix the initial edge length (here 0,2 in our numerical simulations) and we vary the Immirzi parameter until the edge length of the bidual matches that initial edge length. We plotted the difference of the initial edge length with the bidual edge length in Fig. 17, using two different ansatz for the Immirzi parameter: for an Immirzi parameter proportional to the initial one and for a (complex) cosmological constant proportional to the initial one. Both schemes seem to be able to reconstruct the original tetrahedron. This was to be expected as we have an $\mathrm {SU}(2)$ freedom on each normal which does not change the reconstruction. Therefore we expect that a (one-real-parameter) family of Immirzi parameters should work and allow the reconstruction.

These preliminary numerical simulations are very promising but they are not yet conclusive. They should be studied in the more general setting of an arbitrary tetrahedron and we believe this line of research worth investigating further.

Fig. 17

Numerical analysis of the reconstruction procedure of a tetrahedron via the bidual. The original tetrahedron is a square tetrahedron of edge length 0.2. The dual is constructed with Immirzi parameter $\cosh (1)+\mathrm {i}\sinh (1)$. The bidual is constructed using two different schemes as explained in the captions. The plots represent the difference between the caracterizing length of the bidual and that of the original tetrahedron. In both cases, the curves cross the zero-line signaling the success of the procedure, at least in the square tetrahedron case. a We plot the difference between the length of one of the edge at the corner of the bidual of a square tetrahedron with edge length 0.2 at the square and that of the original tetrahedron. The original Immirzi parameter is taken to be $\cosh (1)+\mathrm {i}\sinh (1)$ for the construction of the dual. The bidual is constructed using an Immirzi parameter of the form $\cosh (\eta )+\mathrm {i}\sinh (\eta )$. The difference does cross the zero-line, signaling that the reconstruction procedure works at least in the case of a square tetrahedron. b We plot the difference between the length of one of the edge at the corner of the bidual of a square tetrahedron with edge length 0.2 at the square and that of the original tetrahedron. The original Immirzi parameter is taken to be $\cosh (1)+\mathrm {i}\sinh (1)$ for the construction of the dual. The bidual is constructed using an Immirzi parameter of the form $\eta (\cosh (1)+\mathrm {i}\sinh (1))$. The difference does cross the zero-line, signaling that the reconstruction procedure works at least in the case of a square tetrahedron