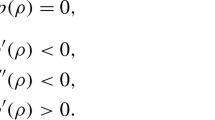Skip to main content
SpringerLink
Log in

Developing an Aw–Rascle model of traffic flow

  • Published:
Journal of Engineering Mathematics Aims and scope Submit manuscript

Abstract

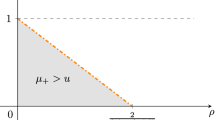
A traffic flow model is established based on the “car following” principle with a maximal constraint on the density–velocity relationship. The model develops the Aw–Rascle model and amends some “nonphysical” features. Moreover, we construct the solutions of the Riemann problem for the model. The Riemann solutions provide a more reasonable invariant region and show the phase-transition phenomena.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17

Similar content being viewed by others

References

  1. Aw A, Rascle M (2000) Resurrection of “second order” models of traffic flow. SIAM J Appl Math 60(3):916–938

    Article  MathSciNet  MATH  Google Scholar 

  2. Aw A, Klar A, Materne T, Rascle M (2002) Derivation of continuum traffic flow models from microscopic follow-the-leader models. SIAM J Appl Math 63(1):259–278

    Article  MathSciNet  MATH  Google Scholar 

  3. Gazis C, Edie C (1968) Traffic flow theory. Proc IEEE 56(4):458–471

    Article  Google Scholar 

  4. Immers LH, Logghe S (2002) Traffic flow theory, vol 40. Faculty of Engineering, Department of Civil Engineering, Section Traffic and Infrastructure, Kasteelpark Arenberg

    Google Scholar 

  5. Berthelin F, Degond P, Delitala M, Rascle M (2008) A model for the formation and evolution of traffic jams. Arch Ration Mech Anal 187(2):185–220

    Article  MathSciNet  MATH  Google Scholar 

  6. Berthelin F, Degond P, Blanc V (2008) A traffic-flow model with constraints for the modeling of traffic jams. Math Models Methods Appl Sci 18(supp01):1269–1298

    Article  MathSciNet  MATH  Google Scholar 

  7. Kerner BS, Rehborn H (1997) Experimental properties of phase transitions in traffic flow. Phys Rev Lett 79(20):4030–4033

    Article  ADS  Google Scholar 

  8. Kerner BS (2000) Phase transitions in traffic flow. Springer, Berlin

    Book  MATH  Google Scholar 

  9. Payne HJ (1971) Models of freeway traffic and control, Mathematical models of public systems

  10. Daganzo F (1995) Requiem for second-order fluid approximations of traffic flow. Transport Res B Methodol 29(4):277–286

    Article  Google Scholar 

  11. Degond P, Delitala M (2008) Modelling and simulation of vehicular traffic jam formation. Kinet Relat Models 1(2):279–293

    Article  MathSciNet  MATH  Google Scholar 

  12. Bellomo N, Dogbe C (2011) On the modeling of traffic and crowds: a survey of models, speculations, and perspectives. SIAM Rev 53(3):409–463

    Article  MathSciNet  MATH  Google Scholar 

  13. Sun M (2009) Interactions of elementary waves for the Aw–Rascle model. SIAM J Appl Math 69(6):1542–1558

    Article  MathSciNet  MATH  Google Scholar 

  14. Tveito A, Winther R (1991) Existence, uniqueness, and continuous dependence for a system of hyperbolic conservation laws modeling polymer flooding. SIAM J Appl Math 22(4):905–933

    Article  MathSciNet  MATH  Google Scholar 

  15. Godvik M, HancheOlsen H (2008) Existence of solutions for the Aw–Rascle traffic flow model with vacuum. J Hyperbol Differ Eq 5(01):45–63

    Article  MathSciNet  MATH  Google Scholar 

  16. Herty M, Rascle M (2006) Coupling conditions for a class of second-order models for traffic flow. SIAM J Appl Math 38(2):595–616

    Article  MathSciNet  MATH  Google Scholar 

  17. Gerlough L, Huber J (1975) Traffic flow theory, Special Report 165, Transportation Research Board, National Research Council, Washington, DC

  18. Zhang HM (2002) A non-equilibrium traffic model devoid of gas-like behavior. Transport. Res. B-Meth. 36(3):275–290

    Article  Google Scholar 

  19. Bellomo N, Bellouquid A, Nieto J, Soler J (2014) On the multiscale modeling of vehicular traffic: from kinetic to hydrodynamics. Discrete Contin Dyn Syst Series B 19(7):1869–1888

    Article  MathSciNet  MATH  Google Scholar 

  20. Smoller J (1983) Shock waves and reaction–diffusion equations. Springer, New York

  21. Freisthler H (1992) Dynamical stability and vanishing viscosity: a case study of a non-strictly hyperbolic system. Commun Pur Appl Math 45(5):561–582

    Article  MathSciNet  Google Scholar 

  22. Bellouquid A, De Angelis E, Fermo L (2012) Towards the modeling of vehicular traffic as a complex system: a kinetic theory approach. Math Models Methods Appl Sci 22(supp01):1140003

    Article  MathSciNet  MATH  Google Scholar 

  23. Dolfin M (2012) From vehicle–driver behaviors to first order traffic flow macroscopic models. Appl Math Lett 25(12):2162–2167

  24. Xiao L, Tong Z (1978) The Riemann problem of non-convex Quasilinear hyperbolic systems. Chin Sci Bull 8:003

    Google Scholar 

Download references

Acknowledgments

Supported by the National Natural Science Foundation of China (11171340).

Author information

Authors and Affiliations

  1. Wuhan Institute of Physics and Mathematics, Chinese Academy of Sciences, Wuhan, 430071, China

    Wei-Feng Jiang & Zhen Wang

Authors
  1. Wei-Feng Jiang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zhen Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Wei-Feng Jiang.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Jiang, WF., Wang, Z. Developing an Aw–Rascle model of traffic flow. J Eng Math 97, 135–146 (2016). https://doi.org/10.1007/s10665-015-9801-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10665-015-9801-2

Keywords

Search

Navigation