Skip to main content
SpringerLink
Log in

Onset conditions for a Rayleigh–Taylor instability with step function density profiles

  • Published:
Journal of Engineering Mathematics Aims and scope Submit manuscript

Abstract

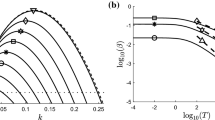
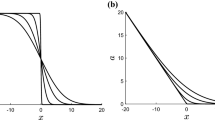
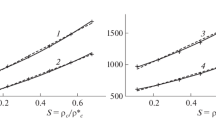
The stability of several density profiles constructed from a linear combination of step functions in a vertically orientated two-dimensional porous medium are considered. A quasi-steady-state approximation is made so that the initial stability of the system can be approximated. Using a similar approach to that of Tan and Homsy (Phys Fluids 29:3549–3556, 1986) a dispersion equation for each density profile is obtained analytically at time zero. Neutral stability curves are then obtained to allow the regions of the parameter space to be divided into stable and unstable regions.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  1. Lord Rayleigh (1883) Investigation of the character of the equilibrium of an incompressible heavy fluid of variable density. Proc Lond Math Soc 14:170–177

  2. Taylor GI (1950) The instability of liquid surfaces when accelerated in a direction perpendicular to their planes. Proc R Soc Lond A 201:192–196

    Article  ADS  MATH  Google Scholar 

  3. Sharp DH (1984) An overview of Rayleigh–Taylor instability. Phys D 12:3–18

    Article  MATH  Google Scholar 

  4. Cabot WH, Cook AW (2006) Reynolds number effects on Rayleigh–Taylor instability with possible implications for type-Ia supernovae. Nat Phys 2:562–568

    Article  Google Scholar 

  5. Conrad CP, Molnar P (1997) The growth of Rayleigh–Taylor-type instabilities in the lithosphere for various rheological and density structures. Geophys J Int 129:95–112

    Article  ADS  Google Scholar 

  6. Citri O, Kagan ML, Kosloff R, Avnir D (1990) The evolution of chemically induced unstable density gradients near horizontal reactive interfaces. Langmuir 6:559–564

    Article  Google Scholar 

  7. Stommel H, Arons AB, Blanchard D (1956) An oceanographic curiosity: the perpetual salt fountain. Deep-Sea Res 3:152–152

    Article  ADS  Google Scholar 

  8. Stern ME (1960) The salt-fountain and thermohaline convection. Tellus 12:172–177

    Article  ADS  Google Scholar 

  9. Huppert HE, Mannis PC (1973) Limiting conditions for salt-fingering at an interface. Deep-Sea Res 20:315–323

    Google Scholar 

  10. Trevelyan PMJ, Almarcha C, De Wit A (2011) Buoyancy-driven instabilities of miscible two-layer stratifications in porous media and Hele–Shaw cells. J Fluid Mech 670:38–65

    Article  ADS  MATH  MathSciNet  Google Scholar 

  11. D’Hernoncourt J, Zebib A, De Wit A (2007) On the classification of buoyancy-driven chemo-hydrodynamic instabilities of chemical fronts. Chaos 17:013109

    Article  ADS  Google Scholar 

  12. Saffman PG, Taylor G (1958) The penetration of a fluid into a medium or Hele–Shaw cell containing a more viscous liquid. Proc Soc Lond A 245:312–329

    Article  ADS  MATH  MathSciNet  Google Scholar 

  13. Homsy GM (1987) Viscous fingering in porous media. Ann Rev Fluid Mech 19:271–311

    Article  ADS  Google Scholar 

  14. Pritchard D (2009) The linear stability of double-diffusive miscible rectilinear displacements in a Hele–Shaw cell. Eur J Mech B 28:564–577

    Article  MATH  MathSciNet  Google Scholar 

  15. Mishra M, Trevelyan PMJ, Almarcha C, De Wit A (2010) Influence of double diffusive effects on miscible viscous fingering. Phys Rev Lett 105:204501

    Article  ADS  Google Scholar 

  16. Hickernell FJ, Yortsos YC (1986) Linear stability of miscible displacement processes in porous media in the absence of dispersion. Stud Appl Math 74:93–115

    MATH  MathSciNet  Google Scholar 

  17. Manickam O, Homsy GM (1993) Stability of miscible displacements in porous media with non-monotonic viscosity profiles. Phys Fluids A 5:1356–1367

    Article  ADS  MATH  Google Scholar 

  18. Manickam O, Homsy GM (1994) Simulation of viscous fingering in miscible displacements with non-monotonic viscosity profiles. Phys Fluids 9:95–107

    Article  ADS  Google Scholar 

  19. Tan CT, Homsy GM (1986) Stability of miscible displacements in porous media: rectilinear flow. Phys Fluids 29:3549–3556

    Article  ADS  MATH  Google Scholar 

  20. Daripa P (2008) Studies on stability in three-layer Hele–Shaw flows. Phys Fluids 20:112101

    Article  ADS  Google Scholar 

  21. Ben Y, Demekhin EA, Chang HC (2002) A spectral theory for small amplitude miscible fingering. Phys Fluids 14:999–1010

    Article  ADS  MATH  MathSciNet  Google Scholar 

  22. Kim MC (2012) Linear stability analysis on the onset of the viscous fingering of a slice in a porous medium. Adv Water Res 35:1–9

    Article  Google Scholar 

  23. Riaz A, Hesse M, Tchelepi HA, Orr FM (2006) Onset of convection in a gravitationally unstable diffusive boundary layer in porous media. J Fluid Mech 548:87–111

    Article  ADS  MathSciNet  Google Scholar 

  24. Nield DA, Bejan A (2006) Convection in porous media. Springer, New York

    MATH  Google Scholar 

  25. Martin J, Rakotomalala N, Salin D (2002) Gravitational instability of miscible fluids in a Hele–Shaw cell. Phys Fluids 14:902–905

    Article  ADS  Google Scholar 

  26. Cross M, Hohenberg PC (1993) Pattern-formation outside of equilibrium. Rev Mod Phys 65:851–1112

    Article  ADS  Google Scholar 

  27. MAPLE 15 (2011) Waterloo Maple Inc., Waterloo Ontario, Canada

Download references

Acknowledgments

PMJT would like to thank Prof. Anne De Wit for numerous fruitful discussions. Both authors would like to thank all three anonymous referees for their constructive comments and suggestions, which helped to improve this paper.

Author information

Authors and Affiliations

  1. Division of Mathematics & Statistics, University of South Wales, Pontypridd, CF37 1DL, Wales, UK

    Jayna Gandhi & Philip M. J. Trevelyan

Authors
  1. Jayna Gandhi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Philip M. J. Trevelyan
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Philip M. J. Trevelyan.

Appendix

Appendix

The dispersion equation for two separated layers is given by

$$\begin{aligned} 0&= -P_{10} P_{11} Q_{10} Q_{11} \mathrm e ^{W(s+k)+2(L_1+L_2)k} \nonumber \\&+\, R_2^2 k^2 P_{10} P_{11} \left( s - k \mathrm e ^{-L_2(s-k)} \right) ^2 \mathrm e ^{W(s+k)+2L_1 k} \nonumber \\&+\, R_1^2 k^2 Q_{10} Q_{11} \left( s - k \mathrm e ^{-L_1(s-k)} \right) ^2 \mathrm e ^{W(s+k)+2L_2 k} \nonumber \\&+\, R_1 R_2 k^2 P_{10}Q_{11} \left( s - k \mathrm e ^{-W(s-k)} \right) ^2 \mathrm e ^{2(L_1+L_2)k+W(s-k)} \nonumber \\&+\, R_1 R_2 k^4 Q_{00} \left( P_{01} +P_{10} \mathrm e ^{2L_1 s} \right) \mathrm e ^{-(2L_1+2L_2+W)(s-k)} \nonumber \\&+\, R_1 R_2 s^2 k^2 P_{00} \left( Q_{01} -Q_{11} \mathrm e ^{2L_2 k} \right) \mathrm e ^{W(s-k)} \nonumber \\&+\, R_1 R_2 k^4 P_{01} Q_{11} \mathrm e ^{-W(s-k)-2L_1(s-k)+2L_2 k} \nonumber \\&-\, R_1 R_2 s^2 k^2 P_{10} Q_{01} \mathrm e ^{W(s-k)+2L_1 k} \nonumber \\&+\, 2 R_1^2 R_2 s^2 k^4 Q_{11} \left( 1 +\mathrm e ^{W(s-k)-L_1(s-k)} \right) \mathrm e ^{2L_2 k} \nonumber \\&+\, 2 R_1 R_2^2 s^2 k^4 P_{10} \left( 1 + \mathrm e ^{W(s-k)-L_2(s-k)} \right) \mathrm e ^{2L_1 k} \nonumber \\&-\, 2 R_1^2 R_2 s k^5 Q_{11} \left( \mathrm e ^{-L_1(s-k)} + \mathrm e ^{-W(s-k)} \right) \mathrm e ^{2L_2 k -L_1(s-k)} \nonumber \\&-\, 2 R_1 R_2^2 s k^5 P_{10} \left( \mathrm e ^{-L_2(s-k)} + \mathrm e ^{-W(s-k)} \right) \mathrm e ^{2L_1 k -L_2(s-k)} \nonumber \\&+\, 4 R_1 R_2 \sigma s^2 k^3 \left( Q_{11} \mathrm e ^{-L_1 s+L_2 k} - P_{10} \mathrm e ^{-L_2 s+L_1 k} \right) \mathrm e ^{(L_1+L_2)k} \nonumber \\&-\, 2 R_1 R_2 s^2 k^4 \left( R_2 P_{00} \mathrm e ^{-L_2(s-k)} + R_1 Q_{01} \mathrm e ^{-L_1(s-k)} \right) \mathrm e ^{W(s-k)} \nonumber \\&-\, 2 R_1 R_2 s k^5 \left( R_2 P_{01} \mathrm e ^{-L_1(s-k)} + R_1 Q_{00} \mathrm e ^{-L_2(s-k)} \right) \mathrm e ^{-(L_2+L_1+W)(s-k)} \nonumber \\&-\, R_1^2 R_2^2 s^2 k^4 \left( s-2k \mathrm e ^{-L_1(s-k)} \right) \left( s-2k \mathrm e ^{-L_2(s-k)} \right) \mathrm e ^{W(s+k)} \nonumber \\&-\, R_1^2 R_2^2 s^2 k^6 \left( 1 - \mathrm e ^{-W(s+k)} \right) ^2 \left( \mathrm e ^{-2L_1(s-k)} + \mathrm e ^{-2L_2(s-k)} \right) \mathrm e ^{W(s+k)} \nonumber \\&+\, 2 R_1^2 R_2^2 s k^7 \left( \mathrm e ^{-L_1(s-k)} + \mathrm e ^{-L_2(s-k)} \right) \mathrm e ^{W(s+k)-(L_1+L_2)(s-k)} \nonumber \\&-\, R_1^2 R_2^2 k^6 \left( s \mathrm e ^{-W(s+k)} +k \right) ^2 \mathrm e ^{W(s+k)-2(L_1+L_2)(s-k)} \nonumber \\&+\, 4 R_1^2 R_2 \sigma s^2 k^4 \left( s - k \mathrm e ^{-2 L_1(s-k)} \right) \mathrm e ^{-L_2(s-k)} \nonumber \\&-\, 4 R_1 R_2^2 \sigma s^2 k^4 \left( s - k \mathrm e ^{-2 L_2(s-k)} \right) \mathrm e ^{-L_1(s-k)} \nonumber \\&+\, 2 R_1^2 R_2^2 s^2 k^6 \left( 1 - \mathrm e ^{-L_1(s-k)} \right) ^2 \mathrm e ^{-W(s+k)-L_2(s-k)} \nonumber \\&+\, 2 R_1^2 R_2^2 s^2 k^6 \left( 1 + \mathrm e ^{-2L_2(s-k)} \right) \mathrm e ^{-W(s+k)-L_1(s-k)} \nonumber \\&+\, 4 R_1^2 R_2^2 s^2 k^6 \left( \mathrm e ^{-W(s-k)} + \mathrm e ^{W(s-k)} \right) \mathrm e ^{-(L_1+L_2)(s-k)} \nonumber \\&-\, 2 R_1^2 R_2^2 s^3 k^5 +8 R_1 R_2 \sigma ^2 s^3 k^3 \mathrm e ^{-(L_1+L_2)(s-k)} -R_1^2 R_2^2 s^2 k^6 \mathrm e ^{-W(s+k)}, \end{aligned}$$
(40)

where \(P_{nm}=R_1 k(s+(-1)^n k)+(-1)^m 2\sigma s\) and \(Q_{nm}=R_2 k(s+(-1)^n k)+(-1)^m 2\sigma s\) such that \(n\) and \(m\) are either 0 or 1.

The cut-off wavenumber for two separated layers satisfies the following equation:

$$\begin{aligned} 0&= R_2^2 ( R_1^2-16 k^2 ) ( 1+L_2 k )^2 \mathrm e ^{2(W+L_1)k} \nonumber \\&+\, R_1^2 ( R_2^2-16 k^2 ) ( 1+L_1 k )^2 \mathrm e ^{2(W+L_2)k} \nonumber \\&-\, ( R_1^2-16 k^2 ) ( R_2^2-16 k^2 ) \mathrm e ^{2(L_1+L_2+W)k} \nonumber \\&-\, R_1 R_2( R_1+4 k ) ( R_2-4 k ) ( 1+(L_2+W)k)^2 \mathrm e ^{2L_1 k} \nonumber \\&-\, R_1 R_2( R_1+4 k )( R_2-4 k ) ( 1+(L_1+W)k )^2 \mathrm e ^{2L_2 k} \nonumber \\&-\, 2 R_1 R_2^2 L_2 W k^2 ( R_1+4 k ) ( 1+(L_2+W)k) \mathrm e ^{2L_1 k} \nonumber \\&-\, 2 R_1^2 R_2 L_1 W k^2 ( R_2-4 k ) ( 1+(L_1+W)k) \mathrm e ^{2L_2 k} \nonumber \\&-\, R_1^2 R_2^2 ( 1+L_2 k )^2 ( 1+L_1 k )^2 \mathrm e ^{2W k} - R_1^2 R_2^2 L_1^2 L_2^2 k^4 \mathrm e ^{-2W k} \nonumber \\&+\, R_1 R_2 ( R_1+4 k ) ( R_2-4 k ) ( 1+W k )^2 \mathrm e ^{2(L_1+L_2)k} \nonumber \\&+\, R_1 R_2 (R_1+4 k) ( R_2-4 k ) (1+(L_1+L_2+W)k)^2 \nonumber \\&+\, 2 R_1^2 R_2 L_1 k^2 ( L_2+W ) ( R_2-4 k ) (1+(L_1+L_2+W)k) \nonumber \\&+\, 2 R_1^2 R_2^2 L_2 W k^2 ( (L_2+W)k +1) + 2 R_1^2 R_2^2 L_1^2 L_2^2 k^4 \nonumber \\&+\, 4 R_1 R_2^2 L_2 k^3 (2W+2L_1 +R_1 L_1 W) ( 1+(L_1+L_2+W)k ). \end{aligned}$$
(41)

Rights and permissions

Reprints and permissions

About this article

Cite this article

Gandhi, J., Trevelyan, P.M.J. Onset conditions for a Rayleigh–Taylor instability with step function density profiles. J Eng Math 86, 31–48 (2014). https://doi.org/10.1007/s10665-013-9649-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10665-013-9649-2

Keywords

Search

Navigation