Two dimensional river flow and sediment transport model

Abstract

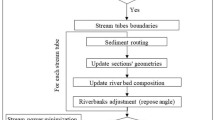
This work presents the development and calibration of a two dimensional river flow, sediment transport and bed evolution model that couples the active-layer and multiple size-class approach for sediment transport modeling with an enhanced advection algorithm. The governing equations are solved using the fractional step method. This resulted in three successive steps (advection, diffusion and continuity) for the flow equations, and two steps (advection and diffusion) for the sediment transport and bed evolution equations. The focus of the research is the improvement of the advection computation (both in water and sediment computation) by reducing the limitation it imposes on the time step. Overcoming this restriction is enabled by introducing modifications to the characteristic method. Implementing these adjustments allows the characteristic curve to extend throughout multiple computational cells. After evaluating the advection computation for both flow and sediment transport by comparison of the proposed algorithm with various classical methods, the developed model is assessed using field measurements conducted on the Danube River. Analysis of the measured and computed values confirmed the developed model’s reliability.

Appendices

Appendix 1: Water flow model

Terms in the diffusion step equation, introduced to enable a more understandable representation of the extensive diffusion equation (2).

$$\begin{aligned} \displaystyle T_{\xi \eta }^{\mathrm{dif}}&= \frac{1}{h_\xi }\,\frac{\partial v}{\partial \xi }-\frac{1}{h_\xi \,h_\eta }\,\left( \frac{\partial h_\xi }{\partial \eta }\,u +\frac{\partial h_\eta }{\partial \xi }\,v\right) +\frac{1}{h_\eta }\,\frac{\partial u}{\partial \eta },\nonumber \\ \displaystyle T_\xi ^{\mathrm{dif}}&= \frac{1}{h_\xi }\,\frac{\partial u}{\partial \xi }+\frac{1}{h_\xi \,h_\eta }\,\frac{\partial h_\xi }{\partial \eta }\,v,\quad T_\eta ^{\mathrm{dif}}=\frac{1}{h_\eta }\,\frac{\partial v}{\partial \eta }+\frac{1}{h_\xi \,h_\eta }\,\frac{\partial h_\eta }{\partial \eta }\,u \end{aligned}$$

(41)

Dispersion terms in the diffusion step equation (2)

$$\begin{aligned} D_u^{\mathrm{disp}}&= \displaystyle -\frac{1}{d\,h_\xi \,h_\eta }\,\left( \frac{\partial }{\partial \xi }\int _{z_b}^{z_s}{h_\eta \,u''\,u''\,dz} -\frac{\partial }{\partial \eta }\int _{z_b}^{z_s}{h_\xi \,u''\,v''\,dz}\right. \nonumber \\&\displaystyle \left. -\frac{\partial h_\xi }{\partial \eta }\,\int _{z_b}^{z_s}{u''\,v''\,dz} +\frac{\partial h_\eta }{\partial \xi }\,\int _{z_b}^{z_s}{v''\,v''\,dz}\right) ,\end{aligned}$$

(42)

$$\begin{aligned} D_v^{\mathrm{disp}}&= \displaystyle -\frac{1}{d\,h_\xi \,h_\eta }\,\left( \frac{\partial }{\partial \xi }\int _{z_b}^{z_s}{h_\eta \,u''\,v''\,dz} -\frac{\partial }{\partial \eta }\int _{z_b}^{z_s}{h_\xi \,v''\,v''\,dz}\right. \nonumber \\&\displaystyle \left. -\frac{\partial h_\eta }{\partial \xi }\,\int _{z_b}^{z_s}{u''\,v''\,dz} +\frac{\partial h_\xi }{\partial \eta }\,\int _{z_b}^{z_s}{u''\,u''\,dz}\right) , \end{aligned}$$

(43)

where upper index $''$ denotes the difference between the actual and depth averaged velocity.

Appendix 2: Sediment transport and bed evolution model

The discretized divergence of the bed-load flux vector in Eqs. (26) and (27).

$$\begin{aligned} x{\mathrm{div}}\left( q_k\right)&= \displaystyle \theta \,\frac{\left[ h_\eta \left( q_\xi \right) _k\right] _{i+\frac{1}{2},j}^{n+1} -\left[ h_\eta \left( q_\xi \right) _k\right] _{i-\frac{1}{2},j}^{n+1}}{\left( h_\xi \right) _{i,j}\,\left( h_\eta \right) _{i,j}}\nonumber \\&\displaystyle +\left( 1-\theta \right) \frac{\left[ h_\eta \left( q_\xi \right) _k\right] _{i+\frac{1}{2},j}^n -\left[ h_\eta \left( q_\xi \right) _k\right] _{i-\frac{1}{2},j}^n}{\left( h_\xi \right) _{i,j}\,\left( h_\eta \right) _{i,j}}\nonumber \\&\displaystyle +\,\,\theta \,\frac{\left[ h_\xi \left( q_\eta \right) _k\right] _{i,j+\frac{1}{2}}^{n+1} -\left[ h_\xi \left( q_\eta \right) _k\right] _{i,j-\frac{1}{2}}^{n+1}}{\left( h_\xi \right) _{i,j}\,\left( h_\eta \right) _{i,j}}\nonumber \\&\displaystyle +\left( 1-\theta \right) \,\frac{\left[ h_\xi \left( q_\eta \right) _k\right] _{i,j+\frac{1}{2}}^n -\left[ h_\xi \left( q_\eta \right) _k\right] _{i,j-\frac{1}{2}}^n}{\left( h_\xi \right) _{i,j}\,\left( h_\eta \right) _{i,j}} \end{aligned}$$

(44)

The dimensionless particle parameter

$$\begin{aligned} \left( D_*\right) _k=D_k\,\left( \frac{\left( \rho _s/\rho -1\right) \,g}{\nu ^2}\right) ^{1/3}, \end{aligned}$$

(45)

and the transport stage parameter

$$\begin{aligned} T_k=\frac{V_*^2-\left( V_*^{\mathrm{cr}}\right) ^2}{\left( V_*^{\mathrm{cr}}\right) ^2}. \end{aligned}$$

(46)

In Eqs. (29) and (37), $\nu$ denotes the kinematic viscosity coefficient, $V_*$ the bed-shear velocity, while $V_*^{\mathrm{cr}}$ stands for the critical bed-shear velocity according to Shields.