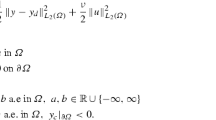Skip to main content
SpringerLink
Log in

A posteriori error estimation and adaptivity for elliptic optimal control problems with state constraints

  • Published:
Computational Optimization and Applications Aims and scope Submit manuscript

Abstract

In this paper optimal control problems governed by elliptic semilinear equations and subject to pointwise state constraints are considered. These problems are discretized using finite element methods and a posteriori error estimates are derived assessing the error with respect to the cost functional. These estimates are used to obtain quantitative information on the discretization error as well as for guiding an adaptive algorithm for local mesh refinement. Numerical examples illustrate the behavior of the method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Becker, R., Kapp, H., Rannacher, R.: Adaptive finite element methods for optimal control of partial differential equations: basic concepts. SIAM J. Control Optim. 39(1), 113–132 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  2. Becker, R., Rannacher, R.: An optimal control approach to a posteriori error estimation. In: Iserles, A. (ed.) Acta Numerica 2001, pp. 1–102. Cambridge University Press, London (2001)

    Google Scholar 

  3. Becker, R., Vexler, B.: A posteriori error estimation for finite element discretizations of parameter identification problems. Numer. Math. 96(3), 435–459 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  4. Becker, R., Vexler, B.: Mesh refinement and numerical sensitivity analysis for parameter calibration of partial differential equations. J. Comput. Phys. 206(1), 95–110 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  5. Braess, D.: Finite Elements: Theory, Fast Solvers and Applications in Solid Mechanics. Cambridge University Press, Cambridge (2007)

    MATH  Google Scholar 

  6. Brenner, S.C., Scott, L.R.: The Mathematical Theory of Finite Element Methods. Springer, Berlin (2002)

    MATH  Google Scholar 

  7. Carey, G.F., Oden, J.T.: Finite Elements. Computational Aspects, vol. 3. Prentice-Hall, New York (1984)

    MATH  Google Scholar 

  8. Casas, E.: Control of an elliptic problem with pointwise state constraints. SIAM J. Control Optim. 24, 1309–1318 (1986)

    Article  MATH  MathSciNet  Google Scholar 

  9. Casas, E.: Boundary control of semilinear elliptic equations with pointwise state constraints. SIAM J. Control Optim. 34, 933–1006 (1993)

    MathSciNet  Google Scholar 

  10. Cherednichenko, S., Krumbiegel, K., Rösch, A.: Error estimates for the Lavrentiev regularization of elliptic optimal control problems. Inverse Probl. (2008, accepted)

  11. Deckelnick, K., Hinze, M.: Convergence of a finite element approximation to a state constrained elliptic control problem. SIAM J. Numer. Anal. 35, 1937–1953 (2007)

    Article  MathSciNet  Google Scholar 

  12. Deckelnick, K., Hinze, M.: A finite element approximation to elliptic control problems in the presence of control and state constraints. Hambg. Beitr. Angew. Math. 2007-01 (2007)

  13. Eriksson, K., Estep, D., Hansbo, P., Johnson, C.: Introduction to adaptive methods for differential equations. In: Iserles, A. (ed.) Acta Numerica 1995, pp. 105–158. Cambridge University Press, London (1995)

    Google Scholar 

  14. The finite element toolkit Gascoigne. http://www.gascoigne.uni-hd.de

  15. Grisvard, P.: Singularities in Boundary Value Problems. Springer, Masson (1992)

    MATH  Google Scholar 

  16. Günther, A., Hinze, M.: A posteriori error control of a state constrained elliptic control problem. J. Numer. Math. (2008, to appear)

  17. Hintermüller, M., Hoppe, R.H.W., Iliash, Y., Kieweg, M.: An a posteriori error analysis of adaptive finite element methods for distributed elliptic control problems with control constraints. ESIAM Control Optim. Calc. Var. 14, 540–560 (2008)

    Article  MATH  Google Scholar 

  18. Hintermüller, M., Hoppe, R.H.W.: Goal-oriented adaptivity in control constrained optimal control of partial differential equations. SIAM J. Control Optim. 47(4), 1721–1743 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  19. Hintermüller, M., Kunisch, K.: Feasible and noninterior path-following in constrained minimization with low multiplier regularity. SIAM J. Control Optim. 45(4), 1198–1221 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  20. Hintermüller, M., Kunisch, K.: Stationary optimal control problems with pointwise state constraints (2007, to appear)

  21. Hinze, M.: A variational discretization concept in control constrained optimization: the linear-quadratic case. Comput. Optim. Appl. 30(1), 45–61 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  22. Hoppe, R.H.W., Kieweg, M.: A posteriori error estimation of finite element approximations of pointwise state constrained distributed control problems (2007, submitted)

  23. Li, R., Liu, W., Ma, H., Tang, T.: Adaptive finite element approximation for distributed elliptic optimal control problems. SIAM J. Control Optim. 41(5), 1321–1349 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  24. Liu, W.: Adaptive multi-meshes in finite element approximation of optimal control. Contemporary Mathematics 383, 113–132 (2005)

    Google Scholar 

  25. Liu, W., Gong, W., Yan, N.: A new finite element approximation of a state constrained optimal control problem. J. Comput. Math. (2008, accepted)

  26. Meidner, D., Vexler, B.: Adaptive space-time finite element methods for parabolic optimization problems. SIAM J. Control Optim. 46(1), 116–142 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  27. Meyer, C.: Error estimates for the finite-element approximation of an elliptic control problem with pointwise state and control constraints. Control Cybern. (2008, to appear)

  28. Meyer, C., Hinze, M.: Stability of infinite dimensional control problems with pointwise state constraints (2007, submitted)

  29. Meyer, C., Prüfert, U., Tröltzsch, F.: On two numerical methods for state-constrained elliptic control problems. Optim. Methods Softw. 22, 871–899 (2007)

    MATH  MathSciNet  Google Scholar 

  30. Meyer, C., Rösch, A., Tröltzsch, F.: Optimal control of PDEs with regularized pointwise state constraints. Comput. Optim. Appl. 33, 209–228 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  31. RoDoBo: a C++ library for optimization with stationary and nonstationary PDEs with interface to Gascoigne. http://www.rodobo.uni-hd.de

  32. Schiela, A.: Barrier methods for optimal control problems with state constraints (2007, submitted)

  33. Schmich, M., Vexler, B.: Adaptivity with dynamic meshes for space-time finite element discretizations of parabolic equations. SIAM J. Sci. Comput. 30(1), 369–393 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  34. Tröltzsch, F.: Optimale Steuerung partieller Differentialgleichungen. Vieweg, Wiesbaden (2005)

    MATH  Google Scholar 

  35. Verfürth, R.: A Review of A Posteriori Error Estimation and Adaptive Mesh-Refinement Techniques. Wiley Teubner, New York/Stuttgart (1996)

    MATH  Google Scholar 

  36. Vexler, B., Wollner, W.: Adaptive finite elements for elliptic optimization problems with control constraints. SIAM J. Control Optim. 47(1), 509–534 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  37. VisuSimple: an interactive VTK-based visualization and graphics/mpeg-generation program. http://www.visusimple.uni-hd.de

Download references

Author information

Authors and Affiliations

  1. Lehrstuhl für Mathematische Optimierung, Fakultät für Mathematik, Technische Universität München, Boltzmannstraße 3, 85748, Garching b. München, Germany

    Olaf Benedix & Boris Vexler

Authors
  1. Olaf Benedix
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Boris Vexler
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Boris Vexler.

Additional information

O. Benedix’s research was supported by the Austrian Science Fund FWF, project P18971-N18 “Numerical analysis and discretization strategies for optimal control problems with singularities”.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Benedix, O., Vexler, B. A posteriori error estimation and adaptivity for elliptic optimal control problems with state constraints. Comput Optim Appl 44, 3–25 (2009). https://doi.org/10.1007/s10589-008-9200-y

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10589-008-9200-y

Keywords

Search

Navigation