Skip to main content
SpringerLink
Log in

Simple formula for integration of polynomials on a simplex

  • Published:
BIT Numerical Mathematics Aims and scope Submit manuscript

Abstract

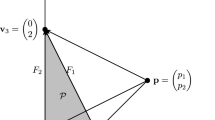
We show that integrating a polynomial f of degree t on an arbitrary simplex (with respect to Lebesgue measure) reduces to evaluating t homogeneous related Bombieri polynomials of degree \(j=1,2,\ldots ,t\), each at a unique point \(\varvec{\xi }_j\) of the simplex. This new and very simple formula could be exploited in finite (and extended finite) element methods, as well as in applications where such integrals must be evaluated. A similar result also holds for a certain class of positively homogeneous functions that are integrable on the canonical simplex.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1

Similar content being viewed by others

Notes

  1. \(f_j\) is the unique form of degree j which is the sum of all monomials of degree j of f (with their coefficient).

  2. A real-valued function \(f:({\mathbb {R}}\setminus \{0\})^n\rightarrow {\mathbb {R}}\) is completely monotone if \((-1)^k\frac{\partial ^k f}{\partial x_{i_1}\cdots \partial x_{i_k}}({\mathbf {x}})\ge 0\) for all \({\mathbf {x}}\in ({\mathbb {R}}\setminus \{0\})^n\) and for all index sequences \(1\le i_1\le \cdots \le i_k\le n\) of arbitrary length k.

  3. In number theory, the Waring problem consists of writing any positive integer as a sum of a fixed number g(n) of nth powers of integers, where g(n) depends only on n. It generalizes to forms as a generic form of degree d can be written as a sum of s d-powers of linear forms; s is called the Waring rank of the form.

References

  1. Antonietti, P.F., Houston, P., Pennesi, G.: Fast numerical integration on polytopic meshes with applications to discontinuous Galerkin finite element methods. J. Sci. Comput. 77, 339–1370 (2018)

    Article  MathSciNet  Google Scholar 

  2. Baldoni, V., Berline, N., De Loera, J.A., Köppe, M., Vergne, M.: How to integrate a polynomial over a simplex. Math. Comput. 80, 297–325 (2011)

    Article  MathSciNet  Google Scholar 

  3. Büeler, B., Enge, A., Fukuda, K.: Exact volume computation for polytopes: a practical study. In Kalai ,G., Ziegler, G.M. (eds) Polytopes—Combinatorics and Computation, volume 29 of DMV Seminar, pp. 131–154. Birkhäuser Verlag, Basel (2000)

  4. Chin, E.B., Lasserre, J.B., Sukumar, N.: Numerical integration of homogeneous functions on convex and nonconvex polygons and polyhedra. Comput. Mech. 56, 967–981 (2015)

    Article  MathSciNet  Google Scholar 

  5. Chin E,B., Sukumar, N.: Modeling curved interfaces without element-partitioning in the extended finite element method. Int. J. Num. Methods Eng. (to appear). https://doi.org/10.1002/nme.6150

  6. Frenning, G.: Efficient Voronoi volume estimation for DEM simulations of granular materials under confined conditions. MethodsX 2, e79–e90 (2015)

    Article  Google Scholar 

  7. Kozhasov, K., Michalek, M., Sturmfels, B.: Positivity certificates via integral representations (2019). arXiv:1908.04191

  8. Lasserre, J.B., Avrachenkov, K.: The multi-dimensional version of \(\int _a^b x^p dx\). Amer. Math. Mon. 108, 151–154 (2001)

    MATH  Google Scholar 

  9. Lasserre, J.B., Zeron, E.S.: Solving a class of multivariable integration problems via Laplace Lechniques. Appl. Math. (Warsaw) 28, 391–405 (2001)

    Article  MathSciNet  Google Scholar 

  10. Lasserre, J.B., Zeron, E.S.: A Laplace transform algorithm for the volume of a convex polytope. J. ACM 48, 1126–1140 (2001)

    Article  MathSciNet  Google Scholar 

  11. Lasserre, J.B.: Integration on a convex polytope. Proc. Am. Math. Soc. 126, 2433–2441 (1998)

    Article  MathSciNet  Google Scholar 

  12. Leoni, F., Yair, Shokef Y.: Attraction controls the inversion of order by disorder in buckled colloidal monolayers. Phys. Rev. Lett. 118, 2180002 (2017)

  13. Nagy, A.P., Benson, D.J.: On the numerical integration of trimmed isogeometric elements. Comput. Methods Appl. Mech. Eng. 284, 165–185 (2015)

    Article  MathSciNet  Google Scholar 

  14. Yong Zhang, Yu., Ma, Hong-Liang Yin, Tan, He-Ping: Natural element method for solving radiative transfer with or without conduction in three-dimensional complex geometries. J. Quant. Spectrosc. Radiat. Transf. 129, 118–130 (2013)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. LAAS-CNRS and Institute of Mathematics, University Fédérale Toulouse Midi-Pyrénées, Toulouse, France

    Jean B. Lasserre

Authors
  1. Jean B. Lasserre
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jean B. Lasserre.

Additional information

Communicated by Elisabeth Larsson.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Research funded by the European Research Council (ERC) under the European’s Union Horizon 2020 research and innovation program (Grant Agreement 666981 TAMING).

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Lasserre, J.B. Simple formula for integration of polynomials on a simplex. Bit Numer Math 61, 523–533 (2021). https://doi.org/10.1007/s10543-020-00828-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10543-020-00828-x

Keywords

Mathematics Subject Classification

Search

Navigation