Quantifying seismic risk in structures via simplified demand–intensity models

Abstract

Performance-based earthquake engineering (PBEE) has traditionally implied the verification of limit states at different earthquake intensities, where recent developments advocate a more risk-consistent approach. This has been primarily investigated for assessing existing structures and typically involves extensive analyses using detailed numerical models and ground motions. For new design, structures must be sized and detailed before more advanced numerical verifications are performed and the final design solution is established. In assessment, simplified procedures have been developed to incorporate further aspects of PBEE and typically comprise extensions to traditional structural analysis methods. Displacement-based assessment is one such method and while it has been extended for PBEE in the past, its use in a risk-oriented context still requires some validation. This article presents such a study, where recent developments in simplified analysis to estimate the exceedance rates of both storey drift and floor acceleration in reinforced concrete frames are described. This gives a method that is simple in its application, since it doesn’t require extensive and detailed numerical modelling or analysis, but also sufficiently accurate in its quantification of performance. While not intended as a substitute to extensive verification analysis, such a method for quantifying structural demand exceedance rates can be used to check results and provide better understanding to risk analysts. The work described herein can also be used in simplified verification analysis of new designs, whereby trial solutions may be verified in a relatively easy manner before more extensive verifications are carried out via non-linear dynamic analysis. It represents a further extension to existing simplified methods that strive toward more advanced performance quantification in line with the needs and goals of PBEE.

Appendix: Intensity measure conversion

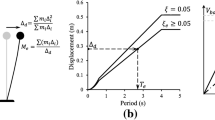
For a smoothed code spectrum of uniform hazard, the shape is generally fixed and the corresponding value of Sd(T₁) can be simply read by scaling it to match the identified point for the equivalent system and reading the value at T₁, as shown in Fig. 12a. When using PSHA data, where seismic hazard data is typically provided for a specified number of vibration periods, the conversion becomes slightly more complicated. Consider Fig. 12b, where the site hazard curves for two different vibration periods are known. What is essentially happening is that knowing Sa(T_e) for a given return period or mean annual frequency of exceedance (MAFE), Sa(T₁) can be computed via the relation between the hazard curves at the two vibration periods. Since PSHA data is typically provided as raw data in the form of a site hazard curve, H(Sa), it would be desirable if a closed-form means of IM conversion could be established. Here, a relatively simple means of converting from one intensity measure to another was sought. It relies simplifying the site hazard curve, but it is noted that other more detailed and thorough ways of converting from one intnesity measure to another are available (e.g. Suzuki and Iervolino 2019).

Fig. 12

Identification of the seismic demand at the first mode period using a smoothed code spectra, or b site hazard curves

For the site hazard curves shown in Fig. 12b, some past researchers have attempted to provide means with which to fit expressions. Cornell et al. (2002) described how the H(Sa) relationship could be approximated by a straight line in logspace. This linear representation was later expanded to become a second-order polynomial, which also better represents the hazard curve over a wider IM range and is described by:

$$H\left( {Sa} \right) = H = k_{0} \exp \left( { - k_{1} \ln Sa - k_{2} \ln^{2} Sa} \right)$$

(19)

where k₀, k₁ and k₂ are best-fit parameters to be established for each individual hazard curve. As shown in Fig. 12b, these will be two separate hazard curves at T_e and T₁ and are denoted H^e and H¹, respectively. What is of interest here is the value of Sa(T₁) when Sa(T_e) is known when H^e and H¹ are equal. Assuming that the best-fit parameters for both hazard curves are known, it follows that:

$$H^{\text{e}} = k_{0}^{\text{e}} \exp \left( { - k_{1}^{\text{e}} \ln Sa\left( {T_{\text{e}} } \right) - k_{2}^{\text{e}} \ln^{2} Sa\left( {T_{\text{e}} } \right)} \right)$$

(20)

$$H^{1} = k_{0}^{1} \exp \left( { - k_{1}^{1} \ln Sa\left( {T_{1} } \right) - k_{2}^{1} \ln^{2} Sa\left( {T_{1} } \right)} \right)$$

(21)

Setting H^e equal to H¹ gives:

$$k_{0}^{\text{e}} \exp \left( { - k_{1}^{\text{e}} \ln Sa\left( {T_{\text{e}} } \right) - k_{2}^{\text{e}} \ln^{2} Sa\left( {T_{\text{e}} } \right)} \right) = k_{0}^{1} \exp \left( { - k_{1}^{1} \ln Sa\left( {T_{1} } \right) - k_{2}^{1} \ln^{2} Sa\left( {T_{1} } \right)} \right)$$

(22)

Rearranging to give:

$$\frac{{k_{0}^{\text{e}} }}{{k_{0}^{1} }} = \frac{{\exp \left( { - k_{1}^{1} \ln Sa\left( {T_{1} } \right) - k_{2}^{1} \ln^{2} Sa\left( {T_{1} } \right)} \right)}}{{\exp \left( { - k_{1}^{\text{e}} \ln Sa\left( {T_{\text{e}} } \right) - k_{2}^{\text{e}} \ln^{2} Sa\left( {T_{\text{e}} } \right)} \right)}}$$

(23)

and taking the natural logarithm of both sides then gives:

$$\ln \frac{{k_{0}^{\text{e}} }}{{k_{0}^{1} }} = - k_{1}^{1} \ln Sa\left( {T_{1} } \right) - k_{2}^{1} \ln^{2} Sa\left( {T_{1} } \right) + k_{1}^{\text{e}} \ln Sa\left( {T_{\text{e}} } \right) + k_{2}^{\text{e}} \ln^{2} Sa\left( {T_{\text{e}} } \right)$$

(24)

Letting ln Sa(T₁) equal X and rearranging gives:

$$\left( {k_{2}^{1} } \right)X^{2} + \left( {k_{1}^{1} } \right){\text{X}} + \left( {\ln \frac{{k_{0}^{\text{e}} }}{{k_{0}^{1} }} - k_{1}^{\text{e}} \ln Sa\left( {T_{\text{e}} } \right) - k_{2}^{\text{e}} \ln^{2} Sa\left( {T_{\text{e}} } \right)} \right) = 0$$

(25)

which results in a quadratic polynomial that can be solved as:

$$X = \frac{{ - k_{1}^{1} \pm \sqrt {\left( {k_{1}^{1} } \right)^{2} - 4\left( {k_{2}^{1} } \right)\left( {\ln \frac{{k_{0}^{\text{e}} }}{{k_{0}^{1} }} - k_{1}^{\text{e}} \ln Sa\left( {T_{\text{e}} } \right) - k_{2}^{\text{e}} \ln^{2} Sa\left( {T_{\text{e}} } \right)} \right)} }}{{2k_{2}^{1} }}$$

(26)

Substituting back in for X gives:

$$Sa\left( {T_{1} } \right) = \exp \left( {\frac{{ - k_{1}^{1} \pm \sqrt {\left( {k_{1}^{1} } \right)^{2} - 4\left( {k_{2}^{1} } \right)\left( {\ln \frac{{k_{0}^{\text{e}} }}{{k_{0}^{1} }} - k_{1}^{\text{e}} \ln Sa\left( {T_{\text{e}} } \right) - k_{2}^{\text{e}} \ln^{2} Sa\left( {T_{\text{e}} } \right)} \right)} }}{{2k_{2}^{1} }}} \right)$$

(27)

which will return two solutions, one of which will be unrealistic. This expression provides a means with which a common intensity measure can be found for any effective period of vibration, T_e, to give a consistent demand–intensity relationship in Sa(T₁).