A constrained integrated imperfect manufacturing-inventory system with preventive maintenance and partial backordering

Abstract

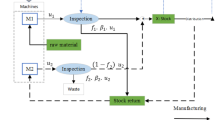
In this paper, a multi-product single-machine economic production quantity model with preventive maintenance, scraped and rework is studied. Shortages are permitted and a fraction of them is backlogged. Capacity and service level are limitations of the production system. It is assumed that preventive maintenance can be performed when the inventory level is positive or negative. Indeed two different scenarios are modeled and according to the comparisons between their costs, a new scenario according to the best time of preventive maintenance is investigated and modeled. The aim of this research is to determine the best time for preventive maintenance, production and back-ordered quantities of each item and common cycle length, such that the expected total cost is minimized. The objective function of the final proposed model is proved to be convex and closed-form optimal solutions are derived. Two numerical examples based on a real application of the proposed model in a turning manufactory with only one computer numerical control machine applied to lathe metal plates to different sizes are used to illustrate the applicability of extended model and proposed solution method.

Appendices

Appendix (A): Holding cost of perfect items

From Eq. (40),

$$\begin{aligned} HCPQ_i= & {} h_i \left[ \frac{H_i }{2}\left( t_i^4 \right) +\frac{H_i +H_i^{Max} }{2}\left( t_i^5 \right) +\frac{H_i^{Max} }{2}\left( t_i^6 \right) \right] \\= & {} h_i \left[ \frac{H_i }{2}\left( t_i^4 +t_i^5 \right) +\frac{H_i^{Max} }{2}\left( t_i^5 +t_i^6 \right) \right] \end{aligned}$$

Knowing $X_i =P_i -\varphi _i -d_i$and assuming $U_i =P_i^1 -\varphi _i^1 -d_i$ we have;

$$\begin{aligned} HCPQ_i= & {} h_i \left[ {\frac{H_i }{2}\left( {\frac{H_i }{N_i }+\frac{x_i q_i }{P_i^1 }} \right) +\frac{H_i^{Max} }{2}\left( {\frac{x_i q_i }{P_i^1 }+\frac{H_i^{Max} }{d_i }} \right) } \right] \\= & {} \frac{h_i }{2}\left[ {\left( {\frac{H_i ^{2}}{X_i }+\frac{x_i H_i d_i }{P_i^1 }} \right) +\left( {\frac{x_i H_i^{Max} q_i }{P_i^1 }+\frac{\left( {H_i^{Max} } \right) ^{2}}{d_i }} \right) } \right] \\= & {} \frac{h_i }{2}\left[ {\begin{array}{l} \frac{\left( {X_i \frac{q_i }{P_i }-\beta _i b_i -d_i t_m } \right) ^{2}}{X_i }+\frac{x_i q_i }{P_i^1 }\left( {X_i \frac{q_i }{P_i }-\beta _i b_i -d_i t_m +U_i \frac{x_i q_i }{P_i^1 }} \right) \\ +\frac{x_i \left( {X_i \frac{q_i }{P_i }-\beta _i b_i -d_i t_m } \right) q_i }{P_i^1 }+\frac{\left( {X_i \frac{q_i }{P_i }-\beta _i b_i -d_i t_m +U_i \frac{x_i q_i }{P_i^1 }} \right) ^{2}}{d_i } \\ \end{array}} \right] \end{aligned}$$

finally,

$$\begin{aligned}= & {} \frac{h_i }{2}\left[ {\begin{array}{l} \frac{\left( {X_i \frac{q_i }{P_i }-\beta _i b_i -d_i t_m } \right) ^{2}}{X_i }+\frac{x_i \left( {X_i \frac{q_i }{P_i }-\beta _i b_i -d_i t_m } \right) q_i }{P_i^1 } \\ +\frac{x_i q_i }{P_i^1 }\left( {X_i \frac{q_i }{P_i }-\beta _i b_i -d_i t_m +U_i \frac{x_i q_i }{P_i^1 }} \right) +\frac{\left( {X_i \frac{q_i }{P_i }-\beta _i b_i -d_i t_m +U_i \frac{x_i q_i }{P_i^1 }} \right) ^{2}}{d_i } \\ \end{array}} \right] \\= & {} \frac{h_i }{2}\left[ {\begin{array}{l} \frac{\left( {\left( {X_i \frac{q_i }{P_i }-\beta _i b_i } \right) -d_i t_m } \right) ^{2}}{X_i }+\frac{x_i \left( {X_i \frac{q_i }{P_i }-\beta _i b_i -d_i t_m } \right) q_i }{P_i^1 } \\ +\frac{x_i q_i }{P_i^1 }\left( {\left( {\frac{X_i }{P_i }+\frac{x_i U_i }{P_i^1 }} \right) q_i -\beta _i b_i -d_i t_m } \right) +\frac{\left( {\left( {\frac{X_i }{P_i }+\frac{x_i U_i }{P_i^1 }} \right) q_i -\beta _i b_i -d_i t_m } \right) ^{2}}{d_i } \\ \end{array}} \right] \\= & {} \frac{h_i }{2}\left[ {\begin{array}{l} \frac{\left( {\left( {X_i \frac{q_i }{P_i }-\beta _i b_i } \right) -d_i t_m } \right) ^{2}}{X_i }+\frac{x_i q_i }{P_i^1 }\left( {\left( {\frac{2X_i }{P_i }+\frac{x_i U_i }{P_i^1 }} \right) q_i -2\beta _i b_i -2d_i t_m } \right) \\ +\frac{\left( {\left( {\frac{X_i }{P_i }+\frac{x_i U_i }{P_i^1 }} \right) q_i -\beta _i b_i -d_i t_m } \right) ^{2}}{d_i } \\ \end{array}} \right] \\= & {} \frac{h_i }{2}\left[ {\begin{array}{l} \frac{\left( {\left( {X_i \frac{q_i }{P_i }-\beta _i b_i } \right) ^{2}-2d_i t_m \left( {X_i \frac{q_i }{P_i }-\beta _i b_i } \right) +d_i ^{2}t_m ^{2}} \right) }{X_i }+\frac{x_i q_i }{P_i^1 }\left( {\left( {\frac{2X_i }{P_i }+\frac{x_i U_i }{P_i^1 }} \right) q_i -2\beta _i b_i -2d_i t_m } \right) \\ +\frac{\left( {\left( {\frac{X_i }{P_i }+\frac{x_i U_i }{P_i^1 }} \right) ^{2}q_i ^{2}+\left( {\beta _i b_i +d_i t_m } \right) ^{2}-2\left( {\frac{X_i }{P_i }+\frac{x_i U_i }{P_i^1 }} \right) q_i \left( {\beta _i b_i +d_i t_m } \right) } \right) }{d_i }\\ \end{array}} \right] \\= & {} \frac{h_i }{2}\left[ {\begin{array}{l} \frac{\left( {\left( {\frac{X_i }{P_i }} \right) ^{2}q_i ^{2}+\beta _i ^{2}b_i ^{2}-2\left( {\frac{X_i }{P_i }} \right) q_i \beta _i b_i -2d_i t_m \left( {X_i \frac{q_i }{P_i }-\beta _i b_i } \right) +d_i ^{2}t_m ^{2}} \right) }{X_i }-2\left( {\frac{X_i }{P_i }+\frac{x_i U_i }{P_i^1 }} \right) \left( {q_i \beta _i b_i +t_m q_i } \right) \\ -\frac{2x_i q_i }{P_i^1 }\left( {\beta _i b_i +d_i t_m } \right) +\frac{\left( {\frac{X_i }{P_i }+\frac{x_i U_i }{P_i^1 }} \right) ^{2}q_i ^{2}+\beta _i ^{2}b_i ^{2}+\left( {d_i t_m } \right) ^{2}+2d_i t_m \beta _i b_i }{d_i }+\left( {\frac{2x_i X_i }{P_i P_i^1 }+\frac{x_i ^{2}U_i }{\left( {P_i^1 } \right) ^{2}}} \right) q_i ^{2} \\ \end{array}} \right] \\= & {} \frac{h_i }{2}\left[ {\begin{array}{l} \left( {\frac{X_i }{P_i ^{2}}} \right) q_i ^{2}+\frac{1}{X_i }\beta _i ^{2}b_i ^{2}-2\left( {\frac{1}{P_i }} \right) q_i \beta _i b_i -2d_i t_m \left( {\frac{1}{P_i }q_i -\frac{1}{X_i }\beta _i b_i } \right) +\frac{d_i ^{2}t_m ^{2}}{X_i } \\ \left( {\frac{2x_i X_i }{P_i P_i^1 }+\frac{x_i ^{2}U_i }{\left( {P_i^1 } \right) ^{2}}} \right) q_i ^{2}-\frac{2x_i }{P_i^1 }q_i \beta _i b_i -\frac{2x_i d_i t_m }{P_i^1 }q_i +\frac{\left( {\frac{X_i }{P_i }+\frac{x_i U_i }{P_i^1 }} \right) ^{2}q_i ^{2}}{d_i } \\ +\frac{\beta _i ^{2}b_i ^{2}}{d_i }+d_i \left( {t_m } \right) ^{2}+2t_m \beta _i b_i -2\left( {\frac{X_i }{P_i }+\frac{x_i U_i }{P_i^1 }} \right) q_i \beta _i b_i -2\left( {\frac{X_i }{P_i }+\frac{x_i U_i }{P_i^1 }} \right) t_m q_i \\ \end{array}} \right] \\= & {} \frac{h_i }{2}\left( {\frac{X_i }{P_i ^{2}}+\frac{2x_i X_i }{P_i P_i^1 }+\frac{x_i ^{2}U_i }{\left( {P_i^1 } \right) ^{2}}+\frac{\left( {\frac{X_i }{P_i }+\frac{x_i U_i }{P_i^1 }} \right) ^{2}}{d_i }} \right) Q_i ^{2}+\frac{h_i \beta _i ^{2}}{2}\left( {\frac{1}{X_i }+\frac{1}{d_i }} \right) B_i ^{2}\\&\quad +\,\frac{C_i^h \left( {d_i ^{2}+D_i X_i } \right) t_m ^{2}}{2X_i }-h_i \beta _i \left( {\frac{1}{P_i }+\frac{x_i }{P_i^1 }+\left( {\frac{X_i }{P_i }+\frac{x_i U_i }{P_i^1 }} \right) } \right) q_i b_i \\&\quad -\,h_i t_m \left( {\frac{d_i +X_i }{P_i }+\frac{x_i \left( {d_i +U_i } \right) }{P_i^1 }} \right) q_i +h_i \beta _i t_m \left( {\frac{X_i +d_i }{X_i }} \right) b_i \end{aligned}$$

Appendix (B): Cyclic objective function

We know,

$$\begin{aligned} TC\left( q,b\right)= & {} K_i +\left( {C_i^P +R_i x_i +V_i x_i \theta _i } \right) q_i\\&\quad +\,\pi _i \left[ {\frac{b_i }{2}\left( t_i^1 +t_i^7 \right) +\frac{H_i^1 }{2}\left( t_i^2 +t_i^3 \right) } \right] +\hat{{\pi }}_i \left[ \left( 1-\beta _i \right) d_i t_i^2 +\left( 1-\beta _i \right) b_i \right] \\&\quad +\,h_i \left[ \frac{H_i }{2}\left( t_i^4 \right) +\frac{H_i +H_i^{Max} }{2}\left( t_i^5 \right) +\frac{H_i^{Max} }{2}\left( t_i^6 \right) \right] \\&\quad +\,{h}'_i \left[ \frac{P_i x_i \theta \left( t_i^1 +t_i^3 +t_i^4 \right) }{2}\left( t_i^5 \right) \right] \\&\quad +\,h_i \left[ \frac{P_i x_i t_i^1 }{2}\left( t_i^1 \right) +P_i x_i t_i^1 \left( t_i^2 \right) +P_i x_i t_i^1 \left( t_i^3 +t_i^4 \right) +\frac{P_i x_i \left( t_i^3 +t_i^4 \right) }{2}\left( t_i^3 +t_i^4 \right) \right. \\&\left. \quad +\,\frac{P_i x_i \left( t_i^1 +t_i^3 +t_i^4 \right) }{2}t_i^5 \right] \end{aligned}$$

after some simplifications the cyclic total cost function is:

$$\begin{aligned}&TC(q,b)=\left( {\frac{h_i }{2}\left( {\frac{X_i }{P_i ^{2}}+\frac{2x_i X_i }{P_i P_i^1 }+\frac{x_i }{P_i }+\frac{x_i ^{2}}{P_i^1 }+\frac{x_i ^{2}U_i }{\left( {P_i^1 } \right) ^{2}}+\frac{\left( {\frac{X_i }{P_i }+\frac{x_i U_i }{P_i^1 }} \right) ^{2}}{d_i }} \right) +\frac{{h}'_i \theta _i \left( {x_i } \right) ^{2}}{2P_i^1 }} \right) q_i ^{2}\\&\quad +\left( {\frac{\pi _i \beta _i Y_i }{2d_i X_i }+\frac{h_i \beta _i ^{2}}{2}\left( {\frac{1}{X_i }+\frac{1}{d_i }} \right) } \right) b_i^2 -h_i \beta _i \left( {\frac{1}{P_i }+\frac{x_i }{P_i^1 }+\left( {\frac{X_i }{P_i }+\frac{x_i U_i }{P_i^1 }} \right) } \right) q_i b_i\\&\quad +\left( {C_i^P +R_i x_i +V_i x_i \theta _i -h_i t_m \left( {\frac{d_i +X_i }{P_i }+\frac{x_i \left( {d_i +U_i } \right) }{P_i^1 }} \right) } \right) q_i\\&\quad +\left( {h_i \beta _i t_m \left( {\frac{X_i +d_i +P_i x_i }{X_i }} \right) +\hat{{\pi }}_i (1-\beta _i )} \right) b_i\\&\quad +\,K_i +\frac{C_i^h \left( {d_i ^{2}+d_i X_i } \right) t_m ^{2}}{2X_i }+\frac{\pi _i d_i (P_i -\varphi _i )\left( {t_m } \right) ^{2}}{2X_i }+\hat{{\pi }}_i (1-\beta _i )d_i t_m \end{aligned}$$

Appendix (C): Convexity of objective function

$$\begin{aligned}&F=TC(T,b_i )=\sum \limits _{i=1}^n {\lambda _i^1 \frac{b_i ^{2}}{T}} +\sum \limits _{i=1}^n {\lambda _i^2 } \frac{b_i }{T}+\sum \limits _{i=1}^n {\lambda _i^3 } T -\sum \limits _{i=1}^n {\lambda _i^4 } b_i +\sum \limits _{i=1}^n {\lambda _i^5 } \frac{1}{T}+\sum \limits _{i=1}^n {\lambda _i^6 }\\&\frac{\partial F}{\partial T}=\frac{-\sum \limits _{i=1}^n {\lambda _i^1 b_i^2 -\sum \limits _{i=1}^n {\lambda _i^2 b_i } -\sum \limits _{i=1}^n {\lambda _i^5 } } }{T^{2}}+\sum \limits _{i=1}^n {\lambda _i^3 } \\&\frac{\partial ^{2}F}{\partial ^{2}T}=\frac{2\sum \limits _{i=1}^n {\lambda _i^1 b_i^2 +2\sum \limits _{i=1}^n {\lambda _i^2 b_i } +2\sum \limits _{i=1}^n {\lambda _i^5 } } }{T^{3}}\\&\frac{\partial ^{2}F}{\partial T\partial b_i }=\frac{-2\lambda _i^1 b_i -\lambda _i^2 }{T^{2}} \end{aligned}$$

(C1)

$$\begin{aligned}&\frac{\partial F}{\partial b_i }=\frac{2\lambda _i^1 b_i +\lambda _i^2 }{T}-\lambda _i^4 \\&\frac{\partial ^{2}F}{\partial ^{2}b_i }=\frac{2\lambda _i^1 }{T}\\&\frac{\partial ^{2}F}{\partial b_i \partial T}=\frac{-2\lambda _i^1 b_i -\lambda _i^2 }{T^{2}}\\&[T, b_1 ,b_2 ,\ldots ,b_n ]\times \left[ {{\begin{array}{lllll} {\frac{\partial ^{2}F}{\partial ^{2}T}}&{} {\frac{\partial ^{2}F}{\partial T\partial b_1 }}&{} {\frac{\partial ^{2}F}{\partial T\partial b_2 }}&{} \cdots &{} {\frac{\partial ^{2}F}{\partial T\partial b_n }} \\ &{}&{}&{}&{} \\ {\frac{\partial ^{2}F}{\partial b_1 \partial T}}&{} {\frac{\partial ^{2}F}{\partial ^{2}b_1 }}&{} {\frac{\partial ^{2}F}{\partial b_1 \partial b_2 }}&{} \cdots &{} {\frac{\partial ^{2}F}{\partial b_1 \partial b_n }} \\ &{}&{}&{}&{} \\ {\frac{\partial ^{2}F}{\partial b_2 \partial T}}&{} {\frac{\partial ^{2}F}{\partial b_2 \partial b_1 }}&{} {\frac{\partial ^{2}F}{\partial ^{2}b_2 }}&{} \cdots &{} {\frac{\partial ^{2}F}{\partial b_2 \partial b_n }} \\ &{}&{}&{}&{} \\ \quad \vdots &{} \quad \vdots &{} \quad \vdots &{} \vdots &{}\quad \vdots \\ &{}&{}&{}&{} \\ {\frac{\partial ^{2}F}{\partial b_n \partial T}}&{} {\frac{\partial ^{2}F}{\partial b_n \partial b_1 }}&{} {\frac{\partial ^{2}F}{\partial b_n \partial b_2 }}&{} \cdots &{} {\frac{\partial ^{2}F}{\partial ^{2}b_n }} \\ \end{array} }} \right] \left[ {{\begin{array}{l} T \\ {b_1 } \\ {b_2 } \\ \vdots \\ {b_n } \\ \end{array} }} \right] \\&=[T, b_1 ,b_2 ,\ldots ,b_n ]\times \left[ {{\begin{array}{lllll} {\frac{2\sum \limits _{i=1}^n {(\lambda _i^1 b_i^2 +\lambda _i^2 b_i +\lambda _i^5 )} }{T^{3}}}&{} {\frac{-2\lambda _1^1 b_1 -\lambda _1^2 }{T^{2}}}&{} {\frac{-2\lambda _2^1 b_2 -\lambda _2^2 }{T^{2}}}&{} \cdots &{} {\frac{-2\lambda _n^1 b_n -\lambda _n^2 }{T^{2}}} \\ &{}&{}&{}&{}\\ {\frac{-2\lambda _1^1 b_1 -\lambda _1^2 }{T^{2}}}&{} {\frac{2\lambda _1^1 }{T}}&{} 0&{} \cdots &{} 0 \\ &{}&{}&{}&{}\\ {\frac{-2\lambda _2^1 b_2 -\lambda _2^2 }{T^{2}}}&{} 0&{} {\frac{2\lambda _2^1 }{T}}&{} \cdots &{} 0 \\ &{}&{}&{}&{}\\ \vdots &{} \vdots &{} \vdots &{} \vdots &{} \vdots \\ &{}&{}&{}&{}\\ {\frac{-2\lambda _n^1 b_n -\lambda _n^2 }{T^{2}}}&{} 0&{} 0&{} \cdots &{} {\frac{2\lambda _n^1 }{T}} \\ \end{array} }} \right] \left[ {{\begin{array}{l} T \\ {b_1 } \\ {b_2 } \\ \vdots \\ {b_n } \\ \end{array} }} \right] \\&=\left[ {{\begin{array}{lllll} {\frac{\sum \limits _{i=1}^n {\psi _i^2 b_i } +\sum \limits _{i=1}^n {\lambda _i^5 } }{T^{2}}}&{} {-\frac{\lambda _1^2 }{T}}&{} {-\frac{\lambda _2^2 }{T}}&{} \cdots &{} {-\frac{\lambda _n^2 }{T}} \\ &{}&{}&{}&{}\\ \end{array} }} \right] \left[ {{\begin{array}{l} T \\ {b_1 } \\ {b_2 } \\ \vdots \\ {b_n } \\ \end{array} }} \right] =\frac{\sum \limits _{i=1}^n {\lambda _i^5 } }{T}\ge 0 \end{aligned}$$

(C2)

Appendix (D): Deriving the optimal solution

Setting the first derivative of objective function respect to T equal to zero gives;

$$\begin{aligned} \frac{\partial F}{\partial T}&=\frac{-\sum \limits _{i=1}^n {\lambda _i^1 b_i^2 -\sum \limits _{i=1}^n {\lambda _i^2 b_i } -\sum \limits _{i=1}^n {\lambda _i^5 } } }{T^{2}}+\sum \limits _{i=1}^n {\lambda _i^3 } =0\\ T^{2}&=\frac{\sum \limits _{i=1}^n {\lambda _i^1 b_i^2 +\sum \limits _{i=1}^n {\lambda _i^2 b_i } +\sum \limits _{i=1}^n {\lambda _i^5 } } }{\sum \limits _{i=1}^n {\lambda _i^3 } } \end{aligned}$$

(D1)

And setting the first derivative of objective function respect to $b_i$ equal to zero gives;

$$\begin{aligned} \frac{\partial F}{\partial b_i }&=\frac{2\lambda _i^1 b_i +\lambda _i^2 }{T}-\lambda _i^4 =0\\ b_i&=\frac{\lambda _i^4 T-\lambda _i^2 }{2\lambda _i^1 } \end{aligned}$$

(D2)

Substituting (D2) in (D1) yields;

$$\begin{aligned}&T^{2}=\frac{\sum \nolimits _{i=1}^n {\lambda _i^1 \left( {\frac{\lambda _i^4 T-\lambda _i^2 }{2\lambda _i^1 }} \right) ^{2}+\sum \nolimits _{i=1}^n {\lambda _i^2 \left( {\frac{\lambda _i^4 T-\lambda _i^2 }{2\lambda _i^1 }} \right) } +\sum \nolimits _{i=1}^n {\lambda _i^5 } } }{\sum \nolimits _{i=1}^n {\lambda _i^3 } }\\&\quad T^{2}=\frac{\sum \nolimits _{i=1}^n {\left( {\frac{\left( {\lambda _i^4 T} \right) ^{2}-2\lambda _i^2 \lambda _i^4 T+\left( {\lambda _i^2 } \right) ^{2}}{4\lambda _i^1 }} \right) +\sum \nolimits _{i=1}^n {\left( {\frac{\lambda _i^2 \lambda _i^4 T-\left( {\lambda _i^2 } \right) ^{2}}{2\lambda _i^1 }} \right) } +\sum \nolimits _{i=1}^n {\lambda _i^5 } } }{\sum \nolimits _{i=1}^n {\lambda _i^3 } }\\&\quad \sum \limits _{i=1}^n {\lambda _i^3 } T^{2}=\sum \limits _{i=1}^n {\left( {\frac{\left( {\lambda _i^4 T} \right) ^{2}-2\lambda _i^2 \lambda _i^4 T+\left( {\lambda _i^2 } \right) ^{2}}{4\lambda _i^1 }} \right) +\sum \limits _{i=1}^n {\left( {\frac{\lambda _i^2 \lambda _i^4 T-\left( {\lambda _i^2 } \right) ^{2}}{2\lambda _i^1 }} \right) } +\sum \limits _{i=1}^n {\lambda _i^5 } }\\&\quad \sum \limits _{i=1}^n {\lambda _i^3 } T^{2}=\sum \limits _{i=1}^n {\left( {\frac{\left( {\lambda _i^4 T} \right) ^{2}+\left( {\lambda _i^2 } \right) ^{2}}{4\lambda _i^1 }} \right) +\sum \limits _{i=1}^n {\left( {\frac{-\left( {\lambda _i^2 } \right) ^{2}}{2\lambda _i^1 }} \right) } +\sum \limits _{i=1}^n {\lambda _i^5 } }\\&\quad \left( {\sum \limits _{i=1}^n {\lambda _i^3 } -\sum \limits _{i=1}^n {\left( {\frac{\left( {\lambda _i^4 } \right) ^{2}}{4\lambda _i^1 }} \right) } } \right) T^{2}=\sum \limits _{i=1}^n {\left( {\frac{4\lambda _i^1 \lambda _i^5 -\left( {\lambda _i^2 } \right) ^{2}}{4\lambda _i^1 }} \right) }\\&\quad T=\sqrt{\frac{\sum \nolimits _{i=1}^n {\left( {\frac{4\lambda _i^1 \lambda _i^5 -\left( {\lambda _i^2 } \right) ^{2}}{4\lambda _i^1 }} \right) } }{\sum \nolimits _{i=1}^n {\left( {\lambda _i^3 -\frac{\left( {\lambda _i^4 } \right) ^{2}}{4\lambda _i^1 }} \right) } }} \end{aligned}$$