The fractional metric dimension of permutation graphs

Abstract

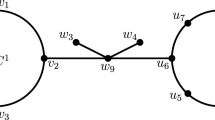
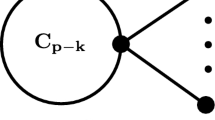
Let G = (V (G),E(G)) be a graph with vertex set V (G) and edge set E(G). For two distinct vertices x and y of a graph G, let R _G {x, y} denote the set of vertices z such that the distance from x to z is not equal to the distance from y to z in G. For a function g defined on V (G) and for U ⊆ V (G), let g(U) = Σ _{s∈ U} g(s). A real-valued function g: V (G) → [0, 1] is a resolving function of G if g(R _G {x, y}) ≥ 1 for any two distinct vertices x, y ∈ V (G). The fractional metric dimension dim _f (G) of a graph G is min{g(V (G)): g is a resolving function of G}. Let G ₁ and G ₂ be disjoint copies of a graph G, and let σ: V (G ₁) → V (G ₂) be a bijection. Then, a permutation graph G _σ = (V, E) has the vertex set V = V (G ₁) ∪ V (G ₂) and the edge set E = E(G ₁) ∪ E(G ₂) ∪ {uv | v = σ(u)}. First, we determine dimf (T) for any tree T. We show that $1 < \dim _f (G_\sigma ) \leqslant \tfrac{1} {2}(|V(G)| + |S(G)|)$ for any connected graph G of order at least 3, where S(G) denotes the set of support vertices of G. We also show that, for any ɛ > 0, there exists a permutation graph G _σ such that dim _f (G _σ) - 1 < ε. We give examples showing that neither is there a function h ₁ such that dim _f (G) < h ₁(dim _f (G _σ)) for all pairs (G, σ), nor is there a function h ₂ such that h ₂(dim _f (G)) > dim _f (G _σ)) for all pairs (G, σ). Furthermore, we investigate dim _f (G _σ)) when G is a complete k-partite graph or a cycle.