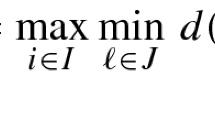Abstract
We study the n-dimensional problem of finding the smallest ball enclosing the intersection of p given balls, the so-called Chebyshev center problem (\({\mathrm{CC}}_{\mathrm{B}}\)). It is a minimax optimization problem and the inner maximization is a uniform quadratic optimization problem (\(\mathrm{UQ}\)). When \(p\le n\), (\(\mathrm{UQ}\)) is known to enjoy a strong duality and consequently (\({\mathrm{CC}}_{\mathrm{B}}\)) is solved via a standard convex quadratic programming (\(\mathrm{SQP}\)). In this paper, we first prove that (\({\mathrm{CC}}_{\mathrm{B}}\)) is NP-hard and the special case when \(n=2\) is polynomially solvable. With the help of a newly introduced linear programming relaxation (LP), the (\(\mathrm{SQP}\)) relaxation is reobtained more directly and the first approximation bound for the solution obtained by (\(\mathrm{SQP}\)) is established for the hard case \(p>n\). Finally, also based on (LP), we show that (\({\mathrm{CC}}_{\mathrm{B}}\)) is polynomially solvable when either n or \(p-n(>0)\) is fixed.
Similar content being viewed by others
Notes
A set \(\varOmega \) is balanced if \(x\in \varOmega \) implies \(-x\in \varOmega \).
Limited-memory BFGS is a practical quasi-Newton method that uses a limited amount of computer memory to approximate the Broyden–Fletcher–Goldfarb–Shanno (BFGS) algorithm.
There are algorithms converging to the square root at least quadratically, for example, Newton’s method. In practice, \(O(\log \log u^{-1})\) can be already regarded as a constant.
Consider two circles sharing the same chord. It is not difficult to verify that the minor arc of the larger circle corresponding to the given chord is covered by the smaller circle.
References
Beck, A., Eldar, Y.C.: Strong duality in nonconvex quadratic optimization with two quadratic constraints. SIAM J. Optim. 17(3), 844–860 (2006)
Beck, A., Eldar, Y.C.: Regularization in regression with bounded noise: a Chebyshev center approach. SIAM J. Matrix Anal. Appl. 29(2), 606–625 (2007)
Beck, A.: On the convexity of a class of quadratic mappings and its application to the problem of finding the smallest ball enclosing a given intersection of balls. J. Global Optim. 39(1), 113–126 (2007)
Beck, A.: Convexity properties associated with nonconvex quadratic matrix functions and applications to quadratic programming. J. Optim. Theory Appl. 142(1), 1–29 (2009)
Belykh, T.I., Bulatov, V.P., Yas’kova, E.N.: Methods of Chebyshev points of convex sets and their applications. Comput. Math. Math. Phys. 48(1), 16–29 (2008)
Bertsekas, D.P.: Nonlinear Programming, 2nd edn. Athena Scientific, Belmont (1999)
Bogdewicz, A., Moszyńska, M.: Čebyšev sets in the space of convex bodies. Rend. Circ. Mat. Palermo 2(Suppl. 77), 19–39 (2006)
Boyd, S., El Ghaoui, L., Feron, E., Balakrishnan, V.: Linear Matrix Inequalities in System and Control Theory. SIAM, Philadelphia (1994)
Bubeck, S., Lee, Y.T., Singh, M.: A geometric alternative to Nesterov’s accelerated gradient descent. arXiv:1506.08187v1 (2015)
Bienstock, D., Michalka, A.: Polynomial solvability of variants of the trust-region subproblem. In: Proceedings of the Twenty-Fifth Annual ACM-SIAM Symposium on Discrete Algorithms, pp. 380–390 (2014)
Celis, M.R., Dennis, J.E., Tapia, R.A.: A trust region strategyfor nonlinear equality constrained optimization. In: Numerical Optimization, 1984 (Boulder, Colo., 1984), pp. 71–82. SIAM, Philadelphia (1985)
Cen, X., Xia, Y., Gao, Y., Yang, T.: On Chebyshev center of the intersection of two ellipsoids. World congress on global optimization (WCGO) 2019. In: Le Thi, H.A., et al. (eds.) Optimization of Complex Systems: Theory, Models, Algorithms and Applications, AISC, vol. 991, pp. 135–144. Springer, Berlin (2020)
Eldar, Y.C., Beck, A., Teboulle, M.: A minimax Chebyshev estimator for bounded error estimation. IEEE Trans. Signal Process. 56(4), 1388–1397 (2008)
Elzinga, J., Hearn, D.: The minimum sphere covering a convex polyhedron. Nav. Res. Logistic Q. 21, 715–718 (1974)
Garey, M.R., Johnson, D.S.: Computers and Intractability: A Guide to the Theory of NP-Completeness. Freeman, San Francisco (1979)
Gholami, M.R., Wymeersch, H., Ström, E.G., Rydström, M.: Wireless network positioning as a convex feasibility problem. EURASIP J. Wirel. Commun. Netw. 1, 161 (2011)
Gholami, M.R., Ström, E.G., Wymeersc, H., Rydström, M.: On geometric upper bounds for positioning algorithms in wireless sensor networks. Signal Process. 111, 179–193 (2015)
Henrion, D., Tarbouriech, S., Arzelier, D.: LMI approximations for the radius of the intersection of ellipsoids: survey. J. Optim. Theory Appl. 108, 1–28 (2001)
Hsia, Y., Wang, S., Xu, Z.: Improved semidefinite approximation bounds for nonconvex nonhomogeneous quadratic optimization with ellipsoid constraints. Oper. Res. Lett. 43(4), 378–383 (2015)
Megiddo, N.: Linear-time algorithms for linear programming in \({\mathbb{R}}^3\) and related problems. SIAM J. Comput. 12, 759–776 (1983)
Milanese, M., Tempo, R.: Optimal algorithms theory for robust estimation and prediction. IEEE Trans. Autom. Control. 30(8), 730–738 (1985)
Nenakhov, E.I., Primak, M.E.: Convergence of the method of Chebyshev centers and some applications. Cybern. Syst. Anal. 22(2), 219–226 (1986)
Nesterov, Y.: Introductory Lectures on Convex Optimizaiton: A Basic Course of Applied Optimization, vol. 87. Kluwer, Boston (2004)
Shor, N.Z.: Quadatric optimization problems. Sov. J. Comput. Syst. Sci. 25, 1–11 (1987)
Sylvester, J.J.: A question in the geometry of situation. Q. J. Math. 1, 79 (1857)
Tseng, P.: Further results on approximating nonconvex quadratic optimization by semidefinite programming relaxation. SIAM J. Optim. 14, 268–283 (2003)
Vandenberghe, L., Boyd, S.: Semidefinite programming. SIAM Rev. 38(1), 49–95 (1996)
Wu, D., Zhou, J., HU, A.: A new approximate algorithm for the Chebyshev center. Automatica 49, 2483–2488 (2013)
Acknowledgements
The authors are grateful to the associated editor and two anonymous referees for very helpful comments and suggestions.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
This research was supported by National Science Fund for Excellent Young Scholars under Grants 11822103, National Natural Science Foundation of China under Grants 11801173, 11571029, 11771056 and Beijing Natural Science Foundation Z180005.
Rights and permissions
About this article
Cite this article
Xia, Y., Yang, M. & Wang, S. Chebyshev center of the intersection of balls: complexity, relaxation and approximation. Math. Program. 187, 287–315 (2021). https://doi.org/10.1007/s10107-020-01479-0
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10107-020-01479-0
Keywords
- Chebyshev center
- Minimax
- Nonconvex quadratic optimization
- Semidefinite programming
- Strong duality
- Linear programming
- Approximation
- Complexity