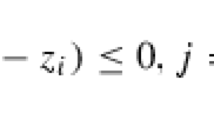Skip to main content
SpringerLink
Log in

On conic QPCCs, conic QCQPs and completely positive programs

  • Full Length Paper
  • Series A
  • Published:
Mathematical Programming Submit manuscript

Abstract

This paper studies several classes of nonconvex optimization problems defined over convex cones, establishing connections between them and demonstrating that they can be equivalently formulated as convex completely positive programs. The problems being studied include: a conic quadratically constrained quadratic program (QCQP), a conic quadratic program with complementarity constraints (QPCC), and rank constrained semidefinite programs. Our results do not make any boundedness assumptions on the feasible regions of the various problems considered. The first stage in the reformulation is to cast the problem as a conic QCQP with just one nonconvex constraint \(q(x) \le 0\), where q(x) is nonnegative over the (convex) conic and linear constraints, so the problem fails the Slater constraint qualification. A conic QPCC has such a structure; we prove the converse, namely that any conic QCQP satisfying a constraint qualification can be expressed as an equivalent conic QPCC. The second stage of the reformulation lifts the problem to a completely positive program, and exploits and generalizes a result of Burer. We also show that a Frank–Wolfe type result holds for a subclass of this class of conic QCQPs. Further, we derive necessary and sufficient optimality conditions for nonlinear programs where the only nonconvex constraint is a quadratic constraint of the structure considered elsewhere in the paper.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1

Similar content being viewed by others

References

  1. Alfakih, A.Y., Anjos, M.F., Piccialli, V., Wolkowicz, H.: Euclidean distance matrices, semidefinite programming, and sensor network localization. Portugal Math 68(1), 53–102 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  2. Alizadeh, F., Goldfarb, D.: Second-order cone programming. Math. Program. 95(1), 3–51 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  3. Anstreicher, K.M.: On convex relaxations for quadratically constrained quadratic programming. Math. Program. 136(2), 233–251 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  4. Auslender, A.: Existence of optimal solutions and duality results under weak conditions. Math. Program. 88, 45–59 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  5. Auslender, A., Teboulle, M.: Asymptotic Cones and Functions in Optimization and Variational Inequalities. Springer, New York (2003)

    MATH  Google Scholar 

  6. Bai, L., Mitchell, J.E., Pang, J.S.: On convex quadratic programs with linear complementarity constraints. Comput. Optim. Appl. 54(3), 517–554 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  7. Bai, L., Mitchell, J.E., Pang, J.S.: Using quadratic convex reformulation to tighten the convex relaxation of a quadratic program with complementarity constraints. Optim. Lett. 8(3), 811–822 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  8. Bao, X., Sahinidis, N.V., Tawarmalani, M.: Multiterm polyhedral relaxations for nonconvex, quadratically-constrained quadratic programs. Optim. Methods Softw. 24(4–5), 485–504 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  9. Bao, X., Sahinidis, N.V., Tawarmalani, M.: Semidefinite relaxations for quadratically constrained quadratic programs: a review and comparisons. Math. Program. 129(1), 129–157 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  10. Bauschke, H.H., Borwein, J.M., Li, W.: Strong conical hull intersection property, bounded linear regularity, Jameson’s property (G), and error bounds in convex optimization. Math. Program. 86, 135–160 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  11. Belousov, E.G.: Introduction to Convex Analysis and Integer Programming. Moscow University Publications, Moscow (1977). In Russian

    MATH  Google Scholar 

  12. Belousov, E.G., Klatte, D.: A Frank–Wolfe type theorem for convex polynomial programs. Comput. Optim. Appl. 22, 37–48 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  13. Bertsekas, D.P., Tseng, P.: Set intersection theorems and existence of optimal solutions. Math. Program. 110, 287–314 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  14. Bomze, I.M., de Klerk, E.: Solving standard quadratic optimization problems via linear, semidefinite and copositive programming. J. Global Optim. 24(2), 163–185 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  15. Bomze, I.M., Dür, M., de Klerk, E., Roos, C., Quist, A.J., Terlaky, T.: On copositive programming and standard quadratic optimization problems. J. Glob. Optim. 18(4), 301–320 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  16. Borwein, J.M., Lewis, A.S.: Convex Analysis and Nonlinear Optimization. Springer, New York (2000)

    Book  MATH  Google Scholar 

  17. Borwein, J.M., Wolkowicz, H.: A simple constraint qualification in infinite dimensional programming. Math. Program. 35, 83–96 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  18. Bundfuss, S., Dür, M.: Algorithmic copositivity detection by simplicial partition. Linear Algebra Appl. 428, 1511–1523 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  19. Bundfuss, S., Dür, M.: An adaptive linear approximation algorithm for copositive programs. SIAM J. Optim. 20(1), 30–53 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  20. Burachik, R.S., Jeyakumar, V.: A simple closure condition for the normal cone intersection formula. Proc. Am. Math. Soc. 133(6), 1741–1748 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  21. Burer, S.: On the copositive representation of binary and continuous nonconvex quadratic programs. Math. Program. 120(2), 479–495 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  22. Burer, S.: Optimizing a polyhedral-semidefinite relaxation of completely positive programs. Math. Prog. Comput. 2(1), 1–19 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  23. Burer, S.: Copositive programming. In: Anjos, M., Lasserre, J. (eds.) Handbook on Semidefinite, Conic and Polynomial Optimization, International Series in Operational Research and Management Science, pp. 201–218. Springer, Berlin (2012)

    Google Scholar 

  24. Burer, S.: A gentle, geometric introduction to copositive programming. Math. Program. 151(1), 89–116 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  25. Burer, S., Dong, H.: Representing quadratically constrained quadratic programs as generalized copositive programs. Operat. Res. Lett. 40(3), 203–206 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  26. Chandrasekaran, V., Sanghavi, S., Parrilo, P.A., Willsky, A.S.: Rank-sparsity incoherence for matrix decomposition. SIAM J. Optim. 21(2), 572–596 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  27. Chen, X., Ye, J.J.: A class of quadratic programs with linear complementarity constraints. Set Valued Variat. Anal. 17(2), 113–133 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  28. Codato, G., Fischetti, M.: Combinatorial Benders’ cuts for mixed-integer linear programming. Oper. Res. 54(4), 758–766 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  29. de Klerk, E., Pasechnik, D.V.: Approximation of the stability number of a graph via copositive programming. SIAM J. Optim. 12, 875–892 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  30. Dickinson, P.J.C.: The Copositive Cone, The Completely Positive Cone and Their Generalizations. PhD thesis, University of Groningen (2013)

  31. Dickinson, P.J.C., Eichfelder, G., Povh, J.: Erratum to: On the set-semidefinite representation of nonconvex quadratic programs over arbitrary feasible sets. Optim. Lett. 7(6), 1387–1397 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  32. Dickinson, P.J.C., Gijben, L.: On the computational complexity of membership problems for the completely positive cone and its dual. Comput. Optim. Appl. 57(2), 403–415 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  33. Ding, C., Sun, D., Ye, J.: First order optimality conditions for mathematical programs with semidefinite cone complementarity constraints. Math. Program. 147(1–2), 539–579 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  34. Dür, M.: Copositive programming—a survey. In: Diehl, M., Glineur, F., Jarlebring, E., Michiels, W. (eds.) Recent Advances in Optimization and its Applications in Engineering, pp. 3–20. Springer, Berlin (2010)

    Chapter  Google Scholar 

  35. Feng, M., Mitchell, J.E., Pang, J.S., Shen, X., Wächter, A.: Complementarity Formulations of l0-Norm Optimization Problems. Technical Report, Department of Mathematical Sciences, Rensselaer Polytechnic Institute, Troy, NY (2013)

  36. Frank, M., Wolfe, P.: An algorithm for quadratic programming. Nav. Res. Logist. Quart. 3, 149–154 (1956)

    Article  MathSciNet  Google Scholar 

  37. Goemans, M.X., Rendl, F.: Combinatorial optimization. In: Wolkowicz, H., Saigal, R., Vandenberghe, L. (eds.) Handbook of Semidefinite Programming, pp. 343–360. Kluwer, Dordrecht (2000)

    Chapter  Google Scholar 

  38. Goemans, M.X., Williamson, D.P.: Improved approximation algorithms for maximum cut and satisfiability problems using semidefinite programming. J. ACM 42(6), 1115–1145 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  39. Hu, J., Mitchell, J.E., Pang, J.S.: An LPCC approach to nonconvex quadratic programs. Math. Program. 133(1–2), 243–277 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  40. Hu, J., Mitchell, J.E., Pang, J.S., Bennett, K.P., Kunapuli, G.: On the global solution of linear programs with linear complementarity constraints. SIAM J. Optim. 19(1), 445–471 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  41. Jiang, H., Ralph, D.: QPECgen, a MATLAB generator for mathematical programs with quadratic objectives and affine variational inequality constraints. Comput. Optim. Appl. 13, 25–59 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  42. Lee, J., Zou, B.: Optimal rank-sparsity decomposition. J. Glob. Optim. 60(2), 307–315 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  43. Linderoth, J.T.: A simplicial branch-and-bound algorithm for solving quadratically constrained quadratic programs. Math. Program. 103(2), 251–282 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  44. Lu, C., Fang, S.-C., Jin, Q., Wang, Z., Xing, W.: KKT solution and conic relaxation for solving quadratically constrained quadratic programming problems. SIAM J. Optim. 21(4), 1475–1490 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  45. Lu, C., Jin, Q., Fang, S.-C., Wang, Z., Xing, W.: Adaptive Computable Approximation to Cones of Nonnegative Quadratic Functions. Technical Report, Department of Mathematical Sciences, Tsinghua University, Beijing, China, (2012)

  46. Luo, Z.-Q., Pang, J.S., Ralph, D.: Mathematical Programs with Equilibrium Constraints. Cambridge University Press, Cambridge (1996)

    Book  MATH  Google Scholar 

  47. Luo, Z.Q., Tseng, P.: Error bound and convergence analysis of matrix splitting algorithms for the affine variational inequality problem. SIAM J. Optim. 2(1), 43–54 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  48. Luo, Z.-Q., Zhang, S.: On extensions of the Frank–Wolfe theorems. Comput. Optim. Appl. 13(1–3), 87–110 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  49. Mitchell, J.E., Pang, J.S., Yu, B.: Convex quadratic relaxations of nonconvex quadratically constrained quadratic programs. Optim. Methods Softw. 29(1), 120–136 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  50. Outrata, J.V., Koçvara, M., Zowe, J.: Nonsmooth Approach to Optimization Problems with Equilibrium Constraints. Kluwer, Dordrecht (1998)

    Book  MATH  Google Scholar 

  51. Ozdaglar, A., Tseng, P.: Existence of global minima for constrained optimization. J. Optim. Theory Appl. 128, 523–546 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  52. Pang, J.S.: Three modeling paradigms in mathematical programming. Math. Program. 125(2), 297–323 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  53. Scheel, H., Scholtes, S.: Mathematical programs with complementarity constraints: stationarity, optimality, and sensitivity. Math. Operat. Res. 25(1), 1–22 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  54. Scholtes, S.: Nonconvex structures in nonlinear programming. Oper. Res. 52(3), 368–383 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  55. Sotirov, R.: SDP relaxations for some combinatorial optimization problems. In: Anjos, M., Lasserre, J. (eds.) Handbook on Semidefinite, Conic and Polynomial Optimization, International Series in Operational Research and Management Science, pp. 795–820. Springer, Berlin (2012)

    Google Scholar 

  56. Sponsel, J., Dür, M.: Factorization and cutting planes for completely positive matrices by copositive projection. Math. Program. 143(1–2), 211–229 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  57. Sturm, J.F., Zhang, S.: On cones of nonnegative quadratic functions. Math. Operat. Res. 28(2), 246–267 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  58. Terlaky, T.: On \(\ell _p\) programming. Eur. J. Oper. Res. 22, 70–100 (1985)

    Article  MathSciNet  Google Scholar 

  59. Yıldırım, E.A.: On the accuracy of uniform polyhedral approximations of the copositive cone. Optim. Methods Softw. 27(1), 155–173 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  60. Yoshise, A.: Complementarity problems over symmetric cones: A survey of recent developments in several aspects. In: Anjos, M.F., Lasserre, J.B. (eds.) Handbook on Semidefinite, Conic and Polynomial Optimization, pp. 339–376. Springer, Berlin (2012)

    Chapter  Google Scholar 

Download references

Acknowledgments

We would like to thank two anonymous referees for their careful reading of the manuscript and thoughtful comments.

Author information

Authors and Affiliations

  1. Optimization Toolbox, The MathWorks Inc., 3 Apple Hill Dr, Natick, MA, 01760, USA

    Lijie Bai

  2. Department of Mathematical Sciences, Rensselaer Polytechnic Institute, Troy, NY, 12180, USA

    John E. Mitchell

  3. Department of Industrial and Systems Engineering, University of Southern California, Los Angeles, CA, 90089, USA

    Jong-Shi Pang

Authors
  1. Lijie Bai
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. John E. Mitchell
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Jong-Shi Pang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to John E. Mitchell.

Additional information

The work of Bai and Mitchell was supported by the Air Force Office of Sponsored Research under Grant FA9550-11-1-0260 and by the National Science Foundation under Grant Number CMMI-1334327. The work of Pang was supported by the National Science Foundation under Grant Number CMMI-1333902 and by the Air Force Office of Scientific Research under Grant Number FA9550-11-1-0151.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Bai, L., Mitchell, J.E. & Pang, JS. On conic QPCCs, conic QCQPs and completely positive programs. Math. Program. 159, 109–136 (2016). https://doi.org/10.1007/s10107-015-0951-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10107-015-0951-9

Keywords

Mathematics Subject Classification

Search

Navigation