Skip to main content
SpringerLink
Log in

Circumcentered Methods Induced by Isometries

  • Original Article
  • Published:
Vietnam Journal of Mathematics Aims and scope Submit manuscript

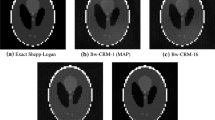
Abstract

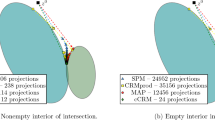
Motivated by the circumcentered Douglas–Rachford method recently introduced by Behling, Bello Cruz and Santos to accelerate the Douglas–Rachford method, we study the properness of the circumcenter mapping and the circumcenter method induced by isometries. Applying the demiclosedness principle for circumcenter mappings, we present weak convergence results for circumcentered isometry methods, which include the Douglas– Rachford method (DRM) and circumcentered reflection methods as special instances. We provide sufficient conditions for the linear convergence of circumcentered isometry/reflection methods. We explore the convergence rate of circumcentered reflection methods by considering the required number of iterations and as well as run time as our performance measures. Performance profiles on circumcentered reflection methods, DRM and method of alternating projections for finding the best approximation to the intersection of linear subspaces are presented.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

Notes

  1. Note that if \(\text {card}(\mathcal {S}(x))=1\), then dx = 0 and so \(CC_{\mathcal {S}}x=T_{1}x\).

  2. Note that if \(\text {card}(\mathcal {S}(x))=1\), then dx = 0 and so \(CC_{\mathcal {S}}x=T_{1}x\).

References

  1. Bauschke, H.H., Bello Cruz, J.Y., Nghia, T.T.A., Phan, H.M., Wang, X.: The rate of linear convergence of the Douglas–Rachford algorithm for subspaces is the cosine of the Friedrichs angle. J. Approx. Theory 185, 63–79 (2014)

    Article  MathSciNet  Google Scholar 

  2. Bauschke, H.H., Borwein, J.M.: On projection algorithms for solving convex feasibility problems. SIAM Rev. 38, 367–426 (1996)

    Article  MathSciNet  Google Scholar 

  3. Bauschke, H.H., Combettes, P.L.: Convex Analysis and Monotone Operator Theory in Hilbert Spaces, 2nd edn. CMS Books in Mathematics. Springer, Cham (2017)

  4. Bauschke, H.H., Ouyang, H., Wang, X.: On circumcenters of finite sets in Hilbert spaces. Linear Nonlinear Anal. 4, 271–295 (2018)

    MathSciNet  Google Scholar 

  5. Bauschke, H.H., Ouyang, H., Wang, X.: On circumcenter mappings induced by nonexpansive operators. arXiv:1811.11420 (2018)

  6. Bauschke, H.H., Ouyang, H., Wang, X.: On the linear convergence of circumcentered isometry methods. arXiv:1912.01063 (2019)

  7. Behling, R., Bello Cruz, J.Y., Santos, L.-R.: Circumcentering the Douglas–Rachford method. Numer. Algor. 78, 759–776 (2018)

    Article  MathSciNet  Google Scholar 

  8. Behling, R., Bello Cruz, J.Y., Santos, L.-R.: On the linear convergence of the circumcentered-reflection method. Oper. Res. Lett. 46, 159–162 (2018)

    Article  MathSciNet  Google Scholar 

  9. Behling, R., Bello Cruz, J.Y., Santos, L.-R.: The block-wise circumcentered-reflection method. Comput. Optim. Appl. 76, 675–699 (2020)

    Article  MathSciNet  Google Scholar 

  10. Dizon, N., Hogan, J., Lindstrom, S.B.: Circumcentering reflection methods for nonconvex feasibility problems. arXiv:1910.04384 (2019)

  11. Deutsch, F.: Best Approximation in Inner Product Spaces. CMS Books in Mathematics. Springer-Verlag, New York (2012)

    Google Scholar 

  12. Dolan, E.D., Moré, J.J.: COPS. http://www.mcs.anl.gov/more/cops/

  13. Dolan, E.D., Moré, J. J.: Benchmarking optimization software with performance profiles. Math. Program 91, 201–213 (2002)

    Article  MathSciNet  Google Scholar 

  14. Gearhart, W.B., Koshy, M.: Acceleration schemes for the method of alternating projections. J. Comput. Appl. Math 26, 235–249 (1989)

    Article  MathSciNet  Google Scholar 

  15. Kreyszig, E.: Introductory Functional Analysis with Applications. John Wiley & Sons, Inc., New York (1989)

  16. Lindstrom, S.B.: Computable centering methods for spiraling algorithms and their duals, with motivations from the theory of Lyapunov functions. arXiv:2001.10784 (2020)

  17. Meyer, C.: Matrix Analysis and Applied Linear Algebra. SIAM Philadelphia, PA (2000)

Download references

Acknowledgements

The authors thank two anonymous referees and the editors for their constructive comments and professional handling of the manuscript. HHB and XW were partially supported by NSERC Discovery Grants.

Author information

Authors and Affiliations

  1. Mathematics, University of British Columbia, Kelowna, B.C. V1V 1V7, Canada

    Heinz H. Bauschke, Hui Ouyang & Xianfu Wang

Authors
  1. Heinz H. Bauschke
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Hui Ouyang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Xianfu Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Heinz H. Bauschke.

Additional information

Publisher’s Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Dedicated to Professor Marco López on the occasion of his 70th birthday.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Bauschke, H.H., Ouyang, H. & Wang, X. Circumcentered Methods Induced by Isometries. Vietnam J. Math. 48, 471–508 (2020). https://doi.org/10.1007/s10013-020-00417-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10013-020-00417-z

Keywords

Mathematics Subject Classification (2010)

Search

Navigation