Passive vibration absorbers for vibration reduction in the multi-bladed rotor with rotor and stator contact

Abstract

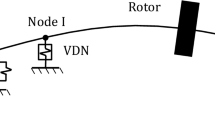
Contact phenomena are among the most undesirable incidences in a rotor dynamic system where its emergence could be due to the centrifugal force generated by an unbalance disk. In this work, a Jeffcott rotor supported by oil film journal bearings and with rigid blades is taken into account. The long oil film lubrication is considered where the axial flow is negligible. The rotational speed is taken as a control parameter, and the analysis is performed for two different clearances considering the rotor with three, four, and five blades. To reduce the vibrations of the system with the purpose of contact elimination, a tuned mass damper and a nonlinear energy sink as passive absorbers are utilized. Then, by an optimization process applying a complex averaging method, the optimum parameters for each absorber are calculated. Due to the critical behavior of the system in the lower clearance values, double coupling of the absorbers is suggested. The results demonstrate that single coupling of the absorbers can considerably reduce the vibration of the system. On the other hand, for the lower clearance value single coupling is not efficient, and double coupling could be utilized to mitigate the unwanted vibrations of the system.

Appendices

Appendix A

(a) The equations of motion for single TMD in complex averaging form

Dynamic part:

$$\begin{aligned}&a_{1}^{'}\left( 1+M_{bl} \right) +a_{1}^{'}e_{2}-\frac{b_{1}\omega }{2}+a_{1}\zeta _{1}+\frac{b_{1}}{2\omega }-\frac{e_{2}\omega ^{2}q_{03}}{2}-\frac{M_{bl}b_{\mathrm {1}}\omega }{\mathrm {2}}-\frac{b_{1}e_{2}\omega }{2}\nonumber \\&\quad =\frac{G\cos H}{24}+\frac{{\bar{\epsilon }}\omega ^{2}\,\cos \, \beta }{2}+\frac{{\bar{R}}_{s}e_{2}\omega ^{2}}{2},\nonumber \\&a_{2}^{'}\left( 1+M_{bl} \right) \, +a_{2}^{'}e_{2}-\frac{b_{2}\omega }{2}+\, a_{2}\zeta _{1}-\frac{M_{bl}b_{\mathrm {2}}\omega }{\mathrm {2}}-\frac{b_{2}e_{2}\omega }{2} =\frac{\bar{\epsilon }\omega ^{2}\,\sin \, \beta }{2}+\frac{G\sin H}{24},\nonumber \\&a_{3}^{'}e_{2}+a_{3}\zeta _{3}-b_{3}e_{2}\omega +\frac{b_{3}{\bar{k}}_{T}}{2\omega }=0,\nonumber \\&b_{1}^{'}\left( 1+M_{bl} \right) +b_{1}^{'}e_{2}+\frac{a_{1}\omega }{2}+b_{1}\zeta _{1}-\frac{a_{1}}{2\omega }+\frac{M_{bl}a_{\mathrm {1}}\omega }{\mathrm {2}}+\frac{a_{1}e_{2}\omega }{2} =\frac{\bar{\epsilon }\omega ^{2}\,\sin \, \beta }{2}+\frac{G\sin H}{24},\nonumber \\&b_{2}^{'}\left( 1+M_{bl} \right) +b_{2}^{'}e_{2}+\frac{a_{2}\omega }{2}+b_{2}\zeta _{1}-\frac{a_{1}}{2\omega }+\frac{e_{2}\omega ^{2}q_{03}}{2}\nonumber \\&\qquad +\frac{M_{bl}a_{\mathrm {2}}\omega }{\mathrm {2}}+\frac{a_{2}e_{2}\omega }{2}=-\frac{G\cos H}{24}-\frac{{\bar{\epsilon }}\omega ^{2}\,\cos \, \beta }{2}-\frac{{\bar{R}}_{s}e_{2}\omega ^{2}}{2},\nonumber \\&b_{3}^{'}e_{2}+b_{3}\zeta _{3}+a_{3}e_{2}\omega -\frac{a_{3}{\bar{k}}_{T}}{2\omega }=0. \end{aligned}$$

(A.1)

Static part:

$$\begin{aligned} q_{01}= & {} 0,\nonumber \\ q_{02}= & {} -\delta _{st},\nonumber \\ {\bar{k}}_{T}q_{03}-e_{2}\omega ^{2}q_{03}-\frac{a_{2}e_{2}\omega }{2}-\frac{b_{1}e_{2}\omega }{2}= & {} \frac{{\bar{R}}_{s}e_{2}\omega ^{2}}{2}. \end{aligned}$$

(A.2)

(b) The equations of motion for single NES in complex averaging form

Dynamic part:

$$\begin{aligned}&a_{\mathrm {1}}^{\mathrm {'}}\left( \mathrm {1+}M_{bl} \right) +a_{\mathrm {1}}^{\mathrm {'}}e_{\mathrm {2}}-\frac{b_{\mathrm {1}}\omega }{\mathrm {2}}+a_{\mathrm {1}}\zeta _{\mathrm {1}}+\frac{b_{\mathrm {1}}}{\mathrm {2}\omega }-\frac{e_{\mathrm {2}}\omega ^{\mathrm {2}}q_{\mathrm {03}}}{\mathrm {2}}-\frac{M_{bl}b_{\mathrm {1}}\omega }{\mathrm {2}}-\frac{b_{\mathrm {1}}e_{\mathrm {2}}\omega }{\mathrm {2}}\nonumber \\&\quad =\frac{G\,\cos \, H}{\mathrm {24}}+\frac{\bar{\epsilon }\omega ^{\mathrm {2}}\,\cos \, \beta }{\mathrm {2}}+\frac{{\bar{R}}_{s}e_{\mathrm {2}}\omega ^{\mathrm {2}}}{\mathrm {2}},\nonumber \\&a_{\mathrm {2}}^{\mathrm {'}}\left( \mathrm {1+}M_{bl} \right) \, +a_{\mathrm {2}}^{\mathrm {'}}e_{\mathrm {2}}-\frac{b_{\mathrm {2}}\omega }{\mathrm {2}}\mathrm {+\, }a_{\mathrm {2}}\zeta _{\mathrm {1}}+\frac{b_{\mathrm {2}}}{\mathrm {2}\omega }-\frac{M_{bl}b_{\mathrm {2}}\omega }{\mathrm {2}}-\frac{b_{\mathrm {2}}e_{\mathrm {2}}\omega }{\mathrm {2}}=\frac{{\bar{\epsilon }}\omega ^{\mathrm {2}} \,\sin \, \beta }{\mathrm {2}}+\frac{G\,\sin \, H}{\mathrm {24}},\nonumber \\&a_{\mathrm {3}}^{\mathrm {'}}e_{\mathrm {2}}+a_{\mathrm {3}}\zeta _{\mathrm {3}}+\frac{\mathrm {3}b_{\mathrm {3}}^{\mathrm {3}}{\bar{k}}_{N}}{\mathrm {8}\omega ^{\mathrm {3}}}-b_{\mathrm {3}}e_{\mathrm {2}}\omega +\frac{\mathrm {3}a_{\mathrm {3}}^{\mathrm {2}}b_{\mathrm {3}}{\bar{k}}_{N}}{\mathrm {8}\omega ^{\mathrm {3}}}+\frac{\mathrm {3}b_{\mathrm {3}}{\bar{k}}_{N}q_{\mathrm {03}}^{\mathrm {2}}}{\mathrm {2}\omega }=0,\nonumber \\&b_{\mathrm {1}}^{\mathrm {'}}\left( \mathrm {1+}M_{bl} \right) +b_{\mathrm {1}}^{\mathrm {'}}e_{\mathrm {2}}+\frac{a_{\mathrm {1}}\omega }{\mathrm {2}}+b_{\mathrm {1}}\zeta _{\mathrm {1}}-\frac{a_{\mathrm {1}}}{\mathrm {2}\omega }+\frac{M_{bl}a_{\mathrm {1}}\omega }{\mathrm {2}}+\frac{a_{\mathrm {1}}e_{\mathrm {2}}\omega }{\mathrm {2}}=\frac{{\bar{\epsilon }}\omega ^{\mathrm {2}}\,\textit{sin}\, \beta }{\mathrm {2}}+\frac{G\,\textit{sin}\, H}{\mathrm {24}},\nonumber \\&b_{\mathrm {2}}^{\mathrm {'}}\left( \mathrm {1+}M_{bl} \right) +b_{\mathrm {2}}^{\mathrm {'}}e_{\mathrm {2}}+\frac{a_{\mathrm {2}}\omega }{\mathrm {2}}+b_{\mathrm {2}}\zeta _{\mathrm {1}}-\frac{a_{\mathrm {2}}}{\mathrm {2}\omega }+\frac{e_{\mathrm {2}}\omega ^{\mathrm {2}}q_{\mathrm {03}}}{\mathrm {2}}+\frac{M_{bl}a_{\mathrm {2}}\omega }{\mathrm {2}}+\frac{a_{\mathrm {2}}e_{\mathrm {2}}\omega }{\mathrm {2}}\nonumber \\&\quad =-\frac{G\,\cos \, H}{\mathrm {24}}-\frac{\bar{\epsilon }\omega ^{\mathrm {2}}\,\cos \, \beta }{\mathrm {2}}-\frac{{\bar{R}}_{s}e_{\mathrm {2}}\omega ^{\mathrm {2}}}{\mathrm {2}},\nonumber \\&b_{\mathrm {3}}^{\mathrm {'}}e_{\mathrm {2}}+b_{\mathrm {3}}\zeta _{\mathrm {3}}\, -\frac{\mathrm {3}a_{\mathrm {3}}^{\mathrm {3}}{\bar{k}}_{N}}{\mathrm {8}\omega ^{\mathrm {3}}}+a_{\mathrm {3}}e_{\mathrm {2}}\omega -\frac{\mathrm {3}a_{\mathrm {3}}b_{\mathrm {3}}^{\mathrm {2}}{\bar{k}}_{N}}{\mathrm {8}\omega ^{\mathrm {3}}}-\frac{\mathrm {3}a_{\mathrm {3}}{\bar{k}}_{N}q_{\mathrm {03}}^{\mathrm {2}}}{\mathrm {2}\omega }=0. \end{aligned}$$

(A.3)

Static part:

$$\begin{aligned} q_{01}= & {} 0,\nonumber \\ q_{02}= & {} -\delta _{st},\nonumber \\ {\bar{k}}_{N}q_{03}^{3}-e_{2}\omega ^{2}q_{03}-\frac{a_{2}e_{\mathrm {2}}\omega }{2}-\frac{b_{1}e_{\mathrm {2}}\omega }{2}+\frac{3a_{3}^{2}{\bar{k}}_{N}q_{03}}{2\omega ^{2}}+\frac{3b_{3}^{2}{\bar{k}}_{N}q_{03}}{2\omega ^{2}}= & {} \frac{{\bar{R}}_{s}e_{\mathrm {2}}\omega ^{2}}{2}. \end{aligned}$$

(A.4)

(c) The equations of motion for double TMD in complex averaging form

Dynamic part:

$$\begin{aligned}&a_{1}^{'}\left( 1+M_{bl} \right) +a_{1}^{'}\left( e_{2}+e_{3} \right) -\frac{b_{1}\omega }{2}+a_{1}\zeta _{1}+\frac{b_{1}}{2\omega }-\frac{M_{bl}b_{\mathrm {1}}\omega }{\mathrm {2}}-\frac{b_{1}\omega }{2}\left( e_{2}+e_{3} \right) \nonumber \\&\quad -\frac{\omega ^{2}}{2}\left( q_{03}e_{2}\cos \beta _{1}+q_{04}e_{3}\cos \beta _{2} \right) =\frac{G\cos H}{24}+\frac{{\bar{\epsilon }}\omega ^{2}}{2}+\frac{{\bar{R}}_{s}\omega ^{2}}{2}\left( e_{2}\cos \beta _{1}+e_{3}\cos \beta _{2} \right) ,\nonumber \\&a_{2}^{'}\left( 1+M_{bl} \right) \, +a_{2}^{'}\left( e_{2}+e_{3} \right) -\frac{b_{2}\omega }{2}+\, a_{2}\zeta _{1}+\frac{b_{2}}{2\omega }-\frac{M_{bl}b_{\mathrm {2}}\omega }{\mathrm {2}}-\frac{b_{2}\omega }{2}\left( e_{2}+e_{3} \right) \nonumber \\&\quad -\frac{\omega ^{2}q_{03}}{2}\left( q_{03}e_{2}\sin \beta _{1}+q_{04}e_{3}\sin \beta _{2} \right) =\frac{G\sin H}{24}+\frac{{\bar{R}}_{s}\omega ^{2}}{2}\left( e_{2}\sin \beta _{1}+e_{3}\sin \beta _{2} \right) ,\nonumber \\&a_{3}^{'}e_{2}+a_{3}\zeta _{3}-b_{3}e_{2}\omega +\frac{b_{3}{\bar{k}}_{T1}}{2\omega }=0,\nonumber \\&a_{4}^{'}e_{3}+a_{4}\zeta _{4}-b_{4}e_{3}\omega +\frac{b_{4}{\bar{k}}_{T2}}{2\omega }=0,\nonumber \\&b_{1}^{'}\left( 1+M_{bl} \right) +b_{1}^{'}\left( e_{2}+e_{3} \right) +\frac{a_{1}\omega }{2}+b_{1}\zeta _{1}-\frac{a_{1}}{2\omega }+\frac{M_{bl}a_{\mathrm {1}}\omega }{\mathrm {2}}+\frac{a_{1}\omega }{2}\left( e_{2}+e_{3} \right) \nonumber \\&\quad -\frac{\omega ^{2}}{2}\left( q_{03}e_{2}\sin \beta _{1}+q_{04}e_{3}\sin \beta _{2} \right) =\frac{G\sin H}{24}+\frac{{\bar{R}}_{s}\omega ^{2}}{2}\left( e_{2}\sin \beta _{1}+e_{3}\sin \beta _{2} \right) ,\nonumber \\&b_{2}^{'} \left( 1+M_{bl} \right) +b_{2}^{'}\left( e_{2}+e_{3} \right) +\frac{a_{2}\omega }{2}+b_{2}\zeta _{1}-\frac{a_{2}}{2\omega }+\frac{M_{bl}a_{\mathrm {2}}\omega }{\mathrm {2}}+\frac{a_{2}\omega }{2}\left( e_{2}+e_{3} \right) \nonumber \\&\quad +\frac{\omega ^{2}}{2}\left( q_{03}e_{2}\cos \beta _{1}+q_{04}e_{3}\cos \beta _{2} \right) =-\frac{G\cos H}{24}-\frac{{\bar{\epsilon }}\omega ^{2}}{2}-\frac{{\bar{R}}_{s}\omega ^{2}}{2}\left( e_{2}\cos \beta _{1}+e_{3}\cos \beta _{2} \right) ,\nonumber \\&b_{3}^{'}e_{2}+b_{3}\zeta _{3}+a_{3}e_{2}\omega -\frac{a_{3}{\bar{k}}_{T1}}{2\omega }=0,\nonumber \\&b_{4}^{'}e_{3}+b_{4}\zeta _{4}+a_{4}e_{3}\omega -\frac{a_{4}{\bar{k}}_{T2}}{2\omega }=0. \end{aligned}$$

(A.5)

Static part:

$$\begin{aligned}&q_{01}=0,\nonumber \\&q_{02}=-\delta _{st},\nonumber \\&{\bar{k}}_{T1}q_{03}-e_{2}\omega ^{2}q_{03}-\frac{a_{2}e_{2}\omega \cos \beta _{1}}{2}-\frac{b_{1}e_{2}\omega \cos \beta _{1}}{2}+\frac{a_{1}e_{2}\omega \sin \beta _{1}}{2}-\frac{b_{2}e_{2}\omega \sin \beta _{1}}{2}=\frac{{\bar{R}}_{s}e_{2}\omega ^{2}}{2},\nonumber \\&{\bar{k}}_{T2}q_{04}-e_{3}\varvec{\omega }^{2}q_{04}-\frac{a_{2}e_{3}\varvec{\omega }\cos \beta _{1}}{2}-\frac{b_{1}e_{3}\varvec{\omega }\cos \beta _{1}}{2}+\frac{a_{1}e_{3}\varvec{\omega }\sin \beta _{1}}{2}-\frac{b_{2}e_{3}\varvec{\omega }\sin \beta _{1}}{2}=\frac{{\bar{R}}_{s}e_{3}\varvec{\omega }^{2}}{2}. \end{aligned}$$

(A.6)

(d) The equations of motion for double NES in complexification averaging form

Dynamic part:

$$\begin{aligned}&\left( 1+M_{bl} \right) +a_{1}^{'}\left( e_{2}+e_{3} \right) -\frac{b_{1}\varvec{\omega }}{2}+a_{1}\zeta _{1}+\frac{b_{1}}{2\varvec{\omega }}-\frac{M_{bl}b_{\mathrm {1}}\varvec{\omega }}{\mathrm {2}}-\frac{b_{1}\varvec{\omega }}{2}\left( e_{2}+e_{3} \right) \nonumber \\&\quad -\frac{\varvec{\omega }^{2}}{2}\left( q_{03}e_{2}\cos \beta _{1}+q_{04}e_{3}\cos \beta _{2} \right) =\frac{G\cos H}{24}+\frac{{\bar{\epsilon }}\varvec{\omega }^{2}}{2}+\frac{{\bar{R}}_{s}\varvec{\omega }^{2}}{2}\left( e_{2}\cos \beta _{1}+e_{3}\cos \beta _{2} \right) ,\nonumber \\&a_{2}^{'}\left( 1+M_{bl} \right) \, +a_{2}^{'}\left( e_{2}+e_{3} \right) -\frac{b_{2}\varvec{\omega }}{2}+\, a_{2}\zeta _{1}+\frac{b_{2}}{2\varvec{\omega }}-\frac{M_{bl}b_{\mathrm {2}}\varvec{\omega }}{\mathrm {2}}-\frac{b_{2}\varvec{\omega }}{2}\left( e_{2}+e_{3} \right) \nonumber \\&-\frac{\varvec{\omega }^{2}}{2}\left( q_{03}e_{2}\sin \beta _{1}+q_{04}e_{3}\sin \beta _{2} \right) =\frac{G\sin H}{24}+\frac{{\bar{R}}_{s}\varvec{\omega }^{2}}{2}\left( e_{2}\sin \beta _{1}+e_{3}\sin \beta _{2} \right) ,\nonumber \\&a_{3}^{'}e_{2}+a_{3}\zeta _{3}+\frac{\mathrm {3}b_{\mathrm {3}}^{\mathrm {3}}{\bar{k}}_{N\mathrm {1}}}{\mathrm {8}\varvec{\omega }^{\mathrm {3}}}-b_{3}e_{2}\varvec{\omega }+\frac{\mathrm {3}\mathrm {a}_{\mathrm {3}}^{\mathrm {2}}b_{3}{\bar{k}}_{N\mathrm {1}}}{\mathrm {8}\varvec{\omega }^{\mathrm {3}}}+\frac{\mathrm {3}b_{\mathrm {3}}q_{03}^{2}{\bar{k}}_{N\mathrm {1}}}{\mathrm {2}\varvec{\omega }}=0,\nonumber \\&a_{4}^{'}e_{3}+a_{4}\zeta _{4}+\frac{\mathrm {3}b_{\mathrm {4}}^{\mathrm {3}}{\bar{k}}_{N\mathrm {2}}}{\mathrm {8}\varvec{\omega }^{\mathrm {3}}}-b_{4}e_{3}\varvec{\omega }+\frac{\mathrm {3}\mathrm {a}_{\mathrm {4}}^{\mathrm {2}}b_{4}{\bar{k}}_{N2}}{\mathrm {8}\varvec{\omega }^{\mathrm {3}}}+\frac{\mathrm {3}b_{4}q_{04}^{2}{\bar{k}}_{N\mathrm {2}}}{\mathrm {2}\varvec{\omega }}=0,\nonumber \\&b_{1}^{'}\left( 1+M_{bl} \right) +b_{1}^{'}\left( e_{2}+e_{3} \right) +\frac{a_{1}\varvec{\omega }}{2}+b_{1}\zeta _{1}-\frac{a_{1}}{2\varvec{\omega }}+\frac{M_{bl}a_{\mathrm {1}}\varvec{\omega }}{\mathrm {2}}+\frac{a_{1}\varvec{\omega }}{2}\left( e_{2}+e_{3} \right) \nonumber \\&\quad -\frac{\varvec{\omega }^{2}}{2}\left( q_{03}e_{2}\sin \beta _{1}+q_{04}e_{3}\sin \beta _{2} \right) =\frac{G\sin H}{24}+\frac{{\bar{R}}_{s}\varvec{\omega }^{2}}{2}\left( e_{2}\sin \beta _{1}+e_{3}\sin \beta _{2} \right) ,\nonumber \\&b_{2}^{'}\left( 1+M_{bl} \right) +b_{2}^{'}\left( e_{2}+e_{3} \right) +\frac{a_{2}\varvec{\omega }}{2}+b_{2}\zeta _{1}-\frac{a_{2}}{2\varvec{\omega }}+\frac{M_{bl}a_{\mathrm {2}}\varvec{\omega }}{\mathrm {2}}+\frac{a_{2}\varvec{\omega }}{2}\left( e_{2}+e_{3} \right) \nonumber \\&+\frac{\varvec{\omega }^{2}}{2}\left( q_{03}e_{2}\cos \beta _{1}+q_{04}e_{3}\cos \beta _{2} \right) =-\frac{G\cos H}{24}-\frac{{\bar{\epsilon }}\varvec{\omega }^{2}}{2}-\frac{{\bar{R}}_{s}\varvec{\omega }^{2}}{2}\left( e_{2}\cos \beta _{1}+e_{3}\cos \beta _{2} \right) ,\nonumber \\&b_{\mathrm {3}}^{\mathrm {'}}e_{\mathrm {2}}+b_{\mathrm {3}}\zeta _{\mathrm {3}}\, -\frac{\mathrm {3}a_{\mathrm {3}}^{\mathrm {3}}{\bar{k}}_{N\mathrm {1}}}{\mathrm {8}\varvec{\omega }^{\mathrm {3}}}+a_{\mathrm {3}}e_{\mathrm {2}}\varvec{\omega }-\frac{\mathrm {3}a_{\mathrm {3}}b_{\mathrm {3}}^{\mathrm {2}}{\bar{k}}_{N\mathrm {1}}}{\mathrm {8}\varvec{\omega }^{\mathrm {3}}}-\frac{\mathrm {3}a_{\mathrm {3}}{\bar{k}}_{N\mathrm {1}}q_{\mathrm {03}}^{\mathrm {2}}}{\mathrm {2}\varvec{\omega }}=0,\nonumber \\&b_{\mathrm {4}}^{\mathrm {'}}e_{\mathrm {3}}+b_{4}\zeta _{\mathrm {4}}\, -\frac{\mathrm {3}a_{4}^{\mathrm {3}}{\bar{k}}_{N25}}{\mathrm {8}\varvec{\omega }^{\mathrm {3}}}+a_{4}e_{3}\varvec{\omega }-\frac{\mathrm {3}a_{4}b_{4}^{\mathrm {2}}{\bar{k}}_{N\mathrm {2}}}{\mathrm {8}\varvec{\omega }^{\mathrm {3}}}-\frac{\mathrm {3}a_{\mathrm {4}}{\bar{k}}_{N\mathrm {2}}q_{\mathrm {04}}^{\mathrm {2}}}{\mathrm {2}\varvec{\omega }}=0. \end{aligned}$$

(A.7)

Static part:

$$\begin{aligned}&q_{01}=0,\nonumber \\&q_{02}=-\delta _{st},\nonumber \\&{\bar{k}}_{N1}q_{03}^{3}-e_{2}\varvec{\omega }^{2}q_{03}-\frac{a_{2}e_{\mathrm {2}}\varvec{\omega }\cos \beta _{1}}{2}-\frac{b_{1}e_{\mathrm {2}}\varvec{\omega }\cos \beta _{1}}{2}+\frac{a_{1}e_{\mathrm {2}}\varvec{\omega }\sin \beta _{1}}{2}-\frac{b_{2}e_{\mathrm {2}}\varvec{\omega }\sin \beta _{1}}{2}\nonumber \\&\quad +\frac{3a_{3}^{2}{\bar{k}}_{N1}q_{03}}{2\varvec{\omega }^{2}}+\frac{3b_{3}^{2}{\bar{k}}_{N1}q_{03}}{2\varvec{\omega }^{2}}=\frac{{\bar{R}}_{s}e_{\mathrm {2}}\varvec{\omega }^{2}}{2},\nonumber \\&{\bar{k}}_{N2}q_{04}^{3}-e_{3}\varvec{\omega }^{2}q_{04}-\frac{a_{2}e_{\mathrm {3}}\varvec{\omega }\cos \beta _{2}}{2}-\frac{b_{1}e_{\mathrm {3}}\varvec{\omega }\cos \beta _{2}}{2}+\frac{a_{1}e_{\mathrm {3}}\varvec{\omega }\sin \beta _{1}}{2}-\frac{b_{2}e_{\mathrm {3}}\varvec{\omega }\sin \beta _{1}}{2}\nonumber \\&\quad +\frac{3a_{4}^{2}{\bar{k}}_{N1}q_{04}}{2\varvec{\omega }^{2}}+\frac{3b_{4}^{2}{\bar{k}}_{N1}q_{04}}{2\varvec{\omega }^{2}}=\frac{{\bar{R}}_{s}e_{\mathrm {3}}\varvec{\omega }^{2}}{2}. \end{aligned}$$

(A.8)

Appendix B

In this Section, a review of the concepts of dominating stiffness, damping, and inertia regions in the frequency response function of a linear single-degree-of-freedom vibration system is considered. The frequency response of a one-degree freedom mass–spring–damper under an external harmonic force has the following form, which is shown in Fig. 17:

$$\begin{aligned} \frac{X}{F}=\frac{1}{\sqrt{\left( k-m\omega ^{2} \right) +\left( c\omega \right) ^{2}} }=\frac{1}{k}\frac{1}{\sqrt{\left( 1-\Omega ^{2} \right) +\left( 2\zeta \Omega \right) ^{2}} },\, \Omega =\frac{\omega }{\omega _{n}},\, \zeta =\frac{c}{2m\omega _{n}},\, \omega _{n}=\sqrt{\frac{k}{m}}. \end{aligned}$$

(B.1)

Fig. 17

The concepts of dominating stiffness, damping, and inertia regions in the frequency response function of a linear single-degree-of-freedom vibration system

In low dimensionless frequencies, for example in the domain of $0\le \Omega \le 0.3$, $1-\Omega ^{2}\approx \, 1$, and $\frac{X}{F}\approx \frac{1}{k}$, the force of the spring is the dominant force. Accordingly, in this region increasing or decreasing the stiffness of spring value, the amount of displacement can be decreased or increased as well. In a damping dominant region, for example in the domain of $0.3\le \Omega \le 1.5$, the force of the damper is the dominant force. Adjusting the damping coefficient in this region, the amount of displacement, especially in the resonance frequency, can be controlled. In this region, the vibration amplitude is approximately $X\approx F/C\omega$. For $\Omega \ge 1.5$, the inertia force is dominating whereby increasing the actuating frequency the amplitude of vibration is approximately given by $X\approx F/m\omega ^{2}$.