Skip to main content
SpringerLink
Log in

Fractional single-phase lag heat conduction and transient thermal fracture in cracked viscoelastic materials

  • Original Paper
  • Published:
Acta Mechanica Aims and scope Submit manuscript

Abstract

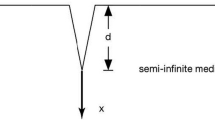
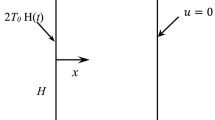
In the present article, a thermo-viscoelastic model is developed to investigate fractional single-phase lag heat conduction and the associated transient thermal mechanical behavior of a cracked viscoelastic material under a thermal shock. To avoid the negative temperature distribution around cracks, which violates the second law of thermodynamics, the time-fractional single-phase lag heat conduction is introduced to analyze the transient temperature field around the cracks. The Fourier and Laplace transforms, coupled with the singular integral equations, are employed to solve the governing partial differential equations numerically. Both the results of temperature field and stress intensity factors (SIFs) show that the fractional single-phase lag heat conduction model is more accurate and reasonable compared to the conventional hyperbolic heat conduction. A significant difference in transient fracture behavior exists between viscoelastic and elastic materials. A sharp pulse of the SIFs at the early stage is observed and should be consider carefully to meet the requirement of increased application of viscoelastic composites under thermal loading.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10

Similar content being viewed by others

References

  1. Drury, J.L., Mooney, D.J.: Hydrogels for tissue engineering: scaffold design variables and applications. Biomaterials 24(24), 4337–4351 (2003)

    Google Scholar 

  2. Guo, M., Pitet, L.M., Wyss, H.M., Vos, M., Dankers, P.Y., Meijer, E.: Tough stimuli-responsive supramolecular hydrogels with hydrogen-bonding network junctions. J. Am. Chem. Soc. 136(19), 6969–6977 (2014)

    Google Scholar 

  3. Luo, F., Sun, T.L., Nakajima, T., Kurokawa, T., Zhao, Y., Sato, K., Ihsan, A.B., Li, X., Guo, H., Gong, J.P.: Oppositely charged polyelectrolytes form tough, self-healing, and rebuildable hydrogels. Adv. Mater. 27(17), 2722–2727 (2015)

    Google Scholar 

  4. Sun, J.-Y., Zhao, X., Illeperuma, W.R., Chaudhuri, O., Oh, K.H., Mooney, D.J., Vlassak, J.J., Suo, Z.: Highly stretchable and tough hydrogels. Nature 489(7414), 133 (2012)

    Google Scholar 

  5. Haag, S., Bernards, M.: Polyampholyte hydrogels in biomedical applications. Gels 3(4), 41 (2017)

    Google Scholar 

  6. Haraguchi, K.: Nanocomposite hydrogels. Curr. Opin. Solid State Mater. Sci. 11(3–4), 47–54 (2007)

    Google Scholar 

  7. Guedes, R.: Durability of polymer matrix composites: viscoelastic effect on static and fatigue loading. Compos. Sci. Technol. 67(11–12), 2574–2583 (2007)

    Google Scholar 

  8. Zhai, S., Zhang, P., Xian, Y., Zeng, J., Shi, B.: Effective thermal conductivity of polymer composites: theoretical models and simulation models. Int. J. Heat. Mass. Transf. 117, 358–374 (2018)

    Google Scholar 

  9. Chen, H., Ginzburg, V.V., Yang, J., Yang, Y., Liu, W., Huang, Y., Du, L., Chen, B.: Thermal conductivity of polymer-based composites: fundamentals and applications. Prog. Polym. Sci. 59, 41–85 (2016)

    Google Scholar 

  10. Ji, H., Sellan, D.P., Pettes, M.T., Kong, X., Ji, J., Shi, L., Ruoff, R.S.: Enhanced thermal conductivity of phase change materials with ultrathin-graphite foams for thermal energy storage. Energy Environ. Sci. 7(3), 1185–1192 (2014)

    Google Scholar 

  11. Li, X., Li, C., Xue, Z., Tian, X.: Analytical study of transient thermo-mechanical responses of dual-layer skin tissue with variable thermal material properties. Int. J. Therm. Sci. 124, 459–466 (2018)

    Google Scholar 

  12. Van Hees, J., Gybels, J.: C nociceptor activity in human nerve during painful and non painful skin stimulation. J. Neurol. Neurosurg. Psychiatry 44(7), 600–607 (1981)

    Google Scholar 

  13. Liu, Y.J., Xu, N.: Modeling of interface cracks in fiber-reinforced composites with the presence of interphases using the boundary element method. Mech. Mater. 32(12), 769–783 (2000)

    Google Scholar 

  14. Zhi-He, J., Naotake, N.: Transient thermal stress intensity factors for a crack in a semi-infinite plate of a functionally gradient material. Int. J. Solids Struct. 31(2), 203–218 (1994)

    MATH  Google Scholar 

  15. Erdogan, F., Wu, B.: The surface crack problem for a plate with functionally graded properties. J. Appl. Mech. 64(3), 449–456 (1997)

    MATH  Google Scholar 

  16. Bao, G., Wang, L.: Multiple cracking in functionally graded ceramic/metal coatings. Int. J. Solids Struct. 32(19), 2853–2871 (1995)

    MATH  Google Scholar 

  17. Wang, B., Mai, Y.: A cracked piezoelectric material strip under transient thermal loading. J. Appl. Mech. 69(4), 539–546 (2002)

    MATH  Google Scholar 

  18. Ueda, S.: Thermally induced fracture of a piezoelectric laminate with a crack normal to interfaces. J. Therm. Stress. 26(4), 311–331 (2003)

    Google Scholar 

  19. Ueda, S.: Thermal stress intensity factors for a normal crack in a piezoelectric material strip. J. Therm. Stress. 29(12), 1107–1125 (2006)

    Google Scholar 

  20. Cattaneo, C.: A form of heat-conduction equations which eliminates the paradox of instantaneous propagation. C. R. 247, 431 (1958)

    MATH  Google Scholar 

  21. Vernotte, P.: Some possible complications in the phenomena of thermal conduction. C. R. 252, 2190–2191 (1961)

    Google Scholar 

  22. Shaw, S., Mukhopadhyay, B.: A discontinuity analysis of generalized thermoelasticity theory with memory-dependent derivatives. Acta Mech. 228(7), 2675–2689 (2017)

    MathSciNet  MATH  Google Scholar 

  23. Mondal, S., Pal, P., Kanoria, M.: Transient response in a thermoelastic half-space solid due to a laser pulse under three theories with memory-dependent derivative. Acta Mech. 230, 179–199 (2019)

    MathSciNet  MATH  Google Scholar 

  24. Youssef, H.M.: Two-dimensional thermal shock problem of fractional order generalized thermoelasticity. Acta Mech. 223(6), 1219–1231 (2012)

    MathSciNet  MATH  Google Scholar 

  25. Sur, A., Kanoria, M.: Fibre-reinforced magneto-thermoelastic rotating medium with fractional heat conduction. Procedia Eng. 127, 605–612 (2015)

    Google Scholar 

  26. Sur, A., Kanoria, M.: Modeling of memory-dependent derivative in a fibre-reinforced plate. Thin Wall Struct. 126, 85–93 (2018)

    Google Scholar 

  27. Mondal, S., Sur, A., Kanoria, M.: Transient response in a piezoelastic medium due to the influence of magnetic field with memory-dependent derivative. Acta Mech. 230, 2325–2338 (2019)

    MathSciNet  Google Scholar 

  28. Sur, A., Pal, P., Mondal, S., Kanoria, M.: Finite element analysis in a fiber-reinforced cylinder due to memory-dependent heat transfer. Acta Mech. 230, 1607–1624 (2019)

    Google Scholar 

  29. Purkait, P., Sur, A., Kanoria, M.: Elasto-thermodiffusive response in a spherical shell subjected to memory-dependent heat transfer. Wave Random Complex Media 1–23 (2019). https://doi.org/10.1080/17455030.2019.1599464

  30. Li, W., Song, F., Li, J., Abdelmoula, R., Jiang, C.: Non-Fourier effect and inertia effect analysis of a strip with an induced crack under thermal shock loading. Eng. Fract. Mech. 162, 309–323 (2016)

    Google Scholar 

  31. Hu, K., Chen, Z.: Thermoelastic analysis of a partially insulated crack in a strip under thermal impact loading using the hyperbolic heat conduction theory. Int. J. Eng. Sci. 51, 144–160 (2012)

    MathSciNet  MATH  Google Scholar 

  32. Chang, D., Wang, B.: Transient thermal fracture and crack growth behavior in brittle media based on non-Fourier heat conduction. Eng. Fract. Mech. 94, 29–36 (2012)

    Google Scholar 

  33. Zhang, X., Chen, Z., Li, X.: Thermal shock fracture of an elastic half-space with a subsurface penny-shaped crack via fractional thermoelasticity. Acta Mech. 229(12), 4875–4893 (2018)

    MathSciNet  Google Scholar 

  34. Zhang, X., Li, X.: Transient thermal stress intensity factors for a circumferential crack in a hollow cylinder based on generalized fractional heat conduction. Int. J. Therm. Sci. 121, 336–347 (2017)

    Google Scholar 

  35. Wang, B.: Transient thermal cracking associated with non-classical heat conduction in cylindrical coordinate system. Acta. Mech. Sin. 29(2), 211–218 (2013)

    MathSciNet  MATH  Google Scholar 

  36. Zhang, X., Xie, Y., Li, X.: Transient thermoelastic response in a cracked strip of functionally graded materials via generalized fractional heat conduction. Appl. Math. Model. 70, 328–349 (2019)

    MathSciNet  Google Scholar 

  37. Xue, Z., Chen, Z., Tian, X.: Thermoelastic analysis of a cracked strip under thermal impact based on memory-dependent heat conduction model. Eng. Fract. Mech. 200, 479–498 (2018)

    Google Scholar 

  38. Xue, Z., Chen, Z., Tian, X.: Transient thermal stress analysis for a circumferentially cracked hollow cylinder based on memory-dependent heat conduction model. Theor. Appl. Fract. Mech. 96, 123–133 (2018)

    Google Scholar 

  39. Kaminski, W.: Hyperbolic heat conduction equation for materials with a nonhomogeneous inner structure. J. Heat Transf. 112(3), 555–560 (1990)

    Google Scholar 

  40. Mitra, K., Kumar, S., Vedevarz, A., Moallemi, M.: Experimental evidence of hyperbolic heat conduction in processed meat. J. Heat Transf. 117(3), 568–573 (1995)

    Google Scholar 

  41. Braznikov, A., Karpychev, V., Luikova, A.: One engineering method of calculating heat conduction process. Inzhenerno Fizicheskij Zhurnal 28(4), 677–680 (1975)

    Google Scholar 

  42. Bai, C., Lavine, A.: On hyperbolic heat conduction and the second law of thermodynamics. J. Heat Transf. 117(2), 256–263 (1995)

    Google Scholar 

  43. Körner, C., Bergmann, H.: The physical defects of the hyperbolic heat conduction equation. Appl. Phys. A 67(4), 397–401 (1998)

    Google Scholar 

  44. Rubin, M.: Hyperbolic heat conduction and the second law. Int. J. Eng. Sci. 30(11), 1665–1676 (1992)

    MathSciNet  MATH  Google Scholar 

  45. Zhang, W., Cai, X., Holm, S.: Time-fractional heat equations and negative absolute temperatures. Comput. Math. Appl. 67(1), 164–171 (2014)

    MathSciNet  MATH  Google Scholar 

  46. Ezzat, M.A., El-Karamany, A.S.: Fractional thermoelectric viscoelastic materials. J. Appl. Polym. Sci. 124(3), 2187–2199 (2012)

    Google Scholar 

  47. Tarasov, V.E., Aifantis, E.C.: On fractional and fractal formulations of gradient linear and nonlinear elasticity. Acta Mech. 230, 2043–2070 (2019). https://doi.org/10.1007/s00707-019-2373-x

    Article  MathSciNet  Google Scholar 

  48. Cajić, M., Lazarević, M., Karličić, D., Sun, H., Liu, X.: Fractional-order model for the vibration of a nanobeam influenced by an axial magnetic field and attached nanoparticles. Acta Mech. 229, 4791–4815 (2018)

    MathSciNet  Google Scholar 

  49. Atanackovic, T.M., Pilipovic, S.: On a constitutive equation of heat conduction with fractional derivatives of complex order. Acta Mech. 229, 1111–1121 (2018)

    MathSciNet  MATH  Google Scholar 

  50. Ezzat, M., El-Karamany, A., El-Bary, A.: Generalized thermo-viscoelasticity with memory-dependent derivatives. Int. J. Mech. Sci. 89, 470–475 (2014)

    Google Scholar 

  51. Ezzat, M., El-Karamany, A., El-Bary, A.: Thermo-viscoelastic materials with fractional relaxation operators. Appl. Math. Model. 39(23–24), 7499–7512 (2015)

    MathSciNet  Google Scholar 

  52. Ezzat, M.A., El-Bary, A.A.: On thermo-viscoelastic infinitely long hollow cylinder with variable thermal conductivity. Microsyst. Technol. 23, 3263–3270 (2017)

    Google Scholar 

  53. Sladek, J., Sladek, V., Zhang, C., Schanz, M.: Meshless local Petrov–Galerkin method for continuously nonhomogeneous linear viscoelastic solids. Comput. Mech. 37(3), 279–289 (2006)

    MATH  Google Scholar 

  54. Cheng, Z., Meguid, S., Zhong, Z.: Thermo-mechanical behavior of a viscoelastic FGMs coating containing an interface crack. Int. J. Fract. 164(1), 15–29 (2010)

    MATH  Google Scholar 

  55. Choi, H.J., Thangjitham, S.: Thermally-induced interlaminar crack-tip singularities in laminated anisotropic composites. Int. J. Fract. 60(4), 327–347 (1993)

    Google Scholar 

  56. Carslaw, H.S., Jaeger, J.C.: Conduction of Heat in Solids. Clarendon Press, Oxford (1959)

    MATH  Google Scholar 

  57. Erdogan, F.: Interface cracking of FGM coatings under steady-state heat flow. Eng. Fract. Mech. 59, 361–380 (1998)

    Google Scholar 

  58. Zhou, Y., Li, X., Yu, D.: A partially insulated interface crack between a graded orthotropic coating and a homogeneous orthotropic substrate under heat flux supply. Int. J. Solids Struct. 47, 768–778 (2010)

    MATH  Google Scholar 

  59. Christensen, R.M., Freund, L.: Theory of viscoelasticity. J. Appl. Mech. 38, 720 (1971)

    Google Scholar 

  60. Eringen, A.C.: Continuum Physics. Academic Press Inc, New York (1975). 632 p

    Google Scholar 

  61. Delale, F., Erdogan, F.: Effect of transverse shear and material orthotropy in a cracked spherical cap. Int. J. Solids Struct. 15(12), 907–926 (1979)

    MATH  Google Scholar 

  62. Miller, M.K., Guy, J.: WT: numerical inversion of the Laplace transform by use of Jacobi polynomials. SIAM J. Numer. Anal. 3(4), 624–635 (1966)

    MathSciNet  MATH  Google Scholar 

  63. Paulino, G., Jin, Z.-H.: Viscoelastic functionally graded materials subjected to antiplane shear fracture. J. Appl. Mech. 68(2), 284–293 (2001)

    MATH  Google Scholar 

Download references

Acknowledgements

Funding was provided by Natural Sciences and Engineering Research Council of Canada (2017–2022), China Scholarship Council (2016–2020).

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, University of Alberta, Edmonton, AB, Canada

    Wenzhi Yang & Zengtao Chen

Authors
  1. Wenzhi Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zengtao Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Zengtao Chen.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix

Appendix

$$\begin{aligned} C_1 (\xi ,p)= & {} [m^{2}-\xi ^{2}]^{-2}D(\xi ,p)p^{3}f_1 (p)f_3 (p) \\ C_{21} (\xi ,p)= & {} [m^{2}-\xi ^{2}]^{-2}\frac{-D(\xi ,p)}{1+\exp (-2mh)}p^{3}f_1 (p)f_3 (p) \\ C_{22} (\xi ,p)= & {} [m^{2}-\xi ^{2}]^{-2}\frac{D(\xi ,p)\exp (-2mh)}{1+\exp (-2mh)}p^{3}f_1 (p)f_3 (p) \\ k_{11} (x,\tau )= & {} \int \limits _0^\infty {[1-4\xi f_{11} (\xi )]} \sin [(x-\tau )\xi ]{\mathrm{d}}\xi \\ k_{22} (x,\tau )= & {} \int \limits _0^\infty {[1-4\xi ^{2}f_{22} (\xi )]} \sin [(x-\tau )\xi ]{\mathrm{d}}\xi \\ k_{12} (x,\tau )= & {} \int \limits _0^\infty {-4\xi f_{12} (\xi )} \cos [(x-\tau )\xi ]{\mathrm{d}}\xi \\ k_{21} (x,\tau )= & {} \int \limits _0^\infty {-4\xi ^{2}f_{21} (\xi )} \cos [(x-\tau )\xi ]{\mathrm{d}}\xi \\ W_1 ^{*}(x,p)= & {} 2\int \limits _0^\infty {\xi w_1 ^{*}(\xi ,p)} \sin (x\xi ){\mathrm{d}}\xi \\ W_2 ^{*}(x,p)= & {} -2\int \limits _0^\infty {\xi ^{2}w_2 ^{*}(\xi ,p)} \cos (x\xi ){\mathrm{d}}\xi \\ w_1 ^{*}(\xi ,p)= & {} -\frac{2g_2 h_{11} +2\left| \xi \right| h_{12} (s_2 g_1 -g_2 )}{8\left| \xi \right| \xi ^{2}}-g_3 \\ w_2 ^{*}(\xi ,p)= & {} -\frac{2g_2 h_{21} +2\left| \xi \right| h_{22} (s_2 g_1 -g_2 )}{8\left| \xi \right| \xi ^{2}}-g_4 \\ h_{11} (\xi )= & {} \left| \xi \right| +\exp (-2\left| \xi \right| h)(-\left| \xi \right| +2h\xi ^{2}) \\ h_{12} (\xi )= & {} 1-\exp (-2\left| \xi \right| h)(1-2h\left| \xi \right| +2h^{2}\xi ^{2}) \\ h_{21} (\xi )= & {} 1-\exp (-2\left| \xi \right| h)(1+2h\left| \xi \right| ) \\ h_{22} (\xi )= & {} 2\left| \xi \right| h^{2}\exp (-2\left| \xi \right| h) \\ f_{11} (\xi )= & {} h_{12} (4\left| \xi \right| )^{-1} \\ f_{12} (\xi )= & {} (-2\xi h_{11} +2\xi \left| \xi \right| h_{12} )(-2\left| \xi \right| )^{-3} \\ f_{21} (\xi )= & {} h_{22} (4\left| \xi \right| )^{-1} \\ f_{22} (\xi )= & {} [-2\xi h_{21} +2\xi \left| \xi \right| )h_{22} ](-2\left| \xi \right| )^{-3} \\ g_1 (\xi )= & {} -\xi ^{2}f_3 ^{{\prime }}-2\left| \xi \right| f_4 ^{{\prime }}+f_5 ^{{\prime }} \\ g_2 (\xi )= & {} -2\left| \xi \right| \xi ^{2}f_3 ^{{\prime }}-3\xi ^{2}f_4 ^{{\prime }}-f_6 ^{{\prime }} \\ g_3 (\xi )= & {} \exp (-\left| \xi \right| h)[(1-h\left| \xi \right| )f_2 ^{{\prime }}-h\xi ^{2}f_1 ^{{\prime }}]-mI_{21} +mI_{22} \\ g_4 (\xi )= & {} \exp (-\left| \xi \right| h)[(1+h\left| \xi \right| )f_1 ^{{\prime }}+hf_2 ^{{\prime }}]-I_{21} -I_{22} \\ I_1 (\xi ,p)= & {} \frac{1}{p^{2}f_1 (p)f_3 (p)}C_1 (\xi ,p) \\ I_{21} (\xi ,p)= & {} \frac{1}{p^{2}f_1 (p)f_3 (p)}C_{21} (\xi ,p) \\ I_{22} (\xi ,p)= & {} \frac{1}{p^{2}f_1 (p)f_3 (p)}C_{22} (\xi ,p) \\ f_1 ^{{\prime }}(\xi )= & {} I_{21} \exp (-mh)+I_{22} \exp (mh) \\ f_2 ^{{\prime }}(\xi )= & {} mI_{21} \exp (-mh)-mI_{22} \exp (mh) \\ f_3 ^{{\prime }}(\xi )= & {} I_1 -I_{21} -I_{22} \\ f_4 ^{{\prime }}(\xi )= & {} -mI_{21} +m(I_{22} -I_1 ) \\ f_5 ^{{\prime }}(\xi )= & {} m^{2}I_{21} +m^{2}(I_{22} -I_1 )+\frac{2}{1+e^{-2mh}}D(\xi ) \\ f_6 ^{{\prime }}(\xi )= & {} m^{3}I_{21} -m^{3}(I_{22} -I_1 ) \end{aligned}$$

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yang, W., Chen, Z. Fractional single-phase lag heat conduction and transient thermal fracture in cracked viscoelastic materials. Acta Mech 230, 3723–3740 (2019). https://doi.org/10.1007/s00707-019-02474-z

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-019-02474-z

Search

Navigation