Skip to main content
SpringerLink
Log in

Spectral finite element for vibration analysis of cracked viscoelastic Euler–Bernoulli beam subjected to moving load

  • Original Paper
  • Published:
Acta Mechanica Aims and scope Submit manuscript

Abstract

In this article, a spectral finite element (SFE) model is presented for vibration analysis of a cracked viscoelastic beam subjected to moving loads. The dynamic shape functions are derived from the exact solution of the governing wave equations and are utilized for frequency-domain representation of a moving load. It is considered with either constant velocity or acceleration; then, the force vector for each spectral element is evaluated. The cracked beam is modeled as two segments connected by a massless rotational spring; thus, the beam dynamic stiffness matrix is extracted in frequency domain by considering compatibility conditions at the crack position. The effects of change in velocity and acceleration of moving load, crack parameters, and viscoelastic material properties on the dynamic response of the SFE beam model are investigated. The accuracy of SFE results is compared with that of finite elements. The results show the ascendency of the SFE model, as compared to FEM, for reducing the number of elements and computational effort, but increasing numerical accuracy.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Abbreviations

SFEM:

Spectral finite element method

FEM:

Finite element method

DSM:

Dynamic stiffness matrix

FFT:

Fast Fourier transform

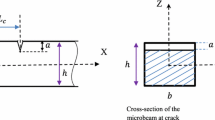
a :

Acceleration of moving load (m/s2)

b :

Width of beam (m)

\({C_{\theta}}\) :

crack depth-to-beam height ratio-dependent function for rotational spring

\({{\varvec{d}}({i\omega})}\) :

Spectral nodal displacement (\({4 \times 1}\)) (m, rad)

\({F({x, i\omega})}\) :

Spectral components of loading (N)

F 0 :

Amplitude of moving load (N)

f 1 :

Fundamental frequency of beam (Hz)

\({{\varvec{f}}_{c}({i\omega})}\) :

Equivalent spectral nodal force vector (\({4 \times 1}\)) (N, N m)

\({{\varvec{f}}_{d} (\omega)}\) :

External spectral nodal force vector (\({4\times}\)) (N, N m)

h :

Height of beam (m)

k :

Complex-valued wave number (rad/m)

L :

Length of beam (m)

\({l_{{\rm c}}}\) :

Crack location in beam (m)

\({l_{{\rm e}}}\) :

Length of spectral element (m)

M(x, t):

Bending moment (N m)

N :

Number of harmonic components of DFT

\({{\varvec{N}}_{{\rm VB}} ({x, i\omega})}\) :

Dynamic shape functions for transverse displacement (\({1 \times 4}\))

P(t):

Position of moving load in any spectral element as a general function (m)

Q(x, t):

Transverse shear force (N)

\({{\varvec{S}}_{{\rm VB}} ({i\omega})}\) :

Dynamic stiffness matrix

t :

Time (s)

V :

Velocity of moving load (m/s)

w(x, t):

Transverse displacement (m)

\({W_{n} ({x; i\omega_{n}})}\) :

Spectral components of transverse displacement (m)

x :

Location coordinate (m)

\({\delta (x)}\) :

Dirac delta function

\({\varepsilon}\) :

Depth of crack (m)

\({\lambda}\) :

Crack depth-to-beam height ratio (m/m)

\({\tau_{{\rm d}}}\) :

Viscoelastic coefficient

\({\omega}\) :

Sampling frequency (rad/s)

FEM:

finite element method

SFEM:

spectral finite element method

DSM:

Dynamic stiffness matrix

\({{\prime}}\) :

Partial differentiation with respect to x

n :

Number of harmonic component of sampling

References

  1. Timoshenko S., Young D.H., Weaver W.: Vibration Problems in Engineering, 4th edn. Wiley, New York (1974)

    Google Scholar 

  2. Lee H.P.: Dynamic response of a beam with a moving mass. J. Sound Vib. 191, 289–294 (1996)

    Article  Google Scholar 

  3. Hasheminejad S.M., Rafsanjani A.: Two-dimensional elasticity solution for transient response of simply supported beams under moving loads. Acta Mech. 217, 205–218 (2011)

    Article  MATH  Google Scholar 

  4. Fryba L.: Vibration of Solids and Structures Under Moving Loads, 3rd edn. Thomas Telford, Groningen (1999)

    Book  Google Scholar 

  5. Di Paola M., Heuer R., Pirrotta A.: Fractional visco-elastic Euler–Bernoulli beam. Int. J. Solid Struct. 50, 3505–3510 (2013)

    Article  Google Scholar 

  6. Lei Y., Murmu T., Adhikari S., Friswell M.I.: Dynamic characteristics of damped viscoelastic nonlocal Euler–Bernoulli beam. Eur. J. Mech. A Solid 42, 125–136 (2013)

    Article  MathSciNet  Google Scholar 

  7. Chondros T.G., Dimarogonas A.D., Yao J.: A continuous cracked beam vibration theory. J. Sound Vib. 215, 17–34 (1998)

    Article  MATH  Google Scholar 

  8. Shifrin E.I., Routolo R.: Natural frequencies of a beam with an arbitrary number of cracks. J. Sound Vib. 222, 409–423 (1999)

    Article  Google Scholar 

  9. Lee H.P., Ng T.Y.: Dynamic response of a cracked beam subject to a moving load. Acta Mech. 106, 221–230 (1994)

    Article  MATH  Google Scholar 

  10. Mahmoud M.A., Zaid M.A.: Dynamic response of a beam with a crack subject to a moving mass. J. Sound Vib. 256, 591–603 (2002)

    Article  Google Scholar 

  11. Patil D.P., Maiti S.K.: Experimental verification of a method of detection of multiple cracks in beams based on frequency measurements. J. Sound Vib. 281, 439–451 (2005)

    Article  Google Scholar 

  12. Nahvi H., Jabbari M.: Crack detection in beams using experimental modal data and finite element model. Int. J. Mech. Sci. 47, 1477–1497 (2005)

    Article  Google Scholar 

  13. Shafiei M., Khaji N.: Analytical solutions for free and forced vibrations of a multiple cracked Timoshenko beam subject to a concentrated moving load. Acta Mech. 221, 79–97 (2011)

    Article  MATH  Google Scholar 

  14. Lin H.P., Chang S.C.: Forced responses of cracked cantilever beams subjected to a concentrated moving load. Int. J. Mech. Sci. 48, 1456–1463 (2006)

    Article  MATH  Google Scholar 

  15. Rieker J.R., Trethewey M.W.: Finite element analysis of an elastic beam structure subjected to a moving distributed mass train. Mech. Syst. Signal Process. 13, 31–51 (1999)

    Article  Google Scholar 

  16. Doyle J.F.: Wave Propagation in Structures, 2nd edn. Springer, New York (1997)

    Book  Google Scholar 

  17. Doyle J.F.: A spectrally formulated finite element for longitudinal wave propagation. Int. J. Anal. Exp. Modal Anal. 3, 1–5 (1988)

    MathSciNet  Google Scholar 

  18. Doyle J.F., Farris T.N.: A spectrally formulated finite element for flexural wave propagation in beams. Int. J. Anal. Exp. Modal Anal. 5, 13–23 (1990)

    Google Scholar 

  19. Gopalakrishnan S., Martin M., Doyle J.F.: A matrix methodology for spectral analysis of wave propagation in multiple connected Timoshenko beams. J. Sound Vib. 158, 11–24 (1992)

    Article  MATH  Google Scholar 

  20. Hajheidari H., Mirdamadi H.R.: Frequency-dependent vibration analysis of symmetric cross-ply laminated plate of Levy-type by spectral element and finite strip procedures. Appl. Math. Model. 37, 7193–7205 (2013)

    Article  MathSciNet  Google Scholar 

  21. Hajheidari H., Mirdamadi H.R.: Free and transient vibration analysis of an un-symmetric cross-ply laminated plate by spectral finite elements. Acta Mech. 223, 2477–2492 (2012)

    Article  MATH  MathSciNet  Google Scholar 

  22. Roy Mahapatra D., Gopalakrishnan S.: A spectral finite element model for analysis of axial–flexural–shear coupled wave propagation in laminated composite beams. Compos. Struct. 59, 67–88 (2003)

    Article  Google Scholar 

  23. Vinod K.G., Gopalakrishnan S., Ganguli R.: Free vibration and wave propagation analysis of uniform and tapered rotating beams using spectrally formulated finite elements. Int. J. Solids Struct. 44, 5875–5893 (2007)

    Article  MATH  Google Scholar 

  24. Lee U., Oh H.: Dynamics of an axially moving viscoelastic beam subject to axial tension. Int. J. Solids Struct. 42, 2381–2398 (2005)

    Article  Google Scholar 

  25. Azizi N., Saadatpour M.M., Mahzoon M.: Using spectral element method for analyzing continuous beams and bridges subjected to a moving load. Appl. Math. Model. 36, 3580–3592 (2012)

    Article  MATH  MathSciNet  Google Scholar 

  26. Lee U.: Spectral Element Method in Structural Dynamics. Wiley, Singapore (2009)

    Book  MATH  Google Scholar 

  27. Ariaei A., Ziaei-Rad S., Ghayour M.: Repair of a cracked Timoshenko beam subjected to a moving mass using piezoelectric patches. Int. J. Mech. Sci. 52, 1074–1091 (2010)

    Article  Google Scholar 

  28. Bathe K.J.: Finite Element Procedures. Prentice-Hall, Englewood Cliffs (1996)

    Google Scholar 

  29. Ariaei A., Ziaei-Rad S., Ghayour M.: Vibration analysis of beams with open and breathing cracks subjected to moving masses. J. Sound Vib. 326, 709–724 (2009)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, Isfahan University of Technology, Isfahan, 84156-83111, Iran

    Vahid Sarvestan, Hamid Reza Mirdamadi, Mostafa Ghayour & Ali Mokhtari

Authors
  1. Vahid Sarvestan
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Hamid Reza Mirdamadi
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Mostafa Ghayour
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Ali Mokhtari
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Hamid Reza Mirdamadi.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sarvestan, V., Mirdamadi, H.R., Ghayour, M. et al. Spectral finite element for vibration analysis of cracked viscoelastic Euler–Bernoulli beam subjected to moving load. Acta Mech 226, 4259–4280 (2015). https://doi.org/10.1007/s00707-015-1491-3

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-015-1491-3

Keywords

Search

Navigation