Skip to main content
SpringerLink
Log in

A variational multiscale stabilized and locking-free meshfree formulation for Reissner–Mindlin plate problems

  • Original Paper
  • Published:
Computational Mechanics Aims and scope Submit manuscript

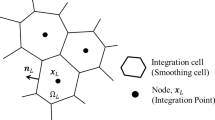
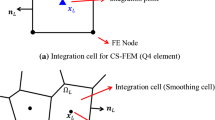
Abstract

In this study, a variational multiscale stabilized locking-free meshfree formulation is introduced for modeling Reissner–Mindlin plate problems under arbitrary plate thickness. Under this framework, the plate quantities are decoupled into coarse-scale and fine-scale components in the variational equations, where the fine-scale solution represents a correction to the residual of the coarse-scale equations that can be solved by an effective collocation-type approach with an approximation method meeting locking free conditions. The substitution of fine-scale solutions in the coarse-scale system leads to a residual-based Galerkin formulation. In the proposed framework, the reproducing kernel approximation, as well as the smoothed gradient and divergence, are adopted to ensure the bending exactness in the Galerkin formulation. The multiscale approach is also beneficial for problems exhibiting localized phenomena. The effectiveness of the proposed method is tested by solving a series of numerical examples and compared with classical methods.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24
Fig. 25
Fig. 26
Fig. 27
Fig. 28
Fig. 29
Fig. 30

Similar content being viewed by others

References

  1. Tanaka S, Dai M, Sadamoto S, Yu T, Bui T (2019) Stress resultant intensity factors evaluation of cracked folded structures by 6DOFs flat shell meshfree modelling. Thin-Walled Struct 144:106285

    Google Scholar 

  2. Kamensky D, Hsu M-C, Schillinger D, Evans JA, Aggarwal A, Bazilevs Y, Sacks MS, Hughes TJ (2015) An immersogeometric variational framework for fluid-structure interaction: application to bioprosthetic heart valves. Comput Methods Appl Mech Eng 284:1005–1053

    MathSciNet  MATH  Google Scholar 

  3. Vu T-V, Nguyen N-H, Khosravifard A, Hematiyan M, Tanaka S, Bui TQ (2017) A simple FSDT-based meshfree method for analysis of functionally graded plates. Eng Anal Boundary Elem 79:1–12

    MathSciNet  MATH  Google Scholar 

  4. Chapelle D, Bathe K-J (2010) The finite element analysis of shells-fundamentals. Springer Science & Business Media, Berlin

    MATH  Google Scholar 

  5. Zienkiewicz O, Taylor R, Too J (1971) Reduced integration technique in general analysis of plates and shells. Int J Numer Meth Eng 3(2):275–290

    MATH  Google Scholar 

  6. Dvorkin EN, Bathe K-J (1984) A continuum mechanics based four-node shell element for general non-linear analysis. Eng Comput 1(1):77–88

    Google Scholar 

  7. Bathe K-J, Dvorkin EN (1985) A four-node plate bending element based on Mindlin/Reissner plate theory and a mixed interpolation. Int J Numer Meth Eng 21(2):367–383

    MATH  Google Scholar 

  8. Bathe K-J, Dvorkin EN (1986) A formulation of general shell elements—the use of mixed interpolation of tensorial components. Int J Numer Meth Eng 22(3):697–722

    MATH  Google Scholar 

  9. Simo JC, Rifai M (1990) A class of mixed assumed strain methods and the method of incompatible modes. Int J Numer Meth Eng 29(8):1595–1638

    MathSciNet  MATH  Google Scholar 

  10. Wilson E, Taylor R, Doherty W, Ghaboussi J (1973) Incompatible displacement models. Numer Comput Methods Struct Mech 43–57

  11. Bletzinger K-U, Bischoff M, Ramm E (2000) A unified approach for shear-locking-free triangular and rectangular shell finite elements. Comput Struct 75(3):321–334

    Google Scholar 

  12. Koschnick F, Bischoff M, Camprubi N, Bletzinger K-U (2005) The discrete strain gap method and membrane locking. Comput Methods Appl Mech Eng 194(21–24):2444–2463

    MATH  Google Scholar 

  13. Cottrell JA, Hughes TJ, Bazilevs Y (2009) Isogeometric analysis: toward integration of CAD and FEA. John Wiley & Sons, Hudson

    MATH  Google Scholar 

  14. Yin S, Hale JS, Yu T, Bui TQ, Bordas SP (2014) Isogeometric locking-free plate element: a simple first order shear deformation theory for functionally graded plates. Compos Struct 118:121–138

    Google Scholar 

  15. Chen J-S, Hillman M, Chi S-W (2017) Meshfree methods: progress made after 20 years. J Eng Mech 143(4):04017001

    Google Scholar 

  16. Kim NH, Choi KK, Chen J-S, Botkin ME (2002) Meshfree analysis and design sensitivity analysis for shell structures. Int J Numer Meth Eng 53(9):2087–2116

    MATH  Google Scholar 

  17. Liew K, Wang J, Ng T, Tan M (2004) Free vibration and buckling analyses of shear-deformable plates based on FSDT meshfree method. J Sound Vib 276(3–5):997–1017

    Google Scholar 

  18. Chen J-S, Liu WK, Hillman M, Chi S-W, Lian Y, Bessa M (2017) Reproducing kernel particle method for solving partial differential equations. Encyclopedia of Computational Mechanics, Second Edition, pp 1–44

  19. Chen J-S, Pan C, Wu C-T, Liu WK (1996) Reproducing kernel particle methods for large deformation analysis of non-linear structures. Comput Methods Appl Mech Eng 139(1–4):195–227

    MathSciNet  MATH  Google Scholar 

  20. Belytschko T, Lu YY, Gu L (1994) Element-free Galerkin methods. Int J Numer Meth Eng 37(2):229–256

    MathSciNet  MATH  Google Scholar 

  21. Liu WK, Jun S, Zhang YF (1995) Reproducing kernel particle methods. Int J Numer Meth Fluids 20(8–9):1081–1106

    MathSciNet  MATH  Google Scholar 

  22. Wang D, Chen J-S (2004) Locking-free stabilized conforming nodal integration for meshfree Mindlin-Reissner plate formulation. Comput Methods Appl Mech Eng 193(12–14):1065–1083

    MATH  Google Scholar 

  23. Chen J-S, Wang D (2006) A constrained reproducing kernel particle formulation for shear deformable shell in Cartesian coordinates. Int J Numer Meth Eng 68(2):151–172

    MATH  Google Scholar 

  24. Wang D, Chen J-S (2008) A Hermite reproducing kernel approximation for thin-plate analysis with sub-domain stabilized conforming integration. Int J Numer Meth Eng 74(3):368–390

    MATH  Google Scholar 

  25. Garcia O, Fancello EA, De Barcellos CS, Duarte CA (2000) hp-Clouds in Mindlin’s thick plate model. Int J Numer Meth Eng 47(8):1381–1400

    MATH  Google Scholar 

  26. Choi YJ, Kim SJ (2003) Bending analysis of Mindlin-Reissner plates by the element free Galerkin method with penalty technique. KSME international journal 17(1):64–76

    Google Scholar 

  27. Donning BM, Liu WK (1998) Meshless methods for shear-deformable beams and plates. Comput Methods Appl Mech Eng 152(1–2):47–71

    MATH  Google Scholar 

  28. Cho J, Atluri S (2001) Analysis of shear flexible beams, using the meshless local Petrov-Galerkin method, based on a locking-free formulation. Eng Comput 18(1–2):215–240

    MATH  Google Scholar 

  29. Hale J, Baiz P (2012) A locking-free meshfree method for the simulation of shear-deformable plates based on a mixed variational formulation. Comput Methods Appl Mech Eng 241:311–322

    MathSciNet  MATH  Google Scholar 

  30. Hillman MC (2013) An arbitrary order variationally consistent integration method for Galerkin meshfree methods. UCLA, Los Angeles

    MATH  Google Scholar 

  31. Chen J-S, Hillman M, Ruter M (2013) An arbitrary order variationally consistent integration for Galerkin meshfree methods. Int J Numer Meth Eng 95(5):387–418

    MathSciNet  MATH  Google Scholar 

  32. Zhang Q, Li S, Zhang A-M, Peng Y, Yan J (2021) A peridynamic Reissner-Mindlin shell theory. Int J Numer Meth Eng 122(1):122–147

    MathSciNet  Google Scholar 

  33. Wang D, Sun Y (2011) A Galerkin meshfree method with stabilized conforming nodal integration for geometrically nonlinear analysis of shear deformable plates. Int J Comput Methods 8(04):685–703

    MathSciNet  MATH  Google Scholar 

  34. Chen J-S, Wu C-T, Yoon S, You Y (2001) A stabilized conforming nodal integration for Galerkin mesh-free methods. Int J Numer Meth Eng 50(2):435–466

    MATH  Google Scholar 

  35. Chen J-S, Yoon S, Wu C-T (2002) Non-linear version of stabilized conforming nodal integration for Galerkin mesh-free methods. Int J Numer Meth Eng 53(12):2587–2615

    MATH  Google Scholar 

  36. Huang T-H, Wei H, Chen J-S, Hillman MC (2020) RKPM2D: an open-source implementation of nodally integrated reproducing kernel particle method for solving partial differential equations. Comput Part Mech 7(2):393–433

    Google Scholar 

  37. Chen J-S, Hu W, Puso M, Wu Y, Zhang X (2007) Strain smoothing for stabilization and regularization of Galerkin meshfree methods. In: Meshfree methods for partial differential equations III. Springer, Berlin, pp 57–75

    MATH  Google Scholar 

  38. Hillman M, Chen J-S (2016) An accelerated, convergent, and stable nodal integration in Galerkin meshfree methods for linear and nonlinear mechanics. Int J Numer Meth Eng 107(7):603–630

    MathSciNet  MATH  Google Scholar 

  39. Puso MA, Zywicz E, Chen J (2007) A new stabilized nodal integration approach. In: Meshfree methods for partial differential equations III. Springer, Berlin, pp 207–217

    MATH  Google Scholar 

  40. Wei H, Chen J-S, Hillman M (2016) A stabilized nodally integrated meshfree formulation for fully coupled hydro-mechanical analysis of fluid-saturated porous media. Comput Fluids 141:105–115

    MathSciNet  MATH  Google Scholar 

  41. Yoshida K, Sadamoto S, Setoyama Y, Tanaka S, Bui T, Murakami C, Yanagihara D (2017) Meshfree flat-shell formulation for evaluating linear buckling loads and mode shapes of structural plates. J Mar Sci Technol 22(3):501–512

    Google Scholar 

  42. Dai M-J, Tanaka S, Sadamoto S, Yu T, Bui TQ (2020) Advanced reproducing kernel meshfree modeling of cracked curved shells for mixed-mode stress resultant intensity factors. Eng Fract Mech 233:107012

    Google Scholar 

  43. Sadamoto S, Ozdemir M, Tanakaa S, Bui T, Okazawa S (2020) Finite rotation meshfree formulation for geometrically nonlinear analysis of flat, curved and folded shells. Int J Non Linear Mech 119:103300

    Google Scholar 

  44. Wang D, Peng H (2013) A Hermite reproducing kernel Galerkin meshfree approach for buckling analysis of thin plates. Springer 51(6):1013–1029

    MathSciNet  MATH  Google Scholar 

  45. Wang D, Lin Z (2010) Free vibration analysis of thin plates using Hermite reproducing kernel Galerkin meshfree method with sub-domain stabilized conforming integration. Comput Mech 46(5):703–719

    MathSciNet  MATH  Google Scholar 

  46. Wang D, Lin Z (2011) Dispersion and transient analyses of Hermite reproducing kernel Galerkin meshfree method with sub-domain stabilized conforming integration for thin beam and plate structures. Comput Mech 48(1):47–63

    MathSciNet  MATH  Google Scholar 

  47. Wu C-T, Chi S-W, Koishi M, Wu Y (2016) Strain gradient stabilization with dual stress points for the meshfree nodal integration method in inelastic analyses. Int J Numer Meth Eng 107(1):3–30

    MathSciNet  MATH  Google Scholar 

  48. Wei H, Chen J-S, Beckwith F, Baek J (2019) A naturally stabilized semi-Lagrangian meshfree formulation for multiphase porous media with application to landslide modelling. J Eng Mech vol. under review.

  49. Thai CH, Ferreira A, Nguyen-Xuan H (2017) Naturally stabilized nodal integration meshfree formulations for analysis of laminated composite and sandwich plates. Compos Struct 178:260–276

    Google Scholar 

  50. Thai CH, Ferreira A, Wahab MA, Nguyen-Xuan H (2018) A moving Kriging meshfree method with naturally stabilized nodal integration for analysis of functionally graded material sandwich plates. Acta Mech 229(7):2997–3023

    MathSciNet  MATH  Google Scholar 

  51. Thai CH, Nguyen-Xuan H (2019) A moving Kriging interpolation meshfree method based on naturally stabilized nodal integration scheme for plate analysis. Int J Comput Methods 16(04):1850100

    MathSciNet  MATH  Google Scholar 

  52. Hughes TJ, Feijoo GR, Mazzei L, Quincy J-B (1998) The variational multiscale method—a paradigm for computational mechanics. Comput Methods Appl Mech Eng 166(1–2):3–24

    MathSciNet  MATH  Google Scholar 

  53. Roth M, Chen J, Slawson T, Danielson K (2016) Stable and flux-conserved meshfree formulation to model shocks. Comput Mech 57(5):773–792

    MathSciNet  MATH  Google Scholar 

  54. Huang TH, Chen JS, Wei H et al. (2019) A MUSCL-SCNI approach for meshfree modeling of shock waves in fluids. Comput Part Mech 7:329–350. https://doi.org/10.1007/s40571-019-00248-x

    Article  Google Scholar 

  55. Belytschko T, Tsay C, Liu W (1981) A stabilization matrix for the bilinear Mindlin plate element. Comput Methods Appl Mech Eng 29(3):313–327

    MathSciNet  MATH  Google Scholar 

  56. Li S, Liu WK (2007) Meshfree particle methods. Springer Science & Business Media, Berlin

    MATH  Google Scholar 

  57. Nitsche J (1971) Uber ein Variationsprinzip zur Losung von Dirichlet-Problemen bei Verwendung von Teilraumen, die keinen Randbedingungen unterworfen sind. Abh Math Sem Univ Hamburg 36: 9–15

  58. Ruter MO, Chen J-S (2017) An enhanced-strain error estimator for Galerkin meshfree methods based on stabilized conforming nodal integration. Comput Math Appl 74(9):2144–2171

    MathSciNet  MATH  Google Scholar 

  59. Chen J-S, Zhang X, Belytschko T (2004) An implicit gradient model by a reproducing kernel strain regularization in strain localization problems. Comput Methods Appl Mech Eng 193(27–29):2827–2844

    MATH  Google Scholar 

  60. Brezzi F, Bristeau M-O, Franca LP, Mallet M, Roge G (1992) A relationship between stabilized finite element methods and the Galerkin method with bubble functions. Comput Methods Appl Mech Eng 96(1):117–129

    MathSciNet  MATH  Google Scholar 

  61. Nakshatrala K, Masud A, Hjelmstad K (2008) On finite element formulations for nearly incompressible linear elasticity. Comput Mech 41(4):547–561

    MathSciNet  MATH  Google Scholar 

  62. Huang T-H, Chen J-S, Tupek MR, Beckwith FN, Koester JJ, Fang HE (2021) A variational multiscale immersed meshfree method for heterogeneous materials. Comput Mech 67(4):1059–1097

    MathSciNet  MATH  Google Scholar 

  63. Chinwuba Ike C (2018) Mathematical solutions for the flexural analysis of Mindlin’s first order shear deformable circular plates. Math Models Eng 4(2):50–72

    Google Scholar 

  64. Timoshenko SP, Woinowsky-Krieger S (1959) Theory of plates and shells. McGraw-hill, New York

    MATH  Google Scholar 

  65. Ferreira A, Batra R, Roque C, Qian L, Jorge R (2006) Natural frequencies of functionally graded plates by a meshless method. Compos Struct 75(1–4):593–600

    Google Scholar 

  66. Roque C, Cunha D, Shu C, Ferreira A (2011) A local radial basis functions-Finite differences technique for the analysis of composite plates. Eng Anal Boundary Elem 35(3):363–374

    MathSciNet  MATH  Google Scholar 

  67. Thai CH, Nguyen-Xuan H, Bordas SPA, Nguyen-Thanh N, Rabczuk T (2015) Isogeometric analysis of laminated composite plates using the higher-order shear deformation theory. Mech Adv Mater Struct 22(6):451–469

    Google Scholar 

  68. Guan P-C, Chi S-W, Chen J-S, Slawson T, Roth MJ (2011) Semi-Lagrangian reproducing kernel particle method for fragment-impact problems. Int J Impact Eng 38(12):1033–1047

    Google Scholar 

Download references

Acknowledgements

The support of this work by the Ministry of Science and Technology (MOST), Taiwan, under project contract number 110-2628-E-007 -008 to the author is greatly acknowledged.

Author information

Authors and Affiliations

  1. Department of Power Mechanical Engineering, National Tsing Hua University, Hsinchu, Taiwan

    Tsung-Hui Huang

Authors
  1. Tsung-Hui Huang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Tsung-Hui Huang.

Ethics declarations

Conflict of interest

The corresponding author reports no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix 1

Appendix 1

In the free vibration test, the frequency of the plate can be determined by solving the eigenvalue problem shown in Eq. (102):

$$\left({\varvec{K}}-{\omega }^{2}{\varvec{M}}\right){\varvec{d}}=\bf{0}$$
(102)

where \({\varvec{K}}\) is the stiffness matrix, \(\omega \) is the frequency to be solved, and \({\varvec{M}}\) is the mass matrix computed by a nodal integration:

$${{\varvec{M}}}_{IJ}=\underset{\Omega }{\overset{}{\int }}{\varvec{\varPsi }}_{I}^{T}{\varvec{I}}{\varvec{\varPsi }}_{J}d\Omega \approx \sum_{L\in S}{\varvec{\varPsi }}_{I}^{T}\left({{\varvec{x}}}_{L}\right){\varvec{I}}{\varvec{\varPsi }}_{J}\left({{\varvec{x}}}_{L}\right){A}_{L}$$
(103)

In Eq. (103), \({\varvec{\Psi }}_{I}\) is the RK shape function shown in Eq. (31), and \({\varvec{I}}\) is a 3 by 3 diagonal inertia matrix, where \({I}_{11}=\rho t\) and \({I}_{22}={I}_{33}=\rho {t}^{3}/12\), with \(\rho \) the plate density and \(t\) the plate thickness.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Huang, TH. A variational multiscale stabilized and locking-free meshfree formulation for Reissner–Mindlin plate problems. Comput Mech 69, 59–93 (2022). https://doi.org/10.1007/s00466-021-02083-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00466-021-02083-5

Keywords

Search

Navigation