Skip to main content
SpringerLink
Log in

A PCE-based multiscale framework for the characterization of uncertainties in complex systems

  • Original Paper
  • Published:
Computational Mechanics Aims and scope Submit manuscript

Abstract

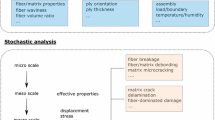
This paper presents a framework for the modeling and analysis of material systems that exhibit uncertainties in their constituents at all scales. The framework integrates multiscale formalism with a polynomial chaos construction enabling an explicit representation of quantities of interests, at any scale, in terms of any form of underlying uncertain parameters, a key feature to model multiscale dependencies. It is demonstrated how the framework can successfully tackle settings where a hierarchy of scales must be explicitly modeled. The application of this framework is illustrated in the construction of stochastic models of mesoscale and macroscale properties of non-crimp fabric composites. Joint statistical properties of upscaled components of the composite, including properties of tow, laminae and laminate, are computed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14

Similar content being viewed by others

References

  1. Altair MDS. http://www.altair.com/. Altair (2015)

  2. Arnst M, Ghanem R (2008) Probabilistic equivalence and stochastic model reduction in multi-scale analysis. Comput Methods Appl Mech Eng 197(43–44):3584–3592

    Article  MATH  Google Scholar 

  3. Asokan BV, Zabaras N (2006) A stochastic variational multiscale method for diffusion in heterogeneous random media. J Comput Phys 218:654–676

    Article  MathSciNet  MATH  Google Scholar 

  4. Chen W, Yin X, Lee S, Liu WK (2010) A multiscale design methodology for hierarchical systems with random field uncertainty. J Mech Des Trans ASME 123:041006

    Article  Google Scholar 

  5. Clemont A, Soize C, Yvonnet J (2013) Uncertainty quantification in computational stochastic multiscale analysis of nonlinear elastic materials. Comput Methods Appl Mech Eng 254:61–82

    Article  MathSciNet  MATH  Google Scholar 

  6. Dakota. http://dakota.sandia.gov/. Algorithms for design exploration and simulation credibility (2016)

  7. Fish J (2014) Practical multiscaling. Wiley, London

    Google Scholar 

  8. Fish J, Wu W (2011) A nonintrusive stochastic multiscale solver. Int J Numer Methods Eng 88(6):862–879

    Article  MathSciNet  MATH  Google Scholar 

  9. Fish J, Yuan Z (2009) N-scale model reduction theory. In: Fish J (ed) Bridging the scales in science and engineering. Oxford University Press, New York

    Chapter  Google Scholar 

  10. Ganapathysubramanian B, Zabaras N (2007) Modeling diffusion in random heterogeneous media: data-driven models, stochastic collocation and the variational multiscale method. J Comput Phys 226:477–510

    Article  MathSciNet  MATH  Google Scholar 

  11. Ghanem R, Spanos P (1991) Stochastic finite elements: a spectral approach. Springer, New York

    Book  MATH  Google Scholar 

  12. Ghanem R, Red-Horse J (2017) Polynomial chaos: modeling, estimation, and approximation, Handbook of uncertainty quantification. Springer, Berlin

    Book  MATH  Google Scholar 

  13. Greene MS, Liu Y, Chen W, Liu WK (2011) Computational uncertainty analysis in multiresolution materials via stochastic constitutive theory. Comput Methods Appl Mech Eng 200(1–4):309–325

    Article  MathSciNet  MATH  Google Scholar 

  14. Guilleminot J, Soize C, Ghanem R (2012) Stochastic representation for anisotropic permeability tensor random fields. Int J Numer Anal Meth Geomech 36:1592–1608

    Article  Google Scholar 

  15. Johnston J, Chattopadhyay A (1996) Stochastic multiscale modeling and damage progression for composite materials. In: Proceedings of the ASME 2013 international mechanics engineering congress and exposition IMEC2013, 15–21 Nov 1996, pp 1–8

  16. Kaminski M, Kleiber M (1996) Stochastic structural interface defects in fiber composites. Int J Solid Struct 33:3035–3056

    Article  MATH  Google Scholar 

  17. Kaminski M, Kleiber M (2000) Perturbation based stochastic finite element method for homogenization of two-phase elastic composites. Comput Struct 78:811–826

    Article  Google Scholar 

  18. Le Maitre OP, Najm H, Ghanem R, Knio O (2004) Multi-resolution analysis of Wiener-type uncertainty propagation schemes. J Comput Phys 197(2):502–531

    Article  MathSciNet  MATH  Google Scholar 

  19. Le Maitre OP, Knio OM (2010) Spectral methods for uncertainty quantification. Springer, New York

    Book  MATH  Google Scholar 

  20. Ma J, Sahraee S, Wriggers P, de Lorenzis L (2015) Stochastic multiscale homogenization analysis of heterogeneous materials under finite deformations with full uncertainty in the microstructure. Comput Mech 55:819–835

    Article  MathSciNet  MATH  Google Scholar 

  21. Noshadravan A, Ghanem R, Guilleminot J, Atodaria I, Pertalta P (2013) Validation of a probalistic model for mesoscale elasticity tensor of random polycrystals. Int J Uncertain Quantif 3(1):73–100

    Article  MathSciNet  Google Scholar 

  22. Oskay C, Fish J (2007) Eigendeformation-based reduced order homogenization. Comput Methods Appl Mech Eng 196:1216–1243

    Article  MATH  Google Scholar 

  23. Rosenblatt W (1952) Remarks on a multivariate transformation. Ann Math Stat 23(3):470–472

    Article  MathSciNet  MATH  Google Scholar 

  24. Sakata S, Ashida F, Kojima T, Zako M (2008) Influence of Uncertainty in microscopic material property on homogenized elastic property of unidirectional fiber reinforced composites. Theoret Appl Mech 56:67–76

    Google Scholar 

  25. Sakata S, Ashida F, Enya K (2012) A microscopic failure probability analysis of a unidirectional fiber reinforced composite material via a multiscale stochastic stress analysis for a microscopic random variation of an elastic property. Comput Mater Sci 62:35–46

    Article  Google Scholar 

  26. Sakata S, Okuda K, Ikeda K (2015) Stochastic analysis of laminated composite plate considering stochastic homogenization problem. Front Struct Civ Eng 9(2):141–153

    Article  Google Scholar 

  27. Sakata S, Torigoe I (2015) A successive perturbation-based multiscale stochastic analysis method for composite materials. Finite Elem Anal Des 102–103:74–84

    Article  Google Scholar 

  28. Soize C (2000) A nonparametric model of random uncertainties for reduced matrix models in structural dynamics. Probab Eng Mech 15:277–294

    Article  Google Scholar 

  29. Soize C, Ghanem R (1938) Physical systems with random uncertainties: chaos representations with arbitrary probability measure. SIAM J Sci Comput 26(2):897–936

    MathSciNet  MATH  Google Scholar 

  30. Sonjoy S, Ghanem R (2009) A bounded random matrix approach for stochastic upscaling. Multiscale Model Simul 8(1):296–325

    Article  MathSciNet  MATH  Google Scholar 

  31. Tipireddy R, Ghanem R (2014) Adaptation in homogeneous chaos spaces. J Comput Phys 259:304–317

    Article  MathSciNet  MATH  Google Scholar 

  32. Tootkaboni M, Graham-Brady L (2010) A multi-scale spectral stochastic method for homogenization of multi-phase periodic composites with random material properties. Int J Numer Methods Eng 83:59–90

    MathSciNet  MATH  Google Scholar 

  33. Wan X, Karniadakis G (2006) Multi-element generalized polynomial chaos for arbitrary probability measures. SIAM J Sci Comput 28(3):902–928

    Article  MathSciNet  MATH  Google Scholar 

  34. Wan J, Zabaras N (2013) A probabilistic graphical model approach to stochastic multiscale partial differential equations. J Comput Phys 250:477–510

    Article  MathSciNet  MATH  Google Scholar 

  35. Wiener N (1938) The homogeneous chaos. Am J Math 60(4):395–410

    Article  MathSciNet  MATH  Google Scholar 

  36. Wu W, Fish J (2010) Toward a nonintrusive stochastic multiscale design system for composite materials. J Multiscale Comput Eng 8(6):549–559

    Article  Google Scholar 

  37. Xiu D, Karniadakis GE (2002) The Wiener–Askey polynomial chaos for stochastic differential equations. SIAM J Sci Comput 24:619–644

    Article  MathSciNet  MATH  Google Scholar 

  38. Yuan Z, Fish J (2009) Multiple scale eigendeformation-based reduced order homogenization. Comput Methods Appl Mech Eng 198(21):2016–2038

    Article  MATH  Google Scholar 

  39. Yuan Z, Fish J (2009) Hierarchical model reduction at multiple scales. Int J Numer Anal Methods Eng 79:314–339

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The information, data, or work presented herein was funded in part by the Office of Energy Efficiency and Renewable Energy (EERE), U.S. Department of Energy, under Award Number DE-EE0006826. The information, data, or work presented herein was funded in part by an agency of the United States Government. Neither the United States Government nor any agency thereof, nor any of their employees, makes any warranty, express or implied, or assumes any legal liability or responsibility for the accuracy, completeness, or usefulness of any information, apparatus, product, or process disclosed, or represents that its use would not infringe privately owned rights. Reference herein to any specific commercial product, process, or service by trade name, trademark, manufacturer, or otherwise does not necessarily constitute or imply its endorsement, recommendation, or favoring by the United States Government or any agency thereof. The views and opinions of authors expressed herein do not necessarily state or reflect those of the United States Government or any agency thereof.

Author information

Authors and Affiliations

  1. Viterbi School of Engineering, University of Southern California, Los Angeles, CA, 90089, USA

    Loujaine Mehrez & Roger Ghanem

  2. Altair Engineering, Inc., Troy, MI, 48083, USA

    Jacob Fish

  3. GM R&D Technical Center, General Motors Company, 30500 Mound Rd, Warren, MI, 48092, USA

    Venkat Aitharaju & Will R. Rodgers

Authors
  1. Loujaine Mehrez
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jacob Fish
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Venkat Aitharaju
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Will R. Rodgers
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Roger Ghanem
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Loujaine Mehrez.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Mehrez, L., Fish, J., Aitharaju, V. et al. A PCE-based multiscale framework for the characterization of uncertainties in complex systems. Comput Mech 61, 219–236 (2018). https://doi.org/10.1007/s00466-017-1502-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00466-017-1502-4

Keywords

Search

Navigation