A coupled global–local shell model with continuous interlaminar shear stresses

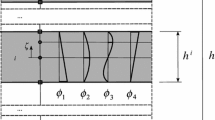
Abstract

In this paper layered composite shells subjected to static loading are considered. The theory is based on a multi-field functional, where the associated Euler–Lagrange equations include besides the global shell equations formulated in stress resultants, the local in-plane equilibrium in terms of stresses and a constraint which enforces the correct shape of warping through the thickness. Within a four-node element the warping displacements are interpolated with layerwise cubic functions in thickness direction and constant shape throughout the element reference surface. Elimination of stress, warping and Lagrange parameters on element level leads to a mixed hybrid shell element with 5 or 6 nodal degrees of freedom. The implementation in a finite element program is simple. The computed interlaminar shear stresses are automatically continuous at the layer boundaries. Also the stress boundary conditions at the outer surfaces are fulfilled and the integrals of the shear stresses coincide exactly with the independently interpolated shear forces without introduction of further constraints. The essential feature of the element formulation is the fact that it leads to usual shell degrees of freedom, which allows application of standard boundary or symmetry conditions and computation of shell structures with intersections.

Appendices

Appendix 1: Data for some selected stress distributions

1.1 Symmetric cross-ply laminate $[0^{\circ }/90^{\circ }/0^{\circ }]$

Table 3 $\tau _{xz}$ at $(x_p=21.429 \, \text {mm} , y_p=0, z)$ for a cross-ply laminate $[0^{\circ }/90^{\circ }/0^{\circ }]$, see Fig. 7

Table 4 $\tau _{yz}$ at $(x_p=0 , y_p=21.429 \, \text {mm}, z)$ for a cross-ply laminate $[0^{\circ }/90^{\circ }/0^{\circ }]$, see Fig. 8

1.2 Unsymmetric cross-ply laminate $[0^{\circ }/90^{\circ }]$

Table 5 $\tau _{xz}$ at $(x_p=21.429\, \text {mm}, y_p=0, z)$ for an unsymmetric lay-up $[0^{\circ }/90^{\circ }]$, see Fig. 11

1.3 Angle-ply laminate with 3 layers $[45^{\circ }/{-}45^{\circ }/45^{\circ }]$

Table 6 $\tau _{xz}$ at $(x_p=21.429\, \text {mm}, y_p=0, z)$ for an angle ply lay-up $[45^{\circ }/{-}45^{\circ }/45^{\circ }]$, see Fig. 13

Table 7 $\tau _{xz}$ at $(x_p=10.937\, \text {mm}, y_p=14.062\, \text {mm}, z)$ for an angle ply lay-up $[45^{\circ }/{-}45^{\circ }/45^{\circ }]$, see Fig. 14

Table 8 $\tau _{yz}$ at $(x_p=10.937\, \text {mm}, y_p=14.062\, \text {mm}, z)$ for an angle ply lay-up $[45^{\circ }/{-}45^{\circ }/45^{\circ }]$, see Fig. 15

Appendix 2: Interpolation matrices of the mixed element formulation

The transverse shear strains at the midside nodes A, B, C, D of the element are as follows

$$\begin{aligned} \begin{array}{rclrcl} \gamma ^M_\xi &{}=&{} [\mathbf{u},_\xi \cdot \bar{\mathbf{D}} + \mathbf{X},_\xi \cdot \Delta \mathbf{d}]^M &{} \qquad M &{}=&{} B,D \\ \gamma ^L_\eta &{}=&{} [\mathbf{u},_\eta \cdot \bar{\mathbf{D}} + \mathbf{X},_\eta \cdot \Delta \mathbf{d}]^L &{} \qquad L &{}=&{} A,C \\ \end{array} \end{aligned}$$

(66)

where the following quantities are given with the bilinear interpolation (44)–(47)

$$\begin{aligned} \bar{\mathbf{D}}^A= & {} \frac{1}{2} \, (\bar{\mathbf{D}}_{4} + \bar{\mathbf{D}}_{1})\qquad \Delta \mathbf{d}^A = \frac{1}{2} \, (\Delta \mathbf{d}_{4} + \Delta \mathbf{d}_{1}) \nonumber \\ \bar{\mathbf{D}}^B= & {} \frac{1}{2} \, (\bar{\mathbf{D}}_{1} + \bar{\mathbf{D}}_{2})\qquad \Delta \mathbf{d}^B = \frac{1}{2} \, (\Delta \mathbf{d}_{1} + \Delta \mathbf{d}_{2}) \nonumber \\ \bar{\mathbf{D}}^C= & {} \frac{1}{2} \, (\bar{\mathbf{D}}_{2} + \bar{\mathbf{D}}_{3})\qquad \Delta \mathbf{d}^C = \frac{1}{2} \, (\Delta \mathbf{d}_{2} + \Delta \mathbf{d}_{3}) \nonumber \\ \bar{\mathbf{D}}^D= & {} \frac{1}{2} \, (\bar{\mathbf{D}}_{3} + \bar{\mathbf{D}}_{4})\qquad \Delta \mathbf{d}^D = \frac{1}{2} \, (\Delta \mathbf{d}_{3} + \Delta \mathbf{d}_{4}) \nonumber \\ \mathbf{X}^A ,_\eta= & {} \frac{1}{2}\, (\mathbf{X}_4 -\mathbf{X}_1)\qquad \mathbf{u}^A, _\eta = \frac{1}{2}\, (\mathbf{u}_{4} - \mathbf{u}_{1}) \nonumber \\ \mathbf{X}^B ,_\xi= & {} \frac{1}{2}\, (\mathbf{X}_2 -\mathbf{X}_1)\qquad \mathbf{u}^B, _\xi = \frac{1}{2}\, (\mathbf{u}_{2} - \mathbf{u}_{1}) \nonumber \\ \mathbf{X}^C ,_\eta= & {} \frac{1}{2}\, (\mathbf{X}_3 -\mathbf{X}_2)\qquad \mathbf{u}^C, _\eta =\frac{1}{2}\, (\mathbf{u}_{3} - \mathbf{u}_{2}) \nonumber \\ \mathbf{X}^D ,_\xi= & {} \frac{1}{2}\, (\mathbf{X}_3 -\mathbf{X}_4)\qquad \mathbf{u}^D, _\xi = \frac{1}{2}\, (\mathbf{u}_{3} - \mathbf{u}_{4}). \end{aligned}$$

(67)

The matrix $\mathbf{B} =[\mathbf{B}_1, \mathbf{B}_2, \mathbf{B}_3, \mathbf{B}_4]$ follows with

$$\begin{aligned}&\mathbf{B}_I = \left[ \begin{array}{l@{\quad }l} N_I,_1 \, \mathbf{X}^T,_1 &{}\quad \mathbf{0} \\ N_I,_2 \, \mathbf{X}^T,_2 &{}\quad \mathbf{0} \\ N_I,_1 \, \mathbf{X}^T,_2 + N_I,_2 \, \mathbf{X}^T,_1 &{}\quad \mathbf{0} \\ N_I,_1 \, \bar{\mathbf{D}}^T , _1 &{}\quad N_I,_1 \, \mathbf{b}^T_{I1} \\ N_I,_2 \, \bar{\mathbf{D}}^T,_2 &{}\quad N_I,_2 \, \mathbf{b}^T_{I2} \\ N_I,_1 \, \bar{\mathbf{D}}^T,_2 +N_I,_2 \, \bar{\mathbf{D}}^T,_1 &{}\quad N_I,_1 \, \mathbf{b}^T_{I2} +N_I,_2 \, \mathbf{b}^T_{I1} \\ \mathbf{J}^{-1} \, \left\{ \begin{array}{l} N_I,_\xi \, \bar{\mathbf{D}}_M^T \\ N_I,_\eta \, \bar{\mathbf{D}}_L^T \\ \end{array} \right\} &{}\quad \mathbf{J}^{-1} \, \left\{ \begin{array}{l} N_I,_\xi \, \xi _I \, \mathbf{b}_{M}^T \\ N_I,_\eta \, \eta _I \, \mathbf{b}_{L}^T \\ \end{array} \right\} \end{array} \right] \nonumber \\ \end{aligned}$$

(68)

We denote by $\mathbf{b}_{I \alpha } = \mathbf{T}^T_I \, \mathbf{X},_\alpha$, $\mathbf{b}_{M} = \mathbf{T}^T_I \, \mathbf{X}^M,_\xi \;$ and $\mathbf{b}_{L} = \mathbf{T}^T_I \, \mathbf{X}^L,_\eta \,,$ where $\mathbf{T}_I$ is introduced in (50). The allocation of the midside nodes to the corner nodes is given by $(I,M,L) \in \{(1,B,A); (2,B,C); (3,D,C); (4,D,A) \} \,.$ To alleviate the notation the superscript h is omitted in the matrix.

According to [32] the interpolation matrix $\mathbf{N}_\sigma$ reads

$$\begin{aligned} \mathbf{N}_{\sigma } = \left[ \begin{array}{cccccc} \mathbf{1}_3 &{} \quad \mathbf{0} &{}\quad \mathbf{0} &{}\quad \mathbf{N}^m_{\sigma } &{}\quad \mathbf{0} &{}\quad \mathbf{0} \\ \mathbf{0} &{}\quad \mathbf{1}_3 &{} \quad \mathbf{0} &{}\quad \mathbf{0} &{} \quad \mathbf{N}^b_{\sigma } &{} \quad \mathbf{0} \\ \mathbf{0} &{}\quad \mathbf{0} &{}\quad \mathbf{1}_2 &{} \quad \mathbf{0} &{} \quad \mathbf{0} &{} \quad \mathbf{N}^s_{\sigma } \\ \end{array}\right] \end{aligned}$$

(69)

where

$$\begin{aligned} \mathbf{N}^m_\sigma= & {} \mathbf{N}^b_\sigma = \mathbf{T}^0_\sigma \, \left[ \begin{array}{l@{\quad }l} \eta -\bar{\eta } &{} 0 \\ 0 &{} \xi -\bar{\xi } \\ 0 &{} 0 \\ \end{array} \right] \qquad \nonumber \\ \mathbf{N}^s_\sigma= & {} \tilde{\mathbf{T}}^0_\sigma \, \left[ \begin{array}{l@{\quad }l} \eta -\bar{\eta } &{} 0 \\ 0 &{} \xi -\bar{\xi } \\ \end{array} \right] \end{aligned}$$

(70)

with the coordinates $\bar{\xi } = \displaystyle \frac{1}{A_e} \int \limits _{\Omega _e} \xi \, \,\text {d}A$ and $\bar{\eta } = \displaystyle \frac{1}{A_e} \int \limits _{\Omega _e} \eta \, \,\text {d}A$ as well as

$$\begin{aligned}&\mathbf{T}^0_\sigma = \left[ \begin{array}{l@{\quad }l@{\quad }l} J^0_{11}J^0_{11} \quad &{} J^0_{21}J^0_{21} \quad &{} 2 J^0_{11}J^0_{21}\\ J^0_{12}J^0_{12}\quad &{} J^0_{22}J^0_{22} \quad &{} 2 J^0_{12}J^0_{22} \\ J^0_{11}J^0_{12} \quad &{} J^0_{21}J^0_{22}\quad &{} J^0_{11}J^0_{22} + J^0_{12}J^0_{21} \\ \end{array} \right] \nonumber \\&\quad \tilde{\mathbf{T}}^0_\sigma = \left[ \begin{array}{c@{\quad }c} J^0_{11} &{} J^0_{21} \\ J^0_{12} &{} J^0_{22} \\ \end{array} \right] _{\,.} \end{aligned}$$

(71)

The constants $J_{\alpha \beta }^0 = J_{\alpha \beta } (\xi =0, \eta = 0)$ are the components of $\mathbf{J}$ in Eq. (46) evaluated at the element centre.

Again from [32] it holds for $\mathbf{N}_\varepsilon ^1$

$$\begin{aligned} \mathbf{N}_\varepsilon ^1 = \left[ \begin{array}{cccccc} \mathbf{1}_3 &{}\quad \mathbf{0} &{}\quad \mathbf{0} &{} \mathbf{N}^{m1}_\varepsilon &{}\quad \mathbf{0} &{}\quad \mathbf{0} \\ \mathbf{0} &{}\quad \mathbf{1}_3 &{}\quad \mathbf{0} &{} \mathbf{0} &{} \quad \mathbf{N}^{b1}_\varepsilon &{} \quad \mathbf{0} \\ \mathbf{0} &{}\quad \mathbf{0} &{} \quad \mathbf{1}_2 &{} \mathbf{0} &{}\quad \mathbf{0} &{} \quad \mathbf{N}^{s1}_\varepsilon \\ \end{array} \right] \end{aligned}$$

(72)

where

$$\begin{aligned} \mathbf{N}^{m1}_\varepsilon = \mathbf{N}^{b1}_\varepsilon = \mathbf{T}^0_\varepsilon \, \left[ \begin{array}{ll} \eta -\bar{\eta } &{}\quad 0 \\ 0 &{}\quad \xi -\bar{\xi } \\ 0 &{}\quad 0 \end{array} \right] \qquad \mathbf{N}^{s1}_\varepsilon = \mathbf{N}^s_\sigma \end{aligned}$$

(73)

and

$$\begin{aligned} \mathbf{T}^0_\varepsilon = \left[ \begin{array}{lll} J^0_{11}J^0_{11} &{}\quad J^0_{21}J^0_{21} &{} \quad J^0_{11}J^0_{21} \\ J^0_{12}J^0_{12} &{}\quad J^0_{22}J^0_{22} &{}\quad J^0_{12}J^0_{22} \\ 2 J^0_{11}J^0_{12} &{}\quad 2 J^0_{21}J^0_{22} &{}\quad J^0_{11}J^0_{22} + J^0_{12}J^0_{21} \end{array} \right] _{\,.} \end{aligned}$$

(74)

A further part with special interpolation functions has been introduced in [32] to improve the membrane and bending behaviour of the element. These functions are constructed orthogonal to the stress resultant interpolation. For the sake of convenience this part is omitted here.