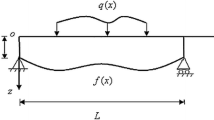
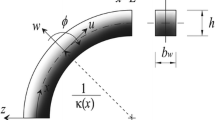
Appendix
The coefficients \(A_i^{+/-} (i=1,2,3)\) of transcendental equation (11), which may be used for the determination of the unknown neutral layer, are obtained as
$$\begin{aligned} \left\{ {\begin{array}{l} A_{\mathrm{1}}^+ =\int _0^{h_1 } {\hbox {e}^{\alpha _1 y/h}y\hbox {d}y} =-\left( {\frac{h^{2}}{\alpha _1^2 }-\frac{hh_1 }{\alpha _1 }} \right) \hbox {e}^{\alpha _1 h_1 /h}+\frac{h^{2}}{\alpha _1^2 }, \\ A_{\mathrm{1}}^- =\int _{-h_2 }^0 {\hbox {e}^{\alpha _2 y/h}y\hbox {d}y} =\left( {\frac{h^{2}}{\alpha _2^2 }+\frac{hh_2 }{\alpha _2 }} \right) \hbox {e}^{-\alpha _2 h_2 /h}-\frac{h^{2}}{\alpha _2^2 }, \\ A_2^+ =-\int _0^{h_1 } {\hbox {e}^{\alpha _1 y/h}y^{2}\hbox {d}y} =-\left( {\frac{2h^{3}}{\alpha _1^3 }-\frac{2h^{2}h_1 }{\alpha _1^2 }+\frac{hh_1^2 }{\alpha _1 }} \right) \hbox {e}^{\alpha _1 h_1 /h}+\frac{2h^{3}}{\alpha _1^3 }, \\ A_2^- =-\int _{-h_2 }^0 {\hbox {e}^{\alpha _2 y/h}y^{2}\hbox {d}y} =\left( {\frac{2h^{2}h_2 }{\alpha _2^2 }+\frac{2h^{3}}{\alpha _2^3 }+\frac{hh_2^2 }{\alpha _2 }} \right) \hbox {e}^{-\alpha _2 h_2 /h}-\frac{2h^{3}}{\alpha _2^3 }, \\ A_{\mathrm{3}}^+ =\int _0^{h_1 } {\hbox {e}^{\alpha _1 y/h}y^{3}\hbox {d}y} =-\left( {\frac{6h^{4}}{\alpha _1^4 }-\frac{6h^{3}h_1 }{\alpha _1^3 }+\frac{3h^{2}h_1^2 }{\alpha _1^2 }-\frac{hh_1^3 }{\alpha _1 }} \right) \hbox {e}^{\alpha _1 h_1 /h}+\frac{6h^{4}}{\alpha _1^4 }, \\ A_{\mathrm{3}}^- =\int _{-h_2 }^0 {\hbox {e}^{\alpha _2 y/h}y^{3}\hbox {d}y} =\left( {\frac{3h^{2}h_2^2 }{\alpha _2^2 }+\frac{hh_2^3 }{\alpha _2 }+\frac{6h^{4}}{\alpha _2^4 }+\frac{6h^{3}h_2 }{\alpha _2^3 }} \right) \hbox {e}^{-\alpha _2 h_2 /h}-\frac{6h^{4}}{\alpha _2^4 }. \\ \end{array}} \right. \end{aligned}$$
(A.1)
The process obtaining the dimensionless stress function is as follows: firstly by making a transformation \(X=x-s\) to reduce the items in coefficients of the power function, thus Eqs. (30) and (28) will be rewritten as
$$\begin{aligned} \varPhi ^{+/-}(X)=\sum _{n=0}^\infty {a_n^{+/-} X^{n}} , \end{aligned}$$
(A.2)
and
$$\begin{aligned}&(X+s)^{3}\frac{\hbox {d}^{4}\varPhi ^{+/-}}{\hbox {d}X^{4}}+[2(X+s)^{2}-2\alpha _i (X+s)^{3}]\frac{\hbox {d}^{3}\varPhi ^{+/-}}{\hbox {d}X^{3}}+[\alpha _i^2 (X+s)^{3}+\mu \alpha _i (X+s)^{2} \nonumber \\&\quad -\,2\alpha _i (X+s)^{2}-(X+s)]\frac{\hbox {d}^{2}\varPhi ^{+/-}}{\hbox {d}X^{2}}+[\alpha _i (X+s)+1-\mu \alpha _i^2 (X+s)^{2}]\frac{\hbox {d}\varPhi ^{+/-}}{\hbox {d}X}=\hbox {0,} \end{aligned}$$
(A.3)
For solving the equation above, we need to calculate the first-order to the fourth-order derivatives of the dimensionless stress function, respectively
$$\begin{aligned} \left. {\begin{array}{l} \frac{\hbox {d}\varPhi ^{+/-}\left( X \right) }{\hbox {d}X}=a_1^{+/-} +2a_2^{+/-} X+3a_3^{+/-} X^{2}+4a_4^{+/-} X^{3}+{\ldots }+na_n^{+/-} X^{n-1}, \\ \frac{\hbox {d}^{2}\varPhi ^{+/-}\left( X \right) }{\hbox {d}X^{2}}=2a_{\mathrm{2}}^{+/-} +6a_3^{+/-} X+12a_4^{+/-} X^{2}+{\ldots }+n(n-1)a_n^{+/-} X^{n-2}, \\ \frac{\hbox {d}^{3}\varPhi ^{+/-}\left( X \right) }{\hbox {d}X^{3}}=6a_3^{+/-} +24a_4^{+/-} X+60a_5^{+/-} X^{2}+{\ldots }+n(n-1)(n-2)a_n^{+/-} X^{n-3}, \\ \frac{\hbox {d}^{4}\varPhi ^{+/-}\left( X \right) }{\hbox {d}X^{4}}=24a_4^{+/-} +120a_5^{+/-} X+360a_6^{+/-} X^{2}+{\ldots }+n(n-1)(n-2)(n-3)a_6^{+/-} X^{n-4}. \\ \end{array}} \right\} \nonumber \\ \end{aligned}$$
(A.4)
Substituting them into Eq. (A.3) will yield
$$\begin{aligned}&(X+s)^{3}[24a_4^{+/-} +120a_5^{+/-} X+360a_6^{+/-} X^{2}+{\ldots }+n(n-1)(n-2)(n-3)a_6^{+/-} X^{n-4}] \nonumber \\&\quad +\,[2(X+s)^{2}-2\alpha _i (X+s)^{3}][6a_3^{+/-} +24a_4^{+/-} X+60a_5^{+/-} X^{2}+{\ldots }+n(n-1)(n-2)a_n^{+/-} X^{n-3}] \nonumber \\&\quad +\,[\alpha _i^2 (X+s)^{3}+\mu \alpha _i (X+s)^{2}-2\alpha _i (X+s)^{2}-(X+s)][2a_1^{+/-} +6a_3^{+/-} X+12a_4^{+/-} X^{2}+{\ldots } \nonumber \\&\quad +\,n(n-1)a_n^{+/-} X^{n-2}]+[\alpha _i (X+s)+1-\mu \alpha _i^2 (X+s)^{2}][a_1^{+/-} +2a_2^{+/-} X+3a_3^{+/-} X^{2} \nonumber \\&\quad +4a_4^{+/-} X^{3}+{\ldots } +\,na_n^{+/-} X^{n-1}]=0. \end{aligned}$$
(A.5)
Then, expanding the item of \(\left( {X+s} \right) ^{n}\) and merging coefficients of \(X^{n}\) by the same exponents, Eq. (A.5) may be rewritten as
$$\begin{aligned}&2\alpha _i \mu s^{2}a_2^{+/-} -\alpha _i^2 \mu s^{2}a_2^{+/-} -4\alpha _i s^{2}a_2^{+/-} +\alpha _i sa_1^{+/-} +a_1^{+/-} +2\alpha _i^2 s^{3}a_2^{+/-} -2sa_2^{+/-} \nonumber \\&\quad +\,24s^{3}a_4^{+/-} +12s^{2}a_3^{+/-} -12\alpha _i s^{3}a_3^{+/-} \nonumber \\&\quad +\,(-2\alpha _i^2 \mu s^{2}a_2^{+/-} +6\alpha _i^2 s^{3}a_3^{+/-} -2\alpha _i^2 \mu sa_1^{+/-} +6\alpha _i^2 s^{2}a_2^{+/-} +6\alpha _i \mu s^{2}a_3^{+/-} -48\alpha _i s^{3}a_4^{+/-} \nonumber \\&\quad +\,4\alpha _i \mu sa_2^{+/-} -48\alpha _i s^{2}a_3^{+/-} +120s^{3}a_5^{+/-} -6\alpha _i sa_2^{+/-} +120s^{2}a_4^{+/-} +\alpha _i a_1^{+/-} \nonumber \\&\quad +\,18sa_3^{+/-} )X \nonumber \\&\quad +\,(-3\alpha _i^2 \mu s^{2}a_3^{+/-} +12\alpha _i^2 s^{3}a_4^{+/-} -4\alpha _i^2 \mu sa_2^{+/-} +18\alpha _i^2 s^{2}a_3^{+/-} +12\alpha _i \mu s^{2}a_4^{+/-} -120\alpha _i s^{3}a_5^{+/-} \nonumber \\&\quad -\,\alpha _i^2 \mu a_1^{+/-} +6\alpha _i^2 sa_2^{+/-} +12\alpha _i \mu sa_3^{+/-} -168\alpha _i s^{2}a_4^{+/-} +360s^{3}a_6^{+/-} +2\alpha _i \mu a_2^{+/-} -57\alpha _i sa_3^{+/-} \nonumber \\&\quad +\,480s^{2}a_5^{+/-} -2\alpha _i a_2^{+/-} +156sa_4^{+/-} +9a_3^{+/-} )X^{2} \nonumber \\&\quad +\,(-4\alpha _i^2 \mu s^{2}a_4^{+/-} +20\alpha _i^2 s^{3}a_5^{+/-} -6\alpha _i^2 \mu sa_3^{+/-} +36\alpha _i^2 s^{2}a_4^{+/-} +20\alpha _i \mu s^{2}a_5^{+/-} \nonumber \\&\quad -\,240\alpha _i s^{3}a_6^{+/-} -2\alpha _i^2 \mu a_2^{+/-} +18\alpha _i^2 sa_3^{+/-} +24\alpha _i \mu sa_4^{+/-} -400\alpha _i s^{2}a_5^{+/-} +840s^{3}a_7^{+/-} \nonumber \\&\quad +\,2\alpha _i^2 a_2^{+/-} +6\alpha _i \mu a_3^{+/-} -188\alpha _i sa_4^{+/-} +\,1320s^{2}a_6^{+/-} -21\alpha _i a_3^{+/-} +580sa_5^{+/-} \nonumber \\&\quad +\,64a_4^{+/-} )X^{3} \nonumber \\&\quad +\,(-5\alpha _i^2 \mu s^{2}a_5^{+/-} +30\alpha _i^2 s^{3}a_6^{+/-} -8\alpha _i^2 \mu sa_4^{+/-} +60\alpha _i^2 s^{2}a_5^{+/-} +30\alpha _i \mu s^{2}a_6^{+/-} \nonumber \\&\quad +225a_5^{+/-} -420\alpha _i s^{3}a_7^{+/-} -3\alpha _i^2 \mu a_3^{+/-} +36\alpha _i^2 sa_4^{+/-} +40\alpha _i \mu sa_5^{+/-} -780\alpha _i s^{2}a_6^{+/-} \nonumber \\&\quad +\,1680s^{3}a_8^{+/-} +6\alpha _i^2 a_3^{+/-} +12\alpha _i \mu a_4^{+/-} -435\alpha _i sa_5^{+/-} +2940s^{2}a_7^{+/-} -68\alpha _i a_4^{+/-} \nonumber \\&\quad +\,1530sa_6^{+/-} )X^{4} \nonumber \\&\quad +\,(-6\alpha _i^2 \mu s^{2}a_6^{+/-} +42\alpha _i^2 s^{3}a_7^{+/-} -10\alpha _i^2 \mu sa_5^{+/-} +90\alpha _i^2 s^{2}a_6^{+/-} +42\alpha _i \mu s^{2}a_7^{+/-} \nonumber \\&\quad -\,672\alpha _i s^{3}a_8^{+/-} -4\alpha _i^2 \mu a_4^{+/-} +60\alpha _i^2 sa_5^{+/-} -1344\alpha _i s^{2}a_7^{+/-} +3204s^{3}a_9^{+/-} \nonumber \\&\quad +\,12\alpha _i^2 a_4^{+/-} +20\alpha _i \mu sa_5^{+/-} -834\alpha _i s^{2}a_6^{+/-} +5712s^{2}a_8^{+/-} -155\alpha _i a_5^{+/-} +3318sa_7^{+/-} \nonumber \\&\quad +\,576a_6^{+/-} )X^{5} \nonumber \\&\quad +\,(-7\alpha _i^2 \mu s^{2}a_7^{+/-} +56\alpha _i^2 s^{3}a_8^{+/-} -12\alpha _i^2 \mu sa_6^{+/-} +126\alpha _i^2 s^{2}a_7^{+/-} +56\alpha _i \mu s^{2}a_8^{+/-} \nonumber \\&\quad -\,1008\alpha _i s^{3}a_9^{+/-} -5\alpha _i^2 \mu a_5^{+/-} +90\alpha _i^2 sa_6^{+/-} +84\alpha _i \mu sa_7^{+/-} -2128\alpha _i s^{2}a_8^{+/-} +5040s^{3}a_{10}^{+/-} \nonumber \\&\quad +\,20\alpha _i^2 a_5^{+/-} +30\alpha _i \mu a_6^{+/-} -1421\alpha _i sa_7^{+/-} +10080s^{2}a_9^{+/-} -294\alpha _i a_6^{+/-} +6328sa_8^{+/-} \nonumber \\&\quad +\,1225a_7^{+/-} )X^{6} \nonumber \\&\quad +\,(-8\alpha _i^2 \mu s^{2}a_8^{+/-} +72\alpha _i^2 s^{3}a_9^{+/-} -14\alpha _i^2 \mu sa_7^{+/-} +168\alpha _i^2 s^{2}a_6^{+/-} +72\alpha _i \mu s^{2}a_9^{+/-} \nonumber \\&\quad -\,1440\alpha _i s^{3}a_{10}^{+/-} -6\alpha _i^2 \mu a_6^{+/-} +126\alpha _i^2 sa_7^{+/-} +112\alpha _i \mu sa_6^{+/-} +30\alpha _i^2 a_6^{+/-} -3168\alpha _i s^{2}a_9^{+/-} \nonumber \\&\quad +\,7920s^{3}a_{11}^{+/-} +42\alpha _i \mu a_7^{+/-} -2232\alpha _i sa_8^{+/-} +16560s^{2}a_{10}^{+/-} -497\alpha _i a_7^{+/-} +11016sa_9^{+/-} \nonumber \\&\quad +\,2304a_6^{+/-} )X^{7} \nonumber \\&\quad +\,(-9\alpha _i \mu s^{2}a_9^{+/-} +90\alpha _i^2 s^{3}a_{10}^{+/-} -16\alpha _i^2 \mu sa_8^{+/-} +216\alpha _i^2 s^{2}a_9^{+/-} +90\alpha _i \mu s^{2}a_{10}^{+/-} -1980\alpha _i s^{3}a_{11}^{+/-} \nonumber \\&\quad -\,7\alpha _i^2 \mu a_7^{+/-} +168\alpha _i^2 sa_8^{+/-} +144\alpha _i \mu sa_9^{+/-} -4500\alpha _i s^{2}a_{10}^{+/-} +11880s^{3}a_{12}^{+/-} +42\alpha _i^2 a_7^{+/-} \nonumber \\&\quad +\,56\alpha _i \mu sa_8^{+/-} -3303\alpha _i sa_9^{+/-} -776\alpha _i a_8^{+/-} +25740s^{2}a_{11}^{+/-} +17910sa_{10}^{+/-} \nonumber \\&\quad +\,3969a_9^{+/-} )X^{8}+{\ldots }=0, \end{aligned}$$
(A.6)
It is obvious that the coefficients and the constants of the term must be zero, so that the left expression of the equation equals zero permanently. Therefore, the following recursive formulas may be obtained
$$\begin{aligned}&2\alpha _i \mu s^{2}a_2^{+/-} -\alpha _i^2 \mu s^{2}a_2^{+/-} -4\alpha _i s^{2}a_2^{+/-} +\alpha _i sa_1^{+/-} +a_1^{+/-} +2\alpha _i^2 s^{3}a_2^{+/-} -2sa_2^{+/-} +24s^{3}a_4^{+/-} \nonumber \\&\quad +\,12s^{2}a_3^{+/-} -12\alpha _i s^{3}a_3^{+/-} =0 \nonumber \\&\quad -\,2\alpha _i^2 \mu s^{2}a_2^{+/-} +6\alpha _i^2 s^{3}a_3^{+/-} -2\alpha _i^2 \mu sa_1^{+/-} +6\alpha _i^2 s^{2}a_2^{+/-} +6\alpha _i \mu s^{2}a_3^{+/-} +18sa_3^{+/-} -48\alpha _i s^{3}a_4^{+/-} \nonumber \\&\quad +\,4\alpha _i \mu sa_2^{+/-} -48\alpha _i s^{2}a_3^{+/-} +120s^{3}a_5^{+/-} -6\alpha _i sa_2^{+/-} +120s^{2}a_4^{+/-} +\alpha _i a_1^{+/-} =0 \nonumber \\&\quad -\,3\alpha _i^2 \mu s^{2}a_3^{+/-} +12\alpha _i^2 s^{3}a_4^{+/-} -4\alpha _i^2 \mu sa_2^{+/-} +18\alpha _i^2 s^{2}a_3^{+/-} +12\alpha _i \mu s^{2}a_4^{+/-} -120\alpha _i s^{3}a_5^{+/-} \nonumber \\&\quad -\,\alpha _i^2 \mu a_1^{+/-} +6\alpha _i^2 sa_2^{+/-} +12\alpha _i \mu sa_3^{+/-} -168\alpha _i s^{2}a_4^{+/-} +360s^{3}a_6^{+/-} +2\alpha _i \mu a_2^{+/-} -57\alpha _i sa_3^{+/-} \nonumber \\&\quad +\,480s^{2}a_5^{+/-} -2\alpha _i a_2^{+/-} +156sa_4^{+/-} +9a_3^{+/-} =0 \nonumber \\&\quad -\,4\alpha _i^2 \mu s^{2}a_4^{+/-} +20\alpha _i^2 s^{3}a_5^{+/-} -6\alpha _i^2 \mu sa_3^{+/-} +36\alpha _i^2 s^{2}a_4^{+/-} +20\alpha _i \mu s^{2}a_5^{+/-} -240\alpha _i s^{3}a_6^{+/-} \nonumber \\&\quad -\,2\alpha _i^2 \mu a_2^{+/-} +18\alpha _i^2 sa_3^{+/-} +24\alpha _i \mu sa_4^{+/-} -400\alpha _i s^{2}a_5^{+/-} +840s^{3}a_7^{+/-} +2\alpha _i^2 a_2^{+/-} +6\alpha _i \mu a_3^{+/-} \nonumber \\&\quad -\,188\alpha _i sa_4^{+/-} +1320s^{2}a_6^{+/-} -21\alpha _i a_3^{+/-} +580sa_5^{+/-} +64a_4^{+/-} =0 \nonumber \\&\quad -\,5\alpha _i^2 \mu s^{2}a_5^{+/-} +30\alpha _i^2 s^{3}a_6^{+/-} -8\alpha _i^2 \mu sa_4^{+/-} +60\alpha _i^2 s^{2}a_5^{+/-} +30\alpha _i \mu s^{2}a_6^{+/-} +225a_5^{+/-} \nonumber \\&\quad -\,420\alpha _i s^{3}a_7^{+/-} -3\alpha _i^2 \mu a_3^{+/-} +36\alpha _i^2 sa_4^{+/-} +40\alpha _i \mu sa_5^{+/-} -780\alpha _i s^{2}a_6^{+/-} +1680s^{3}a_8^{+/-} \nonumber \\&\quad +\,6\alpha _i^2 a_3^{+/-} +12\alpha _i \mu a_4^{+/-} -435\alpha _i sa_5^{+/-} +2940s^{2}a_7^{+/-} -68\alpha _i a_4^{+/-} +1530sa_6^{+/-} =0 \nonumber \\&\quad -\,6\alpha _i^2 \mu s^{2}a_6^{+/-} +42\alpha _i^2 s^{3}a_7^{+/-} -10\alpha _i^2 \mu sa_5^{+/-} +90\alpha _i^2 s^{2}a_6^{+/-} +42\alpha _i \mu s^{2}a_7^{+/-} +576a_6^{+/-} \nonumber \\&\quad -\,672\alpha _i s^{3}a_8^{+/-} -4\alpha _i^2 \mu a_4^{+/-} +60\alpha _i^2 sa_5^{+/-} -1344\alpha _i s^{2}a_7^{+/-} +3204s^{3}a_9^{+/-} +12\alpha _i^2 a_4^{+/-} \nonumber \\&\quad +\,20\alpha _i \mu sa_5^{+/-} -834\alpha _i s^{2}a_6^{+/-} +5712s^{2}a_8^{+/-} -155\alpha _i a_5^{+/-} +3318sa_7^{+/-} =0 \nonumber \\&\quad -\,7\alpha _i^2 \mu s^{2}a_7^{+/-} +56\alpha _i^2 s^{3}a_8^{+/-} -12\alpha _i^2 \mu sa_6^{+/-} +126\alpha _i^2 s^{2}a_7^{+/-} +56\alpha _i \mu s^{2}a_8^{+/-} -1008\alpha _i s^{3}a_9^{+/-} \nonumber \\&\quad -\,5\alpha _i^2 \mu a_5^{+/-} +90\alpha _i^2 sa_6^{+/-} +84\alpha _i \mu sa_7^{+/-} -2128\alpha _i s^{2}a_8^{+/-} +20\alpha _i^2 a_5^{+/-} +30\alpha _i \mu a_6^{+/-} \nonumber \\&\quad -\,1421\alpha _i sa_7^{+/-} +10080s^{2}a_9^{+/-} -294\alpha _i a_6^{+/-} +6328sa_8^{+/-} +5040s^{3}a_{10}^{+/-} +1225a_7^{+/-} =0, \nonumber \\&\quad {\ldots } \end{aligned}$$
(A.7)
Solving the recursive formulas in Eq. (A.7), the unknown coefficients \(a_4^{+/-} \), \(a_5^{+/-} \), \(a_6^{+/-} \), \(a_7^{+/-} \) and \(a_8^{+/-} \)... may be expressed as follows, with the help of constants \(a_1^{+/-} \), \(a_2^{+/-} \) and \(a_3^{+/-} \):
$$\begin{aligned} a_4^{+/-}= & {} -\frac{1}{24s^{3}}(\alpha _i^ 2 \mu s^{2}a_1^{+/-} +2\alpha _i^ 2 s^{3}a_2^{+/-} +2\alpha _i \mu s^{2}a_2^{+/-} -12\alpha _i s^{3}a_3^{+/-} -4\alpha _i s^{2}a_2^{+/-} +a_1^{+/-} \nonumber \\&+\,\alpha _i sa_1^{+/-} +12s^{2}a_3^{+/-} -2sa_2^{+/-} ) \nonumber \\ a_5^{+/-}= & {} -\frac{1}{120s^{4}}(2\alpha _i^ 3 \mu s^{3}a_1^{+/-} +4\alpha _i^ 3 s^{4}a_2^{+/-} +2\alpha _i^ 2 \mu s^{3}a_2^{+/-} -18\alpha _i^ 2 s^{4}a_3^{+/-} -7\alpha _i^ 2 \mu s^{2}a_1^{+/-} \nonumber \\&\quad -\,12\alpha _i^ 2 s^{3}a_2^{+/-} +6\alpha _i \mu s^{3}a_3^{+/-} +2\alpha _i^ 2 s^{2}a_1^{+/-} -6\alpha _i \mu s^{2}a_2^{+/-} +36\alpha _i s^{3}a_3^{+/-} +10\alpha _i s^{2}a_2^{+/-} \nonumber \\&-\,2\alpha _i sa_1^{+/-} -42s^{2}a_3^{+/-} +10sa_2^{+/-} -5a_1^{+/-} ) \nonumber \\ a_6^{+/-}= & {} -\frac{1}{720s^{5}}(3\alpha _i^ 4 \mu s^{4}a_1^{+/-} +6\alpha _i^ 4 s^{5}a_2^{+/-} -\alpha _i^ 3 \mu ^{2}s^{3}a_1^{+/-} -24\alpha _i^ 3 s^{5}a_3^{+/-} -16\alpha _i^ 3 \mu s^{3}a_1^{+/-} \nonumber \\&\quad -\,24\alpha _i^ 3 s^{4}a_2^{+/-} -2\alpha _i^ 2 \mu ^{2}s^{3}a_2^{+/-} +18\alpha _i^ 2 \mu s^{4}a_3^{+/-} +3\alpha _i^ 3 s^{3}a_1^{+/-} -4\alpha _i^ 2 \mu s^{3}a_2^{+/-} -54sa_2^{+/-} \nonumber \\&+\,72\alpha _i^ 2 s^{4}a_3^{+/-} +40\alpha _i^ 2 \mu s^{2}a_1^{+/-} +48\alpha _i^ 2 s^{3}a_2^{+/-} -36\alpha _i \mu s^{3}a_3^{+/-} -7\alpha _i^ 2 s^{2}a_1^{+/-} +28\alpha _i \mu s^{2}a_2^{+/-} \nonumber \\&-\,162\alpha _i s^{3}a_3^{+/-} -\alpha _i \mu sa_1^{+/-} -40\alpha _i s^{2}a_2^{+/-} +7\alpha _i sa_1^{+/-} +198s^{2}a_3^{+/-} +27a_1^{+/-} ) \nonumber \\ a_7^{+/-}= & {} -\frac{1}{5040s^{6}}(4\alpha _i^ 5 \mu s^{5}a_1^{+/-} +8\alpha _i^ 5 s^{6}a_2^{+/-} -3\alpha _i^ 4 \mu ^{2}s^{4}a_1^{+/-} -4\alpha _i^ 4 \mu s^{5}a_2^{+/-} +336sa_2^{+/-} \nonumber \\&-\,30\alpha _i^ 4 s^{6}a_3^{+/-} -27\alpha _i^ 4 \mu s^{4}a_1^{+/-} -40\alpha _i^ 4 s^{5}a_2^{+/-} -4\alpha _i^ 3 \mu ^{2}s^{4}a_2^{+/-} +36\alpha _i^ 3 \mu s^{5}a_3^{+/-} \nonumber \\&+\,4\alpha _i^ 4 s^{4}a_1^{+/-} +12\alpha _i^ 3 \mu ^{2}s^{3}a_1^{+/-} +16\alpha _i^ 3 \mu s^{4}a_2^{+/-} +120\alpha _i^ 3 s^{5}a_3^{+/-} -6\alpha _i^ 2 \mu ^{2}s^{4}a_3^{+/-} \nonumber \\&+\,104\alpha _i^ 3 \mu s^{3}a_1^{+/-} +124\alpha _i^ 3 s^{4}a_2^{+/-} +16\alpha _i^ 2 \mu ^{2}s^{3}a_2^{+/-} -138\alpha _i^ 2 \mu s^{4}a_3^{+/-} -14\alpha _i^ 3 s^{3}a_1^{+/-} \nonumber \\&+\,16\alpha _i^ 2 \mu s^{3}a_2^{+/-} -396\alpha _i^ 2 s^{4}a_3^{+/-} -258\alpha _i^ 2 \mu s^{2}a_1^{+/-} -260\alpha _i^ 2 s^{3}a_2^{+/-} +228\alpha \mu s^{3}a_3^{+/-} \nonumber \\&+\,36\alpha _i^ 2 s^{2}a_1^{+/-} -164\alpha _i \mu s^{2}a_2^{+/-} +924\alpha _i s^{3}a_3^{+/-} +10\alpha _i \mu sa_1^{+/-} +212\alpha _i s^{2}a_2^{+/-} -34\alpha _i sa_1^{+/-} \nonumber \\&\quad -\,1152s^{2}a_3^{+/-} -168a_1^{+/-} ) \nonumber \\ a_8^{+/-}= & {} -\frac{1}{40320s^{7}}(5\alpha _i^6 \mu s^{6}a_1^{+/-} +10\alpha _i^6 s^{7}a_2^{+/-} -6\alpha _i^5 \mu ^{2}s^{5}a_1^{+/-} -10\alpha _i^5 \mu s^{6}a_2^{+/-} \nonumber \\&-\,36\alpha _i^5 s^{7}a_3^{+/-} -40\alpha _i^5 \mu s^{5}a_1^{+/-} -60\alpha _i^5 s^{6}a_2^{+/-} +\alpha _i^4 \mu ^{3}s^{4}a_1^{+/-} -4\alpha _i^4 \mu ^{2}s^{5}a_2^{+/-} \nonumber \\&\quad +\,60\alpha _i^4 \mu s^{6}a_3^{+/-} +5\alpha _i^5 s^{5}a_1^{+/-} +41\alpha _i^4 \mu ^{2}s^{4}a_1^{+/-} +64\alpha _i^4 \mu s^{5}a_2^{+/-} +180\alpha _i^4 s^{6}a_3^{+/-} \nonumber \\&+\,2\alpha _i^3 \mu ^{3}s^{4}a_2^{+/-} -24\alpha _i^3 \mu ^{2}s^{5}a_3^{+/-} +198\alpha _i^4 \mu s^{4}a_1^{+/-} +250\alpha _i^4 s^{5}a_2^{+/-} +34\alpha _i^3 \mu ^{2}s^{4}a_2^{+/-} \nonumber \\&-\,336\alpha _i^3 \mu s^{5}a_3^{+/-} -23\alpha _i^4 s^{4}a_1^{+/-} -113\alpha _i^3 \mu ^{2}s^{3}a_1^{+/-} -148\alpha _i^3 \mu s^{4}a_2^{+/-} -780\alpha _i^3 s^{5}a_3^{+/-} \nonumber \\&\quad +\,72\alpha _i^2 \mu ^{2}s^{4}a_3^{+/-} -749\alpha _i^3 \mu s^{3}a_1^{+/-} -808\alpha _i^3 s^{4}a_2^{+/-} -126\alpha _i^2 \mu ^{2}s^{3}a_1^{+/-} -94\alpha _i^2 \mu s^{3}a_2^{+/-} \nonumber \\&\quad +\,88\alpha _i^3 s^{3}a_1^{+/-} +\alpha _i^2 \mu ^{2}s^{2}a_1^{+/-} +2 652 \alpha _i^2 s^{4}a_3^{+/-} +1881\alpha _i^2 \mu s^{2}a_1^{+/-} +1056\alpha _i^2 \mu s^{4}a_3^{+/-} \nonumber \\&+\,1720\alpha _i^2 s^{3}a_2^{+/-} -1632\alpha _i \mu s^{3}a_3^{+/-} -232\alpha _i^2 s^{2}a_1^{+/-} +1136\alpha _i \mu s^{2}a_2^{+/-} -6288\alpha _i s^{3}a_3^{+/-} \nonumber \\&\quad -\,88\alpha _i \mu sa_1^{+/-} -1376\alpha _i s^{2}a_2^{+/-} +208\alpha _i sa_1^{+/-} +7920s^{2}a_3^{+/-} -2400sa_2^{+/-} +1200a_1^{+/-} ) \nonumber \\&\quad {\ldots } \end{aligned}$$
(A.8)
Substituting all coefficients into Eq. (30), the dimensionless expression of the stress function is obtained as follows,
$$\begin{aligned} \varPhi ^{+/-}(x)= & {} a_0^{+/-} +a_1^{+/-} (x-s)+a_2^{+/-} (x-s)^{2}+a_3^{+/-} (x-s)^{3} \nonumber \\&-\,\frac{1}{24s^{3}}(\alpha _i^ 2 \mu s^{2}a_1^{+/-} +2\alpha _i^ 2 s^{3}a_2^{+/-} +2\alpha _i \mu s^{2}a_2^{+/-} -12\alpha _i s^{3}a_3^{+/-} -4\alpha _i s^{2}a_2^{+/-} \nonumber \\&\quad +\,a_1^{+/-} +\alpha _i sa_1^{+/-} +12s^{2}a_3^{+/-} -2sa_2^{+/-} )(x-s)^{4} \nonumber \\&-\,\frac{1}{120s^{4}}(2\alpha _i^ 3 \mu s^{3}a_1^{+/-} +4\alpha _i^ 3 s^{4}a_2^{+/-} +2\alpha _i^ 2 \mu s^{3}a_2^{+/-} -18\alpha _i^ 2 s^{4}a_3^{+/-} -7\alpha _i^ 2 \mu s^{2}a_1^{+/-} \nonumber \\&-\,12\alpha _i^ 2 s^{3}a_2^{+/-} +6\alpha _i \mu s^{3}a_3^{+/-} +2\alpha _i^ 2 s^{2}a_1^{+/-} -6\alpha _i \mu s^{2}a_2^{+/-} +36\alpha _i s^{3}a_3^{+/-} +10\alpha _i s^{2}a_2^{+/-} \nonumber \\&-\,2\alpha _i sa_1^{+/-} -42s^{2}a_3^{+/-} +10sa_2^{+/-} -5a_1^{+/-} )(x-s)^{5} \nonumber \\&-\,\frac{1}{720s^{5}}(3\alpha _i^ 4 \mu s^{4}a_1^{+/-} +6\alpha _i^ 4 s^{5}a_2^{+/-} -\alpha _i^ 3 \mu ^{2}s^{3}a_1^{+/-} -24\alpha _i^ 3 s^{5}a_3^{+/-} -16\alpha _i^ 3 \mu s^{3}a_1^{+/-} \nonumber \\&-\,24\alpha _i^ 3 s^{4}a_2^{+/-} -2\alpha _i^ 2 \mu ^{2}s^{3}a_2^{+/-} +18\alpha _i^ 2 \mu s^{4}a_3^{+/-} +3\alpha _i^ 3 s^{3}a_1^{+/-} -4\alpha _i^ 2 \mu s^{3}a_2^{+/-} \nonumber \\&-\,54sa_2^{+/-} +72\alpha _i^ 2 s^{4}a_3^{+/-} +40\alpha _i^ 2 \mu s^{2}a_1^{+/-} +48\alpha _i^ 2 s^{3}a_2^{+/-} -36\alpha _i \mu s^{3}a_3^{+/-} \nonumber \\&-\,7\alpha _i^ 2 s^{2}a_1^{+/-} +28\alpha _i \mu s^{2}a_2^{+/-} -162\alpha _i s^{3}a_3^{+/-} -\alpha _i \mu sa_1^{+/-} -40\alpha _i s^{2}a_2^{+/-} \nonumber \\&+\,7\alpha _i sa_1^{+/-} +198s^{2}a_3^{+/-} +27a_1^{+/-} )(x-s)^{6} \nonumber \\&-\,\frac{1}{5040s^{6}}(4\alpha _i^ 5 \mu s^{5}a_1^{+/-} +8\alpha _i^ 5 s^{6}a_2^{+/-} -3\alpha _i^ 4 \mu ^{2}s^{4}a_1^{+/-} -4\alpha _i^ 4 \mu s^{5}a_2^{+/-} +336sa_2^{+/-} \nonumber \\&-\,30\alpha _i^ 4 s^{6}a_3^{+/-} -27\alpha _i^ 4 \mu s^{4}a_1^{+/-} -40\alpha _i^ 4 s^{5}a_2^{+/-} -4\alpha _i^ 3 \mu ^{2}s^{4}a_2^{+/-} +36\alpha _i^ 3 \mu s^{5}a_3^{+/-} \nonumber \\&+\,4\alpha _i^ 4 s^{4}a_1^{+/-} +12\alpha _i^ 3 \mu ^{2}s^{3}a_1^{+/-} +16\alpha _i^ 3 \mu s^{4}a_2^{+/-} +120\alpha _i^ 3 s^{5}a_3^{+/-} -6\alpha _i^ 2 \mu ^{2}s^{4}a_3^{+/-} \nonumber \\&+\,104\alpha _i^ 3 \mu s^{3}a_1^{+/-} +124\alpha _i^ 3 s^{4}a_2^{+/-} +16\alpha _i^ 2 \mu ^{2}s^{3}a_2^{+/-} -138\alpha _i^ 2 \mu s^{4}a_3^{+/-} -14\alpha _i^ 3 s^{3}a_1^{+/-} \nonumber \\&+\,16\alpha _i^ 2 \mu s^{3}a_2^{+/-} -396\alpha _i^ 2 s^{4}a_3^{+/-} -258\alpha _i^ 2 \mu s^{2}a_1^{+/-} -260\alpha _i^ 2 s^{3}a_2^{+/-} +228\alpha \mu s^{3}a_3^{+/-} \nonumber \\&+\,36\alpha _i^ 2 s^{2}a_1^{+/-} -164\alpha _i \mu s^{2}a_2^{+/-} +924\alpha _i s^{3}a_3^{+/-} +10\alpha _i \mu sa_1^{+/-} +212\alpha _i s^{2}a_2^{+/-} \nonumber \\&-\,34\alpha _i sa_1^{+/-} -1152s^{2}a_3^{+/-} -168a_1^{+/-} )(x-s)^{7} \nonumber \\&-\,\frac{1}{40320s^{7}}(5\alpha _i^6 \mu s^{6}a_1^{+/-} +10\alpha _i^6 s^{7}a_2^{+/-} -6\alpha _i^5 \mu ^{2}s^{5}a_1^{+/-} -10\alpha _i^5 \mu s^{6}a_2^{+/-} -36\alpha _i^5 s^{7}a_3^{+/-} \nonumber \\&-\,40\alpha _i^5 \mu s^{5}a_1^{+/-} -60\alpha _i^5 s^{6}a_2^{+/-} +\alpha _i^4 \mu ^{3}s^{4}a_1^{+/-} -4\alpha _i^4 \mu ^{2}s^{5}a_2^{+/-} +60\alpha _i^4 \mu s^{6}a_3^{+/-} +5\alpha _i^5 s^{5}a_1^{+/-} \nonumber \\&+\,41\alpha _i^4 \mu ^{2}s^{4}a_1^{+/-} +64\alpha _i^4 \mu s^{5}a_2^{+/-} +180\alpha _i^4 s^{6}a_3^{+/-} +2\alpha _i^3 \mu ^{3}s^{4}a_2^{+/-} -24\alpha _i^3 \mu ^{2}s^{5}a_3^{+/-} \nonumber \\&+\,198\alpha _i^4 \mu s^{4}a_1^{+/-} +250\alpha _i^4 s^{5}a_2^{+/-} +34\alpha _i^3 \mu ^{2}s^{4}a_2^{+/-} -336\alpha _i^3 \mu s^{5}a_3^{+/-} -23\alpha _i^4 s^{4}a_1^{+/-} \nonumber \\&-\,113\alpha _i^3 \mu ^{2}s^{3}a_1^{+/-} -148\alpha _i^3 \mu s^{4}a_2^{+/-} -780\alpha _i^3 s^{5}a_3^{+/-} +72\alpha _i^2 \mu ^{2}s^{4}a_3^{+/-} -749\alpha _i^3 \mu s^{3}a_1^{+/-} \nonumber \\&-\,808\alpha _i^3 s^{4}a_2^{+/-} -126\alpha _i^2 \mu ^{2}s^{3}a_1^{+/-} -94\alpha _i^2 \mu s^{3}a_2^{+/-} +88\alpha _i^3 s^{3}a_1^{+/-} +\alpha _i^2 \mu ^{2}s^{2}a_1^{+/-} \nonumber \\&+\,2 652 \alpha _i^2 s^{4}a_3^{+/-} +1881\alpha _i^2 \mu s^{2}a_1^{+/-} +1056\alpha _i^2 \mu s^{4}a_3^{+/-} +1720\alpha _i^2 s^{3}a_2^{+/-} -1632\alpha _i \mu s^{3}a_3^{+/-} \nonumber \\&-\,232\alpha _i^2 s^{2}a_1^{+/-} +1136\alpha _i \mu s^{2}a_2^{+/-} -6288\alpha _i s^{3}a_3^{+/-} -88\alpha _i \mu sa_1^{+/-} -1376\alpha _i s^{2}a_2^{+/-} \nonumber \\&+\,208\alpha _i sa_1^{+/-} +7920s^{2}a_3^{+/-} -2400sa_2^{+/-} +1200a_1^{+/-} )(x-s)^{8}+{\ldots } \end{aligned}$$
(A.9)
in which the constants \(a_0^{+/-} \) in the stress function have been omitted since it has no effect on the final stress.
Applying Eqs. (A.8) and (30) to (29), the dimensionless radial and circumference stresses are found, respectively
$$\begin{aligned} \Sigma _{r}^{+/-}= & {} \frac{a_1^{+/-} }{x}+\frac{2(x-s)}{x}a_2^{+/-} +\frac{3(x-s)^{2}}{x}a_3^{+/-} \nonumber \\&-\,\frac{1}{6xs^{3}}(x-s)^{3}(\alpha _i^2 \mu s^{2}a_1^{+/-} +2\alpha _i^2 s^{3}a_2^{+/-} +2\alpha _i \mu s^{2}a_2^{+/-} -12\alpha _i s^{3}a_3^{+/-} -4\alpha _i s^{2}a_2^{+/-} \nonumber \\&+\,\alpha _i sa_1^{+/-} +12s^{2}a_3^{+/-} -2sa_2^{+/-} +a_1^{+/-} ) \nonumber \\&-\,\frac{1}{24xs^{4}}(x-s)^{4}(2\alpha _i^3 \mu s^{3}a_1^{+/-} +4\alpha _i^3 s^{4}a_2^{+/-} +2\alpha _i^2 \mu s^{3}a_2^{+/-} -18\alpha _i^2 s^{4}a_3^{+/-} -7\alpha _i^2 \mu s^{2}a_1^{+/-} \nonumber \\&-\,12\alpha _i^2 s^{3}a_2^{+/-} +6\alpha _i \mu s^{3}a_3^{+/-} +2\alpha _i^2 s^{2}a_1^{+/-} -6\alpha _i \mu s^{2}a_2^{+/-} +36\alpha _i s^{3}a_3^{+/-} +10\alpha _i s^{2}a_2^{+/-} \nonumber \\&-\,2\alpha _i sa_1^{+/-} -42s^{2}a_3^{+/-} +10sa_2^{+/-} -5a_1^{+/-} ) \nonumber \\&-\,\frac{1}{120xs^{5}}(x-s)^{5}(3\alpha _i^4 \mu s^{4}a_1^{+/-} +6\alpha _i^4 \mu s^{4}a_2^{+/-} -\alpha _i^3 \mu ^{2}s^{3}a_1^{+/-} -24\alpha _i^3 s^{5}a_3^{+/-} -16\alpha _i^3 \mu s^{3}a_1^{+/-} \nonumber \\&-\,24\alpha _i^3 s^{4}a_2^{+/-} -2\alpha _i^2 \mu ^{2}s^{3}a_2^{+/-} +18\alpha _i^2 \mu s^{4}a_3^{+/-} +3\alpha _i^3 s^{3}a_1^{+/-} -4\alpha _i^2 \mu s^{3}a_2^{+/-} +72\alpha _i^2 s^{4}a_3^{+/-} \nonumber \\&+\,40\alpha _i^2 \mu ^{1}s^{2}a_1^{+/-} +48\alpha _i^2 s^{3}a_2^{+/-} -36\alpha _i \mu s^{3}a_3^{+/-} -7\alpha _i^2 s^{2}a_1^{+/-} +28\alpha _i \mu s^{2}a_2^{+/-} -162\alpha _i s^{3}a_3^{+/-} \nonumber \\&-\,\alpha _i \mu sa_1^{+/-} -40\alpha _i s^{2}a_2^{+/-} +7\alpha _i sa_1^{+/-} +198s^{2}a_3^{+/-} -54sa_2^{+/-} +27a_1^{+/-} ) \nonumber \\&-\,\frac{1}{720xs^{6}}(x-s)^{6}(4\alpha _i^5 \mu s^{5}a_1^{+/-} +8\alpha _i^5 s^{6}a_2^{+/-} -3\alpha _i^4 \mu ^{2}s^{4}a_1^{+/-} -4\alpha _i^4 \mu s^{5}a_2^{+/-} -30\alpha _i^4 s^{6}a_3^{+/-} \nonumber \\&-\,27\alpha _i^4 \mu s^{4}a_1^{+/-} -40\alpha _i^4 s^{5}a_2^{+/-} -4\alpha _i^3 \mu ^{2}s^{4}a_2^{+/-} +36\alpha _i^3 \mu s^{5}a_3^{+/-} +4\alpha _i^4 s^{4}a_1^{+/-} +12\alpha _i^3 \mu ^{2}s^{3}a_1^{+/-} \nonumber \\&+\,16\alpha _i^3 \mu s^{4}a_2^{+/-} +120\alpha _i^3 s^{5}a_3^{+/-} -6\alpha _i^2 \mu ^{2}s^{4}a_3^{+/-} +104\alpha _i^3 \mu s^{3}a_1^{+/-} +124\alpha _i^3 s^{4}a_2^{+/-} +16\alpha _i^2 \mu ^{2}s^{3}a_2^{+/-} \nonumber \\&-\,138\alpha _i^2 \mu s^{4}a_3^{+/-} -14\alpha _i^3 s^{3}a_1^{+/-} +16\alpha _i^2 \mu s^{3}a_2^{+/-} -396\alpha _i^2 s^{4}a_3^{+/-} -258\alpha _i^2 \mu s^{2}a_1^{+/-} -260\alpha _i^2 s^{3}a_2^{+/-} \nonumber \\&+\,228\alpha _i \mu s^{3}a_3^{+/-} +36\alpha _i^2 s^{2}a_1^{+/-} -164\alpha _i \mu s^{2}a_2^{+/-} +924\alpha _i s^{3}a_3^{+/-} +10\alpha _i \mu sa_1^{+/-} +212\alpha _i s^{2}a_2^{+/-} \nonumber \\&-\,34\alpha _i sa_1^{+/-} -1152s^{2}a_3^{+/-} +336sa_2^{+/-} -168a_1^{+/-} ) \nonumber \\&-\,\frac{1}{5040xs^{7}}(x-s)^{7}(5\alpha _i^6 \mu s^{6}a_1^{+/-} +10\alpha _i^6 s^{7}a_2^{+/-} -6\alpha _i^5 \mu ^{2}s^{5}a_1^{+/-} -10\alpha _i^5 \mu s^{6}a_2^{+/-} -36\alpha _i^5 s^{7}a_3^{+/-} \nonumber \\&-\,40\alpha _i^5 \mu s^{5}a_1^{+/-} -60\alpha _i^5 s^{6}a_2^{+/-} +\alpha _i^4 \mu ^{3}s^{4}a_1^{+/-} -4\alpha _i^4 \mu ^{2}s^{5}a_2^{+/-} +60\alpha _i^4 \mu s^{6}a_3^+ +5\alpha _i^5 s^{5}a_1^+ \nonumber \\&+\,41\alpha _i^4 \mu ^{2}s^{4}a_1^+ +64\alpha _i^4 \mu s^{5}a_2^+ +180\alpha _i^4 s^{6}a_1^+ +2\alpha _i^3 \mu ^{3}s^{4}a_2^+ -24\alpha _i^3 \mu ^{2}s^{5}a_1^{+/-} +198\alpha _i^4 \mu s^{4}a_1^{+/-} \nonumber \\&+\,250\alpha _i^5 s^{5}a_2^{+/-} +34\alpha _i^3 \mu ^{2}s^{4}a_2^{+/-} -336\alpha _i^3 \mu s^{5}a_3^{+/-} -23\alpha _i^4 s^{4}a_i^{+/-} -113\alpha _i^3 \mu ^{2}s^{3}a_1^{+/-} \nonumber \\&-\,148\alpha _i^3 \mu s^{4}a_2^{+/-} -780\alpha _i^3 s^{5}a_3^{+/-} +72\alpha _i^2 \mu ^{2}s^{4}a_3^{+/-} -749\alpha _i^3 \mu s^{3}a_1^{+/-} -808\alpha _i^3 s^{4}a_2^{+/-} \nonumber \\&-\,126\alpha _i^2 \mu ^{2}s^{3}a_2^{+/-} +1056\alpha _i^2 \mu s^{4}a_3^{+/-} +88\alpha _i^3 s^{3}a_1^{+/-} +\alpha _i^2 \mu ^{2}s^{2}a_1^{+/-} -94\alpha _i^2 \mu s^{3}a_2^{+/-} \nonumber \\&+\,2652\alpha _i^2 s^{4}a_3^{+/-} +1881\alpha _i^2 \mu s^{2}a_1^{+/-} +1720\alpha _i^2 s^{3}a_2^{+/-} -1632\alpha _i \mu s^{3}a_3^{+/-} -232\alpha _i^2 s^{2}a_1^{+/-} \nonumber \\&+\,1136\alpha _i \mu s^{2}a_2^{+/-} -6288\alpha _i s^{3}a_3^{+/-} -88\alpha _i \mu sa_1^{+/-} -1376\alpha _i s^{2}a_2^{+/-} +208\alpha _i sa_1^{+/-} \nonumber \\&+\,7920s^{2}a_3^{+/-} -2400sa_2^{+/-} +1200a_1^{+/-} )+{\ldots } \end{aligned}$$
(A.10)
and
$$\begin{aligned} \Sigma _\theta ^+= & {} 2a_2^+ +6(x-s)a_3^+ \nonumber \\&-\,\frac{1}{2s^{3}}(x-s)^{2}(\alpha _i^2 \mu s^{2}a_1^{+/-} +2\alpha _i^2 s^{3}a_2^{+/-} +2\alpha _i \mu s^{2}a_2^{+/-} -12\alpha _i s^{3}a_3^{+/-} -4\alpha _i s^{2}a_2^{+/-} \nonumber \\&+\,\alpha _i sa_1^{+/-} +12s^{2}a_3^{+/-} -2sa_2^{+/-} +a_1^{+/-} ) \nonumber \\&-\,\frac{1}{6s^{4}}(x-s)^{3}(2\alpha _i^3 \mu s^{3}a_1^{+/-} +4\alpha _i^3 s^{4}a_2^{+/-} +2\alpha _i^2 \mu s^{3}a_2^{+/-} -18\alpha _i^2 s^{4}a_3^{+/-} -7\alpha _i^2 \mu s^{2}a_1^{+/-} \nonumber \\&-\,12\alpha _i^2 s^{3}a_2^{+/-} +6\alpha _i \mu s^{3}a_3^{+/-} +2\alpha _i^2 s^{2}a_1^{+/-} -6\alpha _i \mu s^{2}a_2^{+/-} +36\alpha _i s^{3}a_3^{+/-} +10\alpha _i s^{2}a_2^{+/-} \nonumber \\&-\,2\alpha _i sa_1^{+/-} -42s^{2}a_3^{+/-} +10sa_2^{+/-} -5a_1^{+/-} ) \nonumber \\&-\,\frac{1}{24s^{5}}(x-s)^{4}(3\alpha _i^4 \mu s^{4}a_1^{+/-} +6\alpha _i^4 \mu s^{4}a_2^{+/-} -\alpha _i^3 \mu ^{2}s^{3}a_1^{+/-} -24\alpha _i^3 s^{5}a_3^{+/-} -16\alpha _i^3 \mu s^{3}a_1^{+/-} \nonumber \\&-\,24\alpha _i^3 s^{4}a_2^{+/-} -2\alpha _i^2 \mu ^{2}s^{3}a_2^{+/-} +18\alpha _i^2 \mu s^{4}a_3^{+/-} +3\alpha _i^3 s^{3}a_1^{+/-} -4\alpha _i^2 \mu s^{3}a_2^{+/-} +72\alpha _i^2 s^{4}a_3^{+/-} \nonumber \\&+\,40\alpha _i^2 \mu ^{1}s^{2}a_1^{+/-} +48\alpha _i^2 s^{3}a_2^{+/-} -36\alpha _i \mu s^{3}a_3^{+/-} -7\alpha _i^2 s^{2}a_1^{+/-} +28\alpha _i \mu s^{2}a_2^{+/-} -162\alpha _i s^{3}a_3^{+/-} \nonumber \\&-\,\alpha _i \mu sa_1^{+/-} -40\alpha _i s^{2}a_2^{+/-} +7\alpha _i sa_1^{+/-} +198s^{2}a_3^{+/-} -54sa_2^{+/-} +27a_1^{+/-} ) \nonumber \\&-\,\frac{1}{120s^{6}}(x-s)^{5}(4\alpha _i^5 \mu s^{5}a_1^{+/-} +8\alpha _i^5 s^{6}a_2^{+/-} -3\alpha _i^4 \mu ^{2}s^{4}a_1^{+/-} -4\alpha _i^4 \mu s^{5}a_2^{+/-} -30\alpha _i^4 s^{6}a_3^{+/-} \nonumber \\&-\,27\alpha _i^4 \mu s^{4}a_1^{+/-} -40\alpha _i^4 s^{5}a_2^{+/-} -4\alpha _i^3 \mu ^{2}s^{4}a_2^{+/-} +36\alpha _i^3 \mu s^{5}a_3^{+/-} +4\alpha _i^4 s^{4}a_1^{+/-} \nonumber \\&+\,12\alpha _i^3 \mu ^{2}s^{3}a_1^{+/-} +16\alpha _i^3 \mu s^{4}a_2^{+/-} +120\alpha _i^3 s^{5}a_3^{+/-} -6\alpha _i^2 \mu ^{2}s^{4}a_3^{+/-} +104\alpha _i^3 \mu s^{3}a_1^{+/-} \nonumber \\&+\,124\alpha _i^3 s^{4}a_2^{+/-} +16\alpha _i^2 \mu ^{2}s^{3}a_2^{+/-} -138\alpha _i^2 \mu s^{4}a_3^{+/-} -14\alpha _i^3 s^{3}a_1^{+/-} +16\alpha _i^2 \mu s^{3}a_2^{+/-} \nonumber \\&-\,396\alpha _i^2 s^{4}a_3^{+/-} -258\alpha _i^2 \mu s^{2}a_1^{+/-} -260\alpha _i^2 s^{3}a_2^{+/-} +228\alpha _i \mu s^{3}a_3^{+/-} +36\alpha _i^2 s^{2}a_1^{+/-} \nonumber \\&-\,164\alpha _i \mu s^{2}a_2^{+/-} +924\alpha _i s^{3}a_3^{+/-} +10\alpha _i \mu sa_1^{+/-} +212\alpha _i s^{2}a_2^{+/-} -34\alpha _i sa_1^{+/-} \nonumber \\&-\,1152s^{2}a_3^{+/-} +336sa_2^{+/-} -168a_1^{+/-} ) \nonumber \\&-\,\frac{1}{720s^{7}}(x-s)^{6}(5\alpha _i^6 \mu s^{6}a_1^{+/-} +10\alpha _i^6 s^{7}a_2^{+/-} -6\alpha _i^5 \mu ^{2}s^{5}a_1^{+/-} -10\alpha _i^5 \mu s^{6}a_2^{+/-} -36\alpha _i^5 s^{7}a_3^{+/-} \nonumber \\&-\,40\alpha _i^5 \mu s^{5}a_1^{+/-} -60\alpha _i^5 s^{6}a_2^{+/-} +\alpha _i^4 \mu ^{3}s^{4}a_1^{+/-} -4\alpha _i^4 \mu ^{2}s^{5}a_2^{+/-} +60\alpha _i^4 \mu s^{6}a_3^+ +5\alpha _i^5 s^{5}a_1^+ \nonumber \\&+\,41\alpha _i^4 \mu ^{2}s^{4}a_1^+ +64\alpha _i^4 \mu s^{5}a_2^+ +180\alpha _i^4 s^{6}a_1^+ +2\alpha _i^3 \mu ^{3}s^{4}a_2^+ -24\alpha _i^3 \mu ^{2}s^{5}a_1^{+/-} +198\alpha _i^4 \mu s^{4}a_1^{+/-} \nonumber \\&+\,250\alpha _i^5 s^{5}a_2^{+/-} +34\alpha _i^3 \mu ^{2}s^{4}a_2^{+/-} -336\alpha _i^3 \mu s^{5}a_3^{+/-} -23\alpha _i^4 s^{4}a_i^{+/-} -113\alpha _i^3 \mu ^{2}s^{3}a_1^{+/-} \nonumber \\&-\,148\alpha _i^3 \mu s^{4}a_2^{+/-} -780\alpha _i^3 s^{5}a_3^{+/-} +72\alpha _i^2 \mu ^{2}s^{4}a_3^{+/-} -749\alpha _i^3 \mu s^{3}a_1^{+/-} -808\alpha _i^3 s^{4}a_2^{+/-} \nonumber \\&-\,126\alpha _i^2 \mu ^{2}s^{3}a_2^{+/-} +1056\alpha _i^2 \mu s^{4}a_3^{+/-} +88\alpha _i^3 s^{3}a_1^{+/-} +\alpha _i^2 \mu ^{2}s^{2}a_1^{+/-} -94\alpha _i^2 \mu s^{3}a_2^{+/-} \nonumber \\&+\,2652\alpha _i^2 s^{4}a_3^{+/-} +1881\alpha _i^2 \mu s^{2}a_1^{+/-} +1720\alpha _i^2 s^{3}a_2^{+/-} -1632\alpha _i \mu s^{3}a_3^{+/-} -232\alpha _i^2 s^{2}a_1^{+/-} \nonumber \\&+\,1136\alpha _i \mu s^{2}a_2^{+/-} -6288\alpha _i s^{3}a_3^{+/-} -88\alpha _i \mu sa_1^{+/-} -1376\alpha _i s^{2}a_2^{+/-} +208\alpha _i sa_1^{+/-} \nonumber \\&+\,7920s^{2}a_3^{+/-} -2400sa_2^{+/-} +1200a_1^{+/-} )+{\ldots }. \end{aligned}$$
(A.11)