Skip to main content
SpringerLink
Log in

The effect of thickness coordinate to radius ratio on free vibration of moderately thick and deep doubly curved cross-ply laminated shell

  • Original
  • Published:
Archive of Applied Mechanics Aims and scope Submit manuscript

Abstract

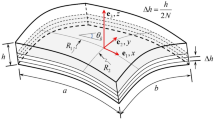
This paper presents the free vibration analysis of moderately thick and deep doubly curved laminated shell with \(C^{0}\) finite element model based on higher-order shear deformation theory. The strain–displacement relationships are developed using the accurate equations of elastic deformation of shell structure. An eight-noded isoparametric shell element with nine degrees of freedom per node is used to formulate the present model. The effect of incorporating the ratio of thickness coordinate to radius of curvature (z / R) in the strain components has been taken into account in the present study. The numerical results in terms of natural frequencies obtained by the present formulations are compared with those available in the published literature to validate the proposed model.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Hildebrand, F.B., Reissner, E., Thomas, G.B.: Note on the foundations of the theory of small displacement of orthotropic shells. NACA TN 1833 (1949)

  2. Nelson, R.B., Lorch, D.R.: A refined theory for laminated orthotropic plates. J. Appl. Mech. 41, 177–183 (1974). doi:10.1115/1.3423219

    Article  Google Scholar 

  3. Whitney, J.M., Sun, C.T.: A higher order theory for extensional motion of laminated anisotropic shells and plates. J. Sound Vib. 30, 85–97 (1973). doi:10.1016/S0022-460X(73)80052-5

    Article  MATH  Google Scholar 

  4. Levinson, M.: An accurate, simple theory of the statics and dynamics of elastic plates. Mech. Res. Commun. 7, 343–350 (1980). doi:10.1016/0093-6413(80)90049-X

    Article  MATH  Google Scholar 

  5. Murthay, M.V.V.: An improved transverse shear deformation theory for laminated anisotropic plates. NASA technical paper 1903 (1981)

  6. Lo, K.H., Christensen, R.M., Wu, E.M.V.: A higher order theory of plate deformation—Part I: Homogeneous plates. J. Appl. Mech. 44(4), 663–668 (1977). doi:10.1115/1.3424154

    Article  MATH  Google Scholar 

  7. Lo, K.H., Christensen, R.M., Wu, E.M.V.: A higher order theory of plate deformation—Part II: Homogeneous plates. J. Appl. Mech. 44(4), 669–676 (1977). doi:10.1115/1.3424155

    Article  MATH  Google Scholar 

  8. Reddy, J.N., Liu, C.F.: A higher-order shear deformation theory of laminated elastic shells. Int. J. Eng. Sci. 23, 319–330 (1985). doi:10.1016/0020-225(85)90051-5

    Article  MATH  Google Scholar 

  9. Reddy, J.N.: A simple higher order theory for laminated composite plate. J. Appl. Mech. 51(4), 745–752 (1984). doi:10.1115/1.3167719

    Article  MATH  Google Scholar 

  10. Mantari, J.L., Oktem, A.S., Guedes Soares, C.: Static and dynamic analysis of laminated composite and sandwich plates and shells by using a new higher order shear deformation theory. Compos. Struct. 94, 37–49 (2011). doi:10.1016/j.compstruct.2011.07.020

    Article  Google Scholar 

  11. Kant, T., Menon, M.P.: Higher order theories for composite and sandwich cylindrical shells with \(\text{ C }^{\rm 0 }\) finite element. Comput. Struct. 33(5), 1191–1204 (1989). doi:10.1016/0045-7949(89)90458-6

    Article  MATH  Google Scholar 

  12. Qatu, M.S.: Accurate equations for laminated composite deep thick shells. Int. J. Solids Struct. 36, 2917–2941 (1999). doi:10.1016/s0020-7683(98)00134-6

    Article  MATH  Google Scholar 

  13. Qatu, M.S.: Theory and vibration analysis of laminated barrel thick shells. J. Vib. Control 10, 319–341 (2004). doi:10.1016/s0020-7683(98)00134-6

    Article  MATH  Google Scholar 

  14. Ganapathi, M., Haboussi, M.: Free vibrations of thick laminated anisotropic non-circular cylindrical shells. Comput. Struct. 60, 125–133 (2003). doi:10.1016/s0263-8223(02)00339-2

    Article  Google Scholar 

  15. Bhimaraddi, A.: Free vibration analysis of doubly curved shallow shells on rectangular planform using three-dimensional elasticity theory. Int. J. Solids Struct. 27(7), 897–913 (1991). doi:10.1016/0020-7683(91)90023-9

    Article  MATH  Google Scholar 

  16. Carrera, E.: The effect of shear deformation and curvature on buckling and vibrations of cross-ply laminated composite shells. J. Sound Vib. 150(3), 405–433 (1991). doi:10.1016/0022-460X(91)90895-Q

    Article  Google Scholar 

  17. Thakur, S.N., Ray, C.: An accurate \({\rm C}^{0}\) finite element model of moderately thick and deep laminated doubly curved shell considering cross sectional warping. Thin Wall Struct. 94, 384–393 (2015). doi:10.1016/j.tws.2015.04.027

    Article  Google Scholar 

  18. Hosseini-Hashemi, S.H., Atashipour, S.R., Fadaee, M., Girhammar, U.A.: An exact closed-form procedure for free vibration analysis of laminated spherical shell panels based on Sanders theory. Arch. Appl. Mech. 82, 985–1002 (2012). doi:10.1007/s00419-011-0606-0

    Article  MATH  Google Scholar 

  19. Civalek, O.: Vibration analysis of laminated composite conical shells by the method of discrete singular convolution based on the shear deformation theory. Compos. Part B Eng. 45, 1001–1009 (2013). doi:10.1016/j.compositesb.2012.05.018

    Article  Google Scholar 

  20. Ye, T., Jin, G., Zhu, Su, Jia, X.Z.: A unified Chebyshev–Ritz formulation for vibration analysis of composite laminated deep open shells with arbitrary boundary conditions. Arch. Appl. Mech. 84, 441–471 (2014). doi:10.1007/s00419-013-0810-1

    Article  MATH  Google Scholar 

  21. Civalek, O.: Geometrically nonlinear dynamic analysis of doubly curved isotropic shells resting on elastic foundation by a combination of HDQ-FD methods. Int. J. Press. Vessels Pip. 82, 470–479 (2005). doi:10.1016/j.ijpvp.2004.12.003

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Civil Engineering, University Institute of Technology, The University of Burdwan, Burdwan, 713104, India

    Sandipan Nath Thakur

  2. Department of Civil Engineering, Indian Institute of Engineering Science and Technology, Shibpur, Howrah, 711103, India

    Chaitali Ray

Authors
  1. Sandipan Nath Thakur
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Chaitali Ray
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Chaitali Ray.

Appendices

Appendix 1

Rigidity matrix of laminates using model 1:

(19)
$$\begin{aligned} \left\{ {{\begin{array}{l} {{\varvec{Q}}_{{\varvec{s}}} } \\ {{\varvec{Q}}_{{\varvec{r}}} } \\ {{\varvec{S}}_{{\varvec{s}}} } \\ {{\varvec{S}}_{{\varvec{r}}} } \\ {{\varvec{Q}}_{{\varvec{s}}}^*} \\ {{\varvec{Q}}_{{\varvec{r}}}^*} \\ {{\varvec{S}}_{{\varvec{s}}}^*} \\ {{\varvec{S}}_{{\varvec{r}}}^*} \\ \end{array} }} \right\}= & {} \left[ {{\begin{array}{llllllll} {\overline{{\varvec{A}}_\mathbf{44}}} &{}\quad {\varvec{A}}_\mathbf{45} &{}\quad {\overline{{\varvec{B}}_\mathbf{44}}}&{}\quad {\varvec{B}}_\mathbf{45} &{}\quad {\overline{{\varvec{C}}_\mathbf{44}}}&{}\quad {\varvec{C}}_\mathbf{45} &{}\quad {\overline{{\varvec{D}}_\mathbf{44}}}&{}\quad {\varvec{D}}_\mathbf{45}\\ {\varvec{A}}_\mathbf{45} &{}\quad {\widetilde{{\varvec{A}}_\mathbf{55}}} &{}\quad {\varvec{B}}_\mathbf{45} &{}\quad {\widetilde{{\varvec{B}}_\mathbf{55}}}&{}\quad {\varvec{C}}_\mathbf{45} &{}\quad {\widetilde{{\varvec{C}}_\mathbf{55}}} &{}\quad {\varvec{D}}_\mathbf{45} &{}\quad {\widetilde{{\varvec{D}}_\mathbf{55}}}\\ {\overline{{\varvec{B}}_\mathbf{44}}} &{}\quad {\varvec{B}}_\mathbf{45} &{}\quad {\overline{{\varvec{C}}_\mathbf{44}}}&{}\quad {\varvec{C}}_\mathbf{45} &{}\quad {\overline{{\varvec{D}}_\mathbf{44}}}&{}\quad {\varvec{D}}_\mathbf{45} &{}\quad {\overline{{\varvec{E}}_\mathbf{44}}}&{}\quad {\varvec{E}}_\mathbf{45}\\ {\varvec{B}}_\mathbf{45} &{}\quad {\widetilde{{\varvec{B}}_\mathbf{55}}} &{}\quad {\varvec{C}}_\mathbf{45} &{}\quad {\widetilde{{\varvec{C}}_\mathbf{55}}}&{}\quad {\varvec{D}}_\mathbf{45} &{}\quad {\widetilde{{\varvec{D}}_\mathbf{55}}} &{}\quad {\varvec{E}}_\mathbf{45} &{}\quad {\widetilde{{\varvec{E}}_\mathbf{55}}}\\ {\overline{{\varvec{C}}_\mathbf{44}}} &{}\quad {\varvec{C}}_\mathbf{45} &{}\quad {\overline{{\varvec{D}}_\mathbf{44}}}&{}\quad {\varvec{D}}_\mathbf{45} &{}\quad {\overline{{\varvec{E}}_\mathbf{44}}}&{}\quad {\varvec{E}}_\mathbf{45} &{}\quad {\overline{{\varvec{F}}_\mathbf{44}}}&{}\quad {\varvec{F}}_\mathbf{45}\\ {\varvec{C}}_\mathbf{45} &{}\quad {\widetilde{{\varvec{C}}_\mathbf{55}}} &{}\quad {\varvec{D}}_\mathbf{45} &{}\quad {\widetilde{{\varvec{D}}_\mathbf{55}}}&{}\quad {\varvec{E}}_\mathbf{45} &{}\quad {\widetilde{{\varvec{E}}_\mathbf{55}}} &{}\quad {\varvec{F}}_\mathbf{45} &{}\quad {\widetilde{{\varvec{F}}_\mathbf{55}}}\\ {\overline{{\varvec{D}}_\mathbf{44}}} &{}\quad {\varvec{D}}_\mathbf{45} &{}\quad {\overline{{\varvec{E}}_\mathbf{44}}}&{}\quad {\varvec{E}}_\mathbf{45} &{}\quad {\overline{{\varvec{F}}_\mathbf{44}}}&{}\quad {\varvec{F}}_\mathbf{45} &{}\quad {\overline{{\varvec{G}}_\mathbf{44}}}&{}\quad {\varvec{G}}_\mathbf{45}\\ {\varvec{D}}_\mathbf{45} &{}\quad {\widetilde{{\varvec{D}}_\mathbf{55}}} &{}\quad {\varvec{E}}_\mathbf{45} &{}\quad {\widetilde{{\varvec{E}}_\mathbf{55}}}&{}\quad {\varvec{F}}_\mathbf{45} &{}\quad {\widetilde{{\varvec{F}}_\mathbf{55}}} &{}\quad {\varvec{G}}_\mathbf{45} &{}\quad {\widetilde{{\varvec{G}}_\mathbf{55}}}\\ \end{array} }} \right] \left\{ {{\begin{array}{l} {{\varvec{\epsilon }}_{{\varvec{szo}}} } \\ {{\varvec{\epsilon }}_{{\varvec{rzo}}} } \\ {{\varvec{\kappa }}_{{\varvec{sz} }}} \\ {{\varvec{\kappa }}_{{\varvec{rz} }}} \\ {{\varvec{\epsilon }}_{{\varvec{szo}}}^*} \\ {{\varvec{\epsilon }}_{{\varvec{rzo}}}^*} \\ {{\varvec{\kappa }}_{{\varvec{sz}}}^*} \\ {{\varvec{\kappa }}_{{\varvec{rz}}}^*} \\ \end{array} }} \right\} \end{aligned}$$
(20)

where the values of above matrix coefficients are given by

$$\begin{aligned} ( {A_{ij}, B_{ij}, C_{ij}, D_{ij}, E_{ij}, F_{ij}, G_{ij}, H_{ij} } )=\mathop \sum \limits _{k=1}^{n} {\bar{Q}}_{ij}^{\left( k \right) } \mathop \int \limits _{z_{k} }^{z_{k+1} } ( {1,z,z^{2},z^{3},z^{4},z^{5},z^{6},z^{7}} )\mathrm{d}z \end{aligned}$$

and

$$\begin{aligned} {\overline{A_{ij}}}= & {} A_{ij} -C_{0} B_{ij},\quad {\widetilde{A_{ij} }} =A_{ij} +C_{0} B_{ij}\\ {\overline{B_{ij}}}= & {} B_{ij} -C_{0} C_{ij},\quad {\widetilde{B_{ij} }} =B_{ij} +C_{0} C_{ij}\\ {\overline{C_{ij}}}= & {} C_{ij} -C_{0} D_{ij},\quad {\widetilde{C_{ij} }} =C_{ij} +C_{0} D_{ij}\\ {\overline{D_{ij}}}= & {} D_{ij} -C_{0} E_{ij},\quad {\widetilde{D_{ij} }} =D_{ij} +C_{0} E_{ij}\\ {\overline{E_{ij}}}= & {} E_{ij} -C_{0} F_{ij},\quad {\widetilde{E_{ij} }} =E_{ij} +C_{0} F_{ij}\\ {\overline{F_{ij}}}= & {} F_{ij} -C_{0} G_{ij},\quad {\widetilde{F_{ij} }} =F_{ij} +C_{0} G_{ij}\\ {\overline{G_{ij}}}= & {} G_{ij} -C_{0} H_{ij},\quad {\widetilde{G_{ij} }} =G_{ij} +C_{0} H_{ij} \end{aligned}$$

where \(i, j= 1, 2, 4, 5, 6\) and \(C_0 =\left( {\frac{1}{R_{s} }-\frac{1}{R_{r} }} \right) \).

Appendix 2

Rigidity matrix of laminates using model 2:

(21)
$$\begin{aligned} \left\{ {{\begin{array}{l} {{\varvec{Q}}_{{\varvec{s}}}} \\ {{\varvec{Q}}_{{\varvec{r}}}} \\ {{\varvec{S}}_{{\varvec{s}}}} \\ {{\varvec{S}}_{{\varvec{r}}} } \\ {{\varvec{Q}}_{{\varvec{s}}}^*} \\ {{\varvec{Q}}_{{\varvec{r}}}^*} \\ {{\varvec{S}}_{{\varvec{s}}}^*} \\ {{\varvec{S}}_{{\varvec{r}}}^*} \\ \end{array} }} \right\}= & {} \left[ {{\begin{array}{llllllll} {\varvec{A}}_\mathbf{44}&{}\quad {\varvec{A}}_\mathbf{45}&{}\quad {\varvec{B}}_\mathbf{44}&{}\quad {\varvec{B}}_\mathbf{45}&{}\quad {\varvec{C}}_\mathbf{44}&{}\quad {\varvec{C}}_\mathbf{45}&{}\quad {\varvec{D}}_\mathbf{44}&{}\quad {\varvec{D}}_\mathbf{45}\\ {\varvec{A}}_\mathbf{45}&{}\quad {\varvec{A}}_\mathbf{55}&{}\quad {\varvec{B}}_\mathbf{45}&{}\quad {\varvec{B}}_\mathbf{55}&{}\quad {\varvec{C}}_\mathbf{45}&{}\quad {\varvec{C}}_\mathbf{55}&{}\quad {\varvec{D}}_\mathbf{45}&{}\quad {\varvec{D}}_\mathbf{55}\\ {\varvec{B}}_\mathbf{44}&{}\quad {\varvec{B}}_\mathbf{45}&{}\quad {\varvec{C}}_\mathbf{44}&{}\quad {\varvec{C}}_\mathbf{45}&{}\quad {\varvec{D}}_\mathbf{44}&{}\quad {\varvec{D}}_\mathbf{45}&{}\quad {\varvec{E}}_\mathbf{44}&{}\quad {\varvec{E}}_\mathbf{45}\\ {\varvec{B}}_\mathbf{45}&{}\quad {\varvec{B}}_\mathbf{55}&{}\quad {\varvec{C}}_\mathbf{45}&{}\quad {\varvec{C}}_\mathbf{55}&{}\quad {\varvec{D}}_\mathbf{45}&{}\quad {\varvec{D}}_\mathbf{55}&{}\quad {\varvec{E}}_\mathbf{45}&{}\quad {\varvec{E}}_\mathbf{55}\\ {\varvec{C}}_\mathbf{44}&{}\quad {\varvec{C}}_\mathbf{45}&{}\quad {\varvec{D}}_\mathbf{44}&{}\quad {\varvec{D}}_\mathbf{45}&{}\quad {\varvec{E}}_\mathbf{44}&{}\quad {\varvec{E}}_\mathbf{45}&{}\quad {\varvec{F}}_\mathbf{44}&{}\quad {\varvec{F}}_\mathbf{45}\\ {\varvec{C}}_\mathbf{45}&{}\quad {\varvec{C}}_\mathbf{55}&{}\quad {\varvec{D}}_\mathbf{45}&{}\quad {\varvec{D}}_\mathbf{55}&{}\quad {\varvec{E}}_\mathbf{45}&{}\quad {\varvec{E}}_\mathbf{55}&{}\quad {\varvec{F}}_\mathbf{45}&{}\quad {\varvec{F}}_\mathbf{55}\\ {\varvec{D}}_\mathbf{44}&{}\quad {\varvec{D}}_\mathbf{45}&{}\quad {\varvec{E}}_\mathbf{44}&{}\quad {\varvec{E}}_\mathbf{45}&{}\quad {\varvec{F}}_\mathbf{44}&{}\quad {\varvec{F}}_\mathbf{45}&{}\quad {\varvec{G}}_\mathbf{44}&{}\quad {\varvec{G}}_\mathbf{45}\\ {\varvec{D}}_\mathbf{45}&{}\quad {\varvec{D}}_\mathbf{55}&{}\quad {\varvec{E}}_\mathbf{45}&{}\quad {\varvec{E}}_\mathbf{55}&{}\quad {\varvec{F}}_\mathbf{45}&{}\quad {\varvec{F}}_\mathbf{55}&{}\quad {\varvec{G}}_\mathbf{45}&{}\quad {\varvec{G}}_\mathbf{55}\\ \end{array} }} \right] \left\{ {{\begin{array}{l} {{\varvec{\epsilon }}_{{\varvec{szo}}} } \\ {{\varvec{\epsilon }}_{{\varvec{rzo}}} } \\ {{\varvec{\kappa }}_{{\varvec{sz}}}} \\ {{\varvec{\kappa }}_{{\varvec{rz}}}} \\ {{\varvec{\epsilon }}_{{\varvec{szo}}}^*} \\ {{\varvec{\epsilon }}_{{\varvec{rzo}}}^*} \\ {{\varvec{\kappa }}_{{\varvec{sz}}}^*} \\ {{\varvec{\kappa }}_{{\varvec{rz}}}^*} \\ \end{array} }} \right\} \end{aligned}$$
(22)

where the values of above matrix coefficients are given by

$$\begin{aligned} ( {A_{ij}, B_{ij}, C_{ij}, D_{ij}, E_{ij}, F_{ij}, G_{ij} } )=\mathop \sum \limits _{k=1}^{n} {\bar{Q}}_{ij}^{\left( k \right) } \mathop \int \limits _{z_{k} }^{z_{k+1} } ( {1,z,z^{2},z^{3},z^{4},z^{5},z^{6}} )\mathrm{d}z \end{aligned}$$

where \(i, j= 1, 2, 4, 5, 6\).

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Thakur, S.N., Ray, C. The effect of thickness coordinate to radius ratio on free vibration of moderately thick and deep doubly curved cross-ply laminated shell. Arch Appl Mech 86, 1119–1132 (2016). https://doi.org/10.1007/s00419-015-1082-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00419-015-1082-8

Keywords

Search

Navigation