Skip to main content
SpringerLink
Log in

An efficient wavelet collocation method for nonlinear two-space dimensional Fisher–Kolmogorov–Petrovsky–Piscounov equation and two-space dimensional extended Fisher–Kolmogorov equation

  • Original Article
  • Published:
Engineering with Computers Aims and scope Submit manuscript

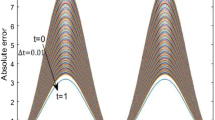
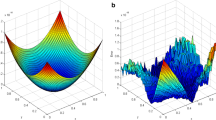
Abstract

In this paper, we consider two-space dimensional nonlinear Fisher–Kolmogorov–Petrovsky–Piscounov (Fisher–KPP) equation and two-space dimensional nonlinear fourth-order extended Fisher–Kolmogorov (EFK) equation which have a lot of applications in different branches of science, especially in mathematical biology. We present a wavelet collocation method based on Chebyshev wavelets combined with two different time discretization schemes. To generate more accurate results for Fisher–KPP equation, in time variable discretization, forward Euler timestepping scheme along with Taylor series expansion is used. Resultant time-discretized scheme has second-order accuracy and includes second-order time derivative which is evaluated from governing equation. On the other hand for EFK equation forward Euler timestepping scheme with first-order accuracy is used. Then space variables situated in the semi-discrete schemes are discretized with Chebyshev wavelet series expansion. In this way a full discrete scheme is obtained. By this approach obtainment of numerical solution of considered partial differential equations is turned into an operation of finding solution of an algebraic system of equations. Actually the solution of the algebraic system of equations is wavelet coefficients in wavelet series expansion. Putting these coefficients into Chebyshev wavelet series expansion the numerical solution of considered partial differential equations can be obtained successively. The main objective of this paper is to demonstrate that Chebyshev wavelet-based method is accurate, efficient, and reliable for nonlinear two-space dimensional high-order partial differential equations. Six test problems are considered and \(L_2\), \(L_{\infty }\) error norms are calculated for comparison of our numerical results with exact results whenever they are available. Also numerical results are plotted for comparison with reference solutions. The obtained results certify the applicability and efficiency of the suggested method for Fisher–KPP and EFK equations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

References

  1. Zheng S (2004) Nonlinear evolution equations. Monographs and surveys in pure and applied mathematics. Chapman & Hall/CRC, CRC Press, Boca Raton

    MATH  Google Scholar 

  2. Dehghan M, Abbaszadeh M (2018) Solution of multi-dimensional Klein–Gordon–Zakharov and Schrödinger/Gross–Pitaevskii equations via local Radial Basis Functions-Differential Quadrature (RBF-DQ) technique on non-rectangular computational domains. Eng Anal Bound Elements 92:156–170

    MathSciNet  MATH  Google Scholar 

  3. Dehghan M, Abbaszadeh M (2017) Numerical investigation based on direct meshless local Petrov Galerkin (direct MLPG) method for solving generalized Zakharov system in one and two dimensions and generalized Gross-Pitaevskii equation. Eng Comput 33(4):983–996

    Google Scholar 

  4. Fisher RA (1937) The wave of advance of advantageous genes. Ann Eugen 7(4):355–369

    MATH  Google Scholar 

  5. Kolmogorov A, Petrovsky N, Piscounov S (1937) Étude de l’équations de la diffusion avec croissance de la quantité de matière et son application a un problème biologique. Bull Univ Moskou 1:1–25

    Google Scholar 

  6. Roessler J, Hüssner H (1997) Numerical solution of the 1+ 2 dimensional Fisher’s equation by finite elements and the Galerkin method. Math Comput Modell 25:57–67

    MathSciNet  MATH  Google Scholar 

  7. José C (1969) Diffusion in nonlinear multiplicative media. J Math Phys 10:1862–1868

    Google Scholar 

  8. Qin W, Ding D, Ding X (2015) Two boundedness and monotonicity preserving methods for a generalized Fisher-KPP equation. Appl Math Comput 252:552–567

    MathSciNet  MATH  Google Scholar 

  9. Dehghan M (2004) Numerical solution of the three-dimensional advection-diffusion equation. Appl Math Comput 150(1):5–19

    MathSciNet  MATH  Google Scholar 

  10. Oruç Ö (2018) A new numerical treatment based on Lucas polynomials for 1D and 2D sinh-Gordon equation. Commun Nonlinear Sci Numer Simul 57:14–25

    MathSciNet  Google Scholar 

  11. Dehghan M, Abbaszadeh M (2017) A local meshless method for solving multi-dimensional Vlasov–Poisson and Vlasov–Poisson–Fokker–Planck systems arising in plasma physics. Eng Comput 33(4):961–981

    Google Scholar 

  12. Tang S, Qin S, Weber RO (1993) Numerical studies on 2-dimensional reaction–diffusion equations. J Aust Math Soc Sen B 35:223–243

    MathSciNet  MATH  Google Scholar 

  13. Macias-Diaz JE (2011) A bounded finite-difference discretization of a two-dimensional diffusion equation with logistic nonlinear reaction. Int J Mod Phys C 22(09):953–966

    MathSciNet  MATH  Google Scholar 

  14. Parand K, Nikarya M (2017) A numerical method to solve the 1D and the 2D reaction diffusion equation based on Bessel functions and Jacobian free Newton-Krylov subspace methods. Eur Phys J Plus. https://doi.org/10.1140/epjp/i2017-11787-x

    Article  Google Scholar 

  15. Coullet P, Elphick C, Repaux D (1987) Nature of spatial chaos. Phys Rev Lett 58:431–434

    MathSciNet  Google Scholar 

  16. Dee GT, van Saarloos W (1988) Bistable systems with propagating fronts leading to pattern formation. Phys Rev Lett 60:2641–2644

    Google Scholar 

  17. van Saarloos W (1987) Dynamical velocity selection: marginal stability. Phys Rev Lett 58:2571–2574

    Google Scholar 

  18. van Saarloos W (1988) Front propagation into unstable states: marginal stability as a dynamical mechanism for velocity selection. Phys Rev Lett A 37:211–229

    MathSciNet  Google Scholar 

  19. Khiari N, Omrani K (2011) Finite difference discretization of the extended Fisher–Kolmogorov equation in two dimensions. Comput Math Appl 62:4151–4160

    MathSciNet  MATH  Google Scholar 

  20. Hornreich RM, Luban M, Shtrikman S (1975) Critical behaviour at the onset of k-space instability at the \(\lambda\) line. Phys Rev Lett 35:1678–1681

    Google Scholar 

  21. Ahlers G, Cannell DS (1983) Vortex-front propagation in rotating Couette–Taylor flow. Phys Rev Lett 50:1583–1586

    Google Scholar 

  22. Aronson DG, Weinberger HF (1978) Multidimensional nonlinear diffusion arising in population genetics. Adv Math 30:33–67

    MathSciNet  MATH  Google Scholar 

  23. Zhu G (1982) Experiments on director waves in nematic liquid crystals. Phys Rev Lett 49:1332–1335

    Google Scholar 

  24. He D (2016) On the \(L^{\infty }\)-norm convergence of a three-level linearly implicit finite difference method for the extended Fisher–Kolmogorov equation in both 1D and 2D. Comput Math Appl 71(12):2594–2607

    MathSciNet  Google Scholar 

  25. Mohanty RK, Kaur D (2017) High accuracy compact operator methods for two-dimensional fourth order nonlinear parabolic partial differential equations. Comput Methods Appl Math 17:4. https://doi.org/10.1515/cmam-2016-0047

  26. Liu F, Zhao X, Liu B (2017) Fourier pseudo-spectral method for the extended Fisher–Kolmogorov equation in two dimensions. Adv Differ Equ 2017:94

    MathSciNet  MATH  Google Scholar 

  27. Ilati M, Dehghan M (2018) Direct local boundary integral equation method for numerical solution of extended Fisher–Kolmogorov equation. Eng Comput 34:203–213

    Google Scholar 

  28. Li X, Zhang L (2018) Error estimates of a trigonometric integrator sine pseudo-spectral method for the extended Fisher–Kolmogorov equation. Appl Numer Math 131:39–53

    MathSciNet  MATH  Google Scholar 

  29. Glowinski R, Lawton W, Ravachol M, Tenenbaum E (1990) Wavelet solutions of linear and non-linear elliptic, parabolic and hyperbolic problems in one space dimension. Comput Methods Appl Sci Eng SIAM Chap 4:55–120

    MATH  Google Scholar 

  30. Qian S, Weiss J (1993) Wavelets and the numerical solution of partial differential equations. J Comput Phys 106:155–175

    MathSciNet  MATH  Google Scholar 

  31. Qian S, Weiss J (1993) Wavelets and the numerical solution of boundary value problems. Appl Math Lett 6:47–52

    MathSciNet  MATH  Google Scholar 

  32. Amaratunga A, Williams J, Qian S, Weiss J (1994) Wavelet Galerkin solutions for one-dimensional partial differential equations. Int J Numer Methods Eng 37:2703–2716

    MathSciNet  MATH  Google Scholar 

  33. Rathish Kumar BV, Mehra M (2005) Wavelet Taylor Galerkin method for the Burgers equation. BIT Numer Math Vol 45:543–560

    MathSciNet  MATH  Google Scholar 

  34. Mehra M, Kumar BVR (2005) Time accurate solution of advection diffusion problems by wavelet Taylor Galerkin method. Commun Numer Methods Eng 21:313–326

    MathSciNet  MATH  Google Scholar 

  35. Priyadarshi G, Kumar BVR (2018) Wavelet Galerkin schemes for higher order time dependent partial differential equations. Numer Methods Partial Differ Equ 34:982–1008

    MathSciNet  MATH  Google Scholar 

  36. Lepik Ü (2007) Application of the Haar wavelet transform to solving integral and differential equations. Proc Estonian Acad Sci Phys Math 56(1):28–46

    MathSciNet  MATH  Google Scholar 

  37. Lepik Ü (2005) Numerical solution of differential equations using Haar wavelets. Math Comput Simul 68:127–143

    MathSciNet  MATH  Google Scholar 

  38. Lepik Ü (2007) Numerical solution of evolution equations by the Haar wavelet method. Appl Math Comput 185:695–704

    MathSciNet  MATH  Google Scholar 

  39. Lepik Ü (2011) Solving PDEs with the aid of two-dimensional Haar wavelets. Comput Math Appl 61:1873–1879

    MathSciNet  MATH  Google Scholar 

  40. Oruç Ö, Bulut F, Esen A (2015) A haar wavelet-finite difference hybrid method for the numerical solution of the modified burgers’ equation. J Math Chem 53(7):1592–1607

    MathSciNet  MATH  Google Scholar 

  41. Oruç Ö, Bulut F, Esen A (2016) Numerical solutions of regularized long wave equation by Haar wavelet method. Mediter J Math 13(5):3235–3253

    MathSciNet  MATH  Google Scholar 

  42. Oruç Ö, Esen A, Bulut F A Haar wavelet collocation method for coupled nonlinear Schrödinger–KdV equations. Int J Mod Phys C. https://doi.org/10.1142/S0129183116501035

  43. Shi Z, Cao Y, Chen QJ (2012) Solving 2D and 3D Poisson equations and biharmonic equations by the Haar wavelet method. Appl Math Model 36:5143–5161

    MathSciNet  MATH  Google Scholar 

  44. Jiwari R (2015) A hybrid numerical scheme for the numerical solution of the Burgers’ equation. Comput Phys Commun 188:59–67

    MathSciNet  MATH  Google Scholar 

  45. Haq S, Ghafoor A (2018) An efficient numerical algorithm for multi-dimensional time dependent partial differential equations. Comput Math Appl 75(8):2723–2734

    MathSciNet  MATH  Google Scholar 

  46. Oruç Ö, Esen A, Bulut F (2018) A haar wavelet approximation for two-dimensional time fractional reaction-subdiffusion equation. https://doi.org/10.1007/s00366-018-0584-8

  47. Razzaghi M, Yousefi S (2000) Legendre wavelets direct method for variational problems. Math Comput Simul 53:185–192

    MathSciNet  Google Scholar 

  48. Sahu PK, Saha Ray S (2015) Legendre wavelets operational method for the numerical solutions of nonlinear Volterra integro-differential equations system. Appl Math Comput 256:715–723

    MathSciNet  MATH  Google Scholar 

  49. Lakestani M, Saray BN, Dehghan M (2011) Numerical solution for the weakly singular Fredholm integro-differential equations using Legendre multiwavelets. J Comput Appl Math 235(11):3291–3303

    MathSciNet  MATH  Google Scholar 

  50. Zhou F, Xu X (2016) Numerical solutions for the linear and nonlinear singular boundary value problems using Laguerre wavelets. Adv Differ Equ 2016:17

    MathSciNet  MATH  Google Scholar 

  51. Babolian E, Fattahzadeh F (2007) Numerical solution of differential equations by using Chebyshev wavelet operational matrix of integration. Appl Math Comput 188:417–426

    MathSciNet  MATH  Google Scholar 

  52. Zhu L, Fan Q (2012) Solving fractional nonlinear Fredholm integro-differential equations by the second kind Chebyshev wavelet. Commun Nonlinear Sci Numer Simul 17:2333–2341

    MathSciNet  MATH  Google Scholar 

  53. Zhou F, Xu X (2014) Numerical solution of the convection diffusion equations by the second kind Chebyshev wavelets. Appl Math Comput 247:353–367

    MathSciNet  MATH  Google Scholar 

  54. Heydari MH, Hooshmandasl MR, Maalek Ghaini FM (2014) A new approach of the Chebyshev wavelets method for partial differential equations with boundary conditions of the telegraph type. Appl Math Model 38:1597–1606

    MathSciNet  MATH  Google Scholar 

  55. Yang C, Hou J (2013) Chebyshev wavelets method for solving Bratu’s problem. Bound Value Probl 142:1–9

    MathSciNet  MATH  Google Scholar 

  56. Celik I (2018) Free vibration of non-uniform Euler–Bernoulli beam under various supporting conditions using Chebyshev wavelet collocation method Author links open overlay panel. Appl Math Modell 54:268–280

    MATH  Google Scholar 

  57. Oruç Ö (2017) A computational method based on Hermite wavelets for two-dimensional Sobolev and regularized long wave equations in fluids. Numer Methods Partial Differ Equ 00:1–23. https://doi.org/10.1002/num.22232

    Article  MathSciNet  Google Scholar 

  58. Oruç Ö (2017) A numerical procedure based on Hermite wavelets for two-dimensional hyperbolic telegraph equation. Eng Comput. https://doi.org/10.1007/s00366-017-0570-6

  59. Kumar KH, Vijesh VA (2016) chebyshev wavelet quasilinearization scheme for coupled nonlinear Sine–Gordon equations. ASME J Comput Nonlinear Dyn 12(1):011018–011018-5. https://doi.org/10.1115/1.4035056

  60. Donea J (1984) A Taylor–Galerkin method for convective transport problems. Int J Numer Methods Eng 20:101–119

    MATH  Google Scholar 

  61. Donea J, Giuliani S, Laval H (1984) Time-accurate solution of advection–diffusion problems by finite elements. Comput Methods Appl Mech Eng 45:123–146

    MathSciNet  MATH  Google Scholar 

  62. Donea J, Quartapelle L, Selmin V (1987) An analysis of time discretization in finite element solution of hyperbolic problems. J Comput Phys 70:463–499

    MathSciNet  MATH  Google Scholar 

  63. Oruç Ö, Bulut F, Esen A (2016) Numerical solution of the KdV equation by Haar wavelet method. Pramana J Phys 87:94. https://doi.org/10.1007/s12043-016-1286-7

    Article  MATH  Google Scholar 

  64. Travis E (2007) Oliphant. Python Sci Comput Comput Sci Eng 9(3):10–20

    Google Scholar 

  65. van der Walt S, Colbert SC, Varoquaux G (2011) The NumPy array: a structure for efficient numerical computation. Comput Sci Eng 13(2):22–30

    Google Scholar 

  66. Hunter JD (2007) Matplotlib: a 2D graphics environment. Comput Sci Eng 9(3):90–95

    Google Scholar 

  67. Alnaes MS, Blechta J, Hake J, Johansson A, Kehlet B, Logg A, Richardson C, Ring J, Rognes ME, Wells GN (2015) The FEniCS Project Version 1.5, Archive of Numerical Software

  68. Logg A, Wells GN (2010) DOLFIN: automated finite element computing. ACM Trans Math Softw 37

Download references

Acknowledgements

The author would like to thank to the reviewers for their useful comments and suggestions towards improvement of the paper.

Author information

Authors and Affiliations

  1. Eğil Vocational and Technical Anatolian High School, Diyarbakır, Turkey

    Ömer Oruç

Authors
  1. Ömer Oruç
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Ömer Oruç.

Additional information

Publisher’s Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Oruç, Ö. An efficient wavelet collocation method for nonlinear two-space dimensional Fisher–Kolmogorov–Petrovsky–Piscounov equation and two-space dimensional extended Fisher–Kolmogorov equation. Engineering with Computers 36, 839–856 (2020). https://doi.org/10.1007/s00366-019-00734-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00366-019-00734-z

Keywords

Mathematics Subject Classification

Search

Navigation