A balance index for phylogenetic trees based on rooted quartets

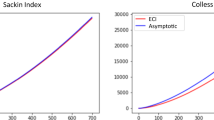
Abstract

We define a new balance index for rooted phylogenetic trees based on the symmetry of the evolutive history of every set of 4 leaves. This index makes sense for multifurcating trees and it can be computed in time linear in the number of leaves. We determine its maximum and minimum values for arbitrary and bifurcating trees, and we provide exact formulas for its expected value and variance on bifurcating trees under Ford’s $\alpha$-model and Aldous’ $\beta$-model and on arbitrary trees under the $\alpha$–$\gamma$-model.

Appendices

Appendices

1.1 A.1: An alternative derivation of the variance of $rQIB _n$ under the Yule model

In this section we give an alternative proof of the following result.

Proposition 4

Under the Yule model,

$$\begin{aligned} Var_{Y}( rQIB _n)=\left( {\begin{array}{c}n\\ 4\end{array}}\right) \frac{5 n^4+ 30 n^3+ 118 n^2 + 408 n+ 630}{33075}. \end{aligned}$$

Proof

By Lemma 7, $rQIB$ on ${\mathcal {BT}}_n$ is a bifurcating recursive tree shape statistic satisfying the recurrence

$$\begin{aligned} rQIB (T\star T')= rQIB (T)+ rQIB (T')+f_{ rQIB }(|L(T)|,|L(T')|) \end{aligned}$$

with $f_{ rQIB }(a,b)=\left( {\begin{array}{c}a\\ 2\end{array}}\right) \left( {\begin{array}{c}b\\ 2\end{array}}\right)$. Then, it satisfies the hypothesis in Cor. 1 of Cardona et al. (2013) with

$$\begin{aligned} \begin{aligned} \displaystyle \varepsilon (a,b-1)&\displaystyle =f_{ rQIB }(a,b)-f_{ rQIB }(a,b-1)\\&\displaystyle =\left( {\begin{array}{c}a\\ 2\end{array}}\right) \left( {\begin{array}{c}b\\ 2\end{array}}\right) -\left( {\begin{array}{c}a\\ 2\end{array}}\right) \left( {\begin{array}{c}b-1\\ 2\end{array}}\right) =(b-1)\left( {\begin{array}{c}a\\ 2\end{array}}\right) \\ \displaystyle R(n-1)&\displaystyle =E_{Y}( rQIB _n)-E_{Y}( rQIB _{n-1})\\&\displaystyle =\frac{1}{3}\left( {\begin{array}{c}n\\ 4\end{array}}\right) -\frac{1}{3}\left( {\begin{array}{c}n-1\\ 4\end{array}}\right) =\frac{1}{3}\left( {\begin{array}{c}n-1\\ 3\end{array}}\right) \end{aligned} \end{aligned}$$

Since $E_{Y}( rQIB _1)=0$ and $f_{ rQIB }(n-1,1)=0$, applying the aforementioned result from Cardona et al. (2013) we have that

$$\begin{aligned} E_{Y}( rQIB _n^2) \displaystyle= & {} \frac{n}{n-1} E_{Y}( rQIB _{n-1}^2)+\frac{4}{n-1} \sum _{k=1}^{n-2} \varepsilon (k,n-k-1) E_{Y}( rQIB _k) \\&\displaystyle +\frac{2}{n-1} \sum _{k=1}^{n-2} R(n-k-1)E_{Y}( rQIB _k) \\&\displaystyle +\frac{1}{n-1}\sum _{k=1}^{n-2} (f_{ rQIB }(k,n-k)^2- f_{ rQIB }(k,n-k-1)^2)\\ \displaystyle= & {} \frac{n}{n-1} E_{Y}( rQIB _{n-1}^2)+\frac{4}{3(n-1)} \sum _{k=1}^{n-2} (n-k-1)\left( {\begin{array}{c}k\\ 2\end{array}}\right) \left( {\begin{array}{c}k\\ 4\end{array}}\right) \\&\displaystyle +\frac{2}{9(n-1)} \sum _{k=1}^{n-2} \left( {\begin{array}{c}n-k-1\\ 3\end{array}}\right) \left( {\begin{array}{c}k\\ 4\end{array}}\right) \\&\displaystyle +\frac{1}{n-1}\sum _{k=1}^{n-2} \left( {\begin{array}{c}k\\ 2\end{array}}\right) ^2\Bigg (\left( {\begin{array}{c}n-k\\ 2\end{array}}\right) ^2-\left( {\begin{array}{c}n-k-1\\ 2\end{array}}\right) ^2\Bigg )\\ \displaystyle= & {} \frac{n}{n-1} E_{Y}( rQIB _{n-1}^2) +\frac{n}{3}\left( {\begin{array}{c}n-2\\ 4\end{array}}\right) \frac{15 n^2 - 35 n + 6}{420}\\&\displaystyle +\frac{n}{9} \left( {\begin{array}{c}n-2\\ 4\end{array}}\right) \frac{n^2 - 13 n + 42}{840}\\&\displaystyle + n\left( {\begin{array}{c}n-2\\ 2\end{array}}\right) \frac{3 n^4 - 18 n^3 + 41 n^2 - 42 n + 36}{1680}\\ \displaystyle= & {} \frac{n}{n-1} E_{Y}( rQIB _{n-1}^2) \\&\displaystyle + \frac{n(n-2)(n-3)(253 n^4-2014 n^3 +6119 n^2-7430 n+ 3504)}{181440} \end{aligned}$$

Dividing by n both sides of this expression for $E_{Y}( rQIB ^2_n)$ and setting $y_n=E_{Y}( rQIB ^2_n)/n$, we obtain the recurrence

$$\begin{aligned} y_n =\displaystyle y_{n-1}+ \frac{(n-2)(n-3)(253 n^4-2014 n^3 +6119 n^2-7430 n+ 3504)}{181440}. \end{aligned}$$

Since $y_0=y_1=0$, its solution is

$$\begin{aligned} y_n \displaystyle= & {} \sum _{k=2}^n \frac{(k-2)(k-3)(253 k^4-2014 k^3 +6119 k^2-7430 k+ 3504)}{181440}\\ \displaystyle= & {} \frac{(n - 3) (n - 2) (n - 1) (1265 n^4 - 7110 n^3 + 14419 n^2 - 4086 n + 5040)}{6350400} \end{aligned}$$

from where we obtain

$$\begin{aligned} E_{Y}( rQIB _n^2)&=ny_n\\&\displaystyle = \left( {\begin{array}{c}n\\ 4\end{array}}\right) \frac{1265 n^4 - 7110 n^3 + 14419 n^2 - 4086 n + 5040}{264600}. \end{aligned}$$

Finally

$$\begin{aligned} Var_{Y}( rQIB _n) \displaystyle= & {} E_{Y}( rQIB _n^2)-E_{Y}( rQIB _n)^2\\ \displaystyle= & {} \left( {\begin{array}{c}n\\ 4\end{array}}\right) \frac{1265 n^4 - 7110 n^3 + 14419 n^2 - 4086 n + 5040}{264600}-\frac{1}{9}\left( {\begin{array}{c}n\\ 4\end{array}}\right) ^2\\ \displaystyle= & {} \left( {\begin{array}{c}n\\ 4\end{array}}\right) \frac{5 n^4 + 30 n^3 + 118 n^2 + 408 n + 630}{33075}, \end{aligned}$$

as we claimed. $\square$

1.2 A.2: Some tables used in Sect. 5

See Tables 3, 4 and 5.

Table 3 Coefficients of the probabilities of the trees in ${\mathcal {BT}}_k^*$, for $k=5,6,7,8$, in the formula for the variance of $rQIB _n$

Table 4 Probabilities under the $\alpha$-model of the trees involved in the formula for the variance of $rQIB _n$

Table 5 Probabilities under the $\beta$-model of the trees involved in the formula for the variance of $rQIB _n$