Geometric singular perturbation analysis of a dynamical target mediated drug disposition model

Abstract

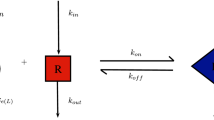
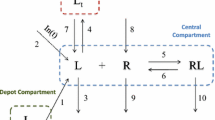
In this paper we present a mathematical analysis of a pharmacological ODE model for target mediated drug disposition (TMDD). It is known that solutions of this model undergo four qualitatively different phases. In this paper we provide a mathematical identification of these separate phases by viewing the TMDD model as a singular perturbed system. Our analysis is based on geometric singular perturbation theory and we believe that this approach systemizes—and sheds further light on—the scalings arguments used by previous authors. In particular, we present a novel description of the third phase through a distinguished solution of a nonlinear differential equation. We also describe the solution curve for large values of initial drug doses and recover, en route, a result by Aston et al. (J Math Biol 68(6):1453–1478, 2014) on rebounding using our alternative perturbation approach. Finally, from our main result we derive a new method for estimating the parameters of the system in the event that detailed data is available. Ideally our approach to the TMDD model should stimulate further research into applications of these methods to more complicated models in pharmacology.

Appendices

Proof of Lemma 3

We consider

$$\begin{aligned} \dot{x}_2&=-x_2y_2+z,\nonumber \\ \dot{y}_2&=\beta -x_2y_2+z, \end{aligned}$$

(70)

with $z\in [0,\infty )$ a parameter. A simple phase plane analysis shows that every point in the first quadrant moves towards $y_2\rightarrow \infty$ with $x_2>0$ bounded. To study $y_2\rightarrow \infty$, we use a version of Poincaré compactification Meiss (2007) that follows our approach for the blowup (45). In particular, we view $(x_2,y_2)$ as coordinates on $(\bar{x},\bar{y},\bar{\epsilon }) \in S^2$ defined by

$$\begin{aligned} x_2&=\bar{\epsilon }^{-1/2}\bar{x},\quad y_2 =\bar{\epsilon }^{-1/2} \bar{y}, \end{aligned}$$

and then study $y_2\rightarrow \infty$ by setting

$$\begin{aligned} \epsilon _3&=y_2^{-2},\quad x_3 =x_2 y_2^{-1}, \end{aligned}$$

(71)

see also (49). Notice that

$$\begin{aligned} \epsilon _3&= \bar{y}^{-2} \bar{\epsilon },\quad x_3 = \bar{y}^{-1}\bar{x}, \end{aligned}$$

and hence the coordinates $(\epsilon _3,x_3)$ cover $\bar{y}>0$ of $(\bar{x},\bar{y},\bar{\epsilon }) \in S^2$. Inserting (71) into (70) gives (56)$_{r_3=0}$ within $z=\text {const}.$ By Proposition 2 (and the existence of $\mathcal {C}_3^3$) there exists a non-unique center manifold of $(\epsilon _3,x_3)=(0,0)$ in these coordinates with asymptotics

$$\begin{aligned} x_3 \approx \epsilon _3 z \end{aligned}$$

for $\epsilon _3\rightarrow 0$. $\epsilon _3$ decreases along this manifold. Returning to the $(x_2,y_2)$-variables using (71) gives the first result in Lemma 3. Secondly, to study $x_2\rightarrow \infty$ we insert

$$\begin{aligned} \epsilon _1&=x_2^{-2},\quad y_1 =y_2 x_2^{-1}, \end{aligned}$$

see also (48), into (70). Notice that

$$\begin{aligned} \epsilon _1&= \bar{x}^{-2} \bar{\epsilon },\quad y_1 = \bar{x}^{-1}\bar{y}, \end{aligned}$$

and hence the coordinates $(\epsilon _1,y_1)$ cover $\bar{x}>0$ of $(\bar{x},\bar{y},\bar{\epsilon }) \in S^2$. This gives (53) within $z=\text {const}.$ and according to Proposition 1 (and the existence of $\mathcal {C}_1^3$) there exists a unique, attracting center manifold $\varGamma _1(z)$ of $(\epsilon _1,y_1)=(0,0)$ in these coordinates with asymptotics

$$\begin{aligned} y_1 \approx \epsilon _1 (z+\beta ), \end{aligned}$$

for $\epsilon _1\rightarrow 0$. $\epsilon _1$ increases along this manifold. Returning to the $(x_2,y_2)$-variables then completes the proof of Lemma 3.

Proof of Theorem 2

The uniformity of $\varGamma _\epsilon \cap \mathcal {B}\rightarrow \varGamma _0\cap \mathcal {B}$ for $x_0\in (1,c]$ for any fixed c follows from the proof of Theorem 1. In particular, we note that if $x_0=1$ then the initial condition belongs to $\mathcal {F}$ and hence $\varGamma ^2=\emptyset$. To study $x_0\gg 1$ we introduce u by

$$\begin{aligned} u = x^{-1}. \end{aligned}$$

(72)

Then $u(0) = x_0^{-1}\ll 1$. Furthermore,

$$\begin{aligned} u'&=-u^2 \left( -y+\epsilon uz -\epsilon \alpha \right) ,\nonumber \\ y'&=-y +\epsilon \beta u (1-y)+\epsilon zu,\nonumber \\ z'&=y-\epsilon u(1+\delta ) z, \end{aligned}$$

(73)

after multiplying the right hand side by u to ensure that $u=0$ is well-defined. Here $\mathcal {S}^2:\,y=0,\,(u,z)\in [0,\infty )^2$ (abusing notation slightly) is normally hyperbolic (linearization gives $-1$ as single non-zero eigenvalue) for any $u\ge 0$. Fenichel’s theory therefore applies and we obtain $\mathcal {S}^2_\epsilon$ in the following form

$$\begin{aligned} \mathcal {S}^2_\epsilon :\quad y = \epsilon u(z+\beta +O(u\epsilon )),\quad (u,z) \in [0,k_1^{-1}]\times [0,k_3], \end{aligned}$$

by a simple calculation. Now, the reduced problem on $\mathcal {S}^2_\epsilon$ is described by the planar system

$$\begin{aligned} \dot{u}&=-u\left( u(\beta +O(u\epsilon ))+\alpha \right) ,\nonumber \\ \dot{z}&= \beta +O(u\epsilon )-\delta z, \end{aligned}$$

(74)

where we have undone the multiplication of the right hand side by u. The time in (74) is therefore the slow time t also used in (6). Notice that $(u,z) = (0,\delta ^{-1}\beta )$ is a hyperbolic equilibrium for these equations with eigenvalues $\alpha$ and $-\delta$. To describe $\varGamma _\epsilon$ for $x_0\gg 1$ we therefore consider an initial condition $(u,z)(0)=(u_0,0)$ of (74) with $0<u_0\ll 1$. See Fig. 11 for a sketch of the phase portrait of (74) near the saddle. Clearly, the forward orbit converges to the (one-sided) stable and unstable manifold of the saddle as $u_0\rightarrow 0^+$. Now, by Sternberg (1958), Sell (1985), there exists a $C^1$-linearization of (74) near $(u,z) = (0,\delta ^{-1}\beta )$ which is also $C^1$ in $\epsilon$. Therefore, although the solution spends an increasing amount of time near the saddle, the forward orbit $\varGamma _\epsilon$ is $O(\epsilon )$-close to $\varGamma _0$ in the (u, y, z)-variables at $u= k_1^{-1}$, uniformly in $u_0>0$. At $u=k_1^{-1}$ (or $x=k_1$ by (72)) we can change back to the (x, y, z)-variables. From here the analysis in Sect. 4 carries over. This completes the proof of the theorem.

Fig. 11

Phase portrait of (74) near the saddle $(u,z) = (0,\delta ^{-1}\beta )$ for $\epsilon =0$ (in blue) and $0<\epsilon \ll 1$ (in red)