Skip to main content
SpringerLink
Log in

Analysis of hydrodynamic and thermal dispersion in porous media by means of a local approach

  • Original
  • Published:
Heat and Mass Transfer Aims and scope Submit manuscript

    We’re sorry, something doesn't seem to be working properly.

    Please try refreshing the page. If that doesn't work, please contact support so we can address the problem.

Abstract

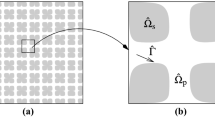
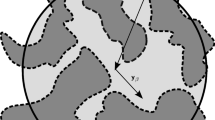
A pore scale analysis is implemented in this numerical study to investigate the behavior of microscopic inertia and thermal dispersion in a porous medium with a periodic structure. The macroscopic characteristics of the transport phenomena are evaluated with an averaging technique of the controlling variables at a pore scale level in an elementary cell of the porous structure. The Darcy–Forchheimer model describes the fluid motion through the porous medium while the continuity and Navier–Stokes equations are applied within the unit cell. An average energy equation is employed for the thermal part of the porous medium. The macroscopic pressure loss is computed in order to evaluate the dominant microscopic inertial effects. Local fluctuations of velocity and temperature at the pore scale are instrumental in the quantification of the thermal dispersion through the total effective thermal diffusivity. The numerical results demonstrate that microscopic inertia contributes significantly to the magnitude of the macroscopic pressure loss, in some instances with as much as 70%. Depending on the nature of the porous medium, the thermal dispersion may have a marked bearing on the heat transfer, particularly in the streamwise direction for a highly conducting fluid and certain values of the Peclet number.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14

Similar content being viewed by others

Abbreviations

A fs :

solid /fluid interfacial area

\(\vec{b}\) :

function vector

c p :

specific heat capacity at constant pressure

d :

solid particle diameter

D :

total effective thermal diffusivity tensor

Da :

Darcy number, K/l 2

k :

thermal conductivity tensor

K :

permeability

l :

length of unit cell

\(\bar{\bar{I}}\) :

identity matrix

\(\vec{n}_{\rm fs}\) :

outward unit normal vector from the fluid phase

Pe l :

Peclet number, 〈u〉l/αf

P :

local pressure

\(\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{P}\) :

periodic pressure

ΔP :

macroscopic pressure drop across a unit cell

Pr :

Prandtl number, ν/α

Re l :

Reynolds number, 〈u〉l/ν

T :

microscopic temperature

\(\vec{u}\) :

microscopic velocity vector

u :

x-velocity component

v :

y-velocity component

V :

volume of the unit cell

V f :

fluid phase volume in the unit cell

V s :

solid phase volume in the unit cell

α:

thermal diffusivity

ɛ:

porosity

θ:

non-dimensional temperature

ν:

kinematic viscosity

ψ:

stream function

ρ:

density

f:

fluid phase

s:

solid phase

eff:

effective

fs:

solid/fluid interface

//:

longitudinal direction

⊥:

transverse direction

〈 〉:

volume average value

d:

thermal dispersion

f:

fluid phase

s:

solid phase

*:

non-dimensional variable

′:

fluctuation

References

  1. Darcy H (1856) Les fontaines publiques de la ville de Dijon, Dalmont, Paris, France

  2. Beaver GS, Sparrow EM, Rodenz DE (1973) Influence of bed size on the flow characteristics and porosity of randomly packed beds of spheres. J Appl Mech 40:655–660

    Google Scholar 

  3. Dybbs A, Edwards RV (1984) A new look at porous media fluid mechanics-Darcy to turbulent. In: Bear, Corapcioglu (eds) Fundamentals of transport phenomena in porous media. Martinis Nijhoff Publishers, Dordrecht, pp 199–254

    Google Scholar 

  4. Ergun S (1952) Fluid flow through a packed column. Chem Eng Prog 48:89–94

    Google Scholar 

  5. McDonald IF, El-Sayed MS, Mow K, Dullien FAL (1979) Flow through porous media-Ergun equation revisited. Ind Eng Chem Fund 18:199–208

    Article  Google Scholar 

  6. Chikh S, Boumedien A, Bouhadef K, Lauriat G (1995) Non-darcian forced convection analysis in an annulus partially filled with a porous material. Num Heat Transf Part A 28:707–722

    Article  Google Scholar 

  7. Coulaud O, Morel P, Caltagirone JP (1988) Numerical modeling of non linear effects in laminar flow through a porous medium. J Fluid Mech 190:393–407

    Article  MATH  Google Scholar 

  8. Sahraoui M, Kaviany M (1992) Slip and no-slip boundary condition at the interface of porous plain media. Int J Heat Mass Transf 35:927–943

    Article  MATH  Google Scholar 

  9. Kuwahara F, Nakayama A, Koyama H (1996) A numerical study of thermal dispersion in porous media. J Heat Transf 118:756–761

    Article  Google Scholar 

  10. Ait saada M, Chikh S (1999) Analyse des effets non linéaires dans un milieu poreux, Proc. 14eme Congrés Français de Mécanique, Toulouse, France

  11. Koch DL, Cox RG, Brenner H, Brady JF (1989) The effect of order on dispersion in porous media. J Fluid Mech 200:173–188

    Article  MATH  MathSciNet  Google Scholar 

  12. Gunn DG, Pryce C (1969) Dispersion in packed beds. Trans Inst Chem Eng 47:341–350

    Google Scholar 

  13. Edwards DA, Brenner H, Shapiro M (1991) Dispersion of inert solutes in spatially periodic two-dimensional model porous media. Trans Porous Media 6:337–358

    Article  Google Scholar 

  14. Eidsath A, Carbonell RG, Whitaker S, Herrmann LR (1983) Dispersion in pulsed systems. Part III, comparison between theory and experiments in packed beds. Chem Eng Sci 38:1803–1816

    Article  Google Scholar 

  15. Sahraoui M, Kaviany M (1994) Slip and no-slip temperature boundary conditions at the interface of porous plain media: convection. Int J Heat Mass Transf 37:1029–1044

    Article  MATH  Google Scholar 

  16. Kuwahara F, Nakayama A (1999) Numerical determination of thermal dispersion coefficient using a periodic porous structure. J Heat Transf 121:160–163

    Article  Google Scholar 

  17. Kelkar KM, Choudhury D (1993) Numerical prediction of periodically fully developed natural convection in a vertical channel with surface mounted heat generating blocks. J Heat Transf 36:1133–1145

    Article  MATH  Google Scholar 

  18. Slattery JC (1969) Single-phase flow through porous media. AIChE J 15:866–872

    Article  Google Scholar 

  19. Gray WG (1975) A deviation of the general convection equation for multiphase systems Part 2: mass, momentum, energy and entropy equation. Adv Water Resour 2:131–141

    Google Scholar 

  20. Kaviany M (1995) Principles of heat transfer in porous media, 2nd edn. Springer, Berlin Heidelberg New York

    MATH  Google Scholar 

  21. Patankar SV (1980) Numerical heat transfer and fluid flow. McGrawHill, Hemisphere, Washington DC

    MATH  Google Scholar 

  22. Shonnard DR, Whitaker S (1989) The effective thermal conductivity for a point contact porous media: an experimental study. Int J Heat Mass Transf 32:503–512

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Département de Génie Mécanique, USTHB, B. P. 32, El Alia, Bab Ezzouar, 16111, Algeria

    Mebrouk Ait Saada & Salah Chikh

  2. Department of Mechanical Engineering, The University of Vermont, Burlington, VT, 05405, USA

    Antonio Campo

Authors
  1. Mebrouk Ait Saada
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Salah Chikh
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Antonio Campo
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Antonio Campo.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Saada, M.A., Chikh, S. & Campo, A. Analysis of hydrodynamic and thermal dispersion in porous media by means of a local approach. Heat Mass Transfer 42, 995–1006 (2006). https://doi.org/10.1007/s00231-005-0061-y

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00231-005-0061-y

Keywords

Search

Navigation