Abstract
We introduce the theory of random tensors, which naturally extends the method of random averaging operators in our earlier work (Deng et al. in: Invariant Gibbs measures and global strong solutions for the nonlinear Schrödinger equations in dimension two, arXiv:1910.08492), to study the propagation of randomness under nonlinear dispersive equations. By applying this theory we establish almost-sure local well-posedness for semilinear Schrödinger equations in the full subcritical range relative to the probabilistic scaling (Theorem 1.1). The solution we construct has an explicit expansion in terms of multilinear Gaussians with adapted random tensor coefficients. As a byproduct we also obtain new results concerning regular data and long-time solutions, in particular Theorem 1.6, which provides long-time control for random homogeneous data, demonstrating the highly nontrivial fact that the first energy cascade happens at a much later time than in the deterministic setting. In the random setting, the probabilistic scaling is the natural scaling for dispersive equations, and is different from the natural scaling for parabolic equations. Our theory of random tensors can be viewed as the dispersive counterpart of the existing parabolic theories (regularity structures, para-controlled calculus and renormalization group techniques).



Similar content being viewed by others
Change history
24 November 2021
A random number appeared in the abstract of the HTML version of the article.
Notes
We will assume \(N\ge 1\) throughout, and only “formally” need to replace N by 1/2 in a few places.
Such structure lives on high frequencies and fine scales. It becomes more explicit when considering low regularity solutions, and may be obscured by the dominant coarse scale profile in high regularity solutions.
In fact there is energy cascade in \(u_{\mathrm {ho}}\) at time \(N^{(p-1)(s-s_{cr})}\), dictated by the continuous resonance (CR) equation; see [17, 38]. Note that, the approximation leading to the CR equation does not work in our case as randomization destroys the differentiability in rescaled Fourier space; in fact the solution \(u_{\mathrm {ho}}\) in Theorem 1.6 has no energy cascade at this time. It is currently unknown whether the solution \(u_{\mathrm {ho}}\) in Theorem 1.6, or the corresponding ensemble average, satisfies some effective equation at time \(N^{(p-1)(s-s_{pr})}\).
We remark that, after the submission of this manuscript, the justification of the wave kinetic equation has been done by the first author and Hani in [34] in certain regimes, including the case \(s=s_{pr}\) and \(\nu =0\) (the case \(s>s_{pr}\) still remains open). This is a probabilistically critical result and is not covered by Theorem 1.6.
In particular,
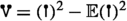 and
and 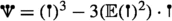 .
.Here \(\pi _>\) is the standard Bony paraproduct.
In practice we will use \(\eta _k=|g_k|^{-1}g_k\), which is uniformly distributed on the unit circle, instead of \(g_k\).
In practice we will also have a ± sign for each index of each tensor, for example a \(+\) sign for the index a in \(h_{abcde}\) or a − sign for the index x in \(h_{uvawx}'\). When precisely defining the merging operations, see Definition 3.6, we will restrict to the cases where for each repeated or paired index, its signs in the two tensors that it appears are the opposite (for example if the sign of b in \(h_{abcde}\) is \(+\) then the sign of u in \(h_{uvawx}'\) must be −, assuming \(b=u\)). In this section (including the simple case Definition 2.4) we will ignore this issue for simplicity.
In [36] we used \(L=N^{1-\delta }\); here we need a smaller value of L which works better in the general setting.
Here \(h^{\circ }_{k}=(u_L)_k\) can be understood as a (0, 1) tensor which has no input variable.
This corresponds to removing \(\circ \)’s and low-frequency \(\bullet \)’s from the iteration trees, or removing low-frequency leaves from the skeleton and flattened trees. In the main proof, in addition to this trimming after merging, we also need to trim the tensors before merging; see (5.23) and (5.24).
See Proposition 5.1 for the full detailed version. In particular there are distinctions between \(h^{(*,0)}\) and \(h^{(*,1)}\) tensors, which we omit here.
Roughly speaking \(\delta =\varepsilon ^{50}\), \(D=\delta ^{-50}\), \(\kappa =D^{50}\) and \(\theta \sim \kappa ^{-50}\) should suffice.
Here a pairing \((k,k_j)\) means \(k=k_j\) and \(\zeta _j=+\).
In this tensor \(k_{\mathcal {V}}\) and \(\lambda _{\mathcal {V}}\) appear as parameters. Note also that the definition does not involve \({\mathcal {P}}\) or \({\mathcal {Y}}\).
If necessary we may replace the unions \(\cup \) by the disjoint unions \(\sqcup \) to avoid repetition of elements.
Here we may also require \(N_{\mathfrak {l}}=N_{{\mathfrak {l}}'}\); whether we do so will not affect the result of this product.
Here and below the phrase “linear combination” will refer to a linear combination with a fixed number of terms and fixed constant coefficients.
In practice, the factor \(\chi _\tau \) will always come with a v which has the form \({\mathcal {I}}_\chi (\cdots )\), so we always have \(v(0)=0\).
Strictly speaking this reduction may not preserve (4.25), but (4.25) is only used to guarantee \(|A_v|\le p-2\) in order to apply Lemma 4.8; after this (4.25) can be replaced by the more general versions where the linear combinations of \(k_j\) and k are fixed \({\mathbb {Z}}^d\) vectors instead of 0, which is preserved under the reduction.
These over-paired variables include a pairing between \((k,k_{P_0})\) and \(k_{Q_0}\) as \(1\in Q_0\), and thus can be treated using the argument in the beginning of the proof.
This matches Definition 3.4.
Strictly speaking the sum over \({\mathscr {O}}\) should carry the coefficients in the linear combination of \({\widetilde{\Psi }}_k^{({\mathcal {S}})}\) that gives (5.22) as above; these are constants, and for simplicity we will treat them as 1.
The same applies to the \(k_E\) variables when measuring \(kk_B\rightarrow k_C\) norms, where \(E={\mathcal {U}}\backslash (B\cup C)\).
i.e. which does not depend on \(\omega \).
Recall that \({\mathcal {Q}}\) is defined in (3.10). Since \({\mathcal {Q}}\) contains the two-element pairings in \({\mathcal {A}}_i\), \(|{\mathcal {A}}_i\cap {\mathcal {Q}}|\) is even.
Note this definition is only for \(j=1\) and not for \(2\le j\le r\), which we will define later.
The support condition (6.13) for type \(R0^+\) can be immediately verified, since \({\mathcal {U}}_j^{\dagger \dagger } = \varnothing \).
We may also need to multiply this h by functions \({\mathbf {1}}_{\langle k\rangle \ge M^2}\) or \({\mathbf {1}}_{\langle k_1\rangle \ge M^2}\), but they do not affect Proposition 4.15 (which can be easily checked), so the proof below will proceed in the same way.
See Definition 3.1. Here simplicity implies that, if \(\zeta =+\) and \(k=k'\), then k must also equal some other \(k_j\), which has already been fixed.
Here we have assumed \(1\le j\le r\). If \(r+1\le j\le q\), then this j would correspond to the input function \(z_{N_j}\) in (5.22), which gains a big power \(N_j^{-D_1}\). Thus the case where the maximum \(N_j\) occurs at this j will be strictly easier than the cases we actually treat in the proof.
When \({\mathcal {S}}\) is fixed, the sum in \((N_j,{\mathcal {S}}_j)\) etc. for \(j\ge 2\) involves at most \((\log L)^{\kappa }\) terms, which is negligible in view of the \(L^{\varepsilon \delta }\) gain we will obtain below. The sum in \((N_1,{\mathcal {S}}_1)\) involves at most \(\kappa \) terms if \(N_1\sim M\) and \({\mathcal {S}}_1={\mathcal {S}}'\), and at most \((\log M)^{\kappa }\) terms otherwise; either way this is negligible in view of the gain from \(\Upsilon \), and the gain of at least \(M^{\delta ^5}\) coming from trimming assuming \({\mathcal {S}}_1\ne {\mathcal {S}}'\), which is evident from the proof of Proposition 6.1.
If needed, we can always view an \({\mathbb {R}}\)-multilinear operator of degree \(q-1\) as one of degree q by adding a trivial input function.
Because we do not need to distinguish the low-frequency inputs as there is only one scale.
The choice of \(N^{1+\theta }\) is because \(\phi \) is not compactly supported and may have a Schwartz tail.
The reader may notice the similarity with the construction of \(z_M\) in (5.28).
Even with the \({\mathcal {N}}_{\mathrm {np}}\) nonlinearity, some renormalization may still be needed for higher order iterations, but not for the first nonlinear iteration which is discussed here. Also whether u is real or complex valued, and whether \({\mathcal {N}}_{\mathrm {np}}\) contains complex conjugates, does not affect the scaling heuristics.
This distinction may be artificial from the regularity structure perspective, but is convenient in comparison with the dispersive case here.
For example, invariance of Gibbs measure and the associated almost-sure global well-posedness result would imply that there is no stable blowup mechanism at the regularity of the support of the Gibbs measure.
References
Aizenman, M.: Geometric analysis of \(\Phi ^4\) fields and Ising models. Part I and II. Commun. Math. Phys. 86(1), 1–48 (1982)
Aizenman, M., Copin, H.: Marginal triviality of the scaling limits of critical 4D Ising and \(\Phi _4^4\) models. Ann. Math. (2) 194(1), 163–235 (2021)
Alazard, T., Burq, N., Zuily, C.: Strichartz estimates and the Cauchy problem for the gravity water waves equations. Memoirs of the AMS, vol. 256, no. 1229 (2014)
Albeverio, S., Kusuoka, S.: The invariant measure and the flow associated to the \(\Phi ^ 4_3\)-quantum field model. Ann. Sc. Norm. Super. Pisa Cl. Sci. (5) XX, 1359–1427 (2020)
Bailleul, I., Bernicot, F.: Heat semigroup and singular PDEs. J. Funct. Anal. 270(9), 3344–3452 (2016)
Bailleul, I., Bernicot, F.: High order paracontrolled calculus. Forum Math. Sigma 7(e44), 1–94 (2019)
Barashkov, N., Gubinelli, M.: A variational method for \(\Phi _3^4\). Duke Math. J. 169(17), 3339–3415 (2020)
Barashkov, N., Gubinelli, M.: The \(\Phi _3^4\) measure via Girsanov’s theorem. Electron. J. Probab. 26, 1–29 (2021)
Bényi, A., Oh, T., Pocovnicu, O.: Higher order expansions for the probabilistic local Cauchy theory of the cubic nonlinear Schrödinger equation on \({\mathbb{R}}^3\). Trans. Am. Math. Soc. 6, 114–160 (2019)
Bourgain, J.: Periodic nonlinear Schrödinger equation and invariant measures. Commun. Math. Phys. 166, 1–26 (1994)
Bourgain, J.: Invariant measures for the 2D-defocusing nonlinear Schrödinger equation. Commun. Math. Phys. 176, 421–445 (1996)
Bourgain, J.: Invariant measures for the Gross–Pitaevskii equation. J. Math. Pures Appl. 76, 649–702 (1997)
Bourgain, J.: Refinements of Strichartz inequality and applications to 2D-NLS with critical nonlinearity. Int. Math. Res. Notices 5, 253–283 (1998)
Bringmann, B.: Almost sure local well-posedness for a derivative nonlinear wave equation. Int. Math. Res. Notices 11, 8657–8697 (2021)
Bruned, Y., Chandra, A., Chevyrev, I., Hairer, M.: Renormalising SPDEs in regularity structures. J. Eur. Math. Soc. 23(3), 869–947 (2020)
Bruned, Y., Hairer, M., Zambotti, L.: Algebraic renormalisation of regularity structures. Invent. Math. 215(3), 1039–1156 (2019)
Buckmaster, T., Germain, P., Hani, Z., Shatah, J.: Effective dynamics of the nonlinear Schrödinger equation on large domains. Commun. Pure Appl. Math. 71(7), 1407–1460 (2018)
Buckmaster, T., Germain, P., Hani, Z., Shatah, J.: Onset of the wave turbulence description of the longtime behavior of the nonlinear Schrödinger equation. Invent. Math. 225, 787–855 (2021)
Burq, N., Tzvetkov, N.: Random data Cauchy theory for supercritical wave equations I: local theory. Invent. Math. 173(3), 449–475 (2008)
Catellier, R., Chouk, K.: Paracontrolled distributions and the 3-dimensional stochastic quantization equation. Ann. Prob. 46(5), 2621–2679 (2018)
Chandra, A., Hairer, M.: An analytic BPHZ theorem for regularity structures. arXiv:1612.08138
Chandra, A., Moinat, A., Weber, H.: A priori bounds for the \(\Phi ^4\) equation in the full sub-critical regime. arXiv:1910.13854
Chandra, A., Weber, H.: Stochastic PDEs, regularity structures, and interacting particle systems. Ann. Fac. Sci. Toulouse Math. (6) 26(4), 847–909 (2017)
Cheung, K., Li, G., Oh, T.: Almost conservation laws for stochastic nonlinear Schrödinger equations. J. Evol. Equ. 21, 1865–1894 (2021)
Cheung, K., Mosincat, R.: Stochastic nonlinear Schrödinger equations on tori. Stoch. Partial Differ. Equ. Anal. Comput. 7(2), 169–208 (2019)
Colliander, J., Keel, M., Staffilani, G., Takaoka, H., Tao, T.: Almost conservation laws and global rough solutions to a nonlinear Schrödinger equation. Math. Res. Lett. 9(5), 659–682 (2002)
Colliander, J., Oh, T.: Almost sure well-posedness of the cubic nonlinear Schrödinger equation below \(L^2({\mathbb{T}})\). Duke Math. J. 161(3), 367–414 (2012)
Collot, C., Germain, P.: On the derivation of the homogeneous kinetic wave equation. arXiv:1912.10368
Da Prato, G., Debussche, A.: Two-dimensional Navier-Stokes equations driven by a space-time white noise. J. Funct. Anal. 196(1), 180–210 (2002)
Da Prato, G., Debussche, A.: Strong solutions to the stochastic quantization equations. Ann. Probab. 31(4), 1900–1916 (2003)
de Bouard, A., Debussche, A.: The stochastic nonlinear Schrödinger equation in \(H^1\). Stoch. Anal. Appl. 21(1), 97–126 (2003)
Deng, Y.: Two dimensional nonlinear Schrödinger equation with random radial data. Anal. PDE 5(5), 913–960 (2012)
Deng, Y., Hani, Z.: On the derivation of the wave kinetic equation for NLS. Forum Math. Pi 9(E6), 1–37 (2021)
Deng, Y., Hani, Z.: Full derivation of the wave kinetic equation. arXiv:2104.11204
Deng, Y., Nahmod, A.R., Yue, H.: Optimal local well-posedness for the periodic derivative nonlinear Schrödinger equation. Commun. Math. Phys. 384, 1061–1107 (2021)
Deng, Y., Nahmod, A., Yue, H.: Invariant Gibbs measures and global strong solutions for nonlinear Schrödinger equations in dimension two. arXiv:1910.08492
Fan, C., Xu, W.: Global well-posedness for the defocusing mass-critical stochastic nonlinear Schrödinger equation on \({\mathbb{R}}\) at \(L^ 2\) regularity. arXiv:1810.07925
Faou, E., Germain, P., Hani, Z.: The weakly nonlinear large-box limit of the 2D cubic nonlinear Schröinger equation. J. Am. Math. Soc. 29(4), 915–982 (2016)
Forlano, J., Oh, T., Wang, Y.: Stochastic nonlinear Schrödinger equation with almost space-time white noise. J. Aust. Math. Soc. 109, 44–67 (2018)
Friz, P., Hairer, M.: A course on rough paths. In: With an Introduction to Regularity Structures (2nd edn). Universitext. Springer, Cham (2020)
Fröhlich, J.: On the triviality of \(\lambda \Phi _d^4\) theories and the approach to the critical point in \(d_{(-)}>4\) dimensions. Nucl. Phys. B 200(2), 281–296 (1982)
Furlan, M., Gubinelli, M.: Paracontrolled quasilinear SPDEs. Ann. Probab. 47(2), 1096–1135 (2019)
Gerencsér, M., Hairer, M.: A solution theory for quasilinear singular SPDEs. Commun. Pure Appl. Math. 72(9), 1983–2005 (2019)
Glimm, J., Jaffe, A.: Quantum physics. In: A Functional Integral Point of View (2nd edn). Springer, New York (1987)
Grünrock, A., Herr, S.: Low regularity local well-posedness of the derivative nonlinear Schrödinger equation with periodic initial data. SIAM J. Math. Anal. 39(6), 1890–1920 (2008)
Gubinelli, M., Imkeller, P., Perkowski, N.: Paracontrolled distributions and singular PDEs. In: Forum of Mathematics, Pi 3, e6 (2015)
Gubinelli, M., Koch, H., Oh, T.: Paracontrolled approach to the three-dimensional stochastic nonlinear wave equation with quadratic nonlinearity. arXiv:1811.07808
Gubinelli, M., Perkowski, N.: Lectures on singular stochastic PDEs. Ensaios Matemáticos, Mathematical Surveys, 29. Sociedade Brasileira de Matemática, Rio de Janeiro (2015)
Gubinelli, M., Perkowski, N.: KPZ reloaded. Commun. Math. Phys. 349(1), 165–269 (2017)
Gubinelli, M., Perkowski, N.: An introduction to singular SPDEs. In: Stochastic Partial Differential Equations and Related Fields, pp. 69–99. Springer Proceedings in Mathematics and Statistics, vol. 229. Springer, Cham (2018)
Guo, Z., Oh, T.: Non-existence of solutions for the periodic cubic NLS below \(L^2\). Int. Math. Res. Notices 6, 1656–1729 (2018)
Hairer, M.: A theory of regularity structures. Invent. Math. 198(2), 269–504 (2014)
Hairer, M.: Singular stochastic PDE. Proc. ICM-Seoul I, 685–709 (2014)
Hairer, M.: Introduction to regularity structures. Braz. J. Probab. Stat. 29(2), 175–210 (2015)
Hairer, M.: Regularity structures and the dynamical \(\Phi ^4_3\) model. In: Current Developments in Mathematics 2014, pp. 1–49. International Press Institute, Somerville, MA (2016)
Hairer, M., Ryser, M.D., Weber, H.: Triviality of the 2D stochastic Allen–Cahn equation. Electron. J. Probab. 17(39), 1–14 (2012)
Klainerman, S., Rodnianski, I., Szeftel, J.: The bounded \(L^2\) curvature conjecture. Invent. Math. 202(1), 91–216 (2015)
Kupiainen, A.: Renormalization group and stochastic PDEs. Ann. Henri Poincaré 17(3), 497–535 (2016)
Lebowitz, J., Rose, R., Speer, E.: Statistical mechanics of the nonlinear Schrödinger equation. J. Stat. Phys. 50, 657–687 (1988)
Merle, F., Raphael, P., Rodnianski, I., Szeftel, J.: On blow up for the energy super critical defocusing non linear Schrödinger equations. Invent. Math. (2021). https://doi.org/10.1007/s00222-021-01067-9
Moinat, A., Weber, H.: Space-time localisation for the dynamic \(\Phi _3^4\) model. Commun. Pure Appl. Math. 73(12), 2519–2555 (2020)
Mourrat, J.C., Weber, H.: The dynamic \(\Phi ^4_3\) model comes down from infinity. Commun. Math. Phys. 356(3), 673–753 (2017)
Nelson, E.: Construction of quantum fields from Markoff fields. J. Funct. Anal. 12, 97–112 (1973)
Oh, T., Tzvetkov, N., Wang, Y.: Solving the 4NLS with white noise initial data. Forum Math. Sigma 8, E48 (2020)
Parisi, G., Wu, Y.S.: Perturbation theory without gauge fixing. Sci. Sinica. Zhongguo Kexue 24(4), 483–496 (1981)
Simon, B.: The \(P(\varphi )_2\) Euclidean (quantum) Field Theory. Princeton Series in Physics. Princeton University Press, Princeton, NJ (1974)
Sun, C., Tzvetkov, N.: Gibbs measure dynamics for the fractional NLS. SIAM J. Math. Anal. 52(5), 4638–4704 (2020)
Acknowledgements
The authors would like to thank Hendrik Weber for helpful comments regarding the regularity structures theory and the reference [22]. They would also like to thank the referees for their useful comments and suggestions.
Author information
Authors and Affiliations
Corresponding author
Additional information
Y.D. is funded in part by NSF DMS-1900251 and Sloan Fellowship.
A.N. is funded in part by NSF DMS-1800852, DMS-2101381 and the Simons Foundation Collaboration Grant on Wave Turbulence (Nahmod’s Award ID 651469).
Rights and permissions
About this article
Cite this article
Deng, Y., Nahmod, A.R. & Yue, H. Random tensors, propagation of randomness, and nonlinear dispersive equations. Invent. math. 228, 539–686 (2022). https://doi.org/10.1007/s00222-021-01084-8
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00222-021-01084-8





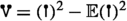 and
and 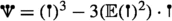 .
.