Summary.
The simplest and the best-known method for numerical approximation of high-dimensional integrals is the Monte Carlo method (MC), i.e. random sampling. MC has also become the most popular method for constructing numerically solvable approximations of stochastic programs. However, certain modern integration quadratures are often superior to crude MC in high-dimensional integration, so it seems natural to try to use them also in discretization of stochastic programs. This paper derives conditions that guarantee the epi-convergence of the resulting objectives to the original one. Our epi-convergence result is closely related to some of the existing ones but it is easier to apply to discretizations and it allows the feasible set to depend on the probability measure. As examples, we prove epi-convergence of quadrature-based discretizations of three different models of portfolio management and we study their behavior numerically. Besides MC, our discretizations are the only existing ones with guaranteed epi-convergence for these problem classes. In our tests, modern quadratures seem to result in faster convergence of optimal values than MC.
Similar content being viewed by others
References
Artstein, Z., Wets, R.J.-B.: Stability results for stochastic programs and sensors, allowing for discontinuous objective functions. SIAM J. Optim. 4(3), 537–550 (1994)
Artstein, Z., Wets, R.J.-B.: Consistency of minimizers and the SLLN for stochastic programs. J. Convex Anal. 2(1–2), 1–17 (1995)
Attouch, H.: Variational convergence for functions and operators. Pitman (Advanced Publishing Program), Boston, MA, 1984
Attouch, H., Wets, R.J.-B.: Quantitative stability of variational systems. I. The epigraphical distance. Trans. Amer. Math. Soc. 328(2), 695–729 (1991)
Beer, G.: A geometric algorithm for approximating semicontinuous function. J. Approx. Theory 49(1), 31–40 (1987)
Bertsekas, D.P., Shreve, S.E.: Stochastic optimal control, volume 139 of Mathematics in Science and Engineering. Academic Press Inc. [Harcourt Brace Jovanovich Publishers], New York, 1978. The discrete time case
Billingsley, P.: Convergence of probability measures. John Wiley & Sons Inc., New York, 1968
Billingsley, P.: Convergence of probability measures. John Wiley & Sons Inc., New York, second edition, 1999. A Wiley-Interscience Publication
Birge, J.R., Wets, R.J.-B.: Designing approximation schemes for stochastic optimization problems, in particular for stochastic programs with recourse. Math. Programming Stud., Stochastic programming 84. I. (27), 54–102 (1986)
Bratley, P., Fox, B.L., Niederreiter, H.: Implementation and tests of low-discrepancy sequences. ACM Trans. Model. Comput. Simul. 2(3), 195–213 (1992)
Chen, X., Womersley, R.S.: A parallel inexact Newton method for stochastic programs with recourse. Ann. Oper. Res. 64, 113–141 (1996); Stochastic programming, algorithms and models (Lillehammer, 1994)
Deák, I.: Multidimensional integration and stochastic programming. In Numerical techniques for stochastic optimization, volume 10 of Springer Ser. Comput. Math. Springer, Berlin, 1988, pp. 187–200
Dempster, M.A.H., Thompson, R.T.: EVPI-based importance sampling solution procedures for multistage stochastic linear programmes on parallel MIMD architectures. Ann. Oper. Res. 90, 161–184 (1999)
Dupačová, J., Wets, R.J.-B.: Asymptotic behavior of statistical estimators and of optimal solutions of stochastic optimization problems. Ann. Statist. 16(4), 1517–1549 (1988)
Faure, H.: Discrépance de suites associées à un système de numération (en dimension s). Acta Arith. 41(4), 337–351 (1982)
Fox, B.L.: Algorithm 647: Implementation and relative efficiency of quasirandom sequence generators. ACM Transactions on Mathematical Software 12(4), 362–376 (1986)
Frauendorfer, K.: Stochastic two-stage programming, volume 392 of Lecture Notes in Economics and Mathematical Systems. Springer-Verlag, Berlin, 1992 Habilitationsschrift, University of Zürich, Zürich, 1992
Halton, J.H.: On the efficiency of certain quasi-random sequences of points in evaluating multi-dimensional integrals. Numer. Math. 2, 84–90 (1960)
Hammersley, J.M.: Monte Carlo methods for solving multivariable problems. Ann. New York Acad. Sci. 86, 844–874 (1960) 1960
Høyland, K., Kaut, M., Wallace, S.W.: Heuristic for moment-matching scenario generation. Comput. Optim. Appl. 24(2–3), 169–185 (2003)
Høyland, K., Wallace, S.W.: Generating scenario trees for multistage decision problems. Managament Science 47(2), 295–307 (2001)
Infanger, G.: Monte Carlo (importance) sampling within a Benders decomposition algorithm for stochastic linear programs. Ann. Oper. Res. 39(1–4), 69–95 (1993, 1992)
Kall, P., Ruszczyński, A., Frauendorfer, K.: Approximation techniques in stochastic programming. In Numerical techniques for stochastic optimization, volume 10 of Springer Ser. Comput. Math. Springer, Berlin, 1988, pp. 33–64
King, A.J.: Duality and martingales: a stochastic programming perspective on contingent claims. Math. Program. 91(3, Ser. B), 543–562 (2002); ISMP 2000, Part 1 (Atlanta, GA)
Korf, L.A.: Stochastic programming duality:
 multipliers for unbounded constraints with an application to mathematical finance. Math. Program. 99(2, Ser. A), 241–259 (2004)
multipliers for unbounded constraints with an application to mathematical finance. Math. Program. 99(2, Ser. A), 241–259 (2004)Korobov, N.M.: Approximate evaluation of repeated integrals. Dokl. Akad. Nauk SSSR 124, 1207–1210 (1959)
L’Ecuyer, P., Lemieux, C.: Variance reduction via lattice rules. Management Science 46(2), 1214–1235 (2000)
Lepp, R.: Approximations to stochastic programs with complete recourse. SIAM J. Control Optim. 28(2), 382–394 (1990)
Lucchetti, R., Salinetti, G., Wets, R.J.-B.: Uniform convergence of probability measures: topological criteria. J. Multivariate Anal. 51(2), 252–264 (1994)
Lucchetti, R., Wets, R.J.-B.: Convergence of minima of integral functionals, with applications to optimal control and stochastic optimization. Statist. Decisions 11(1), 69–84 (1993)
Niederreiter, H.: Random number generation and quasi-Monte Carlo methods, volume 63 of CBMS-NSF Regional Conference Series in Applied Mathematics. Society for Industrial and Applied Mathematics (SIAM), Philadelphia, PA, 1992
Pennanen, T., Koivu, M.: Integration quadratures in discretization of stochastic programs. Stochastic Programming E-Print Series, 2002
Pflug, G.: Scenario tree generation for multiperiod financial optimization by optimal discretization. Math. Program., 89(2, Ser. B), 251–271 (2001); Mathematical programming and finance
Pflug, G., Hochreiter, R.: Scenario generation for stochastic multi-stage decision processes as facility location problems. Technical report, Department of Statistics and Decision Support Systems, University of Vienna, 2003
Press, W.H., Teukolsky, S.A., Vetterling, W.T., Flannery, B.P.: Numerical recipes in C. Cambridge University Press, Cambridge, second edition, 1992 The art of scientific computing
Svetlozar T.: Rachev and Werner Römisch. Quantitative stability in stochastic programming: the method of probability metrics. Math. Oper. Res. 27(4), 792–818 (2002)
Robinson, S.M., Wets, R.J.-B.: Stability in two-stage stochastic programming. SIAM J. Control Optim. 25(6), 1409–1416 (1987)
Rockafellar, R.T., Wets, R.J.-B.: Variational analysis, volume 317 of Grundlehren der Mathematischen Wissenschaften [Fundamental Principles of Mathematical Sciences]. Springer-Verlag, Berlin, 1998
Römisch, W., Schultz, R.: Stability of solutions for stochastic programs with complete recourse. Math. Oper. Res. 18(3), 590–609 (1993)
Schultz, R.: Some aspects of stability in stochastic programming. Ann. Oper Res. 100, 55–84 (2001), 2000. Research in stochastic programming (Vancouver, BC, 1998)
Shapiro, A.: Stochastic programming by monte carlo simulation methods. Stochastic Programming E-Print Series, 2000
Shapiro, A.: Quantitative stability in stochastic programming. Math. Programming 67(1, Ser. A), 99–108 (1994)
Sloan, I.H., Joe, S.: Lattice methods for multiple integration. Oxford Science Publications. The Clarendon Press Oxford University Press, New York, 1994
Sobol’, I.M.: The distribution of points in a cube and the approximate evaluation of integrals. U.S.S.R. Comput. Math. And Math. Phys. (4), 86–112 (1967)
Vogel, S., Lachout, P.: On continuous convergence and epi-convergence of random functions. II. Sufficient conditions and applications. Kybernetika (Prague) 39(1), 99–118 (2003)
Zervos, M.: On the epiconvergence of stochastic optimization problems. Math. Oper. Res. 24(2), 495–508 (1999)
Author information
Authors and Affiliations
Corresponding author
Additional information
Mathematics Subject Classification (2000): 90C15, 49M25
The work of this author was partially supported by The Finnish Foundation for Economic Education under grant no. 21599 and by Finnish Academy under contract no. 3385
Rights and permissions
About this article
Cite this article
Pennanen, T., Koivu, M. Epi-convergent discretizations of stochastic programs via integration quadratures. Numer. Math. 100, 141–163 (2005). https://doi.org/10.1007/s00211-004-0571-4
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00211-004-0571-4



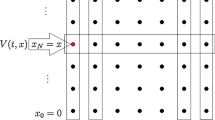
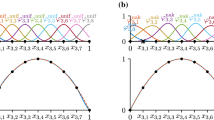
 multipliers for unbounded constraints with an application to mathematical finance. Math. Program. 99(2, Ser. A), 241–259 (2004)
multipliers for unbounded constraints with an application to mathematical finance. Math. Program. 99(2, Ser. A), 241–259 (2004)