The Insulated Conductivity Problem with p-Laplacian

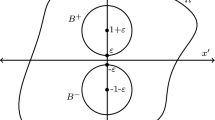
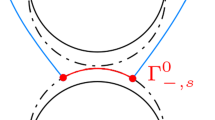
Abstract

We study the insulated conductivity problem with closely spaced insulators embedded in a homogeneous matrix where the current-electric field relation is the power law $J = |E|^{p-2}E$. The gradient of solutions may blow up as $\varepsilon$, the distance between insulators, approaches to 0. We prove an upper bound of the gradient to be of order $\varepsilon ^{-\alpha }$, where $\alpha = 1/2$ when $p \in (1,n+1]$ and any $\alpha > n/(2(p-1))$ when $p > n + 1$. We provide examples to show that this exponent is almost optimal in 2D. Additionally, in dimensions $n \geqq 3$, for any $p > 1$, we prove another upper bound of order $\varepsilon ^{-1/2 + \beta }$ for some $\beta > 0$, and show that $\beta \nearrow 1/2$ as $n \rightarrow \infty$.

Appendix A.

In the appendix, we provide an alternative proof of the gradient estimates of order $\varepsilon ^{-1/2}$ using a Bernstein type argument. This proof also requires the assumptions that $h_1$ and $h_2$ are $C^{2,\text {Dini}}$ functions and satisfy (1.10) for some $\kappa _1,\kappa _2 > 0$, in addition to (1.3) imposed in Theorem 1.1.

Theorem A.1

Let $h_1$, $h_2$ be $C^{2,\text {Dini}}$ functions satisfying (1.10), $p > 1$, $n \geqq 2$, $\varepsilon \in (0,1)$, and $u \in W^{1,p}(\Omega _{1})$ be a solution of (1.7). Then there exists a positive constant C depending only on n, p, $\kappa _1$, and $\kappa _2$, such that

$$\begin{aligned} |D u(x)| \leqq C \Vert u\Vert _{L^\infty (\Omega _{1})} (\varepsilon + |x'|^2)^{-1/2} \quad \text{ for }~~x \in \Omega _{1/2}. \end{aligned}$$

(A.1)

Proof

Without loss of generality, we may assume $\kappa _1\in (0,1]$ and $\kappa _2>1$. The case $p=2$ has been shown in [6, 34]. It remains to show the cases when $p > 2$ and $p \in (1, 2)$.

Case 1: For $p > 2$, we consider the quantity $F^{q/2}$, where

$$\begin{aligned} F = Q|D u|^2 + A u^2, \quad Q = \varepsilon + |x'|^2 - 4\kappa _1^{-1} \kappa _2^2 x_n^2, \end{aligned}$$

(A.2)

$q \geqq 2$ and A are some positive $\varepsilon$-independent constants to be determined later. Let

$$\begin{aligned} S_A: = \{ Q|D u|^2 > 100 Au^2 \}. \end{aligned}$$

We will show that $F^{q/2}$ does not achieve its maximum on $(\Gamma _+ \cup \Gamma _-) \cap {\overline{\Omega }}_{r_0}\cap S_A$ or in $\Omega _{r_0} \cap S_A$ for some suitable q, A, and $r_0$. Therefore, $F^{q/2}$ can only achieve its maximum in

$$\begin{aligned} \Omega _{r_0} \cap \{ Q|D u|^2 \leqq 100 Au^2 \}, \end{aligned}$$

or on

$$\begin{aligned} \{|x'| = r_0\} \cap \Omega _{1}, \end{aligned}$$

so (A.1) follows from either case.

First we show that $F^{q/2}$ does not achieve its maximum on $\Gamma _+ \cap {\overline{\Omega }}_{r_0}\cap S_A$. A similar argument applies to $\Gamma _- \cap {\overline{\Omega }}_{r_0}\cap S_A$. On $\Gamma _+$, the normal vector $\nu$ is given by (6.2). Then

$$\begin{aligned}&D_\nu F^{q/2} = \frac{q}{2} F^{q/2-1} (D_\nu Q |D u|^2 + Q D_\nu |D u|^2)\\&\quad = \frac{q}{2} F^{q/2-1} \Bigg ( \frac{-2}{\sqrt{1 + |D_{x'} h_1|^2}} \Big [ \sum _{j = 1}^{n-1} D_j h_1 x_j + 4\frac{\kappa _2^2}{\kappa _1} (\varepsilon /2 + h_1) \Big ]|D u|^2 + Q D_\nu |D u|^2) \Bigg ). \end{aligned}$$

We choose $r_0$ small enough such that

$$\begin{aligned} \frac{-2}{\sqrt{1 + |D_{x'} h_1|^2}} \leqq \frac{-2}{\sqrt{1 + |\kappa _2 x'|^2}} \leqq -1 \quad \text{ for }~~|x'|< r_0. \end{aligned}$$

By (1.3) and (1.10), we have

$$\begin{aligned} \sum _{j = 1}^{n-1} D_j h_1 x_j \geqq \kappa _1 |x'|^2 \quad \text{ and } \quad h_1 \geqq \frac{1}{2}\kappa _1|x'|^2. \end{aligned}$$

Therefore, by (6.1) with $s=2$, we have

$$\begin{aligned}&D_\nu F^{q/2} \\&\quad \leqq \frac{q}{2} F^{q/2-1} |D u|^2 \Bigg [ -\Bigg (\kappa _1|x'|^2 + 2 \frac{\kappa _2^2}{\kappa _1}\varepsilon + 2\kappa _2^2|x'|^2\Bigg ) + 2\kappa _2\Bigg (\varepsilon + |x'|^2 - 4 \frac{\kappa _2^2}{\kappa _1} x_n^2\Bigg )\Bigg ]\\&\quad = - \frac{q}{2} F^{q/2-1} |D u|^2 \Bigg [ 2\kappa _2 \Bigg (\frac{\kappa _2}{\kappa _1}-1\Bigg ) \varepsilon + 2\kappa _2(\kappa _2-1)|x'|^2 + \kappa _1|x'|^2 + 8 \frac{\kappa _2^2}{\kappa _1} x_n^2 \Bigg ]\\&\quad < 0. \end{aligned}$$

Hence $F^{q/2}$ does not achieve its maximum on $\Gamma _+ \cap {\overline{\Omega }}_{r_0}\cap S_A$. Next, we will show $F^{q/2}$ does not achieve its maximum in $\Omega _{r_0} \cap S_A$ by proving that $a^{ij} D_{ij} F^{q/2} > 0$, where $a^{ij}$ is given in (6.5). By direct computations, we have

$$\begin{aligned} a^{ij} D_{ij} F^{q/2}&= \frac{q}{2} F^{q/2 - 1} a^{ij} D_{ij} F + \frac{q}{2}\Big ( \frac{q}{2}-1 \Big ) F^{q/2-2} a^{ij} D_i F D_j F,\\ D_i F&= D_i Q |D u|^2 + 2Q D_{ik}u D_k u + 2Au D_i u, \end{aligned}$$

and

$$\begin{aligned} D_{ij} F =&D_{ij}Q |D u|^2 + 2 D_i Q D_k u D_{jk} u +2 D_j Q D_k u D_{ik} u \\&+ 2Q(D_{ik}u D_{jk} u + D_k u D_{ijk} u) + 2A(u D_{ij}u + D_i u D_j u). \end{aligned}$$

Then by (6.5) and because $a^{ij}D_{ij} u = 0$,

$$\begin{aligned}&a^{ij} D_{ij} F \\&\quad = a^{ij} D_{ij} Q |D u|^2 + 4(p-2)|D u|^{-2} D_i u D_i Q \Delta _\infty u + 4 D_i Q D_k u D_{ik} u + 2Q |D^2 u|^2\\&\qquad + 2(p-2)Q |D |D u||^2 + 2Q a^{ij} D_{ijk} u D_k u + 2A a^{ij} D_i u D_j u, \end{aligned}$$

where $\Delta _\infty u:= D_i u D_j u D_{ij} u$. By (6.9),

$$\begin{aligned}&a^{ij} D_{ij} F \\&\quad = a^{ij} D_{ij} Q |D u|^2 + 4(p-2)|D u|^{-2} D_i u D_i Q \Delta _\infty u + 4 D_i Q D_k u D_{ik} u + 2Q |D^2 u|^2\\&\qquad - 2(p-2)Q |D |D u||^2 + 4 (p-2) Q|D u|^{-4} |\Delta _\infty u|^2 + 2A a^{ij} D_i u D_j u. \end{aligned}$$

By another direction computation, we have

$$\begin{aligned}&a^{ij} D_i F D_j F \\&\quad = a^{ij} D_i Q D_j Q |D u|^4 + 4Q^2 a^{ij} D_{ik}u D_ku D_{jl}u D_l u + 4A^2 u^2 a^{ij} D_iu D_ju\\&\qquad + 4Q|D u|^2 a^{ij} D_i Q D_{jl} u D_lu + 4Au |D u|^2a^{ij} D_i Q D_j u + 8AQ u a^{ij} D_{ik} u D_k u D_ju\\&\quad = a^{ij} D_i Q D_j Q |D u|^4 + 4(p-2)Q^2 |D u|^{-2} |\Delta _\infty u|^2 + 4Q^2 |D u|^2 |D |D u||^2\\&\qquad + 4(p-1)A^2 u^2|D u|^2 + 4(p-2) Q D_i u D_i Q \Delta _\infty u +4Q D_i Q D_{ik}u D_k u |D u|^2\\&\qquad + 4 A(p-1) |D u|^2 u D_i u D_i Q + 8(p-1)AQu \Delta _\infty u. \end{aligned}$$

Therefore,

$$\begin{aligned}&a^{ij} D_{ij} F^{q/2}\nonumber \\&\quad = \frac{q}{2} F^{q/2 -2} \big [ a^{ij} D_{ij} Q |D u|^2 F +2Q|D |D u||^2 \big ( (q-2)Q|D u|^2 - (p-2)F \big ) \nonumber \\&\qquad + 4(p-2) D_i u D_i Q \Delta _\infty u |D u|^{-2} (F + (q-2)Q|D u|^2/2) + 2QF|D^2 u|^2\nonumber \\&\qquad + 4 D_i Q D_k u D_{ik}u (F + (q-2)Q|D u|^2/2)\nonumber \\&\qquad + 4(p-2)Q|D u|^{-4} (F + (q-2)Q|D u|^2/2) |\Delta _\infty u|^2 \nonumber \\&\qquad + 2AFa^{ij} D_i u D_j u + (q-2)a^{ij}|Du|^4 D_i Q D_j Q/2 + 2(p-1)(q-2) A^2 u^2 |D u|^2 \nonumber \\&\qquad + 2A(p-1)(q-2)|D u|^2 u D_i u D_i Q + 4(p-1)(q-2)AQu \Delta _\infty u \big ]. \end{aligned}$$

(A.3)

Note that in $S_A$,

$$\begin{aligned} Q|D u|^2 \leqq F \leqq \frac{101}{100} Q |D u|^2. \end{aligned}$$

(A.4)

By (6.6),

$$\begin{aligned} (q-2)a^{ij}D_i Q D_j Q/2 \geqq (q-2)|D Q|^2 /2 \geqq 0 \quad \text{ for }~~q \geqq 2, \end{aligned}$$

and

$$\begin{aligned}&a^{ij} D_{ij} Q |D u|^2 F + 2AFa^{ij} D_i u D_j u\nonumber \\&\quad = [2(n-1) - 8\kappa _1^{-1}\kappa _2^2 + (p-2)|D u|^{-2} (2|D_{x'} u|^2 - 8\kappa _1^{-1}\kappa _2^2 |D_n u|^2)] |D u|^2 F\nonumber \\&\qquad + 2AFa^{ij} D_i u D_j u\nonumber \\&\quad \geqq \Big ( 2(n-1) - 8\kappa _1^{-1}\kappa _2^2(p-1) + 2A \Big ) |D u|^2 F\nonumber \\&\quad \geqq \Big ( 2(n-1) - 8\kappa _1^{-1}\kappa _2^2(p-1) + 2A \Big ) |D u|^4 Q. \end{aligned}$$

(A.5)

We choose $q = \frac{101}{100}(p-2) + 2 > p$, so that

$$\begin{aligned}&2Q|D |D u||^2 \big ( (q-2)Q|D u|^2 - (p-2)F \big )\\&\quad \geqq 2Q|D |D u||^2 \bigg ( (q-2) - \frac{101}{100}(p-2) \bigg )Q|D u|^2 = 0. \end{aligned}$$

It remains to control

$$\begin{aligned}&4(p-2) D_i u D_i Q \Delta _\infty u |D u|^{-2} (F + (q-2)Q|D u|^2/2)\\&\qquad +4 D_i Q D_k u D_{ik}u (F + (q-2)Q|D u|^2/2)\\&\qquad + 2A(p-1)(q-2)|D u|^2 u D_i u D_i Q + 4(p-1)(q-2)AQu \Delta _\infty u\\&\quad =: I + II + III + IV. \end{aligned}$$

Since $p > 2$, we have

$$\begin{aligned} \frac{2(p-2)q}{(p-1)(q-2)} = \frac{2 \Big [ \frac{101}{100}(p-2) + 2\Big ]}{\frac{101}{100}(p-1)} > 2. \end{aligned}$$

Fix a constant $B \in (2, \frac{2(p-2)q}{(p-1)(q-2)})$. We shrink $r_0$ if necessary so that $|D Q|^2 \leqq 8 Q$. By Young’s inequality and (A.4),

$$\begin{aligned} |I| \leqq&\left( \frac{p-2}{2} - \frac{B(p-1)(q-2)}{4q} \right) |D u|^{-4} |D Q|^2 |\Delta _\infty u|^2 (F + (q-2)Q|D u|^2/2)\nonumber \\&+ C(p) |D u|^2 (F + (q-2)Q|D u|^2/2) \nonumber \\ \leqq&\left( 4(p-2) - \frac{2B(p-1)(q-2)}{q} \right) Q |D u|^{-4} |\Delta _\infty u|^2 (F + (q-2)Q|D u|^2/2)\nonumber \\&+ C(p) |D u|^4Q, \end{aligned}$$

(A.6)

where C(p) is some positive constant depending on p. By Young’s inequality and (A.4),

$$\begin{aligned} |II|&\leqq 4 \Big (\frac{101}{100} + \frac{q-2}{2}\Big ) |D Q| |D^2 u| |D u|^3 Q\nonumber \\&\leqq 4 \Big (\frac{101}{100} + \frac{q-2}{2}\Big )^2 |D Q|^2 |D u|^4 + Q^2 |D^2 u|^2 |D u|^2 \nonumber \\&\leqq 32 \Big (\frac{101}{100} + \frac{q-2}{2}\Big )^2 |D u|^4 Q+ QF |D^2 u|^2, \end{aligned}$$

(A.7)

$$\begin{aligned} |III|&\leqq 2(p-1)(q-2) A |D u|^3|u| |D Q|\nonumber \\&\leqq \left( 2 - \frac{4}{B} \right) (p-1)(q-2) A^2 u^2 |D u|^2 + \frac{4B}{B-2}(p-1)(q-2) |D u|^4 Q, \end{aligned}$$

(A.8)

and

$$\begin{aligned} |IV| \leqq&\frac{4}{B}(p-1)(q-2) A^2 u^2 |D u|^2 + B(p-1)(q-2)Q^2 |D u|^{-2} |\Delta _\infty u|^2 \nonumber \\ \leqq&\frac{4}{B}(p-1)(q-2) A^2 u^2 |D u|^2 \nonumber \\&+ \frac{2B(p-1)(q-2)}{q} Q |D u|^{-4} |\Delta _\infty u|^2 (F + (q-2)Q|D u|^2/2). \end{aligned}$$

(A.9)

Now we choose A large such that

$$\begin{aligned}{} & {} 2(n-1) - 8\kappa _1^{-1}\kappa _2^2(p-1) + 2A - C(p)- 32 \Big (\frac{101}{100} + \frac{q-2}{2}\Big )^2\\{} & {} \quad - \frac{4B}{B-2}(p-1)(q-2) > 0. \end{aligned}$$

Then by (A.3), (A.5), (A.6), (A.7), (A.8), and (A.9), $a^{ij} D_{ij} F^{q/2} > 0$ in $\Omega _{r_0} \cap S_A$, and hence $F^{q/2}$ does not achieve its maximum in $\Omega _{r_0} \cap S_A$. This concludes the proof for the case when $p > 2$.

Case 2: For $p \in (1,2)$, we consider the quantify F given in (A.2). A similar argument as above shows that F does not achieve maximum on $(\Gamma _+ \cup \Gamma _-) \cap {\overline{\Omega }}_{r_0}\cap S_A$. In $\Omega _{r_0} \cap S_A$, we compute

$$\begin{aligned}&a^{ij} D_{ij} F\nonumber \\&\quad = a^{ij} D_{ij} Q |D u|^2 - 4(2-p)|D u|^{-2} D_i u D_i Q \Delta _\infty u + 4 D_i Q D_k u D_{ik} u + 2Q |D^2 u|^2\nonumber \\&\qquad + 2(2-p)Q |D |D u||^2 - 4 (2-p) Q|D u|^{-4} |\Delta _\infty u|^2 + 2A a^{ij} D_i u D_j u, \end{aligned}$$

(A.10)

where $a^{ij}$ is given in (6.5) satisfying (6.16). By a direct computation and (6.16), we have

$$\begin{aligned}&a^{ij} D_{ij} Q |D u|^2 + 2Aa^{ij} D_i u D_j u\nonumber \\&\quad = [2(n-1) - 8\kappa _1^{-1}\kappa _2^2 + (p-2)|D u|^{-2} (2|D_{x'} u|^2 - 8\kappa _1^{-1}\kappa _2^2 |D_n u|^2)] |D u|^2 \nonumber \\&\qquad + 2Aa^{ij} D_i u D_j u\nonumber \\&\quad \geqq \Big ( 2(n-1) - 8\kappa _1^{-1}\kappa _2^2 -2(2-p) + 2A(p-1) \Big ) |D u|^2. \end{aligned}$$

(A.11)

Note that

$$\begin{aligned} |D u|^{-4} |\Delta _\infty u|^2 \leqq |D |D u||^2 \leqq |D^2 u|^2. \end{aligned}$$

Therefore,

$$\begin{aligned}&2Q |D^2 u|^2 + 2(2-p)Q |D |D u||^2 - 4 (2-p) Q|D u|^{-4} |\Delta _\infty u|^2\nonumber \\&\quad \geqq 2(p-1)Q |D^2 u|^2. \end{aligned}$$

(A.12)

It remains to control

$$\begin{aligned} - 4(2-p)|D u|^{-2} D_i u D_i Q \Delta _\infty u + 4 D_i Q D_k u D_{ik} u =: I + II. \end{aligned}$$

By Young’s inequality and $|D Q|^2 \leqq 8Q$ for small $r_0$, we have

$$\begin{aligned} |I| \leqq&4(2-p) |D u|^{-1} |D Q| |\Delta _\infty u|\nonumber \\ \leqq&\frac{p-1}{16} |D Q|^2 |D u|^{-4} |\Delta _\infty u|^2 + \frac{64(2-p)^2}{p-1} |D u|^2\nonumber \\ \leqq&\frac{p-1}{2} Q|D u|^{-4} |\Delta _\infty u|^2 + \frac{64(2-p)^2}{p-1} |D u|^2, \end{aligned}$$

(A.13)

and

$$\begin{aligned} |II| \leqq&4|D Q| |D u| |D^2 u|\nonumber \\ \leqq&\frac{p-1}{16} |D Q|^2|D^2 u|^2 + \frac{64}{p-1}|D u|^2\nonumber \\ \leqq&\frac{p-1}{2} Q|D^2 u|^2 + \frac{64}{p-1}|D u|^2. \end{aligned}$$

(A.14)

Now we choose A large such that

$$\begin{aligned} 2(n-1) - 8\kappa _1^{-1}\kappa _2^2 -2(2-p) + 2A(p-1) - \frac{64(2-p)^2}{p-1} - \frac{64}{p-1} > 0. \end{aligned}$$

Then by (A.10), (A.11), (A.12), (A.13), and (A.14), $a^{ij} D_{ij} F > 0$ in $\Omega _{r_0} \cap S_A$, and hence F does not achieve its maximum in $\Omega _{r_0} \cap S_A$. This concludes the proof for the case when $p \in (1, 2)$. $\quad \square$