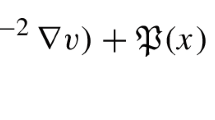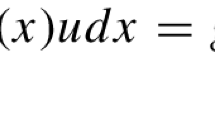Skip to main content
SpringerLink
Log in

Geometric Optics and Instability for Semi-Classical Schrödinger Equations

  • Published:
Archive for Rational Mechanics and Analysis Aims and scope Submit manuscript

Abstract

We prove some instability phenomena for semi-classical (linear or) nonlinear Schrödinger equations. For some perturbations of the data, we show that for very small times, we can neglect the Laplacian, and the mechanism is the same as for the corresponding ordinary differential equation. Our approach allows smaller perturbations of the data, where the instability occurs for times such that the problem cannot be reduced to the study of an ordinary differential equation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Boyd R.W. (1992). Nonlinear Optics. Academic Press, New York

    Google Scholar 

  2. Burq N., Gérard P., Tzvetkov N. (2002). An instability property of the nonlinear Schrödinger equation on S d. Math. Res. Lett. 9:323–335

    MathSciNet  Google Scholar 

  3. Burq N., Gérard P., Tzvetkov N. (2005). Multilinear eigenfunction estimates and global existence for the three dimensional nonlinear Schrödinger equations. Ann. Sci. École Norm. Sup. (4) 38:255–301

    Google Scholar 

  4. Burq N., Zworski M. (2005). Instability for the semiclassical non-linear Schrödinger equation. Comm. Math. Phys. 260:45–58

    Article  ADS  MathSciNet  Google Scholar 

  5. Carles R. (2000). Geometric optics with caustic crossing for some nonlinear Schrödinger equations. Indiana Univ. Math. J. 49:475–551

    Article  MathSciNet  Google Scholar 

  6. Carles R. (2003). Semi-classical Schrödinger equations with harmonic potential and nonlinear perturbation. Ann. Inst. H. Poincaré Anal. Non Linéaire 20:501–542

    Article  MathSciNet  Google Scholar 

  7. Carles, R.: Cascade of phase shifts for nonlinear Schrödinger equations. Preprint, available as arXiv:math.AP/0502242 (2005).

  8. Carles R.: WKB analysis for nonlinear Schrödinger equations with potential. Comm. Math. Phys., to appear (2006)

  9. Carles R., Fermanian Kammerer C., Gallagher I. (2003). On the role of quadratic oscillations in nonlinear Schrödinger equations. J. Funct. Anal. 203:453–493

    Article  MathSciNet  Google Scholar 

  10. Carles, R., Keraani, S.: On the role of quadratic oscillations in nonlinear Schrödinger equations II. The L 2-critical case. Trans. Amer. Math. Soc., to appear (2006)

  11. Chemin J.-Y. (1990). Dynamique des gaz à masse totale finie. Asymptotic Anal. 3:215–220

    MathSciNet  Google Scholar 

  12. Cheverry, C.: Cascade of phases in turbulent flows. Bull. Soc. Math. France, to appear (2005)

  13. Cheverry, C., Guès, O.: Counter-examples to the concentration-cancellation property. Preprint, (2005)

  14. Cheverry C., Guès O., Métivier G. (2004). Large amplitude high frequency waves for quasilinear hyperbolic systems. Adv. Differential Equations 9:829–890

    MathSciNet  Google Scholar 

  15. Christ M., Colliander J., Tao T. (2003). Asymptotics, frequency modulation, and low regularity ill-posedness for canonical defocusing equations. Amer. J. Math. 125:1235–1293

    MathSciNet  Google Scholar 

  16. Christ, M., Colliander, J., Tao, T.: Ill-posedness for nonlinear Schrödinger and wave equations. Ann. Inst. H. Poincaré Anal. Non Linéaire (2006), see also arXiv:math.AP/0311048

  17. Donnat P. (1994). Quelques Contributions Mathématiques en Optique Non Linéaire. Ph.D. thesis, École Polytechnique, Palaiseau (France)

    Google Scholar 

  18. Gérard, P.: Remarques sur l’analyse semi-classique de l’équation de Schrödinger non linéaire. Séminaire sur les Équations aux Dérivées Partielles, 1992–1993, École Polytech., Palaiseau, pp. Exp. No. XIII, 13, 1993

  19. Ginibre, J., Velo, G.: Sur une équation de Schrödinger non linéaire avec interaction non locale. Nonlinear Partial Differential Equations and their Applications, Collège de France Seminar (Brézis, H., Lions, J.-L. Eds.), Vol. 2, Research Notes in Mathematics, no. 60, Pitman, pp. 155–199, 1982

  20. Grassin M. (1998). Global smooth solutions to Euler equations for a perfect gas. Indiana Univ. Math. J. 47:1397–1432

    Article  MathSciNet  Google Scholar 

  21. Grenier E. (1998). Semiclassical limit of the nonlinear Schrödinger equation in small time. Proc. Amer. Math. Soc. 126:523–530

    Article  MathSciNet  Google Scholar 

  22. Hunter J., Keller J. (1987). Caustics of nonlinear waves. Wave Motion 9:429–443

    Article  MathSciNet  Google Scholar 

  23. Joly J.-L., Métivier G., Rauch J. (1995). Focusing at a point and absorption of nonlinear oscillations. Trans. Amer. Math. Soc. 347:3921–3969

    Article  MathSciNet  Google Scholar 

  24. Joly J.-L., Métivier G., Rauch J. (2000). Caustics for dissipative semilinear oscillations. Mem. Amer. Math. Soc. 144:viii+72

    Google Scholar 

  25. Joly J.-L., Métivier G., Rauch J. (2000). Nonlinear hyperbolic smoothing at a focal point. Michigan Math. J. 47:295–312

    Article  MathSciNet  Google Scholar 

  26. Kuksin S.B. (1995). On squeezing and flow of energy for nonlinear wave equations. Geom. Funct. Anal. 5:668–701

    Article  MathSciNet  Google Scholar 

  27. Lebeau, G.: Optique non linéaire et ondes sur critiques. Séminaire: Équations aux Dérivées Partielles, 1999–2000, École Polytech., Palaiseau, pp. Exp. No. IV, 13, 2000

  28. Lebeau G. Non linear optic and supercritical wave equation. Hommage à Pascal Laubin. Bull. Soc. Roy. Sci. Liège 70 (2001), 267–306 (2002).

    Google Scholar 

  29. Lebeau G. (2005). Perte de régularité pour les équations d’ondes sur-critiques. Bull. Soc. Math. France 133:145–157

    MathSciNet  Google Scholar 

  30. Majda A. (1984). Compressible Fluid Flow and Systems of Conservation Laws in Several Space Variables. Applied Mathematical Sciences, Vol. 53, Springer-Verlag, New York

    MATH  Google Scholar 

  31. Makino T., Ukai S., Kawashima S. (1986). Sur la solution à support compact de l’équation d’Euler compressible. Japan J. Indust. Appl. Math. 3:249–257

    MathSciNet  Google Scholar 

  32. Métivier G. (2004). Exemples d’instabilités pour des équations d’ondes non linéaires (d’après G. Lebeau). Astérisque 294:63–75

    Google Scholar 

  33. Niederer U. (1974). The maximal kinematical invariance groups of Schrödinger equations with arbitrary potentials. Helv. Phys. Acta 47:167–172

    MathSciNet  Google Scholar 

  34. Rauch, J., Keel, M.: Lectures on geometric optics. Hyperbolic Equations and Frequency Interactions (Park City, UT, 1995), American Mathematical Society, Providence, RI, pp. 383–466, 1999

  35. Sideris T. (1985). Formation of singularities in three-dimensional compressible fluids. Comm. Math. Phys. 101:475–485

    Article  ADS  MathSciNet  Google Scholar 

  36. Zakharov V.E., Shabat A.B. (1971). Exact theory of two-dimensional self-focusing and one-dimensional self-modulation of waves in nonlinear media. vZ. Èksper. Teoret. Fiz. 61:118–134

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Mathématiques Appliquées de Bordeaux, Université de Bordeaux 1, 351 cours de la Libération, 33405, Talence Cedex, France

    Rémi Carles

Authors
  1. Rémi Carles
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Rémi Carles.

Additional information

Communicated by L.C. Evans

Rights and permissions

Reprints and permissions

About this article

Cite this article

Carles, R. Geometric Optics and Instability for Semi-Classical Schrödinger Equations. Arch Rational Mech Anal 183, 525–553 (2007). https://doi.org/10.1007/s00205-006-0017-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00205-006-0017-5

Keywords

Search

Navigation