Skip to main content
SpringerLink
Log in

Beyond the limitation of geometrical shock dynamics for diffraction over wedges

  • Original Article
  • Published:
Shock Waves Aims and scope Submit manuscript

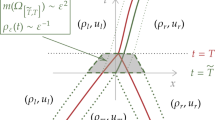
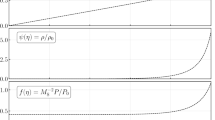
Abstract

Geometrical shock dynamics (GSD) is a simplified model for nonlinear shock wave propagation for which the front evolution is governed by a local relation between the geometry of the shock and its velocity, the so-called A–M rule. Numerous studies have proven the ability of the GSD model to estimate correctly the leading shock front in interactions with obstacles. Nevertheless, a solution for the problem of diffraction over a convex corner does not always exist, especially for weak shocks. To overcome this limitation, we propose an ad hoc modification of the A–M relation for two-dimensional configurations: an extra term based on the transverse variation of the Mach number is added. This new closure is fitted against experimental observations, which ensures, by construction, a correct behaviour for expansive shocks. A Lagrangian numerical solver is developed, for which this new term is activated only on specific parts of the front. Results of this new model are compared with the original GSD model, experiments, and Eulerian simulations for several cases of increasing complexity. A noticeable improvement in the solution is observed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24

Similar content being viewed by others

References

  1. Whitham, G.: A new approach to problems of shock dynamics. Part I: Two-dimensional problems. J. Fluid Mech. 2(2), 145–171 (1957). https://doi.org/10.1017/S002211205700004X

    Article  MathSciNet  MATH  Google Scholar 

  2. Whitham, G.: A new approach to problems of shock dynamics. Part II: Three-dimensional problems. J. Fluid Mech. 5(3), 369–386 (1959). https://doi.org/10.1017/S002211205900026X

    Article  MathSciNet  MATH  Google Scholar 

  3. Whitham, G.: Linear and Nonlinear Waves, 3rd edn., Chap. 8: Shock Dynamics. Wiley, London (1999). https://doi.org/10.1002/9781118032954

    Book  Google Scholar 

  4. Best, J.: A generalisation of the theory of geometrical shock dynamics. Shock Waves 1(4), 251–273 (1991). https://doi.org/10.1007/BF01418882

    Article  MATH  Google Scholar 

  5. Schwendeman, D., Whitham, G.: On converging shock waves. Proc. R. Soc. Lond. A Math. Phys. Eng. Sci. 413(1845), 297–311 (1987). https://doi.org/10.1098/rspa.1987.0116

    Article  MathSciNet  MATH  Google Scholar 

  6. Schwendeman, D.: On converging shock waves of spherical and polyhedral form. J. Fluid Mech. 454, 365–386 (2002). https://doi.org/10.1017/S0022112001007170

    Article  MathSciNet  MATH  Google Scholar 

  7. Catherasoo, C., Sturtevant, B.: Shock dynamics in non-uniform media. J. Fluid Mech. 127, 539–561 (1983). https://doi.org/10.1017/S0022112083002876

    Article  MathSciNet  MATH  Google Scholar 

  8. Besset, C., Blanc, E.: Propagation of vertical shock waves in the atmosphere. J. Acoust. Soc. Am. 95(4), 1830–1839 (1994). https://doi.org/10.1121/1.408689

    Article  Google Scholar 

  9. Mostert, W., Pullin, D., Samtaney, R., Wheatley, V.: Geometrical shock dynamics for magnetohydrodynamic fast shocks. J. Fluid Mech. 811, R2 (2017). https://doi.org/10.1017/jfm.2016.767

  10. Skews, B.: The shape of a diffracting shock wave. J. Fluid Mech. 29(02), 297–304 (1967). https://doi.org/10.1017/S0022112067000825

    Article  Google Scholar 

  11. Bazhenova, T., Gvozdeva, L., Zhilin, Y.: Change in the shape of the diffracting shock wave at a convex corner. Acta Astronaut. 6(3), 401–412 (1979). https://doi.org/10.1016/0094-5765(79)90107-3

    Article  Google Scholar 

  12. Sharma, V., Radha, C.: On one-dimensional planar and nonplanar shock waves in a relaxing gas. Phys. Fluids 6(6), 2177–2190 (1994). https://doi.org/10.1063/1.868220

    Article  MathSciNet  MATH  Google Scholar 

  13. Sharma, V., Radha, C.: Three dimensional shock wave propagation in an ideal gas. Int. J. Nonlinear Mech. 30(3), 305–322 (1995). https://doi.org/10.1016/0020-7462(95)00005-9

    Article  MATH  Google Scholar 

  14. Pandey, M., Sharma, V.: Kinematics of a shock wave of arbitrary strength in a non-ideal gas. Q. Appl. Math. 67(3), 401–418 (2009). https://doi.org/10.1090/S0033-569X-09-01111-5

    Article  MathSciNet  MATH  Google Scholar 

  15. Ridoux, J., Lardjane, N., Monasse, L., Coulouvrat, F.: Comparison of geometrical shock dynamics and kinematic models for shock wave propagation. Shock Waves 28(2), 401–416 (2017). https://doi.org/10.1007/s00193-017-0748-2

    Article  Google Scholar 

  16. Oshima, K., Sugaya, K., Yamamoto, M., Totoki, T.: Diffraction of a plane shock wave around a corner. ISAS Rep. 30(2), 51–82 (1965)

    Google Scholar 

  17. Oshima, K.: Propagation of spacially non-uniform shock waves. ISAS Rep. 30(6), 195 (1965)

    Google Scholar 

  18. Henshaw, W., Smyth, N., Schwendeman, D.: Numerical shock propagation using geometrical shock dynamics. J. Fluid Mech. 171, 519–545 (1986). https://doi.org/10.1017/S0022112086001568

    Article  MATH  Google Scholar 

  19. Schwendeman, D.: A numerical scheme for shock propagation in three dimensions. Proc. R. Soc. Lond. A Math. Phys. Eng. Sci. 416(1850), 179–198 (1988). https://doi.org/10.1098/rspa.1988.0033

    Article  MathSciNet  MATH  Google Scholar 

  20. Schwendeman, D.: A new numerical method for shock wave propagation based on geometrical shock dynamics. Proc. R. Soc. Lond. A Math. Phys. Eng. Sci. 441(1912), 331–341 (1993). https://doi.org/10.1098/rspa.1993.0064

    Article  MathSciNet  MATH  Google Scholar 

  21. Schwendeman, D.: A higher-order Godunov method for the hyperbolic equations modelling shock dynamics. Proc. R. Soc. Lond. A Math. Phys. Eng. Sci. 455(1984), 1215–1233 (1999). https://doi.org/10.1098/rspa.1999.0356

    Article  MathSciNet  MATH  Google Scholar 

  22. Tariq, D., Bdzil, J., Stewart, D.: Level set methods applied to modeling detonation shock dynamics. J. Comput. Phys. 126(2), 390–409 (1996). https://doi.org/10.1006/jcph.1996.0145

    Article  MathSciNet  MATH  Google Scholar 

  23. Noumir, Y., Le Guilcher, A., Lardjane, N., Monneau, R., Sarrazin, A.: A fast-marching like algorithm for geometrical shock dynamics. J. Comput. Phys. 284, 206–229 (2015). https://doi.org/10.1016/j.jcp.2014.12.019

    Article  MathSciNet  MATH  Google Scholar 

  24. Huynh, H.: Accurate monotone cubic interpolation. SIAM J. Numer. Anal. 30(1), 57–100 (1993). https://doi.org/10.1137/0730004

    Article  MathSciNet  MATH  Google Scholar 

  25. Gottlieb, S., Shu, C.: Total variation diminishing Runge–Kutta schemes. Math. Comput. Am. Math. Soc. 67(221), 73–85 (1998). https://doi.org/10.1090/S0025-5718-98-00913-2

    Article  MathSciNet  MATH  Google Scholar 

  26. Osher, S., Shu, C.: High-order essentially nonoscillatory schemes for Hamilton–Jacobi equations. SIAM J. Numer. Anal. 28(4), 907–922 (1991). https://doi.org/10.1137/0728049

    Article  MathSciNet  MATH  Google Scholar 

  27. Romon, P.: Introduction à la géométrie différentielle discrète. Ellipses (2013). https://www.eyrolles.com/Sciences/Livre/introduction-a-la-geometrie-differentielle-discrete-9782729883072/

  28. Shi, W., Cheung, C.: Performance evaluation of line simplification algorithms for vector generalization. Cartogr. J. 43(1), 27–44 (2006). https://doi.org/10.1179/000870406X93490

    Article  Google Scholar 

  29. Kvasov, B.: Monotone and convex interpolation by weighted quadratic splines. Adv. Comput. Math. 40(1), 91–116 (2014). https://doi.org/10.1007/s10444-013-9300-9

    Article  MathSciNet  MATH  Google Scholar 

  30. Jourdren, H.: HERA: a hydrodynamic AMR platform for multi-physics simulations. In: Adaptive Mesh Refinement—Theory and Applications, pp. 283–294. Springer, Berlin (2005). https://doi.org/10.1007/3-540-27039-6_19

  31. Lorensen, W., Cline, H.: Marching cubes: A high resolution 3D surface construction algorithm. ACM Siggr. Comput. Graph. 21(4), 163–169 (1987). https://doi.org/10.1145/37401.37422

    Article  Google Scholar 

  32. Ridoux, J.: Contribution au développement d’une méthode de calcul rapide de propagation des ondes de souffle en présence d’obstacles. PhD Thesis, Université Pierre et Marie Curie (2017)

  33. Skews, B.: Shock wave diffraction on multi-facetted and curved walls. Shock Waves 14(3), 137–146 (2005). https://doi.org/10.1007/s00193-005-0266-5

    Article  MATH  Google Scholar 

  34. Ben-Dor, G.: Handbook of Shock Waves, vol. 2, Chap. 8.1: Oblique Shock Wave Reflections, pp. 68–179. Academic, New York (2000). https://doi.org/10.1016/B978-012086430-0/50022-1

    Google Scholar 

  35. Schwendeman, D.: Numerical shock propagation in non-uniform media. J. Fluid Mech. 188, 383–410 (1988). https://doi.org/10.1017/S0022112088000771

    Article  MATH  Google Scholar 

Download references

Acknowledgements

Part of this work has been possible thanks to the LETMA collaboration: a contractual research laboratory between CEA, CNRS, École Centrale Lyon, C-Innov, and Sorbonne Université. We thank D.W. Schwendeman, from Rensselaer Polytechnic Institute, Troy, NY, USA, for useful discussions about the Lagrangian scheme.

Author information

Authors and Affiliations

  1. CEA, DAM, DIF, 91297, Arpajon, France

    J. Ridoux & N. Lardjane

  2. Université Côte d’Azur, Inria, CNRS, LJAD, EPC COFFEE, Parc Valrose, 06108, Nice, France

    L. Monasse

  3. Institut Jean Le Rond d’Alembert, UMR 7190 CNRS, Sorbonne Université, Paris, France

    J. Ridoux & F. Coulouvrat

Authors
  1. J. Ridoux
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. N. Lardjane
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. L. Monasse
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. F. Coulouvrat
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to N. Lardjane.

Additional information

Communicated by M. Brouillette and A. Higgins.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ridoux, J., Lardjane, N., Monasse, L. et al. Beyond the limitation of geometrical shock dynamics for diffraction over wedges. Shock Waves 29, 833–855 (2019). https://doi.org/10.1007/s00193-018-00885-w

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00193-018-00885-w

Keywords

Search

Navigation