Mortality, fertility, education and capital accumulation in a simple OLG economy

Abstract

We develop a simple OLG model to analytically show that aging leads to increased educational efforts through a general equilibrium effect. The mechanism is that scarcity of raw labor increases the return of human relative to physical capital. While a reduction in the birth rate is shown to unambiguously increase educational efforts, increases in the survival rate have ambiguous effects. Falling birth rates also increase capital per worker, but the effects of rising survival rates are again ambiguous. We conclude that our model is a useful laboratory to highlight potentially offsetting effects in models with endogenous education and overlapping generations.

Appendices

Appendix

1.1 A Proofs

Proof of Proposition 1

We have that

$$ k_{t+1} = \frac{K_{t+1}}{A_{t+1}L_{t+1}}, $$

which, by Eq. 22, can be rewritten as

$$k_{t+1} \frac{L_{t+1}}{N_{t,0}} = \frac{a_{t,0}}{A_{t+1}} $$

(32)

We first work on the left-hand side (LHS) of Eq. 32. Using Eq. 23, we get

$$ k_{t+1} \frac{L_{t+1}}{N_{t,0}} = k_{t+1} h_0 \left((1-e_{t+1}) \gamma^N_{t+1} + \omega s_{t+1} (1+g(e_{t})) \right). $$

(33)

Next, we focus on the right-hand side (RHS) of Eq. 32). Using Eqs. 5, 9, and 20 in Eq. 14 and bringing the terms involving a _t,0 to the LHS of the resulting expression, we get

$$\begin{array}{rll} &a_{t,0}&\!\left(1\!+\!\frac{(1\!-\!s_{t+1})(1\!-\!\lambda)}{(1+\beta s_{t+1})\zeta_{t+1}}\right)\\ &=& \frac{h_0}{1+ \beta s_{t+1} } \left(\frac{}{}\beta s_{t+1} (1-e_t) (1-\tau_t) w_t\right. \nonumber\\&&{\kern50pt} \left. -\,\frac{w_{t+1}}{\zeta_{t+1}(1+r_{t+1})} \left( s_{t+1} \omega (1+g(e_t))\!+\!\tau_{t+1} (1\!-\!e_{t+1}) \gamma^N_{t+1} \right) \right). \end{array}$$

Bringing the term postmultiplying a _t,0 to the RHS, replacing r _t and w _t with their marginal products from Eq. 18, and dividing by A _t + 1 gives

$$\begin{array}{rll} \frac{a_{t,0}}{A_{t+1}}&=& \varphi_t \frac{h_0}{1\!+\!\beta s_{t+1}} \left(\beta s_{t+1} (1\!-\!e_t) (1\!-\!\tau_t) (1\!-\!\alpha)k_t^{\alpha}\frac{1}{\gamma^A} \right. \nonumber\\ &&{\kern54pt} \left. -\,\frac{1\!-\!\alpha}{\alpha \zeta_{t+1}}k_{t+1} \left(s_{t+1} \omega (1\!+\!g(e_t)) \! +\! \tau_{t+1} (1\!-\!e_{t+1})\gamma^N_{t+1} \right) \right), \end{array}$$

(34)

where

$$ \varphi_t = \frac{(1+\beta s_{t+1})\zeta_{t+1}}{(1+\beta s_{t+1})\zeta_{t+1}+(1-s_{t+1})(1-\lambda)}. $$

(35)

Next, use the equation above and combine it with Eq. 33 to get

$$\begin{array}{rll} &k_{t+1}& \left(\frac{}{} (1-e_{t+1}) \gamma^N_{t+1} + s_{t+1} \omega(1+g(e_{t})) \right. \nonumber\\&&{\kern12pt} \left. +\, \varphi_t \frac{1-\alpha}{\alpha (1+ \beta s_{t+1}) \zeta_{t+1}} \left( s_{t+1} \omega (1+g(e_t)) + \tau_{t+1} (1-e_{t+1}) \gamma^N_{t+1} \right) \right) \nonumber\\[6pt]&=& \varphi_t \frac{\beta s_{t+1} (1-\alpha)}{\gamma^A(1+ \beta s_{t+1})} (1-e_t) (1-\tau_t) k_t^\alpha. \end{array}$$

Multiply the above by α(1 + βs _t + 1) and simplify to get

$$\begin{array}{rll} &k_{t+1}& \left( (1-e_{t+1}) \gamma^N_{t+1} \left(\alpha (1+ \beta s_{t+1}) + \varphi_t \frac{(1-\alpha) \tau_{t+1}}{\zeta_{t+1}} \right) \right. \nonumber\\&&{\kern12pt} \left.+\, s_{t+1} \omega (1+g(e_{t})) \left( \alpha (1+ \beta s_{t+1}) + \varphi_t \frac{1-\alpha}{\zeta_{t+1}} \right) \right) \nonumber\\&=& \varphi_t \frac{\alpha (1-\alpha) \beta s_{t+1} }{\gamma^A} (1-e_t) (1-\tau_t) k_t^\alpha. \end{array}$$

The expression for e _t immediately follows from replacing wages and interest rates by their respective counterparts from Eqs. 18a and 18b. Using $\hat{\rho}=\frac{1}{\beta s_{t+1}}-1$ proves the claim in the proposition.□

Proof of Proposition 2

First, given that the function g(e) satisfies the lower Inada condition with lim_{e →0} g′(e) → ∞, the solution with zero education is excluded for ω ∈ (0,1]. Second, having full educational investment (i.e., e = 1), labor supply and, thus, wage income of the young generation are zero. By the lower Inada condition of the utility function, we have that c _t,0 > 0 for positive wages. Consequently, savings in the first period would be negative, as would be the capital stock of the economy. Thus, if there is an equilibrium with finite and positive capital stock, education will always be lower than unity.

To show that education always converges to the steady state solution, use Eq. 24a in Eq. 24b and rewrite the resulting expression as

$$ e_t^{1-\psi} = \frac{s_{t+1}}{\zeta_{t+1}} \omega \xi \psi \frac{(1-\alpha) (1-\tau_{t+1}) }{ \left( \Gamma_1\displaystyle\frac{1-e_{t+1}}{1-e_t} + \Gamma_2\frac{1+g(e_{t})}{1-e_t} \right) }, $$

where

$$ \begin{array}{lll} \Gamma_1&\equiv&\left(\alpha (2+ \hat{\rho}_{t+1} ) + \varphi_t \frac{(1-\alpha) \tau_{t+1}}{\zeta_{t+1}} (1+ \hat{\rho}_{t+1}) \right) \gamma^N_{t+1}\\[8pt] \Gamma_2 &\equiv& \omega s_{t+1} \left( \alpha (2+ \hat{\rho}_{t+1} ) + \varphi_t \frac{1-\alpha}{\zeta_{t+1}} (1+ \hat{\rho}_{t+1} )\right) \end{array} $$

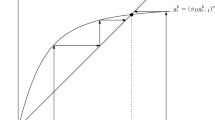
$\Delta_t \equiv e_t-e_{t+1}(k^*)$ is defined as measuring the distance between e _t and e _t + 1, which is ultimately a function of the steady state capital stock. Thus, Δ _t measures the change in education between t and t + 1 outside the steady state. Rearranging gives

$$ F(e_t,\Delta_t)=e_t^{1-\psi}-\frac{s_{t+1}}{\zeta_{t+1}} \omega \xi \psi \frac{(1-\alpha) (1-\tau_{t+1})}{\left(\Gamma_1\displaystyle\frac{1-e_{t}+\Delta_t}{1-e_t}+ \Gamma_2\frac{1+g(e_{t})}{1-e_t} \right)}. $$

(36)

Taking the derivative of e _t with respect to the distance to the steady state gives

$$ \frac{\partial e_t}{\partial \Delta_t}=-\displaystyle\frac{\partial F / \partial \Delta_t}{\partial F / \partial e_t}<0 $$

(37a)

$$ \frac{\partial^2 e_t}{\partial \Delta_t^2}>0. $$

(37b)

Therefore, if education is, e.g., below its new steady state level after an exogenous shock (i.e., Δ _t  < 0), e _t will always converge monotonically to the new steady state value.□

Proof of Proposition 3

Existence:

Using Eq. 22 and the assumption of constant population growth, we have

$$ k_{t+1} =\frac{1}{\gamma^N\gamma^A}\tilde{a}_{t,0}, $$

where $\tilde{a}_{t,0}$ is Eq. 22 divided by A _t to transform a _t,0 into savings per efficient worker. Define the function

$$ d(w_t,r_{t+1})=\gamma^A\gamma^Nk_{t+1}-\tilde{a}(w_t(k_t),r_{t+1}(k_{t+1}),e_t(k_{t+1})), $$

(38a)

where d(·) is the change in the capital stock per effective worker. Given that we use log-utility, e _t  ∈ (0,1), and a Cobb–Douglas production function, it holds that

$$ 0<\tilde{a}(w_t,r_{t+1},e_t)<\tilde{w}_t,$$

(38b)

$$ 0<\displaystyle\frac{\tilde{a}(w_t,r_{t+1},e_t)}{k_{t+1}}<\frac{\tilde{w}_t}{k_{t+1}}, $$

(38c)

where $\tilde{w_t}$ denotes wages scaled by the level of technology. All we have to show is that d(·) has opposite signs for k _t + 1 going to zero and infinity. Then, by continuity of d(·), there is at least one capital stock satisfying d(·) = 0. This holds since

$$ \frac{d(w_t,r_{t+1})}{k_{t+1}}=\gamma^N\gamma^A-\frac{\tilde{a}(w_t,r_{t+1},e_t)}{k_{t+1}}, $$

(38d)

and, taking the limits, gives

$$ \lim\limits_{k_{t+1}\rightarrow \infty}\displaystyle\frac{d(w_t,r_{t+1})}{k_{t+1}}=\gamma^N\gamma^A>0$$

(38e)

$$ \lim\limits_{k_{t+1}\rightarrow 0}\displaystyle\frac{d(w_t,r_{t+1})}{k_{t+1}}=-\infty <0 $$

(38f)

for sufficiently small k _t + 1. For uniqueness, it is sufficient to show that $\partial d(w_t,r_{t+1})/\partial k_{t+1}>0$ for all k, i.e., that for a given wage rate, d(w _t ,r _t + 1) is nondecreasing in the capital stock. Taking Eq. 25a and recalling that $\partial e/\partial k>0$ establishes the result. By using Eq. 26b, it is clear that a unique solution for the capital stock automatically gives a unique e.□

Proof of Proposition 4

From Eq. 26, define

$$ F_1(k,e;\gamma^N,s,\lambda,\omega) = \Omega(e,\gamma^N,s,\lambda,\omega)^{\frac{1}{1-\alpha}}-k = 0$$

(39a)

$$ F_2(k,e;\gamma^N,s,\lambda,\omega) = c \cdot \left( \frac{s}{\zeta} \right)^{\frac{1}{1-\psi}} \cdot k^{\frac{1-\alpha}{1-\psi}}-e = 0, $$

(39b)

where

$$ \Omega(e,\gamma^N,s,\lambda,\omega) \equiv \frac{\varphi}{\phi} (1-\tau)\alpha (1 - \alpha) \beta $$

(40)

$$ c \equiv \left[\omega\xi\psi\displaystyle\frac{\gamma^A}{\alpha}\right]^{\frac{1}{1-\psi}} $$

(41)

and ϕ is as in Eq. 27a and φ is as in Eq. 27b.

• 1. For the case where $\tau = \bar{\tau}$, we can ignore that τ is related to γ ^N and s by the steady state version of Eq. 21. The general problem with two implicitly defined endogenous variables can be written as

  $$ \begin{bmatrix} \displaystyle\frac{\partial k}{\partial X} \\[12pt] \displaystyle\frac{\partial e}{\partial X} \end{bmatrix} = - \begin{bmatrix} \displaystyle\frac{\partial F_1}{\partial k} & \displaystyle\frac{\partial F_1}{\partial e} \\[12pt] \displaystyle\frac{\partial F_2}{\partial k} & \displaystyle\frac{\partial F_2}{\partial e} \end{bmatrix}^{-1} \begin{bmatrix} \displaystyle\frac{\partial F_1}{\partial X} \\[12pt] \displaystyle\frac{\partial F_2}{\partial X} \end{bmatrix} = - A^{-1} \begin{bmatrix} \displaystyle\frac{\partial F_1}{\partial X} \\[12pt] \displaystyle\frac{\partial F_2}{\partial X}, \end{bmatrix} $$

  (42)

  where X is any variable from the vector of exogenous variables {γ _N ,s,ω,}, and therefore,

  $$ \begin{bmatrix} \displaystyle\frac{\partial k}{\partial X} \\[12pt] \displaystyle\frac{\partial e}{\partial X} \end{bmatrix} = - | A |^{-1} \begin{bmatrix} \displaystyle\frac{\partial F_2}{\partial e} & -\displaystyle\frac{\partial F_1}{\partial e} \\[12pt] -\displaystyle\frac{\partial F_2}{\partial k} & \displaystyle\frac{\partial F_1}{\partial k} \end{bmatrix} \begin{bmatrix} \displaystyle\frac{\partial F_1}{\partial X} \\[12pt] \displaystyle\frac{\partial F_2}{\partial X}, \end{bmatrix} $$

  (43)

  and rearranging gives

  $$ \begin{bmatrix} \displaystyle\frac{\partial k}{\partial X} \\[12pt] \displaystyle\frac{\partial e}{\partial X} \end{bmatrix} = - | A |^{-1} \begin{bmatrix} \displaystyle\frac{\partial F_2}{\partial e}\displaystyle\frac{\partial F_1}{\partial X} & -\displaystyle\frac{\partial F_1}{\partial e}\displaystyle\frac{\partial F_2}{\partial X} \\[12pt] -\displaystyle\frac{\partial F_2}{\partial k} \displaystyle\frac{\partial F_1}{\partial X} & + \displaystyle\frac{\partial F_1}{\partial k}\displaystyle\frac{\partial F_2}{\partial X} \end{bmatrix} $$

  (44)

  Since $\tau = \bar{\tau}$, we get

  $$ \frac{\partial F_1}{\partial k} = -1 < 0 $$

  (45a)
  $$ \frac{\partial F_1}{\partial e} = \frac{1}{1-\alpha} \Omega^{1/(1-\alpha)-1} \displaystyle\frac{\partial \Omega}{\partial e} < 0 $$

  (45b)
  $$ \frac{\partial F_2}{\partial k} = c \left( \frac{s}{\zeta} \right)^{\frac{1}{1-\psi}} \displaystyle\frac{1-\alpha}{1-\psi} k^{\frac{1-\alpha}{1-\psi}-1} > 0 $$

  (45c)
  $$ \frac{\partial F_2}{\partial e} = -1 < 0, $$

  (45d)

  whereby the sign in Eq. 45b follows from $\frac{\partial \Omega}{\partial e} < 0$. Consequently,

  $$ | A | = \underbrace{\frac{\partial F_1}{\partial k} \frac{\partial F_2}{\partial e}}_{=1} - \underbrace{\frac{\partial F_1}{\partial e} \frac{\partial F_2}{\partial k}}_{<0} > 0. $$

  (46)

  • a. To determine the effect of a changing population growth rate γ _N on k and e, we have to replace X by γ _N in Eq. 42, which gives

    $$ \frac{\partial F_1}{\partial \gamma^N} = \frac{1}{1-\alpha} \Omega^{1/(1-\alpha)-1} \frac{\partial \Omega}{\partial \gamma^N} < 0 $$

    (47a)
    $$ \frac{\partial F_2}{\partial \gamma^N} = 0, $$

    (47b)

    whereby Eq. 47a follows from $\frac{\partial \Omega}{\partial \gamma^N} < 0$, cf. Eqs. 40 and 27a. To get an intuitive idea of what is determining the sign, note that we can write

    $$ \frac{\partial \Omega}{\partial \gamma^N}=\frac{\partial \varphi/\partial \gamma^N \phi -\varphi \partial \phi/\partial \gamma^N}{\phi^2}=-\frac{\varphi \partial \phi/\partial \gamma^N}{\phi^2}<0 $$

    (48)

    since φ is independent of γ ^N and ϕ is a positive function of γ ^N, cf. Eqs. 26a and 27. Thus, γ ^N has a direct effect on k but only an indirect effect on e via changing relative prices (this is the reason why $\partial F_2/\partial \gamma^N=0$). Formally, we have

    $$ \frac{\partial k}{\partial \gamma^N} = - |A|^{-1} \left( \underbrace{\frac{\partial F_2}{\partial e} \frac{\partial F_1}{\partial \gamma^N}}_{>0}-\underbrace{\frac{\partial F_1}{\partial e} \frac{\partial F_2}{\partial \gamma^N}}_{=0}\right) < 0 $$

    (49a)
    $$ \frac{\partial e}{\partial \gamma^N} = -|A|^{-1} \left( - \underbrace{\frac{\partial F_2}{\partial k} \frac{\partial F_1}{\partial \gamma^N}}_{<0} + \underbrace{\frac{\partial F_1}{\partial k} \frac{\partial F_2}{\partial \gamma^N}}_{=0}\right) < 0. $$

    (49b)

  • b. To derive the analogous steps for differentiation of Eq. 39 with respect to s, replace the terms in Eq. 47 by

    $$ \frac{\partial F_1}{\partial s} = \displaystyle\frac{1}{1-\alpha} \Omega^{1/(1-\alpha)-1} \frac{\partial \Omega}{\partial s} \gtrless 0 $$

    (50a)
    $$ \frac{\partial F_2}{\partial s} = c k^{\frac{1-\alpha}{1-\psi}} \displaystyle\frac{\partial \frac{s}{\zeta}}{\partial s} \geq 0. $$

    (50b)

    giving

    $$ \frac{\partial k}{\partial s} = - |A|^{-1} \left( \underbrace{\frac{\partial F_2}{\partial e} \frac{\partial F_1}{\partial s}}_{\gtrless0}-\underbrace{\frac{\partial F_1}{\partial e} \frac{\partial F_2}{\partial s}}_{\leq0}\right) \gtrless0 $$

    (51a)
    $$ \frac{\partial e}{\partial s} = -|A|^{-1} \left( - \underbrace{\frac{\partial F_2}{\partial k} \frac{\partial F_1}{\partial s}}_{\gtrless0} + \underbrace{\frac{\partial F_1}{\partial k} \frac{\partial F_2}{\partial s}}_{\leq0}\right) \gtrless0. $$

    (51b)

    Intuitively, the ambiguity of $\frac{\partial e}{\partial s}$ results from the fact that, holding k constant, e is increasing in s as long as λ > 0 (direct effect), but the capital stock may increase or decrease in s for given education e. As e increases in k monotonically, the ambiguity of $\frac{\partial k}{\partial s}$ translates into the ambiguity of $\frac{\partial e}{\partial s}$ (indirect effect of s on e).

    Arguing formally, the ambiguity of $\frac{\partial k}{\partial s}$ comes from

    $$ \frac{\partial \Omega}{\partial s}=\frac{\partial (\varphi/\phi)}{\partial s}= \alpha(1-\alpha)\beta(1-\tau)\frac{\varphi'\phi-\varphi \phi'}{\phi^2} \gtrless 0, $$

    (52)

    where $\phi'=\partial \phi/\partial s$ and $\varphi' = \partial \varphi/\partial s$, cf. Eq. 26a. It can be shown that φ′ > 0. Consequently, the sign of $\frac{\partial \phi}{\partial s}$ determines the sign of $\frac{\partial F_1}{\partial s}$ (and thus, $\frac{\partial \Omega}{\partial s}$), and therefore, the sign of Eq. 50a is unambiguous only if $\frac{\partial \phi}{\partial s}<0$.

    To see what determines the sign of ϕ′, observe from Eq. 27a that s enters in three places: (1) s pre-multiplies the term $\omega \frac{1+g(e)}{1-e}$; (2) s decreases the effective discount rate $\hat{\rho}$; and (3) s increases the annuity factor, ζ, as long as λ < 1. Consequently, ϕ increases in s by effect 1, whereas it decreases in s by the effects 2 and 3. We can therefore study an upper bound of ϕ′ by setting λ = 1 so that effect 3 is not at work.

    This helps to clarify the interaction at the cost of introducing a special case. Using $\hat{\rho}=\frac{1}{\beta s}-1$ in Eq. 27a and taking the derivative of the resulting equation with respect to s gives

    $$ \frac{\partial \phi}{\partial s} \frac{1}{\gamma_A}=\omega \alpha \frac{1+g(e)}{1-e}-\frac{\gamma^N}{s^2\beta}\left[1-(1-\alpha)\frac{}{}(1-\tau)\right]\gtrless 0, $$

    which is ambiguous.¹² The right part of this equation consists only of exogenous variables. The left part involves the endogenous education decision e for which no closed-form solution is available. Thus, it is not possible to show analytically that the derivative has an unambiguous sign. However, constructing a few special cases clarifies under which conditions $\frac{\partial \phi}{\partial s}<0$ may hold.

    • For ω → 0, the left part converges to zero (e also converges to zero), and thus, $\frac{\partial \phi}{\partial s}<0$.

    • For ω = 1, which implies that τ = 0, we have

      $$ \frac{\partial \phi}{\partial s} \frac{1}{\gamma_A}=\alpha \left(\frac{1+g(e)}{1-e} -\frac{\gamma^N}{s^2 \beta} \right) \gtrless 0. $$

    • For ξ → 0 or ψ → 0, we have that e → 0, which means that

      $$ \frac{\partial \phi}{\partial s} \frac{1}{\gamma_A}=\omega \alpha -\frac{\gamma^N}{s^2\beta}\left[1-(1-\alpha)\frac{}{}(1-\tau)\right]\gtrless 0. $$

    Summarizing the arguments made so far, the sign of $\frac{\partial \phi}{\partial s}$ is negative (implying that k is increasing in s) if

    • Returns to education are low (low ξ and/or ψ)

    • The horizon over which the benefits can be reaped is short (low ω)

    • The discount factor β is low (i.e., high discount rate)

    • The population growth rate γ ^N is high

    • The survival probability s is low

  • c. Changing the planning horizon ω gives

    $$ \frac{\partial F_1}{\partial \omega} = \displaystyle\frac{\partial \Omega}{\partial \omega} < 0 $$

    (53a)
    $$ \frac{\partial F_2}{\partial \omega} = \displaystyle\frac{1}{1-\psi}\omega^{\frac{\psi}{1-\psi}} \left( \xi \psi \frac{\gamma^A }{\alpha }\right)^{\frac{1}{1-\psi}} \left( \frac{s}{\zeta} \right)^{\frac{1}{1-\psi}} k^{\frac{1-\alpha}{1-\psi}} >0, $$

    (53b)

    and therefore,

    $$ \frac{\partial k}{\partial \omega} = - |A|^{-1} \left(\underbrace{\frac{\partial F_2}{\partial e} \frac{\partial F_1}{\partial \omega}}_{ > 0} - \underbrace{\frac{\partial F_1}{\partial e} \frac{\partial F_2}{\partial \omega}}_{<0} \right) < 0 $$

    (54a)
    $$ \frac{\partial e}{\partial \omega} = - |A|^{-1} \left(- \underbrace{\frac{\partial F_2}{\partial k} \frac{\partial F_1}{\partial \omega}}_{<0} + \underbrace{\frac{\partial F_1}{\partial k} \frac{\partial F_2}{\partial \omega} }_{<0} \right) \gtrless 0. $$

    (54b)

    Some intuition as to why the sign of $\partial e/\partial \omega$ is indeterminate can be gained by writing out Eq. 54b and inserting the derivatives from above, which gives

    $$ \frac{\partial e}{\partial \omega}=|A|^{-1} \left(\frac{s}{\zeta}\right)^{\frac{1}{1-\psi}} \frac{k^{\frac{1-\alpha}{1-\psi}}}{1-\psi}\left( (1-\alpha)\frac{\partial \Omega}{\partial \omega}k^{-1}+\omega^{-1}\right). $$

    Hence, the ambiguity is caused by the negative effect of rising labor market participation on the capital stock ($\partial \Omega/\partial \omega<0$) and the positive counterbalancing effect of more education (ω ^− 1) due to a higher lifetime labor supply ω.

    On the contrary, the reason why the sign of $\partial k/\partial \omega$ can always be determined is that the effects of ω on k and e work into the same direction. Writing out Eq. 54a and simplifying yields

    $$ \frac{\partial k}{\partial \omega}=|A|^{-1}\left( \frac{\partial \Omega}{\partial \omega}-\frac{1}{1-\psi}\omega^{-1}e\right)<0, $$

    where $\partial \Omega/ \partial \omega<0$ captures the direct effect of more labor and the second part captures the additional effect of changing education.

• 2. In case $\varrho=\bar{\varrho}$, there is a direct (d) and an indirect effect in the partial derivatives of Ω, $\frac{\partial \Omega}{\partial X}=(\frac{\partial \Omega}{\partial X})^d+\frac{\partial \Omega}{\partial \tau}\frac{\partial \tau}{\partial X}$. Observe from Eq. 21 that

  $$ \frac{\partial \tau}{\partial s}=\frac{\gamma^N\bar{\rho} (1-\omega)}{(s(\bar{\rho}(1-\omega)+\omega)+\gamma^N)^2}>0 $$

  (55)
  $$ \frac{\partial \tau}{\partial \gamma^N}=-\frac{s\bar{\rho}(1-\omega)}{(s(\bar{\rho}(1-\omega)+\omega)+\gamma^N)^2}<0 $$

  (56)
  $$ \frac{\partial \tau}{\partial \omega}=-\frac{s\bar{\rho}(s+\gamma^N)}{(s(\bar{\rho}(1-\omega)+\omega)+\gamma^N)^2}<0 $$

  (57)

  Therefore, for given γ ^N, s, and ω, the strength of the indirect effect increases in $\bar{\varrho}$. Note that changing the adjustment rule of the social security system affects only F ₁ because there is no direct effect of τ on the education decision in steady state. Due to the additional indirect effect, it is not possible any more to determine the sign of the derivatives. We can only say whether the effects become smaller or larger, compared to the $\tau=\bar{\tau}$ case.

  • a. The difference between the two social security scenarios if γ ^N changes and τ adjusts is given by

    $$ \frac{\partial F_1}{\partial \gamma^N}=\Omega^{1/(1-\alpha)-1}\alpha\beta \left(\frac{\partial \varphi/\phi}{\partial \gamma^N} (1-\tau)-\frac{\varphi}{\phi}\frac{\partial \tau}{\partial \gamma^N}\right), $$

    (58)

    with

    $$ \frac{\partial \phi}{\partial \gamma^N} = \gamma^A \left(\alpha (2+ \hat{\rho} ) + \varphi \frac{(1-\alpha)}{\zeta} (1+ \hat{\rho})\left(\tau+ \gamma^N\frac{\partial \tau}{\partial \gamma^N}\right) \right)>0, $$

    (59)

    where the difference to the $\tau=\bar{\tau}$ scenario is only the term $\gamma^N\frac{\partial \tau}{\partial \gamma^N}$. Using Eq. 56 implies that

    $$ \left.\frac{\partial F_1}{\partial \gamma^N}\right|_{\rho=\bar{\rho}}> \left.\frac{\partial F_1}{\partial \gamma^N}\right|_{\tau=\bar{\tau}}, $$

    (60)

    which proves that

    $$ \left. \frac{\partial k}{\partial \gamma^N} \right|_{\varrho=\bar{\varrho}} > \left. \frac{\partial k}{\partial \gamma^N} \right|_{\tau=\bar{\tau}} \; \text{ and } \; \left. \frac{\partial e}{\partial \gamma^N} \right|_{\varrho=\bar{\varrho}} > \left. \frac{\partial e}{\partial \gamma^N} \right|_{\tau=\bar{\tau}}. $$

    (61)

  • b. To see how changes in the survival rate affect k and e with fixed replacement rate, we have to evaluate

    $$ \frac{\partial F_1}{\partial s}=\Omega^{1/(1-\alpha)-1}\beta\alpha \left( \frac{\partial \varphi/\phi}{\partial s}(1-\tau)-\frac{\varphi}{\phi}\frac{\partial \tau}{\partial s}\right). $$

    (62)

    The right part in the parentheses is obviously negative. To obtain the total effect, we have to evaluate $\frac{\partial (\varphi/\phi)}{\partial s}$. Since φ does not vary with τ, there is no indirect effect. Thus, we again only have to evaluate the change in ϕ, including now the change in the contribution rate τ. Again, differentiating Eq. 27a with respect to s gives

    $$ \frac{\partial \phi}{\partial s} \frac{1}{\gamma_A}=\omega \alpha \frac{1+g(e)}{1-e}-\frac{\gamma^N}{s^2\beta}\left[1-(1-\alpha)\frac{}{}(1-\tau)\right] +(1-\alpha)\frac{\gamma^N}{s\beta}\frac{\partial \tau}{\partial s}< 0, $$

    where we see that the derivative is identical to the case with $\tau=\bar{\tau}$, except for the last positive term. Using Eq. 52 and knowing that $\partial \phi / \partial s$ evaluated with the indirect effect is larger (smaller in absolute value) gives

    $$ \left. \frac{\partial \varphi/\phi}{\partial s}\right|_{\rho=\bar{\rho}} < \left. \frac{\partial \varphi/\phi}{\partial s}\right|_{\tau=\bar{\tau}}\quad \Rightarrow \quad \left.\frac{\partial F_1}{\partial s}\right|_{\rho=\bar{\rho}} < \left.\frac{\partial F_1}{\partial s}\right|_{\tau=\bar{\tau}}, $$

    (63)

    which implies that

    $$ \left. \frac{\partial k}{\partial s} \right|_{\varrho=\bar{\varrho}} < \left. \frac{\partial k}{\partial s} \right|_{\tau=\bar{\tau}} \; \text{ and } \; \left. \frac{\partial e}{\partial s} \right|_{\varrho=\bar{\varrho}} < \left. \frac{\partial e}{\partial s} \right|_{\tau=\bar{\tau}}. $$

    (64)

  • c. Differences between the two social security scenarios if ω changes are given by

    $$ \frac{\partial F_1}{\partial \omega}=\Omega^{1/(1-\alpha)-1}\beta\alpha \left( \frac{\partial \varphi/\phi}{\partial \omega}(1-\tau)-\frac{\varphi}{\phi}\frac{\partial \tau}{\partial \omega}\right). $$

    (65)

    Differentiating Eq. 27a with respect to ω gives

    $$\begin{array}{lll} \frac{\partial \phi}{\partial \omega} = \gamma^A \left( \varphi \frac{(1-\alpha)}{\zeta} (1+ \hat{\rho}) \gamma^N\frac{\partial \tau}{\partial \omega} \right. \\ \qquad \quad \qquad \quad \,\; \left. + s \left( \alpha (2+ \hat{\rho} ) + \varphi \frac{1-\alpha}{\zeta} (1+ \hat{\rho} )\right) \frac{1+g(e)}{1-e} \right), \end{array}$$

    (66)

    where the difference is only the adjusting contribution rate $\frac{\partial \tau}{\partial \omega}$. Using Eq. 57, it holds that

    $$ \left.\frac{\partial F_1}{\partial \omega}\right|_{\rho=\bar{\rho}}> \left.\frac{\partial F_1}{\partial \omega}\right|_{\tau=\bar{\tau}} $$

    (67)

    proving that

    $$ \left. \frac{\partial k}{\partial \omega} \right|_{\varrho=\bar{\varrho}} > \left. \frac{\partial k}{\partial \omega} \right|_{\tau=\bar{\tau}} \; \text{ and } \; \left. \frac{\partial e}{\partial \omega} \right|_{\varrho=\bar{\varrho}} > \left. \frac{\partial e}{\partial \omega} \right|_{\tau=\bar{\tau}}. $$

    (68)

□

Proof of Proposition 5

The effect of the degree of annuitization (λ) on the capital stock and education decision is given by

$$ \frac{\partial F_1}{\partial \lambda} = \frac{\partial \Omega}{\partial \lambda} > 0$$

(69a)

$$ \frac{\partial F_2}{\partial \lambda} = c \cdot s ^{\frac{1}{1-\psi}} k ^{\frac{1-\alpha}{1-\psi}}\frac{\partial \zeta^{-1}}{\partial \lambda} <0. $$

(69b)

Replacing the terms in Eq. 47 with the ones from above gives

$$ \frac{\partial k}{\partial \lambda} = - |A|^{-1} \left(\underbrace{\frac{\partial F_2}{\partial e} \frac{\partial F_1}{\partial \lambda}}_{<0} - \underbrace{\frac{\partial F_1}{\partial e} \frac{\partial F_2}{\partial \lambda}}_{>0} \right) >0$$

(70a)

$$ \frac{\partial e}{\partial \lambda} = - |A|^{-1} \left( - \underbrace{\frac{\partial F_2}{\partial k} \frac{\partial F_1}{\partial \lambda}}_{>0} + \underbrace{\frac{\partial F_1}{\partial k} \frac{\partial F_2}{\partial \lambda} }_{>0} \right) \gtrless 0 $$

(70b)

Qualitatively, changing λ has the same effects in both social security scenarios because the availability of annuity markets does not interact with the adjustment of contribution or replacement rates.□

B Numerical results: no annuity markets

This appendix presents additional numerical results of our sensitivity analysis for the case of perfect annuity markets, cf. our discussion in Subsection 3.3.2. Calibration parameters are reported in Table 2. Results for the elasticities of e with respect to s and w are shown in the corresponding Figs. 5 and 6.

Figures 1, 2, 3, 4, 5, and 6, and Tables 1, 2, and 3.

Fig. 5

Elasticity of e with respect to s: no annuity markets (a, b)

Fig. 6

Elasticity of e with respect to ω: no annuity markets (a, b)