On Gravity Inversion by No-Topography and Rigorous Isostatic Gravity Anomalies

Abstract

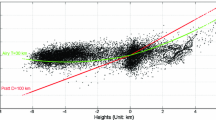
We discuss some theoretical aspects and practical consequences of using traditional versus “new”/rigorous formulations of the Bouguer and isostatic gravity anomalies/disturbances. In principle, the differences between these two concepts are in the definition of the so-called secondary indirect topographic effect (SITE) on the gravity data. Although we follow the tradition to call this effect SITE, we show that it is formally a direct topographic effect (DITE), needed to remove all topographic signal, but in practice not regarded as such. Consequently, there is a need for a no-topography gravity anomaly, which removes all topographic effects, leaving the below-crust Earth transparent for gravity inversion. Similarly, a rigorous isostatic gravity anomaly includes also a compensation effect for the SITE. By using a simple topographic model, we confirm a theoretically found ratio of 2/(n + 1) between the magnitudes of the SITE and DITE by wavelength (spherical harmonic degree n), both for the Bouguer and isostatic gravity anomalies. Finally, global gravity inversions are applied by utilizing the Vening Meinesz-Moritz isostatic model to determine the Moho geometry using the Bouguer gravity disturbances/anomalies and the no-topography gravity anomalies, and the results are compared. The numerical results confirm our theoretical findings that the Bouguer gravity disturbances and the no-topography gravity anomalies provide very similar results. A comparison of these gravimetrically computed Moho depths with the CRUST1.0 seismic model shows rms agreements of 4.3 and 4.5 km, respectively. This is a significant improvement when compared to the Moho result obtained by using the Bouguer gravity anomalies, yielding the rms difference of 7.3 km for the CRUST1.0 model. These results confirm a theoretical deficiency of the classical definition of the Bouguer and isostatic gravity anomalies, which do not take into consideration the SITE effects on the topography and its compensation.

Appendix

The detailed formulas for computing the topographic potentials and attractions of the spherical cylinder (cf. Sjöberg 2007) and its isostatic compensation by Airy–Heiskanen’s compensation model.

The topographic potential V ^T _P at any point P of radius r _P along its axis is given by

$$ V^{T} \left( P \right) = 2\pi \mu \int\limits_{0}^{{\psi_{0} }} {\int\limits_{R}^{{r_{s} }} {\frac{{r^{2} {\text{d}}r\sin \psi d\psi }}{{l_{P0}^{{}} }}} } = \frac{2\pi \mu }{{r_{P} }}\int\limits_{R}^{{r_{s} }} {\left( {l_{P0}^{{}} - \left| {r_{P} - r} \right|} \right)} {\text{rdr}}, $$

(14)

where $\mu = G\rho$, $l_{P0} = \sqrt {r_{P}^{2} + r^{2} - 2r_{P} r\cos \psi_{0} }$. Eq. (14) can be integrated to

$$ \begin{aligned} V^{T} \left( P \right) & = \frac{2\pi \mu }{{r_{P} }}\left( {I\left( {r_{P} ,R,r_{S} } \right) - r_{P} \frac{{r_{s}^{2} - R^{2} }}{2} + \frac{{r_{s}^{3} - R^{3} }}{3}} \right) \\ & {\text{if}}\;r_{P} \ge r_{s} , \\ \end{aligned} $$

(15)

where

$$ \begin{gathered} I\left( {r_{P} ,R,r_{S} } \right) = \int\limits_{R}^{{r_{s} }} {l_{P0} r{\text{d}}r} \hfill \\ { = }\left[ {\frac{{l_{P0}^{3} }}{3} + r_{P} t_{0} \left\{ {\frac{{r - r_{P} t_{0} }}{2}} \right.} \right.l_{P0} + \frac{{r_{P}^{2} }}{2} \hfill \\ \, \left. {\left. { \times \left( {1 - t_{0}^{2} } \right)\ln 2\left( {r - r_{P} t_{0} + l_{P0} } \right)} \right\}} \right]_{r = R}^{{r = r_{s} }} . \hfill \\ \end{gathered} $$

(16)

Generally, the topographic gravity anomaly and gravity disturbance effects become

$$ \Delta g^{T} = \delta g^{T} + 2\frac{{V^{T} }}{{r_{P} }}\,\,{\text{and}}\,\,\,\delta g^{T} = - A^{T} = \frac{{\partial V^{T} }}{{\partial r_{P} }}. $$

(17)

For the spherical cylinder one obtains

$$ A^{T} = - \delta g^{T} \, = \frac{2\pi \mu }{{r_{P}^{2} }}\left( {I\left( {r_{P} ,R,r_{S} } \right) - r_{P} I^{{\prime }} \left( {r_{P} ,R,r_{S} } \right) + \frac{{r_{s}^{3} - R^{3} }}{3}} \right), $$

(18)

where

$$ I^{{\prime }} \left( {r_{P} ,R,r_{S} } \right) = J_{1} \left( {r_{P} ,R,r_{S} } \right) - J_{2} \left( {r_{P} ,R,r_{S} } \right) $$

(19)

with

$$ J_{1} \left( {r_{P} ,R,r_{S} } \right) = r_{P} \int\limits_{R}^{{r_{s} }} {\frac{r}{{l_{P0} }}} {\text{d}}r = \left[ {l_{P0} + r_{P} t_{0} \ln \left| {2r - 2r_{P} t_{0} + 2l_{p0} } \right|} \right]_{r = R}^{{r = r_{S} }} $$

(20)

and

$$ J_{2} \left( {r_{P} ,R,r_{S} } \right) = - t_{0} \int\limits_{R}^{{r_{s} }} {\frac{{r^{2} }}{{l_{P0} }}} {\text{d}}r = \left[ {\frac{{r + 3r_{P} t_{0} }}{2}l_{P0} + \frac{{3r_{P}^{2} t_{0}^{2} - r_{P}^{2} }}{2}\ln \left| { - 2r_{P} t_{0} + 2r - 2l_{P0} } \right|} \right]_{r = R}^{{r = r_{S} }}. $$

(21)

The compensation potential for Airy–Heiskanen’s isostatic model applied to the spherical cylinder is given by the integral

$$ V_{C} \left( P \right) = 2\pi G\Delta \rho \int\limits_{0}^{{\psi_{0} }} {\int\limits_{R - (T + t)}^{R - T} {\frac{{r^{2} {\text{d}}r\sin \psi {\text{d}}\psi }}{{l_{P0}^{{}} }}} }, $$

(22)

where t = 4.45H, where H is the topographic height (cf. Heiskanen and Moritz 1967, pp. 135–136), and Δρ is the density contrast between the crust and mantle. The integral can be evaluated to

$$ \begin{aligned} V_{C} \left( P \right) = \frac{2\pi G\Delta \rho }{{r_{P} }}\left( {I\left( {r_{P} ,R - T - t,R - T} \right) - r_{P} \frac{{\left( {R - T} \right)^{2} - \left( {R - (T + t)} \right)^{2} }}{2} + \frac{{\left( {R - T} \right)^{3} - \left( {R - T - t} \right)^{3} }}{3}} \right) \\ & {\text{if}}\,\,r_{P} \ge r_{s} , \\ \end{aligned} $$

(23)

and the compensation attraction becomes

$$ \begin{gathered} A_{C} = - \frac{{\partial V_{C} }}{{\partial r_{P} }} \hfill \\ \, = \frac{2\pi G\Delta \rho }{{r_{P}^{2} }}\left( {I\left( {r_{P} ,R - T - r_{S} ,R - T} \right) - r_{P} I^{{\prime }} \left( {r_{P} ,R - T - r_{S} ,R - T} \right) + \frac{{\left( {R - T} \right)_{{}}^{3} - \left( {R - T - t} \right)^{3} }}{3}} \right). \hfill \\ \end{gathered} $$

(24)