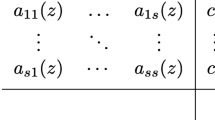Skip to main content
SpringerLink
Log in

High order explicit methods for parabolic equations

  • Published:
BIT Numerical Mathematics Aims and scope Submit manuscript

Abstract

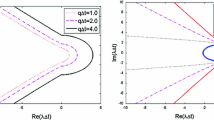
This paper discusses explicit embedded integration methods with large stability domains of order 3 and 4. The high order produces accurate results, the large stability domains allow some reasonable stiffness, the explicitness enables the method to treat very large problems, often space discretization of parabolic PDEs, and the embedded formulas permit an efficient stepsize control. The construction of these methods is achieved in two steps: firstly we compute stability polynomials of a given order with optimal stability domains, i.e., possessing a Chebyshev alternation; secondly we realize a corresponding explicit Runge-Kutta method with the help of the theory of composition methods.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. S. N. Bernstein,Leçons sur les propriétés extrémales et la meilleure approximation des fonctions analytiques d'une variable réelle, in Professées á la Sorbonne, Paris, 1926.

  2. E. Hairer, S. P. Nørsett, and G. Wanner,Solving Ordinary Differential Equations I. Nonstiff Problems, 2nd ed., Springer-Verlag, Berlin, 1993.

    MATH  Google Scholar 

  3. E. Hairer and G. Wanner,Solving Ordinary Differential Equations II. Stiff and Differential-Algebraic Problems, 2nd ed., Springer-Verlag, Berlin, 1996.

    MATH  Google Scholar 

  4. P. J. van der Houwen and J. Kok,Numerical solution of a maximal problem, Report TW 124/71, Mathematical Center, Amsterdam, 1971.

    Google Scholar 

  5. P. J. van der Houwen,Construction of Integration Formulas for Initial Value Problems, North-Holland, Amsterdam, 1977.

    MATH  Google Scholar 

  6. P. J. van der Houwen and B. P. Sommeijer,On the internal stability of explicit m-stage Runge-Kutta methods for large m-values, Z. Angew. Math. Mech., 60 (1980), pp. 479–485.

    MATH  MathSciNet  Google Scholar 

  7. V. I. Lebedev and A. A. Medovikov,Methods of second order accuracy with variable time steps, Izvestiya Vuzov, Matematika, N10, Russia, 1995.

    Google Scholar 

  8. V. I. Lebedev,How to solve stiff systems of differential equations by explicit methods, in Numerical Methods and Applications, CRC Press, Boca Raton, 1994, pp. 45–80.

    Google Scholar 

  9. V. I. Lebedev,Zolotarev polynomials and extremum problem, Russ. J. Numer. Anal. Math. Modelling. V. 9, N 3 (1994), pp. 191–314.

    Google Scholar 

  10. V. I. Lebedev,A new method for determing the roots of polynomials of least deviation on a segment with weight and subject to additional conditions. Part I, Part II, Russ. J. Numer. Anal. Math. Modelling. V. 8, N 3 (1993), pp. 195–222; V. 8, N 5 (1993), pp. 397–426.

    Article  MATH  Google Scholar 

  11. V. I. Lebedev,Explicit difference schemes with time-variable steps for the solution of stiff system of equations, Preprint DNM AS USSR N177, 1987.

  12. H. Lomax,On the construction of highly stable, explicit numerical methods for integrating coupled ordinary differential equations with parasitic eigenvalues, NASA Technical Note NASATND/4547, 1968.

  13. A. A. Markov,Lectures on the functions of least deviation, 1892; in Selected Works, OGIZ, Moscow, 1948, pp. 244–291, (in Russian).

    Google Scholar 

  14. A. A. Medovikov,Explicit and explicit-implicit methods of solution of unstationary problems, PhD Thesis, INM, Moscow, 1992.

    Google Scholar 

  15. C. L. Metzger,Méthodes de Runge-Kutta de rang supérieur à l'orde, Thèse (troisième cycle), Université de Grenoble, France, 1967.

    Google Scholar 

  16. W. Riha,Optimal stability polynomials, Computing, 9 (1972), pp. 37–43.

    Article  MATH  MathSciNet  Google Scholar 

  17. G. Szegö,Orthogonal Polynomials, Amer. Math. Soc., New York, 1949.

    Google Scholar 

  18. P. L. Chebyshev,On the problems about minimal values connected with appropriate representation of functions, Mem. Acad. St. Petersburg (1859); Collected Works, Vol. 2, GITTL, Moscow-Leningrad, 1947 (in Russian); Oeuvres, Vol. I, p. 271.

  19. P. L. Chebyshev,On the functions slightly deviating from zero for certain values of the variables, Prilojenie k XI tomy Zapisok Imperatorskoi Akademii nauk N3 (1881);Collected Works, Vol. 3, GITTL, Moscow-Leningrad, 1948, p. 108; Oeuvres, Vol. II, p. 373 (in Russian).

  20. J. G. Verwer,A class of stabilized three-step Runge-Kutta methods for the numerical integration of parabolic equations, J. Comput. Appl. Math., 3 (1977), pp. 155–166.

    Article  MATH  MathSciNet  Google Scholar 

  21. J. G. Verwer,Explicit Runge-Kutta methods for parabolic partial differential equations, 1996. To appear in the Runge-Kutta centennial special issue of Appl. Numer. Math.

  22. E. I. Zolotarev,On applying elliptic functions to the study of functions of least and greatest deviation from zero, Zapiski Sankt-Peterburgskoi Akademii Nauk XXX, No 5 (1887), pp. 1–59 (in Russian).

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute of Numerical Mathematics, Gubkina street 8, 117333, Moscow, Russia

    Alexei A. Medovikov

Authors
  1. Alexei A. Medovikov
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

Communicated by Syvert P. Nørsett.

This work was supported by the Russian Fund Fundamental Researches and the Swiss National Science Foundation 20-43.314.95.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Medovikov, A.A. High order explicit methods for parabolic equations. Bit Numer Math 38, 372–390 (1998). https://doi.org/10.1007/BF02512373

Download citation

  • Received:

  • Revised:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF02512373

AMS subject classification

Key words

Search

Navigation