Abstract
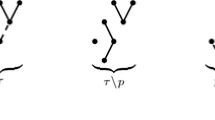
Runge-Kutta methods are formulated via coordinate independent operations on manifolds. It is shown that there is an intimate connection between Lie series and Lie groups on one hand and Butcher's celebrated theory of order conditions on the other. In Butcher's theory the elementary differentials are represented as trees. In the present formulation they appear as commutators between vector fields. This leads to a theory for the order conditions, which can be developed in a completely coordinate free manner. Although this theory is developed in a language that is not widely used in applied mathematics, it is structurally simple. The recursion for the order conditions rests mainly on three lemmas, each with very short proofs. The techniques used in the analysis are prepared for studying RK-like methods on general Lie groups and homogeneous manifolds, but these themes are not studied in detail within the present paper.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
R. Abraham, J. E. Marsden and T. Ratiu,Manifolds, Tensor Analysis, and Applications, Springer-Verlag, 1980.
P. Albrecht,The extension of the theory of A-methods to RK-methods, in Numerical Treatment of Differential Equations, in Proceedings 4th Seminar NUMDIFF-4, ed. K. Strehmel, Teubner-Texte zur Mathematik, Leipzig, (1987), pp. 8–18.
R. L. Bryant,An Introduction to Lie Groups and Symplectic Geometry, in Geometry and Quantum field theory, Eds. Freed and Uhlenbeck, AMS IAS/Parc City math. series vol. 1, (1995), pp. 1–182.
J. C. Butcher,Coefficients for the study of Runge-Kutta integration processes, J. Austral. Math. Soc., 3 (1963), pp. 185–201.
J. C. Butcher,An algebraic theory of integration methods, Math. Comput. 26 (1972), pp. 79–106.
P. E. Crouch and R. Grossman,Numerical integration of ordinary differential equations on manifolds, J. Nonlinear Sci., 3 (1993), pp. 1–33.
E. Hairer, S. P. Nørsett and G. Wanner,Solving Ordinary Differential Equations I, Nonstiff Problems, Springer-Verlag, Berlin, 1987.
E. Hairer and G. Wanner,On the Butcher group and general multi-value methods, Computing, 13 (1974), pp. 1–15.
S. Helgason,Differential Geometry, Lie Groups and Symmetric Spaces, Academic Press, New York, 1978.
M. Haveraaen, V. Madsen and H. Munthe-Kaas,Algebraic programming technology for partial differential equations, in Proceedings of Norsk Informatikk Konferanse (NIK), Ed. A. Maus, Tapir Trondheim Norway, (1992), pp. 55–68.
H. Munthe-Kaas and M. Haveraaen,Coordinate free numerics I; How to avoid index wrestling in tensor computations, Report no. 101, Department of Informatics, University of Bergen, Norway (1994).
P. J. Olver,Applications of Lie Groups to Differential Equations, Springer-Verlag GTM no. 107, New York, 1986.
J. M. Sanz-Serna and M. P. Calvo,Numerical Hamiltonian Problems, Chapman & Hall, London, 1994.
J. C. Simo and K. K. Wong,Unconditionally stable algorithms for rigid body dynamics that exactly preserve energy and momentum, Internat. J. Numer. Methods in Engrg., 31 (1989), pp. 19–52.
V. S. Varadarajan,Lie Groups, Lie Algebras and Their Representations, Springer-Verlag GTM no. 102, New York, 1984.
F. M. Warner,Foundations of Differential Manifolds and Lie Groups, Springer-Verlag GTM no. 94, 1983.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Munthe-Kaas, H. Lie-Butcher theory for Runge-Kutta methods. Bit Numer Math 35, 572–587 (1995). https://doi.org/10.1007/BF01739828
Received:
Revised:
Issue Date:
DOI: https://doi.org/10.1007/BF01739828