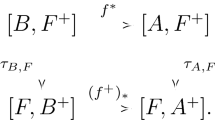Skip to main content
SpringerLink
Log in

Pic is a contracted functor

  • Published:
Inventiones mathematicae Aims and scope

Summary

We show that there is a natural decomposition

$$Pic(A[t,t^{ - 1} ]) \cong Pic(A) \oplus NPic(A) \oplus NPic(A) \oplus H^1 (A)$$

for any commutative ringA, where Pic(A) is the Picard group of invertibleA-modules, andH 1 (A) is the étale cohomology groupH 1 (Spec(A),ℤ). A similar decomposition of Pic(X[t, t −1]) holds for any schemeX. This makes Pic a “contracted functor” in the sense of Bass.H 1 (A) is always a torsionfree group, and is zero ifA is normal. For pseudo-geometric rings,H 1 (A) is an effectively computable, finitely generated free abelian group. We also show thatH 1 (A[t, t −1])≊H 1 (A), i.e.,NH 1=LH 1=0. This yields the formula for group rings:

$$Pic(A[t_1 ,t_1^{ - 1} ,...,t_m ,t_m^{ - 1} ) \cong Pic(A) \oplus \coprod\limits_{i = 1}^m {H^1 (A) \oplus \coprod\limits_{k = 1}^m {\coprod\limits_{i = 1}^{2^k \left( {_k^m } \right)} {N^k Pic(A).} } }$$

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • [Artin] Artin, M.: Grothendieck Topologies. Harvard Seminar Notes, 1962

  • [And] Anderson, D.F.: The Picard group of a monoid domain. J. Algebra115, 342–351 (1988)

    Google Scholar 

  • [Bass] Bass, H.: AlgebraicK-theory. Benjamin, New York, 1968

    Google Scholar 

  • [BLR] Bhatwadekar, S., Lindel, H., Rao, R.: The Bass-Murthy question: Serre dimension of Laurent polynomial extensions. Invent. Math.81, 189–203 (1985)

    Google Scholar 

  • [BM] Bass, H., Murthy, M.P.: Grothendieck groups and Picard groups of abelian group rings. Ann. Math.86, 16–73 (1967)

    Google Scholar 

  • [Bour] Bourbaki, N.: Algèbre Commutative, ch. 7, Diviseurs. Hermann, Paris, 1965

    Google Scholar 

  • [DW] Dayton, B., Weibel, C.: On the naturality of Pic,SK 0 andSK 1. AlgebraicK-theory: Connections with Geometry and Topology. NATO ARI Series C, Kluwer Press, 1989

  • [EGA] Grothendieck, A., Dieudonné, J.: Eléments de Géométrie Algébrique. Publ. Math. I.H.E.S. Part I: 4 (1960); Part II: 8 (1961); Part IV: 20 (1964), 24 (1965), 28 (1966), 32 (1967), Part I (revised version), Springer, Berlin Heidelberg New York, 1971

    Google Scholar 

  • [Fuchs] Fuchs, L.: Infinite Abelian Groups. Vol. II, Academic Press, New York, 1973

    Google Scholar 

  • [G] Greco, S.: Seminormality and quasinormality of group rings. J. Pure Appl. Algebra18, 129–142 (1980)

    Google Scholar 

  • [Isch] Ischebeck, F.: Zwei Bemerkungen über seminormale Ringe. Math. Z.152, 101–106 (1977)

    Google Scholar 

  • [Kap] Kaplansky, I.: Projective Modules. Ann. Math.68, 372–377 (1958)

    Google Scholar 

  • [Kr] Kratzer, C.: λ-structure enK-théorie algébrique. Comm. Math. Helv.55, 233–254 (1980)

    Google Scholar 

  • [KS] Kato, K., Saito, S.: Global class theory of arithmetic schemes. AMS Contemp. Math.55 (Part I), 255–331 (1986)

    Google Scholar 

  • [Lam] Lam, T.Y.: Serre's Conjecture. (Lect. Notes Math. Vol. 635) Springer, Berlin Heidelberg New York, 1978

    Google Scholar 

  • [Lantz] Lantz, D.: On the Picard group of an abelian group ring, Group and Semigroup Rings. N. Holland Math. Studies Vol. 126, Amsterdam, 1986

  • [Milne] Milne, J.: Étale Cohomology. Princeton University Press, Princeton, 1980

    Google Scholar 

  • [N] Nagata, M.: Local Rings. Wiley and Sons, New York, 1962

    Google Scholar 

  • [Nis] Nisnevich, Y.: Adeles and Grothendieck topologies (Preprint) 1982

  • [Nis2] Nisnevich, Y.: The completely decomposed topology on schemes and associated descent spectral sequences in algebraicK-theory. AlgebraicK-theory: Connections with Geometry and Topology. NATO ARI Series C, Kluwer Press, 1989

  • [No] Nöbeling, G.: Verallgemeinerung eines Satzes von Herrn E. Specker. Invent. Math.6, 41–55 (1968)

    Google Scholar 

  • [Or] Orecchia, F.: Sui gruppi delle unità et i gruppi di Picard relativi a una varietà affine, ridotta e alla sua normalizzata. Boll. U.M.I.14B, 786–809 (1977)

    Google Scholar 

  • [OSY] Onoda, N., Sugatani, T., Yoshida, K.: Local quasinormality and closedness type criteria. Houston J. Math.11, 247–256 (1985)

    Google Scholar 

  • [OY] Onoda, N., Yoshida, K.: Remarks on quasinormal rings. J. Pure Appl. Alg.33, 59–67 (1984)

    Google Scholar 

  • [P] Pedrini, C.: On theK 0 of certain polynomial extensions (Lect. Notes Math., Vol. 342) Springer, Berlin Heidelberg New York, 1973

    Google Scholar 

  • [Pierce] Pierce, R.S.: Modules over commutative regular rings. Memoirs AMS, No. 70, Providence, 1967

  • [Q] Quillen, D.: Higher algebraicK-theory: I. (Lect. Notes Math., Vol. 341) Springer, Berlin Heidelberg New York, 1973

    Google Scholar 

  • [Q76] Quillen, D.: Projective modules over polynomial rings. Invent. Math.36, 167–171 (1976)

    Google Scholar 

  • [Rush] Rush, D.: Picard groups in abelian group rings. J. Pure Appl. Alg.26, 101–114 (1982)

    Google Scholar 

  • [Reid] Reid, L.:N-dimensional rings with an isolated singular point having non-zeroK −N .K-theory1, 197–206 (1987)

    Google Scholar 

  • [SGA1] Grothendieck, A.: Revêtements étales et groupe fondamental. (Lect. Notes Math., Vol. 224) Springer, Berlin Heidelberg New York, 1971

    Google Scholar 

  • [SGA6] Berthelot, P., Grothendieck, A., Illusie, L.: Théorie des intersections et théorème de Riemann-Roch. (Lect. Notes Math., Vol. 225) Springer, Berlin Heidelberg New York, 1971

    Google Scholar 

  • [Sou] Soulé, C.: Opérations enK-théorie algébrique. Can. J. Math.37, 488–550 (1985)

    Google Scholar 

  • [S] Stienstra, J.: Operations in the higherK-Theory of endomorphisms. CMS Conf. Proc.2 (Part I), 59–115 (1982)

    Google Scholar 

  • [Sw] Swan, R.: Projective modules over Laurent polynomial rings. Trans. AMS237, 111–120 (1978)

    Google Scholar 

  • [Swan] Swan, R.: On seminormality. J. Algebra67, 210–229 (1980)

    Google Scholar 

  • [TT] Thomason, R., Trobaugh, T.: Higher algebraicK-Theory of schemes and of derived categories, preprint, 1988, Grothendieck Festschrift (Progr. Math. series) Birkhäuser Press Basel Boston (to appear)

  • [V] Vorst, T.: Localization of theK-Theory of polynomial extensions. Math. Ann.244, 33–53 (1979)

    Google Scholar 

  • [vdK] Kallen, W. van der: Descent for theK-Theory of polynomial rings. Math. Z.191, 405–415 (1986)

    Google Scholar 

  • [W] Weibel, C.:K-theory and analytic isomorphisms. Invent. Math.61, 177–197 (1980)

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Mathematics Department, Rutgers University, 08903, New Brunswick, NJ, USA

    Charles A. Weibel

Authors
  1. Charles A. Weibel
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

Oblatum 1-IX-1989 & 30-V-1990

Partially supported by NSF grant DMS-8803497

Rights and permissions

Reprints and permissions

About this article

Cite this article

Weibel, C.A. Pic is a contracted functor. Invent Math 103, 351–377 (1991). https://doi.org/10.1007/BF01239518

Download citation

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF01239518

Keywords

Search

Navigation