Abstract
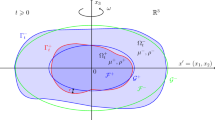
Incompressible, inviscid, free boundary fluid flows are Hamiltonian systems, i.e., the evolution of the flow is determined by a Poisson bracket and a Hamiltonian function. The Poisson structure for such flows generalizes a previous structure of Zakharov for irrotational free boundary flow and a structure of Arnold for flow of an incompressible fluid in a region with fixed boundary. The Poisson bracket is determined by reduction from canonical variables in the Lagrangian (material) description.
The Hamiltonian structure of the flow is used in the “Energy-Casimir” method to demonstrate formal stability of a rigidly rotating circular drop. That method involves the determination of an appropriate combination of the Hamiltonian and conserved quantities associated to the symmetries of the system. This combination is chosen such as to make the equilibrium in question a critical point of the combined function whose second variation is then tested for positive (or negative) definiteness at that point.
One of our main results is that a formally stable relative equilibrium of the equations of motion of a planar drop with a free boundary with surface tension and self-gravitation or charge is (conditionally) nonlinearly stable. Here conditional simply means that the result is conditional on the assumption of existence of solutions in the function spaces we construct for the stability analysis. The proof of this stability theorem is based on techniques from the classical calculus of varialions. A careful Taylor series analysis shows that an equilibrium with positive-definite second variation is a strong minimum of the functional. This conclusion about the minimum, which is applicable to a large class of variational problems, is used in this paper to obtain bounds on the variation of the Hamiltonian for the rotating liquid drop. The existence of these bounds implies that the equilibrium is conditionally nonlinearly stable.
Similar content being viewed by others
References
Abarbanel, H., Brown, R., & Yang, Y. (1987) Hamiltonian formulation of inviscid flows with free boundaries. (preprint)
Abraham, R., & Marsden, J. E. (1978) Foundations of Mechanics, second edition. Benjamin/Cummings Publishing Company.
Adams, R. A. (1975) Sobolev spaces. Academic Press.
Arnold, V. I. (1966a) Sur un principe variationnel pour les écoulements stationnaires des liquides parfaits et ses applications aux problèmes de stabilité non linéaires. J. Mécanique 5, 29–43.
Arnold, V. I. (1966b) An a priori estimate in the theory of hydrodynamic stability. Izv. Vyssh. Uchebn. Z. Math. 54, 3–5. (English translation: Transl. AMS 19 (1969), 267—269.)
Arnold, V. I. (1978) Mathematical methods of classical mechanics. Springer-Verlag.
Ball, J. M., Knops, R. J., & Marsden, J. E. (1978) Two examples in nonlinear elasticity. Springer Lecture Notes in Mathematics 466, 41–49.
Ball, J. M., & Marsden, J. E. (1984) Quasiconvexity at the boundary, positivity of the second variation and elastic stability. Arch. Rational Mech. An. 86, 251–277.
Benjamin, T. B. (1972) The stability of solitary waves. Proc. Roy. Soc. London 328 A, 153–183.
Benjamin, T. B. (1987) Hamiltonian theory for motions of bubbles in an infinite liquid. J. Fluid Mech. 181, 349–397.
Benjamin, T. B., & Olver, P. J. (1982) Hamiltonian structure, symmetries and conservation laws for water waves. J. Fluid Mech. 125, 137–185.
Bolza, O. (1960) Lectures on the calculus of variations, 2nd ed., Chelsea Publishing Company.
Brown, R. A., & Scriven, L. E. (1980) The shape and stability of rotating liquid drops. Proc. R. Soc. London 371 A, 331–357.
Chandrasekhar, S. (1965) The stability of a rotating liquid drop. Proc. R. Soc. London 284 A, 1–26.
Chandrasekhar, S. (1969) Ellipsoidal figures of equilibrium. Yale University Press.
Chorin, A. J., & Marsden, J. E. (1979) A mathematical introduction to fluid mechanics. Springer Universitext.
Deem, G. S., & Zabusky, N. J. (1978) Vortex waves: Stationary ‘V-states’, interactions, recurrence, and breaking. Phys. Rev. Lett. 40, 859–862.
Hestenes, M. (1980) Calculus of variations and optimal control theory, second edition. Robert E. Krieger Publishing Company.
Holm, D., Marsden, J., Ratiu, T., & Weinstein, A. (1985) Nonlinear stability of fluid and plasma equilibria. Physics Reports 123 (1 & 2). 1–116. See also Physics Lett. 98 A (1983), 15–21.
Joseph, D. D. (1976) Stability of Fluid Motions I. Springer-Verlag.
Lawson, H. B. (1980) Lectures on minimal surfaces. Publish or Perish, Inc.
Lewis, D. (1987) Rotating liquid drops: Hamiltonian structure, stability and bifurcation. Ph.D. thesis, University of California, Berkeley.
Lewis, D., Marsden, J., Montgomery, R., & Ratiu, T. (1986) The Hamiltonian structure for dynamic free boundary problems. Physica D. 18, 391–404.
Lewis, D., Marsden, J., & Ratiu, T. (1986) Formal stability of liquid drops with surface tension. Proceedings of Conference on New Perspectives in Nonlinear Dynamics ed. M. Schlesinger, R. Cawley, A. Saenz & W. Zachary. World Scientific Pub. 71–83.
Marsden, J. E., & Ratiu, T. (1988) Symmetry and Mechanics (to appear).
Marsden, J. E., & Weinstein, A. (1974) Reduction of symplectic manifolds with symmetry. Rep. Math. Phys. 5, 121–130.
Marsden, J. E., & Weinstein, A. (1983) Coadjoint orbits, vortices and Clebsch variables for incompressible fluids. Physica D. 7, 305–323.
Mazer, A., & Ratiu, T. (1987a) A Hamiltonian formulation of adiabatic free boundary Euler flows (preprint).
Mazer, A., & Ratiu, T. (1987b) Formal stability of two-dimensional self-gravitating rotating disks (preprint).
Miles, J. W. (1981) Hamiltonian formulations for surface waves. Appl. Sci. Res. 37, 153–168.
Montgomery, R. (1986) The bundle picture in mechanics. Ph.D. thesis, University of California, Berkeley.
Montgomery, R., Marsden, J., & Ratiu, T. (1984) Gauged Lie-Poisson structures. Cont. Math. AMS 28, 101–114.
Rudin, W. (1987) Real and Complex Analysis, third edition. McGraw-Hill.
Swiatecki, W. J. (1974) The rotating, charged or gravitating liquid drop and problems in nuclear physics and astronomy. In Proceedings of the International Colloquium on Drops and Bubbles (ed. D. J. Collins, M. S. Plesset & M. M. Saffren) 52–78.
Vogel, T. I. (1987) Weak conditions for capillary surfaces (preprint).
Wan, Y. H., & Pulvirenti, F. (1985) The nonlinear stability of circular vortex patches. Comm. Math. Phys. 99, 435–450.
Wang, T. G., Trinh, E. H., Croonquist, A. P., & Elleman, D. D. (1986) Shapes of rotating free drops: Spacelab experimental results. Phys. Rev. Lett. 56, 452–455.
Zakharov, V. E. (1968) Stability of periodic waves of finite amplitude on the surface of a deep fluid. J. Prikl. Mekh. Tekhn. Fiziki 9, 86–94. (Received April 5, 1988)
Author information
Authors and Affiliations
Additional information
Communicated by P. Holmes
Rights and permissions
About this article
Cite this article
Lewis, D. Nonlinear stability of a rotating planar liquid drop. Arch. Rational Mech. Anal. 106, 287–333 (1989). https://doi.org/10.1007/BF00281351
Received:
Issue Date:
DOI: https://doi.org/10.1007/BF00281351