Exploring an Interface Model for CKA

Abstract

Concurrent Kleene Algebras (CKAs) serve to describe general concurrent systems in a unified way at an abstract algebraic level. Recently, a graph-based model for CKA has been defined in which the incoming and outgoing edges of a graph define its input/output interface. The present paper provides a simplification and a significant extension of the original model to cover notions of states, predicates and assertions in the vein of algebraic treatments using modal semirings. Moreover, it uses the extension to set up a variant of the temporal logic $\mathsf {CTL}^*$ for the interface model.

Appendix: Deferred Proofs

Proof of Lemma 3.5.

We only show Part 1, since Part 2 is analogous. First,

We simplify the first two summands; the other two are analogous. For summand number one we have

For summand number two we have

Altogether, the claim is shown.    $\square$

Proof of Theorem 3.6.

First we observe that $(\mathsf {G},|)$ is an aggregation algebra in the sense of [15] and $\mathrm{CS}$ and $\mathrm{CC}(G,G') {\,=_{ df}\, }\mathsf{TRUE}$ can be viewed as independence relations with $\mathrm{CS}\subseteq \mathrm{CC}$. With this, the claim follows from Lemmas 3.4 and 3.5 and Proposition 3.6 of [15] if we can show that the restricting predicates $\mathrm{CS}$ and $\mathrm{CC}$ are bilinear, i.e., satisfy.

$$\begin{aligned} \mathrm{CS}(G\,|\,G', G'')&\,\Longleftrightarrow \, \mathrm{CS}(G,G'') \ \,\wedge \, \mathrm{CS}(G',G''),\nonumber \\ \mathrm{CS}(G, G'\,|\,G'')&\,\Longleftrightarrow \, \mathrm{CS}(G,G') \ \ \,\wedge \, \mathrm{CS}(G,G'') \end{aligned}$$

(11)

and the analogous property for $\mathrm{CC}$, and $\mathrm{CC}$ is symmetric. For $\mathrm{CC}$ both claims are trivial. To show (11), we exploit that the definition of $\mathrm{CS}$ is symmetric in both arguments, so that it suffices to consider the first argument.

Proof of Lemma 3.18.

By the definitions of; and their result, and its interfaces, coincides with that of parallel composition whenever it is defined.

We start by showing the first equation. By the above remark and Lemma 3.5,

For the second summand we calculate

Concerning the first summand we have

Therefore the first summand reduces to ${ in}(G)$, as required.

The equation ${ in}(G) = { in}(G \!\downharpoonright \!{ in}(G'))$ is immediate, since the definition of $\downharpoonright$ and ${ in}(G') = { out}(G)$ entail $G \!\downharpoonright \!{ in}(G') = G$.

The equations for ${ out}$ are proved completely symmetrically.

Now we can show associativity of  . Assume graphlets $G,G,G''$. If any of them is $\Box$ then the associativity equation is immediate from the definition of  . Otherwise we only need to check the case where ${ in}(G') = { out}(G)$ and ${ in}(G'') = { out}(G')$ so that both and are defined. By the just proved equations for ${ in}$ and ${ out}$ then also and , so that also and are defined; by definition of and associativity of ; they coincide.

Next, by the definition of the restriction operators, $G \!\downharpoonright \!C$ and $C \!\downharpoonleft \!G'$ are defined iff ${ out}(G) \subseteq C$ and ${ in}(G') \subseteq C$. In that case $G \!\downharpoonright \!C = G$ and $C \!\downharpoonleft \!G' = G'$ and therefore

Finally, the last two claims are immediate from the definition of restriction and the first two claims.    $\square$

Proof of Lemma 3.24.

We only show the first equation, since the second is analogous. By the definition of $\mathbin {|||}$ and Lemma 3.5 we have

$$\begin{aligned} { in}(G\,|\,G') \,=\, ({ in}(G) \cap \overline{E'} \mathbin {\!\times \!}E) \,\cup \, ({ in}(G') \cap \overline{E} \mathbin {\!\times \!}E'). \end{aligned}$$

The first summand reduces to ${ in}(G)$ if we can show ${ in}(G) \subseteq \overline{E'} \mathbin {\!\times \!}E$, equivalently, ${ in}(G) \cap \overline{\overline{E'} \mathbin {\!\times \!}E} = \emptyset$. We calculate,

Symmetrically, the second summand reduces to ${ in}(G')$.    $\square$

Proof of Theorem 4.6.

• 1.

  

• 2. We show by induction on $i$ that $\bigcap \limits _{j \le i} \mathsf {X}^j \cdot {[\![\psi ]\!]} = (S \!\downharpoonleft \!\mathsf {X})^i \cdot {[\![\psi ]\!]}$. The base case $i=0$ is trivial. The induction step proceeds as follows:

  

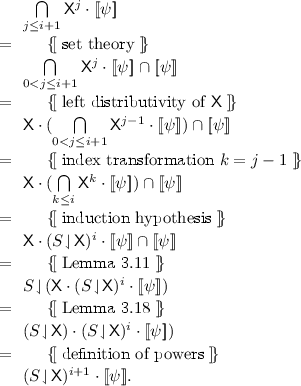
  By this,

  

  An easy induction shows $(S \!\downharpoonleft \!\mathsf {X})^\omega \subseteq (S \!\downharpoonleft \!\mathsf {X})^i \cdot {[\![\psi ]\!]}$ for all $i$ and hence $(S \!\downharpoonleft \!\mathsf {X})^\omega \subseteq {[\![\mathsf {G}\psi ]\!]}$. For the reverse inclusion it suffices to show that ${[\![\mathsf {G}\psi ]\!]} \,=\, (S \!\downharpoonleft \!\mathsf {X}) \cdot {[\![\mathsf {G}\psi ]\!]}$. Indeed,

  

     $\square$