Sequential Solutions in Machine Scheduling Games

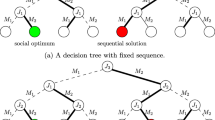
Abstract

We consider the classical machine scheduling, where n jobs need to be scheduled on m machines, and where job j scheduled on machine i contributes $p_{i,j}\in \mathbb {R}$ to the load of machine i, with the goal of minimizing the makespan, i.e., the maximum load of any machine in the schedule. We study inefficiency of schedules that are obtained when jobs arrive sequentially one by one, and the jobs choose themselves the machine on which they will be scheduled, aiming at being scheduled on a machine with small load. We measure the inefficiency of a schedule as the ratio of the makespan obtained in the worst-case equilibrium schedule, and of the optimum makespan. This ratio is known as the sequential price of anarchy (SPoA). We also introduce two alternative inefficiency measures, which allow for a favorable choice of the order in which the jobs make their decisions. As our first result, we disprove the conjecture of  [22] claiming that the sequential price of anarchy for $m=2$ machines is at most 3. We show that the sequential price of anarchy grows at least linearly with the number n of players, assuming arbitrary tie-breaking rules. That is, we show $\mathbf{SPoA} \in \varOmega (n)$. Complementing this result, we show that $\mathbf{SPoA} \in O(n)$, reducing previously known exponential bound for 2 machines. Furthermore, we show that there exists an order of the jobs, resulting in makespan that is at most linearly larger than the optimum makespan. To the end, we show that if an authority can change the order of the jobs adaptively to the decisions made by the jobs so far (but cannot influence the decisions of the jobs), then there exists an adaptive ordering in which the jobs end up in an optimum schedule.