Abstract
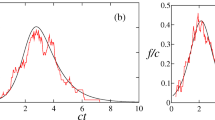
The typical duration of an epidemic in a sequence of linearly ordered populations shows a surprising nonmonotonic behaviour with respect to population size, which was noted by Swinton (1998) [Bull. Math. Biol., 60, 215–230]. This paper gives the sketch of a proof of the phenomenon.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Athreya, K. B. and P. E. Ney (1972). Branching Processes, Berlin: Springer.
Bailey, N. T. J. (1975). The Mathematical Theory of Infectious Diseases and its Applications, London: Griffin.
Barbour, A. D. (1974). On a functional central limit theorem for Markov population processes. Adv. Appl. Prob. 6, 21–39.
Barbour, A. D. (1975). The duration of the closed stochastic epidemic. Biometrika 62, 477–482.
Barbour, A. D. (1980). Density dependent Markov population processes, in Biological Growth and Spread, Lecture Notes in Biomathematics 38, W. Jäger, H. Rost and P. Tautu (Eds), Berlin: Springer, pp. 36–49.
Diekmann, O. and J. A. P. Heesterbeek (2000). Mathematical Epidemiology of Infectious Diseases: Model Building, Analysis and Interpretation, Chichester: Wiley.
Gnedenko, B. V. and A. N. Kolmogorov (1954). Limit Distributions for Sums of Independent Random Variables, Cambridge, MA: Addison-Wesley.
Kendall, D. G. (1966). Branching processes since 1873. J. London Math. Soc. 41, 385–406.
Kurtz, T. G. (1970). Solutions of ordinary differential equations as limits of pure jump Markov processes. J. Appl. Prob. 7, 49–58.
Swinton, J. (1998). Extinction times for epidemics in spatially structured closed populations. Bull. Math. Biol. 60, 215–230.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Barbour, A.D., Kälin, R. The duration of a sequence of epidemics. Bull. Math. Biol. 65, 397–405 (2003). https://doi.org/10.1016/S0092-8240(03)00010-7
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1016/S0092-8240(03)00010-7