Robust Stability Analysis of Grid-Connected Converters Based on Parameter-Dependent Lyapunov Functions

Abstract

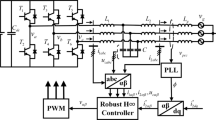
This paper deals with the problem of robust stability analysis of grid-connected converters with LCL filters controlled through a digital signal processor and subject to uncertain grid inductance. To model the uncertain continuous-time plant and the digital control gain, a discretization procedure, described in terms of a Taylor series expansion, is employed to determine an accurate discrete-time model. Then, a linear matrix inequality-based condition is proposed to assess the robust stability of the polynomial discrete-time augmented system that includes the filter state variables, the states of resonant controllers and the delay from the digital control implementation. By means of a parameter-dependent Lyapunov function, the proposed strategy has as main advantage to provide theoretical certification of stability of the uncertain continuous-time closed-loop system, circumventing the main disadvantages of previous approaches that employ approximate discretized models, neglecting the errors. Numerical simulations illustrate the benefits of the discretization technique and experimental results validate the proposed approach.

Appendices

State-Space Description of Resonant Controllers

In order to ensure zero steady-state error and also to reject harmonic disturbances, resonant controllers can be used. In continuous-time, it is possible to consider the following resonant controller

$$\begin{aligned} {\dot{\xi }}_i(t)=R_{ci} \xi _i(t) + M_{ci} e(t) \end{aligned}$$

(19)

where

$$\begin{aligned} R_{ci} = \left[ {\begin{array}{*{20}{c}} 0 &{}1\\ { - {\omega _i}^2}&{}{ - 2{\zeta _i}{\omega _i}} \end{array}} \right] ~,~ M_{ci} = \left[ {\begin{array}{*{20}{c}} 0\\ 1 \end{array}} \right] \end{aligned}$$

and the tracking error is given by

$$\begin{aligned} e(t)=i_{ref}(t)-z(t). \end{aligned}$$

(20)

In this representation, $\omega _i$ is the frequency of resonant controller and $\zeta _i$ is a damping factor, employed to avoid problems in the discrete-time implementation related with placing controller poles at the border of the unit circle.

A state-space discrete-time representation of this controller is given by

$$\begin{aligned} \xi _i(k+1)= R_i \xi _i(k)+ M_i e(k) \end{aligned}$$

(21)

where $\xi _i(k) \in \mathbb {R}^{2 \times 1}$, $R_i \in \mathbb {R}^{2 \times 2}$, $M_i \in \mathbb {R}^{2 \times 1}$.

Note that (21) also describes a resonant controller, but specialized for state feedback.

In the general case, a set of the above controllers can be used to represent multiple resonant controllers as

$$\begin{aligned} \xi (k+1)= R \xi (k) - M C_{\mathrm{dist}} x(k) + M i_{\mathrm{ref}}(k) \end{aligned}$$

(22)

where

$$\begin{aligned} \xi = \begin{bmatrix} \xi _1\\ \vdots \\ \xi _n \\ \end{bmatrix}, ~~ R = \begin{bmatrix} R_1&\quad&\quad \\&\quad \ddots&\quad \\&\quad&\quad R_n \\ \end{bmatrix}, ~~ M = \begin{bmatrix} M_1\\ \vdots \\ M_n \\ \end{bmatrix}. \end{aligned}$$

(23)

In this representation, the state vector has dimension 2n, where n is the number of resonant controllers to be implemented, and each resonant controller is discretized independently, resulting in the matrices R (block diagonal matrix) and M.

System Parameters and Control Gains

The parameters of the system used for controller design and to obtain the experimental results are given in Table 2.

Table 2 Parameters of the converter

Considering the parameters of Table 2 and tunning the resonant controllers at the frequencies of 60, 180, 300 and 420 Hz, the control gains designed in Maccari et al. (2014), used in Sect. 6, are given by (truncated with 4 decimal digits)

$$\begin{aligned} \mathbf{K }= \begin{bmatrix} -13.0046 \\ -0.8727 \\ -3.2444 \\ -0.5887 \\ 87.2641 \\ -86.5638 \\ 43.0993 \\ -41.8932 \\ 38.4751 \\ -37.7920 \\ 37.8061 \\ -36.2425 \end{bmatrix}'. \end{aligned}$$

(24)

Multi-nomial Series Development

In the matrix case, products in multi-nomial series are non-commutative. In this case, one can write $A_{c}(\alpha )$ as

$$\begin{aligned} A_{c}(\alpha )^q&= \left( \sum \limits _{i=1}^{N} \alpha _i A_{ci} \right) ^q =\sum \limits _{p \in {\mathcal {P}}(q)} \prod \limits _{i=1}^{q} \alpha _{p_i} A_{c_{p_i}} \nonumber \\&= \sum \limits _{p \in {\mathcal {P}}(q)} \alpha _{p_1} A_{c_{p_1}} \cdots \alpha _{p_q} A_{c_{p_q}} \nonumber \\&= \sum \limits _{p \in {\mathcal {P}}(q)} \alpha _{p} A_{c_{p}}, = \sum \limits _{k\in {\mathcal {K}}(q)} \alpha ^{k} \sum \limits _{p \in {\mathcal {R}}(k)} A_{c_{p}} \end{aligned}$$

(25)

where, $A_{c_{p}} = A_{c_{p_1}} \cdots A_{c_{p_q}}$ $\alpha ^k=\alpha _1^{k_1} \alpha _2^{k_2} \cdots \alpha _N^{k_N}$, $k=(k_1 k_2 \cdots k_N)$, $\alpha _p=(\alpha _{p_1}, \alpha _{p_2}, \ldots , \alpha _{p_q}$), $p=(p_1 p_2 \cdots p_q)$,

$$\begin{aligned} {\mathcal {K}}(q) \triangleq \Big \{ k = ( k_1 \cdots k_N ) \in \mathbb {N}^N : \sum _{j=1}^N k_j = q, ~~ k_j \ge 0 \Big \}, \end{aligned}$$

${\mathcal {P}}(q)$ is the set of q-tuples obtained as all possible combinations of nonnegative integers $p_i$, $i = 1, \ldots , q$, such that $p_i \in \{ 1,\ldots ,N \}$, that is,

$$\begin{aligned} {\mathcal {P}}(q) \triangleq \Big \{ p \in \mathbb {N}^q : p_i \in \{1,\ldots ,N \}, ~ i = 1, \ldots , q \Big \} \end{aligned}$$

and ${\mathcal {R}}(k)$, $k\in {\mathcal {K}}(q)$, is the subset of all q-tuples $p \in {\mathcal {P}}(q)$ such that the elements j of p have multiplicity $k_j$, for $j=1,\ldots ,N$, that is,

$$\begin{aligned} {\mathcal {R}}(k) \triangleq \Big \{ p \in \mathbb {N}^q : m_p(j) = k_j, ~j = 1, \ldots , N \Big \} \end{aligned}$$

where $m_p(j)$ denotes the multiplicity of the element j in p.

By definition, for N-tuples k and $k'$, one has that $k \ge k'$ if $k_{i} \ge k'_{i}$, $i = 1, \ldots , N$. Operations of summation $k + k'$ and subtraction $k - k'$ (whenever $k' \le k$) are defined componentwise.

Proof of Theorem 1

To prove Theorem 1, apply Schur’s complement in (18) to get

$$\begin{aligned} \underbrace{\begin{bmatrix} W(\alpha ) - \varPsi (\alpha )&\star \\ -X(\alpha )' + Y(\alpha ) A_{cl}^{\ell }(\alpha )&\Omega (\alpha ) - W(\alpha ) \end{bmatrix}}_{\varGamma } - \\ \lambda _{{\tilde{A}}} \begin{bmatrix} \delta _{{\tilde{A}}} I \\ 0 \end{bmatrix} \begin{bmatrix} \delta _{{\tilde{A}}} I \\ 0 \end{bmatrix}' - \lambda _{{\tilde{A}}}^{-1} \underbrace{\begin{bmatrix} X(\alpha ) \\ Y(\alpha ) \end{bmatrix}}_{V'} \begin{bmatrix} X(\alpha ) \\ Y(\alpha ) \end{bmatrix}' > 0. \end{aligned}$$

Knowing that $\varDelta A_{cl}^{\ell }(\alpha )' \varDelta A_{cl}^{\ell }(\alpha ) < \delta _{{\tilde{A}}}^{2}I$ and using Lemma 1, one obtains

$$\begin{aligned} \varGamma - \begin{bmatrix} \varDelta A_{cl}^{\ell }(\alpha ) \\ 0 \end{bmatrix} V - V' \begin{bmatrix} \varDelta A_{cl}^{\ell }(\alpha ) \\ 0 \end{bmatrix}' > 0 \end{aligned}$$

(26)

which is equivalent to

$$\begin{aligned} \begin{bmatrix} \begin{pmatrix} W(\alpha ) -{A_{cl}}(\alpha )' X(\alpha ) ' \\ - X(\alpha ) {A_{cl}}(\alpha ) \end{pmatrix}&\star \\ -X(\alpha )' + Y(\alpha ) {A_{cl}}(\alpha )&\Omega (\alpha ) -W(\alpha ) \end{bmatrix} > 0 \end{aligned}$$

(27)

where ${A_{cl}}(\alpha )$ is given by (16). Finally, multiplying (27) by ${\mathcal {B}}' = \begin{bmatrix} -I&{A_{cl}}(\alpha )'\end{bmatrix}$ on the left and by ${\mathcal {B}}$ on the right, one gets $W(\alpha ) - (A_{cl}^{\ell }(\alpha ) + \varDelta A_{cl}^{\ell }(\alpha ))' W(\alpha ) (A_{cl}^{\ell }(\alpha ) + \varDelta A_{cl}^{\ell }(\alpha )) > 0,$ that ensures the stability of the closed-loop system (15).