Skip to main content
SpringerLink
Log in

Numerical and exact analyses of Bures and Hilbert–Schmidt separability and PPT probabilities

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

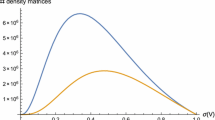
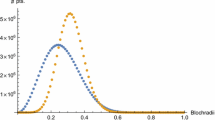
Abstract

We employ a quasirandom methodology, recently developed by Martin Roberts, to estimate the separability probabilities, with respect to the Bures (minimal monotone/statistical distinguishability) measure, of generic two-qubit and two-rebit states. This procedure, based on generalized properties of the golden ratio, yielded, in the course of almost seventeen billion iterations (recorded at intervals of five million), two-qubit estimates repeatedly close to nine decimal places to \(\frac{25}{341} =\frac{5^2}{11 \cdot 31} \approx 0.073313783\). However, despite the use of over twenty-three billion iterations, we do not presently perceive an exact value (rational or otherwise) for an estimate of 0.15709623 for the Bures two-rebit separability probability. The Bures qubit–qutrit case—for which Khvedelidze and Rogojin gave an estimate of 0.0014—is analyzed too. The value of \(\frac{1}{715}=\frac{1}{5 \cdot 11 \cdot 13} \approx 0.00139860\) is a well-fitting value to an estimate of 0.00139884. Interesting values \(\big (\frac{16}{12375} =\frac{4^2}{3^2 \cdot 5^3 \cdot 11}\) and \(\frac{625}{109531136}=\frac{5^4}{2^{12} \cdot 11^2 \cdot 13 \cdot 17}\big )\) are conjectured for the Hilbert–Schmidt (HS) and Bures qubit–qudit (\(2 \times 4\)) positive-partial-transpose (PPT)-probabilities. We re-examine, strongly supporting, conjectures that the HS qubit–qutrit and rebit–retrit separability probabilities are \(\frac{27}{1000}=\frac{3^3}{2^3 \cdot 5^3}\) and \(\frac{860}{6561}= \frac{2^2 \cdot 5 \cdot 43}{3^8}\), respectively. Prior studies have demonstrated that the HS two-rebit separability probability is \(\frac{29}{64}\) and strongly pointed to the HS two-qubit counterpart being \(\frac{8}{33}\) and a certain operator monotone one (other than the Bures) being \(1 -\frac{256}{27 \pi ^2}\).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9

Similar content being viewed by others

References

  1. Lovas, A., Andai, A.: Invariance of separability probability over reduced states in \(4 \times 4\) bipartite systems. J. Phys. A: Math. Theor. 50, 295303 (2017)

    Article  MathSciNet  Google Scholar 

  2. Życzkowski, K., Sommers, H.-J.: Hilbert–Schmidt volume of the set of mixed quantum states. J. Phys. A: Math. Gen. 36, 10115 (2003)

    Article  ADS  MathSciNet  Google Scholar 

  3. Bengtsson, I., Życzkowski, K.: Geometry of Quantum States: An Introduction to Quantum Entanglement. Cambridge University Press, Cambridge (2017)

    Book  Google Scholar 

  4. Caves, C.M., Fuchs, C.A., Rungta, P.: Entanglement of formation of an arbitrary state of two rebits. Found. Phys. Lett. 14(3), 199–212 (2001). https://doi.org/10.1023/A:1012215309321

    Article  MathSciNet  Google Scholar 

  5. Slater, P.B.: Master Lovas–Andai and equivalent formulas verifying the \(\frac{8}{33}\) two-qubit Hilbert–Schmidt separability probability and companion rational-valued conjectures. Quantum Inf. Process. 17, 83 (2018)

    Article  ADS  Google Scholar 

  6. Khvedelidze, A., Rogojin, I.: On the generation of random ensembles of qubits and qutrits: computing separability probabilities for fixed rank states. In: EPJ Web of Conferences (EDP Sciences), vol. 173 (2018)

    Article  Google Scholar 

  7. Milz, S., Strunz, W.T.: Volumes of conditioned bipartite state spaces. J. Phys. A: Math. Theor. 48, 035306 (2014)

    Article  ADS  MathSciNet  Google Scholar 

  8. Fei, J., Joynt, R.: Numerical computations of separability probabilities. Rep. Math. Phys. 78, 177 (2016)

    Article  ADS  MathSciNet  Google Scholar 

  9. Shang, J., Seah, Y.-L., Ng, H.K., Nott, D.J., Englert, B.-G.: Monte Carlo sampling from the quantum state space. I. New J. Phys. 17, 043017 (2015)

    Article  ADS  Google Scholar 

  10. Slater, P.B.: A concise formula for generalized two-qubit Hilbert–Schmidt separability probabilities. J. Phys. A: Math. Theor. 46, 445302 (2013)

    Article  ADS  MathSciNet  Google Scholar 

  11. Slater, P.B., Dunkl, C.F.: Moment-based evidence for simple rational-valued Hilbert–Schmidt generic 2\(\times 2\) separability probabilities. J. Phys. A: Math. Theor. 45, 095305 (2012)

    Article  ADS  MathSciNet  Google Scholar 

  12. Slater, P.B.: Dyson indices and Hilbert–Schmidt separability functions and probabilities. J. Phys. A: Math. Theor. 40, 14279 (2007)

    Article  ADS  MathSciNet  Google Scholar 

  13. Slater, P.B.: Extensions of generalized two-qubit separability probability analyses to higher dimensions, additional measures and new methodologies. Quantum Inf. Process. (2018b, to appear). arXiv preprint arXiv:1809.09040

  14. Adler, S.L.: Quaternionic Quantum Mechanics and Quantum Fields, vol. 88. Oxford University Press, Oxford (1995)

    MATH  Google Scholar 

  15. Życzkowski, K., Sommers, H.-J.: Induced measures in the space of mixed quantum states. J. Phys. A: Math. Gen. 34, 7111 (2001)

    Article  ADS  MathSciNet  Google Scholar 

  16. Szarek, S.J., Bengtsson, I., Życzkowski, K.: On the structure of the body of states with positive partial transpose. J. Phys. A: Math. Gen. 39, L119 (2006)

    Article  ADS  MathSciNet  Google Scholar 

  17. Samuel, J., Shivam, K., Sinha, S.: Lorentzian geometry of qubit entanglement (2018). arXiv preprint arXiv:1801.00611

  18. Avron, J., Kenneth, O.: Entanglement and the geometry of two qubits. Ann. Phys. 324, 470 (2009)

    Article  ADS  MathSciNet  Google Scholar 

  19. Braga, H., Souza, S., Mizrahi, S.S.: Geometrical meaning of two-qubit entanglement and its symmetries. Phys. Rev. A 81, 042310 (2010)

    Article  ADS  Google Scholar 

  20. Gamel, O.: Entangled Bloch spheres: Bloch matrix and two-qubit state space. Phys. Rev. A 93, 062320 (2016)

    Article  ADS  MathSciNet  Google Scholar 

  21. Jevtic, S., Pusey, M., Jennings, D., Rudolph, T.: Quantum steering ellipsoids. Phys. Rev. Lett. 113, 020402 (2014)

    Article  ADS  Google Scholar 

  22. Aubrun, G., Szarek, S.J.: Alice and Bob Meet Banach: The Interface of Asymptotic Geometric Analysis and Quantum Information Theory, vol. 223. American Mathematical Soc, Providence (2017)

    Book  Google Scholar 

  23. Życzkowski, K., Horodecki, P., Sanpera, A., Lewenstein, M.: Volume of the set of separable states. Phys. Rev. A 58, 883 (1998)

    Article  ADS  MathSciNet  Google Scholar 

  24. Petz, D., Sudár, C.: Geometries of quantum states. J. Math. Phys. 37, 2662 (1996)

    Article  ADS  MathSciNet  Google Scholar 

  25. Rexiti, M., Felice, D., Mancini, S.: The volume of two-qubit states by information geometry. Entropy 20, ISSN 1099-4300 (2018). http://www.mdpi.com/1099-4300/20/2/146

    Article  ADS  Google Scholar 

  26. Singh, R., Kunjwal, R., Simon, R.: Relative volume of separable bipartite states. Phys. Rev. A 89, 022308 (2014)

    Article  ADS  Google Scholar 

  27. Batle, J., Abdel-Aty, M.: Geometric approach to the distribution of quantum states in bipartite physical systems. JOSA B 31, 2540 (2014)

    Article  ADS  Google Scholar 

  28. Slater, P.B.: Bayesian quantum mechanics. Nature 367, 328 (1994)

    Article  ADS  Google Scholar 

  29. Slater, P.B.: Quantum coin-tossing in a Bayesian Jeffreys framework. Phys. Lett. A 206, 66 (1995)

    Article  ADS  Google Scholar 

  30. Kwek, L., Oh, C., Wang, X.-B.: Quantum Jeffreys prior for displaced squeezed thermal states. J. Phys. A: Math. Gen. 32, 6613 (1999)

    Article  ADS  MathSciNet  Google Scholar 

  31. Reconcile a pair of two-qubit boundary-state separability probability analyses. https://physics.stackexchange.com/questions/422887/reconcile-a-pair-of-two-qubit-boundary-state-separability-probability-analyses. Accessed 2018

  32. Slater, P.B.: Formulas for generalized two-qubit separability probabilities. Adv. Math. Phys. 2018, 9365213 (2018)

    Article  MathSciNet  Google Scholar 

  33. Slater, P.B.: Exact Bures probabilities that two quantum bits are classically correlated. Eur. Phys. J. B-Condens. Matter Complex Syst. 17, 471 (2000)

    Article  Google Scholar 

  34. Sarkar, A., Kumar, S.: Bures–Hall ensemble: spectral densities and average entropies (2019). arXiv preprint arXiv:1901.09587

    Article  Google Scholar 

  35. Šafránek, D.: Discontinuities of the quantum Fisher information and the Bures metric. Phys. Rev. A 95, 052320 (2017)

    Article  ADS  Google Scholar 

  36. Forrester, P.J., Kieburg, M.: Relating the Bures measure to the Cauchy two-matrix model. Commun. Math. Phys. 342, 151 (2016)

    Article  ADS  MathSciNet  Google Scholar 

  37. Braunstein, S.L., Caves, C.M.: Statistical distance and the geometry of quantum states. Phys. Rev. Lett. 72, 3439 (1994)

    Article  ADS  MathSciNet  Google Scholar 

  38. Bej, P., Deb, P.: Geometry of quantum state space and entanglement (2018). arXiv preprint arXiv:1805.11292

  39. Dunkl, C.F., Slater, P.B.: Separability probability formulas and their proofs for generalized two-qubit X-matrices endowed with Hilbert–Schmidt and induced measures. Random Matrices Theory Appl. 4, 1550018 (2015)

    Article  MathSciNet  Google Scholar 

  40. Penson, K.A., Życzkowski, K.: Product of Ginibre matrices: Fus–Catalan and Raney distributions. Phys. Rev. E 83, 061118 (2011)

    Article  ADS  Google Scholar 

  41. Al Osipov, V., Sommers, H.-J., Życzkowski, K.: Random Bures mixed states and the distribution of their purity. J. Phys. A: Math. Theor. 43, 055302 (2010)

    Article  ADS  MathSciNet  Google Scholar 

  42. Borot, G., Nadal, C.: Purity distribution for generalized random Bures mixed states. J. Phys. A: Math. Theor. 45, 075209 (2012)

    Article  ADS  MathSciNet  Google Scholar 

  43. Slater, P.B.: A priori probability that two qubits are unentangled. Quantum Inf. Process. 1, 397 (2002)

    Article  MathSciNet  Google Scholar 

  44. Slater, P.B.: Silver mean conjectures for 15-dimensional volumes and 14-dimensional hyperareas of the separable two-qubit systems. J. Geom. Phys. 53, 74 (2005)

    Article  ADS  MathSciNet  Google Scholar 

  45. Leobacher, G., Pillichshammer, F.: Introduction to Quasi-Monte Carlo Integration and Applications. Springer, Berlin (2014)

    Book  Google Scholar 

  46. Livio, M.: The Golden Ratio: The Story of Phi, the World’s Most Astonishing Number. Broadway Books, New York (2008)

    MATH  Google Scholar 

  47. The unreasonable effectiveness of quasirandom sequences. http://extremelearning.com.au/unreasonable-effectiveness-of-quasirandom-sequences/. Accessed 2018

  48. How can one generate an open ended sequence of low discrepancy points in 3D? https://math.stackexchange.com/questions/2231391/how-can-one-generate-an-open-ended-sequence-of-low-discrepancy-points-in-3d. Accessed 2018

  49. Devroye, L.: Non-uniform Random Variate Generation. Springer, Berlin (1986)

    Book  Google Scholar 

  50. Can I use compile to speed up inverseCDF? https://mathematica.stackexchange.com/questions/181099/can-i-use-compile-to-speed-up-inversecdf. Accessed 2019

  51. Slater, P.B.: Extended studies of separability functions and probabilities and the relevance of Dyson indices. J. Geom. Phys. 58, 1101 (2008)

    Article  ADS  MathSciNet  Google Scholar 

  52. Compute a certain ‘separability probability’ via a constrained 4D integration over \([-1,1]^4\). https://mathematica.stackexchange.com/questions/193337/compute-a-certain-separability-probability-via-a-constrained-4d-integration-ov. Accessed 2019

  53. A pair of integrals involving square roots and inverse trigonometric functions over the unit disk. https://mathoverflow.net/questions/325697/a-pair-of-integrals-involving-square-roots-and-inverse-trigonometric-functions-o. Accessed 2019

  54. Approximate/estimate the ratio of two multidimensional constrained integrals. https://mathematica.stackexchange.com/questions/193796/approximate-estimate-the-ratio-of-two-multidimensional-constrained-integrals. Accessed 2019

  55. Ye, D.: On the Bures volume of separable quantum states. J. Math. Phys. 50, 083502 (2009)

    Article  ADS  MathSciNet  Google Scholar 

  56. Slater, P.B.: Invariance of bipartite separability and PPT-probabilities over Casimir invariants of reduced states. Quantum Inf. Process. 15, 3745 (2016)

    Article  ADS  MathSciNet  Google Scholar 

  57. Chen, K., Wu, L.-A.: A matrix realignment method for recognizing entanglement. Quant. Inform. Comput. 3(3), 193–202 (2003)

    MathSciNet  MATH  Google Scholar 

  58. Bae, J., Chruściński, D., Hiesmayr, B.C.: Entanglement witness 2.0: Compressed entanglement witnesses. (2018). arXiv preprint arXiv:1811.09896

  59. Gabdulin, A., Mandilara, A.: Investigating bound entangled two-qutrit states via the best separable approximation. (2019). arXiv preprint arXiv:1906.08963

  60. Xiong, C., Spehner, D., Wu, J.: Geometric quantum discord for two-qubit X-states (2017). arXiv preprint arXiv:1710.04007

  61. Compute the two-fold partial integral, where the three-fold full integral is known. https://mathoverflow.net/questions/322958/compute-the-two-fold-partial-integral-where-the-three-fold-full-integral-is-kno. Accessed 2019

  62. Do these polynomials with harmonic number-related coefficients lie in some particular known class? https://math.stackexchange.com/questions/3115582/do-these-polynomials-with-harmonic-number-related-coefficients-lie-in-some-parti. Accessed 2019

  63. Ozawa, M.: Entanglement measures and the Hilbert–Schmidt distance. Phys. Lett. A 268, 158 (2000)

    Article  ADS  MathSciNet  Google Scholar 

  64. Sum a certain hypergeometric-function-based expression pertaining to an integration problem. https://mathematica.stackexchange.com/questions/189538/sum-a-certain-hypergeometric-function-based-expression-pertaining-to-an-integrat. Accessed 2019

  65. Tilma, T., Byrd, M., Sudarshan, E.: A parametrization of bipartite systems based on SU (4) Euler angles. J. Phys. A: Math. Gen. 35, 10445 (2002)

    Article  ADS  MathSciNet  Google Scholar 

  66. Slater, P.B.: Eigenvalues, separability and absolute separability of two-qubit states. J. Geom. Phys. 59, 17 (2009)

    Article  ADS  MathSciNet  Google Scholar 

  67. Maziero, J.: Random sampling of quantum states: a survey of methods. Braz. J. Phys. 45, 575 (2015)

    Article  ADS  Google Scholar 

  68. Can experimental data from a quantum computer be used to test separability probability conjectures? https://quantumcomputing.stackexchange.com/questions/5355/can-experimental-data-from-a-quantum-computer-be-used-to-test-separability-pro. Accessed 2019

  69. Smart, S.E., Schuster, D.I., Mazziotti, D.A.: Experimental data from a quantum computer verifies the generalized Pauli exclusion principle. Commun. Phys. 2, 2 (2019)

    Article  Google Scholar 

  70. Puchała, Z., Miszczak, J.A.: Probability measure generated by the superfidelity. J. Phys. A: Math. Theor. 44, 405301 (2011)

    Article  MathSciNet  Google Scholar 

  71. Zyczkowski, K., Slomczynski, W.: The Monge metric on the sphere and geometry of quantum states. J. Phys. A: Math. Gen. 34, 6689 (2001)

    Article  ADS  MathSciNet  Google Scholar 

  72. Slater, P.B.: Quantum and Fisher information from the Husimi and related distributions. J. Math. Phys. 47, 022104 (2006)

    Article  ADS  MathSciNet  Google Scholar 

  73. Rexiti, M., Felice, D., Mancini, S.: The volume of two-qubit states by information geometry. Entropy 20, 146 (2018)

    Article  ADS  Google Scholar 

Download references

Acknowledgements

This research was supported by the National Science Foundation under Grant No. NSF PHY-1748958.

Author information

Authors and Affiliations

  1. Kavli Institute for Theoretical Physics, University of California, Santa Barbara, CA, 93106-4030, USA

    Paul B. Slater

Authors
  1. Paul B. Slater
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Paul B. Slater.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Slater, P.B. Numerical and exact analyses of Bures and Hilbert–Schmidt separability and PPT probabilities. Quantum Inf Process 18, 312 (2019). https://doi.org/10.1007/s11128-019-2431-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-019-2431-2

Keywords

Search

Navigation