Generalized performance analysis of uplink and downlink dual-hop AF mixed RF/FSO relaying systems with pointing errors

Abstract

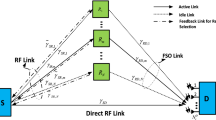
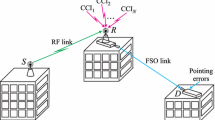
We present the performance analysis of dual-hop mixed radio frequency (RF)/free space optical (FSO) systems with fixed-gain amplify-and-forward (AF) relaying in the presence of pointing errors. The RF link undergoes the $\kappa$-$\mu$ shadowed fading and the FSO link experiences Fisher-Snedecor $\mathcal{F}$ turbulence with pointing errors. For uplink and downlink scenarios, we derive closed-form approximate expressions of the end-to-end signal-to-noise ratio (SNR) statistics, such as the cumulative distribution function (CDF) and the probability density function (PDF). By using both two statistics, the average bit error rate (BER), the ergodic capacity (EC), and the effective capacity are derived in terms of the bivariate Fox’s H function. These results illustrate the effects of system and channel parameters on the considered system, including atmospheric turbulence, pointing errors, multipath cluster numbers, shadowing parameter, quality parameter and different modulation schemes. Note that our results provide more general expressions. Finally, the derived approximate expressions are confirmed through Monte Carlo (MC) simulations. This work would contribute to the design and development of dual-hop mixed RF/FSO relaying systems.

Appendices

Appendix A Proof of Eq. (8)

The CDF of $\gamma$ can be written as

$$\begin{aligned} \begin{aligned} {{F}_{\gamma }}\left( \gamma \right)&=\int _{0}^{\infty }{\Pr \left( \frac{{{\gamma }_{1}}{{\gamma }_{2}}}{{{\gamma }_{2}}+C}<\gamma \left| {{\gamma }_{2}} \right. \right) {{f}_{{{\gamma }_{2}}}}\left( {{\gamma }_{2}} \right) d{{\gamma }_{2}}} \\&=\int _{x=0}^{\infty }{\int _{t=0}^{\gamma }{{{f}_{{{\gamma }_{1}}}}\left( t \right) {{f}_{{{\gamma }_{2}}}}\left( x \right) dtdx}} \\&\quad +\int _{x=0}^{\infty }{\int _{t=\gamma }^{\gamma +\frac{C\gamma }{x}}{{{f}_{{{\gamma }_{1}}}}\left( t \right) {{f}_{{{\gamma }_{2}}}}\left( x \right) dtdx}}. \end{aligned} \end{aligned}$$

(A.1)

Integrating over the same region and interchanging the integration order, we obtain

$$\begin{aligned} \begin{aligned} F_{\gamma } \left( \gamma \right) =F_{\gamma _{1} } \left( \gamma \right) +\int _{t=\gamma }^{\infty }F_{\gamma _{2} } \left( \frac{C\gamma }{t-\gamma } \right) f_{\gamma _{1} } \left( t\right) dt. \end{aligned} \end{aligned}$$

(A.2)

Inserting (3), (4), and (7) into (A.2) yields

$$\begin{aligned} \begin{aligned} {{F}_{\gamma }}\left( \gamma \right)&=1-\frac{\alpha {{\xi }_{\bmod }}^{2}}{r\Gamma \left( \delta \right) \Gamma \left( a \right) \Gamma \left( b \right) }\int _{t=\gamma }^{\infty }{{{t}^{-1}}\textrm{G}_{0,1}^{1,0}\left[ \delta {{\left( \frac{t}{{{\Omega }_{\alpha }}} \right) }^{\alpha }}\left| \begin{matrix} - \\ \delta \\ \end{matrix} \right. \right] } \\&\times {\textrm{H}}_{3,3}^{3,1}\left[ \frac{a\varphi {{\left( \frac{C\gamma }{t-\gamma } \right) }^{\frac{1}{r}}}}{\left( b-1 \right) {{\mu }_{r}}^{\frac{1}{r}}}\left| \begin{matrix} \left( 1-b,1 \right) ,\left( {{\xi }_{\bmod }}^{2}+1,1 \right) ,\left( 1,\frac{1}{r} \right) \\ \left( 0,\frac{1}{r} \right) ,\left( a,1 \right) ,\left( {{\xi }_{\bmod }}^{2},1 \right) \\ \end{matrix} \right. \right]\;dt. \end{aligned} \end{aligned}$$

(A.3)

Using the change of variable $x=t-\gamma$, resorting to Eq. (2.9.1) in Kilbas (2004) and expanding the Fox’s H function using Eq. (1.1.1) in Kilbas (2004), ${{F}_{\gamma }}\left( \gamma \right)$ can be derived as

$$\begin{aligned} \begin{aligned} {{F}_{\gamma }}\left( \gamma \right)&=1-\frac{\alpha {{\xi }_{\bmod }}^{2}}{r\Gamma \left( \delta \right) \Gamma \left( a \right) \Gamma \left( b \right) }\frac{1}{{{\left( 2\pi i \right) }^{2}}}\int _{{{\mathcal {C}}_{1}}}{\int _{{{\mathcal {C}}_{2}}}{\Gamma \left( \frac{t}{r}+\alpha s \right) }} \\&\times \frac{\Gamma \left( \delta +s \right) }{\Gamma \left( 1+\alpha s \right) }\frac{\Gamma \left( -\frac{t}{r} \right) \Gamma \left( a-t \right) \Gamma \left( {{\xi }_{\bmod }}^{2}-t \right) \Gamma \left( b+t \right) }{\Gamma \left( {{\xi }_{\bmod }}^{2}+1-t \right) } \\&\times {{\left( \frac{{{\Omega }_{\alpha }}^{\alpha }}{\delta {{\gamma }^{\alpha }}} \right) }^{s}}{{\left( \frac{a\varphi {{C}^{\frac{1}{r}}}}{\left( b-1 \right) {{\mu }_{r}}^{\frac{1}{r}}} \right) }^{t}}dsdt, \end{aligned}\ \end{aligned}$$

(A.4)

where ${{\mathcal {C}}_{1}}$ and ${{\mathcal {C}}_{2}}$ represent the t-plane and the s-plane contours, respectively.

Employing Eq. (1.1) in Mittal and Gupta (1972), ${{F}_{\gamma }}\left( \gamma \right)$ can be derived in closed form as (8).

Appendix B Proof of Eq. (9)

Differentiating (A.4) with respect to $\gamma$, the PDF of $\gamma$ can be expressed as

$$\begin{aligned} \begin{aligned} {{f}_{\gamma }}\left( \gamma \right)&=\frac{\alpha {{\xi }_{\bmod }}^{2}}{r\Gamma \left( \delta \right) \Gamma \left( a \right) \Gamma \left( b \right) \gamma }\frac{1}{{{\left( 2\pi i \right) }^{2}}}\int _{{{\mathcal {C}}_{1}}}{\int _{{{\mathcal {C}}_{2}}}{\Gamma \left( \frac{t}{r}+\alpha s \right) }} \\&\times \frac{\Gamma \left( \delta +s \right) }{\Gamma \left( \alpha s \right) }\frac{\Gamma \left( -\frac{t}{r} \right) \Gamma \left( a-t \right) \Gamma \left( {{\xi }_{\bmod }}^{2}-t \right) \Gamma \left( b+t \right) }{\Gamma \left( {{\xi }_{\bmod }}^{2}+1-t \right) } \\&\times {{\left( \frac{{{\Omega }_{\alpha }}^{\alpha }}{\delta {{\gamma }^{\alpha }}} \right) }^{s}}{{\left( \frac{a\varphi {{C}^{\frac{1}{r}}}}{\left( b-1 \right) {{\mu }_{r}}^{\frac{1}{r}}} \right) }^{t}}dsdt. \end{aligned} \end{aligned}$$

(B.1)

With the help of Eq. (1.1) in Mittal and Gupta (1972), ${{f}_{\gamma }}\left( \gamma \right)$ can be obtained in closed form as (9).

Appendix C Proof of Eq. (14)

Inserting (8) into (13) and then employing Eq.(3.381/4) in Gradshteyn and Ryzhik (2014), $\bar{P}_{e}$ can be obtained as

$$\begin{aligned} \begin{aligned} {{{\bar{P}}}_{e}}&=\frac{1}{2}-\frac{\alpha {{\xi }_{\bmod }}^{2}}{2r\Gamma \left( p \right) \Gamma \left( \delta \right) \Gamma \left( a \right) \Gamma \left( b \right) }\frac{1}{{{\left( 2\pi i \right) }^{2}}}\int _{{{\mathcal {C}}_{1}}}{\int _{{{\mathcal {C}}_{2}}}{\Gamma \left( \frac{t}{r}+\alpha s \right) }} \\&\times \frac{\Gamma \left( \delta +s \right) \Gamma \left( p-\alpha s \right) }{\Gamma \left( 1+\alpha s \right) }\frac{\Gamma \left( -\frac{t}{r} \right) \Gamma \left( a-t \right) \Gamma \left( {{\xi }_{\bmod }}^{2}-t \right) \Gamma \left( b+t \right) }{\Gamma \left( {{\xi }_{\bmod }}^{2}+1-t \right) } \\&\times {{\left( \frac{{{\Omega }_{\alpha }}^{\alpha }{{q}^{\alpha }}}{\delta } \right) }^{s}}{{\left( \frac{a\varphi {{C}^{\frac{1}{r}}}}{\left( b-1 \right) {{\mu }_{r}}^{\frac{1}{r}}} \right) }^{t}}dsdt. \end{aligned} \end{aligned}$$

(C.1)

Applying Eq. (1.1) in Mittal and Gupta (1972), $\bar{P}_{e}$ can be derived in closed form as (14).

Appendix D Proof of Eq. (21)

Substituting (B.1) into (20), $\overline{C}$ can be expressed as

$$\begin{aligned} \begin{aligned} \overline{C}&=\frac{\alpha {{\xi }_{\bmod }}^{2}}{2r\Gamma \left( a \right) \Gamma \left( b \right) \Gamma \left( \delta \right) }\frac{1}{{{\left( 2\pi i \right) }^{2}}}\int _{{{\mathcal {C}}_{1}}}{\int _{{{\mathcal {C}}_{2}}}{\Gamma \left( \frac{t}{r}+\alpha s \right) }} \\&\times \frac{\Gamma \left( \delta +s \right) }{\Gamma \left( \alpha s \right) }\frac{\Gamma \left( -\frac{t}{r} \right) \Gamma \left( a-t \right) \Gamma \left( {{\xi }_{\bmod }}^{2}-t \right) \Gamma \left( b+t \right) }{\Gamma \left( {{\xi }_{\bmod }}^{2}+1-t \right) } \\&\times {{\left( \frac{{{\Omega }_{\alpha }}^{\alpha }}{\delta } \right) }^{s}}{{\left( \frac{a\varphi {{C}^{\frac{1}{r}}}}{\left( b-1 \right) {{\mu }_{r}}^{\frac{1}{r}}} \right) }^{t}}\int _{0}^{\infty }{{{\gamma }^{-1-\alpha s}}\ln \left( 1+c\gamma \right) }d\gamma dsdt. \end{aligned} \end{aligned}$$

(D.1)

To evaluate (D.1), we can express the logarithmic function as Meijer’s G-function by

$$\begin{aligned} \begin{aligned} \ln (1+c \gamma )=\textrm{G}_{2,2}^{1,2}\left[ c \gamma \left| \begin{matrix} 1,1 \\ 1,0 \end{matrix}\right. \right] . \end{aligned} \end{aligned}$$

(D.2)

Then employing Eq. (07.34.21.0009.01) in Wolfram Research Inc. (2020), $\overline{C}$ can be obtained as

$$\begin{aligned} \begin{aligned} \overline{C}&=\frac{\alpha {{\xi }_{\bmod }}^{2}}{2r\Gamma \left( a \right) \Gamma \left( b \right) \Gamma \left( \delta \right) }\frac{1}{{{\left( 2\pi i \right) }^{2}}}\int _{{{\mathcal {C}}_{1}}}{\int _{{{\mathcal {C}}_{2}}}{{}}} \\&\times \Gamma \left( \frac{t}{r}+\alpha s \right) \frac{\Gamma \left( -\alpha s+1 \right) \Gamma \left( \alpha s \right) \Gamma \left( \delta +s \right) }{\Gamma \left( 1+\alpha s \right) } \\&\times \frac{\Gamma \left( -\frac{t}{r} \right) \Gamma \left( a-t \right) \Gamma \left( {{\xi }_{\bmod }}^{2}-t \right) \Gamma \left( b+t \right) }{\Gamma \left( {{\xi }_{\bmod }}^{2}+1-t \right) } \\&\times {{\left( \frac{{{c}^{\alpha }}{{\Omega }_{\alpha }}^{\alpha }}{\delta } \right) }^{s}}{{\left( \frac{a\varphi {{C}^{\frac{1}{r}}}}{\left( b-1 \right) {{\mu }_{r}}^{\frac{1}{r}}} \right) }^{t}}dsdt. \end{aligned} \end{aligned}$$

(D.3)

Applying Eq. (1.1) in Mittal and Gupta (1972), $\overline{C}$ can be expressed in closed form as (21).