Abstract
A method for designing the key parameters of rotational centrifugal pendulum vibration absorbers is proposed herein. The vibration equations of the pendulum and rotor were established by utilizing Lagrange’s equations. It was found that there were two kinds of rotational centrifugal pendulum vibration absorbers (CPVAs): a linear rotational CPVA and a nonlinear rotational CPVA, which both behaved approximately as linear oscillators. The choice of the linear rotational coefficient and the nonlinear rotational coefficient (only for the nonlinear rotational CPVA) was analyzed, and the selection of the tuning order and trajectories of the centroid of the pendulum was investigated based on tautochronic design theory. In this study, the time variables were transformed to angle variables in the vibration equations. The parameters in the equations were then non-dimensionalized, and the equations were decoupled. Finally, the vibration responses of the pendulum and rotor were solved using the harmonic balance method. The results demonstrated that the linear rotational CPVA designed by utilizing tautochronic theory provided a better damping effect than the nonlinear rotational and non-rotational CPVAs. Furthermore, the linear rotational CPVA could decrease the impact effect on the rotor by adjusting the linear rotational angle and ensure its damping performance.






Similar content being viewed by others
Availability of data and material
The data used or analyzed during the current study are available from the corresponding author on reasonable request.
Code availability
The self-made calculation program during the current study are available from the corresponding author on reasonable request.
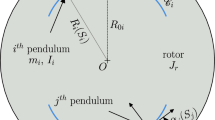
Abbreviations
- a :
-
Radius of a stationary circle
- a r :
-
Radius of a roller
- A 1 :
-
Linear rotational coefficient
- A 3 :
-
Nonlinear rotational coefficient
- b :
-
Radius of a rolling circle
- B c ,1 :
-
Coefficient of 1st-order cosine term
- B c ,2 :
-
Coefficient of 2nd-order cosine term
- B c , k :
-
Coefficient of kth-order cosine term
- B s ,1 :
-
Coefficient of 1st-order sine term
- B s ,2 :
-
Coefficient of 2nd-order sine term
- B s , k :
-
Coefficient of kth-order sine term
- c :
-
Damping coefficient of the pendulum
- C :
-
Distance from the vertex on the pendulum path to the center of rotor
- D :
-
Distance from the centers of the rollers at the initial point (β = 0) to the horizontal axis
- g(s):
-
Non-dimensional version of G(S)
- G(S):
-
A projection of the tangent of the path onto the moment arm to the center of the rotor at S
- H :
-
Distance from the centers of the rollers at the initial point (β = 0) to the vertical axis
- J :
-
Moment of inertia of the rotor
- J p :
-
Moment of inertia of the pendulum about its centroid
- k :
-
Order of the harmonic
- m p :
-
Mass of the pendulum
- n :
-
Excitation order
- \(\tilde{n}\) :
-
Linear tuning value
- r 1 :
-
Radius of gyration of the pendulum about its centroid
- R p :
-
Distance from any point on the pendulum path to the center of the rotor
- s :
-
Non-dimensional version of S
- \(s^{^{\prime}}\) :
-
Derivative of s with respect to θ
- \(s^{^{\prime\prime}}\) :
-
Second derivative of s with respect to θ
- S :
-
Arc length variable of the centroid of the pendulum originating from its vertex in xoy
- \(\dot{S}\) :
-
Derivative of S with respect to time
- \(\ddot{S}\) :
-
Second derivative of S with respect to time
- \(S^{^{\prime}}\) :
-
Derivative of S with respect to θ
- \(S^{^{\prime\prime}}\) :
-
Second derivative of S with respect to θ
- S 0 :
-
Vibration amplitude of the pendulum
- t :
-
Time
- T :
-
Amplitude of the fluctuating torque applied on the rotor
- T tol :
-
Total kinetic energy of the pendulum and rotor
- x :
-
Horizontal coordinate of the centroid of the pendulum
- \(x^{^{\prime}}\) :
-
Derivative of x with respect to φ
- \(x^{^{\prime\prime}}\) :
-
Second derivative of x with respect to φ
- xoy :
-
A coordinate system rotating with a velocity equal to the angular velocity of the rotor; the origin of xoy coincides with the center of the rotor
- y :
-
Vertical coordinate of the centroid of the pendulum
- \(y^{^{\prime}}\) :
-
Derivative of y with respect to φ
- \(y^{^{\prime\prime}}\) :
-
Second derivative of y with respect to φ
- α :
-
Angle between the line connecting the centroid of the pendulum with the center of the rotor and the negative y-axis
- α 0 :
-
Phase angle of the pendulum
- β :
-
Rotational angle of the pendulum about its centroid in xoy
- ε :
-
Scaling parameter
- θ :
-
Rotational angle of the rotor
- \(\dot{\theta }\) :
-
Derivative of θ with respect to time
- \(\ddot{\theta }\) :
-
Second derivative of θ with respect to time
- λ :
-
Nonlinear tuning value
- λ e 1 :
-
Nonlinear tuning value of the tautochronic non-rotational CPVA
- λ e 2 :
-
Nonlinear tuning value of the tautochronic linear rotational CPVA
- \(\tilde{\lambda }_{e}\) :
-
Nonlinear tuning value of the tautochronic nonlinear rotational CPVA
- μ :
-
Non-dimensional version of c
- \(\tilde{\mu }\) :
-
Scaled version of μ
- \(v = {{\dot{\theta }} \mathord{\left/ {\vphantom {{\dot{\theta }} \Omega }} \right. \kern-\nulldelimiterspace} \Omega }\) :
-
Non-dimensional rotor speed
- \(v^{^{\prime}} = {{dv} \mathord{\left/ {\vphantom {{dv} {d\theta }}} \right. \kern-\nulldelimiterspace} {d\theta }}\) :
-
Non-dimensional rotor acceleration
- ρ :
-
Path’s radius of curvature at the point at an arc length distance S from the vertex
- ρ 0 :
-
Path’s radius of curvature at the vertex
- σ 1 :
-
Adjustment factor of the linear rotational coefficient in Scheme II
- σ 2 :
-
Adjustment factor of the linear rotational coefficient in Scheme III
- Γ:
-
Non-dimensional version of T
- \(\tilde{\Gamma }\) :
-
Scaled version of Γ
- φ :
-
Sweeping angle of the line connecting the centers of the stationary and rolling circles
- \(\phi\) :
-
Sweeping angle of the radius of curvature from the vertex
- \(\phi_{1}\) :
-
Angle between the tangent line at any point on the left cutout on the rotor and the horizontal axis
- \(w^{^{\prime}}\) :
-
Scaled version of \(v^{^{\prime}}\)
- \(\left| {w^{^{\prime}} } \right|_{n}\) :
-
Amplitude of nth-order harmonic of \(w^{^{\prime}}\)
- Ω:
-
Mean rotor speed
- (x b 1,y b 1):
-
Coordinate of the left vertex of the cutout on the pendulum
- (x b 2, y b 2):
-
Coordinate of the right vertex of the cutout on the pendulum
- (x r 1, y r 1):
-
Coordinate of the center of the left roller
- (x r 2, y r 2):
-
Coordinate of the center of the right roller
- (x E, y E):
-
Coordinate of the midpoint of the line connecting (xb1, yb1) and (xb2, yb2)
- (x 1, y 1):
-
Coordinates of curve C1
- (x 2, y 2):
-
Coordinates of curve C2
- Non-rotational CPVA:
-
Pendulum moves along the prescribed path, but no rotational motion occurs in xoy.
- Rotational CPVA:
-
Pendulum moves along the prescribed path with rotational motions occurring in xoy.
- Linear rotational CPVA:
-
Rotational angle of the pendulum varies linearly with the arc length displacement in xoy.
- Nonlinear rotational CPVA:
-
Rotational angle of the pendulum varies nonlinearly with the arc length displacement in xoy
References
McCutcheon (KD) No short days: The struggle to Develop the R-2800 “Double Wasp” Crankshaft, 2010. http://www.enginehistory.org
Alsuwaiyan AS (1999) Performance, stability, and localization of systems of vibration absorbers. PhD thesis, Michigan State University
Haddow AG, Shaw SW (2003) Centrifugal pendulum vibration absorbers: an experimental and theoretical investigation. Nonlinear Dyn 34(3–4):293–3071
Alsuwaiyan AS, Shaw SW (2002) Performance and dynamic stability of general-path centrifugal pendulum vibration absorbers. J Sound Vib 252(5):791–815
Alsuwaiyan AS, Shah SW et al (2014) Non-synchronous and localized responses of systems of identical centrifugal pendulum vibration absorbers. Arab J SciEng. https://doi.org/10.1007/s13369-014-1464-1
Issa JS, Shaw SW (2015) Synchronous and non-synchronous responses of systems with multiple identical nonlinear vibration absorbers. J Sound Vib 348:105–125
Shaw SW, Geist B (2010) Tuning for performance and stability in systems of nearly tautochronic torsional vibration absorbers. J VibAcoust 132(4):1266–1266
Monroe RJ, Shaw SW, Haddow AH et al (2011) Accounting for roller dynamics in the design of bifilar torsional vibration absorbers. J VibAcoust 10(1115/1):4003942
Vidmar BJ, Shaw SW, Feeny BF et al (2013) Nonlinear interactions in systems of multiple order centrifugal pendulum vibration absorbers. J VibAcoust 135(6):061012
Shi CZ, Parker RG (2012) Modal properties and stability of centrifugal pendulum vibration absorber systems with equally spaced, identical absorbers. J Sound Vib 331(21):4807–4824
Shi C, Parker RG (2013) Modal structure of centrifugal pendulum vibration absorber systems with multiple cyclically symmetric groups of absorbers. J Sound Vib 332(18):4339–4353
Monroe RJ, Shaw SW (2013) Nonlinear transient dynamics of pendulum torsional vibration absorbers—Part I: theory. J VibAcoust 135(1):011017.1-011017.10
Monroe RJ, Shaw SW (2013) Nonlinear transient dynamics of pendulum torsional vibration absorbers—Part II: Experimental results. J VibAcoust 135(1):0110181–0110187
Vidmar BJ, Feeny BF, Shaw SW et al (2012) The effects of Coulomb friction on the performance of centrifugal pendulum vibration absorbers. Nonlinear Dyn 69(1–2):589–600
Mayet J, Ulbrich H (2014) Tautochronic centrifugal pendulum vibration absorbers: General design and analysis. J Sound Vib 333(3):711–729
Mustafa A, Shaw SW (2016) Application of the harmonic balance method to centrifugal pendulum vibration absorbers. Special Topics in Structural Dynamics, Vol 6. Springer.
Denman HH (1992) Tautochronic bifilar pendulum torsion absorbers for reciprocating engines. J Sound Vib 159(2):251–277
Funding
This study was sponsored by the Project funded by China Postdoctoral Science Foundation under Grant No. 2019M663888XB and Natural Science Foundation of Chongqing, China under Grant No. cstc2020jcyj-msxmX0121.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflicts of interest
The authors declare that they have no conflict of interest.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix A: General path representation
In Fig.
7, the epicycloidal path represented by a dotted line was formed by tracing the path that a perimeter point of a rolling circle of radius b produces as it rolls around a stationary circle of radius a.
φ represents the sweeping angle of the line connecting the centers of the two circles. We can obtain the equations of this epicycloid as follows:
We differentiate Eq. (43) with respect to φ and obtain
The second derivative of Eq. (43) with respect to φ can be obtained as follows:
The radius of curvature of this epicycloid can be obtained as follows:
The radius of curvature at the vertex can be obtained while φ = 0, as follows:
In Fig. 7, the angle between the tangent line at point P2 and the negative vertical axis is represented by \(\phi\), which is the sweeping angle of the radius of curvature from the initial point P1. Since \(\phi\) is a function of φ, the arc length starts from P1 to P2, can be expressed as
where \(\phi = - \arctan ({{x{^{\prime}} } \mathord{\left/ {\vphantom {{x{^{\prime}} } {y{^{\prime}} }}} \right. \kern-\nulldelimiterspace} {y{^{\prime}} }})\). The derivative of \(\phi\) with respect to φ can then be obtained as follows:
where
The expression of S can be obtained as follows:
Therefore, the equation relating ρ and S can be obtained as follows:
If a = 0, then λ2 = 0, the rolling circle actually rolls on a fixed point, and the trace becomes a circle. However, if a = ∞, then λ2 = 1, the rolling circle actually rolls on a straight line, and the trace becomes a cycloid. The other values of λ within the lower and upper boundaries of 0 and 1 cause the paths to become epicycloids, but only a special value provides the tautochrone.
In Fig. 8, the angles between the tangent line of any point on the trace and horizontal axis are represented by \(\phi\). The expression of \(\phi\) can be obtained using Eq. (52), as follows:
The relationships between the parameters in Eq. (53) are shown in Fig. 9, where
The coordinate of the centroid of the pendulum in Fig. 8 can be expressed as
If λ ≠ 1, we can obtain
The square of the distance between the centroid of the pendulum and the center of rotor can be expressed as
According to tuning theory, \(\sqrt {{{(C - \rho_{0} )} \mathord{\left/ {\vphantom {{(C - \rho_{0} )} {\rho_{0} }}} \right. \kern-\nulldelimiterspace} {\rho_{0} }}} { = }n\), and therefore, \(C = \rho_{0} (n^{2} + 1)\). The substitution of Eq. (57) into Eq. (11) in the main text leads to the condition of the tautochrone of the epicycloid:
Furthermore, it can be proven that the center of the stationary circle coincides with the center of the rotor if Eq. (58) is satisfied [8].
Appendix B: Equations of cutouts of rotational CPVA
Figure 10 shows the schematic diagram of a rotating pendulum, in which (0, −C) represents the vertex of the trajectory of the centroid of the pendulum, and H and D represent the distances from the centers of the rollers at the initial point (β = 0) to the vertical and horizontal axes, respectively. If the radius of the roller is ar, the vertices of the cutouts on the rotor can be represented by (−H, −D−ar) and (H, −D−ar), respectively. (xb1, yb1) and (xb2, yb2) represent the vertices of the cutouts on the pendulums, respectively, (xE, yE) represents the midpoint of the line connecting (xb1, yb1) and (xb2, yb2), and (xr1, yr1) and (xr2, yr2) represent the centers of the two rollers. The left cutout curves on the rotor and pendulum can be expressed by C1 and C2, respectively.
Now that the coordinates of the centroid of the pendulum can be expressed by Eq. (56), according to the structural relations, the coordinate of (xE, yE) can be obtained as follows:
Since point E is located at the middle of the line connecting (xb1, yb1) and (xb2, yb2), the coordinates of point b1 can be expressed as
Similarly, (xr1, yr1) is located at the middle of the line connecting (xb1, yb1) and (− H, − D − ar), and therefore,
In Fig. 10, supposing that the angle between the tangent line at any point on the left cutout on the rotor and the horizontal axis is represented by \(\phi_{1}\), then the curve C1 can be expressed as
We differentiate Eq. (61) with respect to S and obtain
\(\phi_{1}\) in Eq. (62) can be expressed as
Therefore, \(\sin \phi_{1}\) and \(\cos \phi_{1}\) in Eq. (62) can be expressed as
Substitution of Eq. (65) into Eq. (62) yields the equation of C1 without \(\phi_{1}\). In the general structural design of the CPVA, curve C2 is regarded as anti-symmetric with curve C1 about the center of the roller. Therefore, if the centroid of the pendulum is located at (0, −C), the curve C2 is anti-symmetric with the curve C1 about (−H, −D), and the expression of C2 at the initial point can also be obtained:
The right cutouts on the rotor and pendulum can also be obtained using the symmetric property.
If β = 0, then \(\phi_{1} = \phi\), and Eqs. (62) and (66) become equations describing the cutouts on the rotor and pendulum of the non-rotational CPVA system, respectively.
Rights and permissions
About this article
Cite this article
Tan, X., Yang, S., Yang, J. et al. Study of dynamics of rotational centrifugal pendulum vibration absorbers based on tautochronic design. Meccanica 56, 1905–1920 (2021). https://doi.org/10.1007/s11012-021-01340-4
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11012-021-01340-4