Skip to main content
SpringerLink
Log in

Implicit–Explicit Runge–Kutta Schemes and Applications to Hyperbolic Systems with Relaxation

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

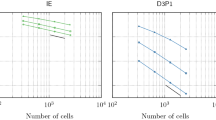
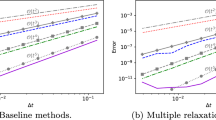
Abstract

We consider new implicit–explicit (IMEX) Runge–Kutta methods for hyperbolic systems of conservation laws with stiff relaxation terms. The explicit part is treated by a strong-stability-preserving (SSP) scheme, and the implicit part is treated by an L-stable diagonally implicit Runge–Kutta method (DIRK). The schemes proposed are asymptotic preserving (AP) in the zero relaxation limit. High accuracy in space is obtained by Weighted Essentially Non Oscillatory (WENO) reconstruction. After a description of the mathematical properties of the schemes, several applications will be presented

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Arora, M., and Roe, P. L. (1998). Issues and strategies for hyperbolic problems with stiff source terms in Barriers and challenges in computational fluid dynamics, Hampton, VA, 1996, Kluwer Academic Publication, Dordrecht, pp. 139–154.

  2. U. Ascher L. Petzold (1998) Computer Methods for Ordinary Differential Equations, and Differential Algebraic Equations SIAM Philadelphia

    Google Scholar 

  3. U. Ascher S. Ruuth R.J. Spiteri (1997) ArticleTitleImplicit-explicit Runge–Kutta methods for time dependent partial differential equations Appl. Numer. Math. 25 151–167 Occurrence Handle98i:65054 Occurrence Handle10.1016/S0168-9274(97)00056-1

    Article  MathSciNet  Google Scholar 

  4. U. Ascher S. Ruuth B. Wetton (1995) ArticleTitleImplicit-explicit methods for time dependent PDE’s SIAM J. Numer. Anal. 32 797–823 Occurrence Handle96j:65076

    MathSciNet  Google Scholar 

  5. A. Aw M. Rascle (2000) ArticleTitleResurrection of second order models of traffic flow? SIAM. J. Appl. Math. 60 916–938 Occurrence Handle2001a:35111 Occurrence Handle10.1137/S0036139997332099

    Article  MathSciNet  Google Scholar 

  6. A. Aw A. Klar T. Materne M. Rascle (2002) ArticleTitleDerivation of continuum traffic flow models from microscopic follow-the-leader models SIAM J. Appl. Math. 63 259–278 Occurrence Handle2003m:35148 Occurrence Handle10.1137/S0036139900380955

    Article  MathSciNet  Google Scholar 

  7. R.E. Caflisch S. Jin G. Russo (1997) ArticleTitleUniformly accurate schemes for hyperbolic systems with relaxation SIAM J. Numer. Anal. 34 246–281 Occurrence Handle98a:65112 Occurrence Handle10.1137/S0036142994268090

    Article  MathSciNet  Google Scholar 

  8. G.Q. Chen D. Levermore T.P. Liu (1994) ArticleTitleHyperbolic conservations laws with stiff relaxation terms and entropy Comm. Pure Appl. Math. 47 787–830 Occurrence Handle95h:35133

    MathSciNet  Google Scholar 

  9. B.O. Dia M. Schatzman (1996) ArticleTitleCommutateur de certains semi-groupes holomorphes et applications aux directions alternées Math. Modelling Num. Anal. 30 343–383 Occurrence Handle97e:47055

    MathSciNet  Google Scholar 

  10. S. Gottlieb C.-W. Shu (1998) ArticleTitleTotal variation diminishing Runge–Kutta schemes Math. Comp. 67 73–85 Occurrence Handle98c:65122 Occurrence Handle10.1090/S0025-5718-98-00913-2

    Article  MathSciNet  Google Scholar 

  11. S. Gottlieb C.-W. Shu E. Tadmor (2001) ArticleTitleStrong-stability-preserving high order time discretization methods SIAM Review 43 89–112 Occurrence Handle2002f:65132 Occurrence Handle10.1137/S003614450036757X

    Article  MathSciNet  Google Scholar 

  12. E. Hairer (1981) ArticleTitleOrder conditions for numerical methods for partitioned ordinary differential equations Numerische Mathematik 36 431–445 Occurrence Handle0462.65049 Occurrence Handle82j:65047 Occurrence Handle10.1007/BF01395956

    Article  MATH  MathSciNet  Google Scholar 

  13. E. Hairer S.P. Nørsett G. Wanner (1987) Solving Ordinary Differential Equations, Vol 1 Nonstiff problems Springer-Verlag New York

    Google Scholar 

  14. E. Hairer G. Wanner (1987) Solving Ordinary Differential Equations, Vol.2 Stiff and Differential-algebraic Problems Springer-Verlag New York

    Google Scholar 

  15. Jahnke T., Lubich C. (2000). Error bounds for exponential operator splitting. BIT 735–744

  16. J. Jenkins M. Richman (1985) ArticleTitleGrad’s 13-moment system for a dense gas of inelastic spheres Arch. Rat. Mech. Anal. 87 355–377 Occurrence Handle86b:73032 Occurrence Handle10.1007/BF00250919

    Article  MathSciNet  Google Scholar 

  17. S. Jin (1995) ArticleTitleRunge–Kutta methods for hyperbolic systems with stiff relaxation terms J. Comput. Phys. 122 51–67 Occurrence Handle0840.65098 Occurrence Handle96g:65084 Occurrence Handle10.1006/jcph.1995.1196

    Article  MATH  MathSciNet  Google Scholar 

  18. S. Jin Z.P. Xin (1995) ArticleTitleThe relaxation schemes for systems of conservation laws in arbitrary space dimensions Comm. Pure Appl. Math. 48 IssueID3 235–276 Occurrence Handle96c:65134

    MathSciNet  Google Scholar 

  19. C.A. Kennedy M.H. Carpenter (2003) ArticleTitleAdditive Runge–Kutta schemes for convection-diffusion-reaction equations Appl. Numer. Math. 44 139–181 Occurrence Handle2003m:65111 Occurrence Handle10.1016/S0168-9274(02)00138-1

    Article  MathSciNet  Google Scholar 

  20. R.J. LeVeque (1992) Numerical Methods for Conservation Laws BirkhauserVerlag Basel

    Google Scholar 

  21. S.F. Liotta V. Romano G. Russo (2000) ArticleTitleCentral schemes for balance laws of relaxation type SIAM J. Numer. Anal. 38 1337–1356 Occurrence Handle2001j:65128 Occurrence Handle10.1137/S0036142999363061

    Article  MathSciNet  Google Scholar 

  22. T.P. Liu (1987) ArticleTitleHyperbolic conservation laws with relaxation Comm. Math. Phys. 108 153–175 Occurrence Handle0633.35049 Occurrence Handle88f:35092 Occurrence Handle10.1007/BF01210707

    Article  MATH  MathSciNet  Google Scholar 

  23. Marquina, A., and Serna, S. (2004). Capturing Shock Waves in Inelastic Granular Gases, UCLA-CAM Report, 04–04

  24. L. Pareschi (2001) ArticleTitleCentral differencing based numerical schemes for hyperbolic conservation laws with stiff relaxation terms SIAM J. Num. Anal. 39 1395–1417 Occurrence Handle1020.65048 Occurrence Handle2002j:65086

    MATH  MathSciNet  Google Scholar 

  25. L. Pareschi G. Russo (2001) ArticleTitleImplicit-explicit Runge-Kutta schemes for stiff systems of differential equations. Adv Theory Comput Math. 3 269–289 Occurrence Handle2005a:65065

    MathSciNet  Google Scholar 

  26. Pareschi, L., and Russo, G. (2003). High order asymptotically strong-stability-preserving methods for hyperbolic systems with stiff relaxation, Proceedings HYP2002, Pasadena USA, Springer, Berlin, 241–255.

  27. Pareschi, L., and Russo, G. (2004). Stability analysis of implicit–explicit Runge–Kutta schemes. preprint.

  28. J. Qiu C.-W. Shu (2002) ArticleTitleOn the construction, comparison, and local characteristic decomposition for high-order central WENO schemes J. Comput. Phys. 183 IssueID1 187–209 Occurrence Handle2003j:65083 Occurrence Handle10.1006/jcph.2002.7191

    Article  MathSciNet  Google Scholar 

  29. C.-W. Shu (1988) ArticleTitleTotal variation diminishing time discretizations SIAM J. Sci. Stat. Comput. 9 1073–1084 Occurrence Handle0662.65081 Occurrence Handle90a:65196 Occurrence Handle10.1137/0909073

    Article  MATH  MathSciNet  Google Scholar 

  30. Shu C.-W. (2000). Essentially Non Oscillatory and Weighted Essentially Non Oscillatory Schemes for Hyperbolic Conservation Laws, in Advanced numerical approximation of nonlinear hyperbolic equations, Lecture Notes in Mathematics, 1697, 325–432.

  31. C.-W. Shu S. Osher (1988) ArticleTitleEfficient implementation of essentially nonoscillatory shock-capturing schemes J. Comput. Phys. 77 IssueID2 439–471 Occurrence Handle89g:65113 Occurrence Handle10.1016/0021-9991(88)90177-5

    Article  MathSciNet  Google Scholar 

  32. R.J. Spiteri S.J. Ruuth (2002) ArticleTitleA new class of optimal strong-stability-preserving time discretization methods. SIAM J. Num. Anal. 40 IssueID2 469–491 Occurrence Handle2003g:65083

    MathSciNet  Google Scholar 

  33. G. Strang (1968) ArticleTitleOn the construction and comparison of difference schemes SIAM J. Numer. Anal. 5 505–517 Occurrence Handle38 #4057 Occurrence Handle10.1137/0705041

    Article  MathSciNet  Google Scholar 

  34. Toscani G. Kinetic and hydrodinamic models of nearly elastic granular flows. Monatsch. Math. 142(1–2):179–192

  35. G.B. Whitham (1974) Linear and Nonlinear waves Wiley New York

    Google Scholar 

  36. X. Zhong (1996) ArticleTitleAdditive semi-implicit Runge–Kutta methods for computing high speed nonequilibrium reactive flows J. Comp. Phys. 128 19–31 Occurrence Handle0861.76057

    MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, University of Ferrara, Via Machiavelli 35, I-44100, Ferrara, Italy

    Lorenzo Pareschi

  2. Department of Mathematics and Computer Science, University of Catania, Via A.Doria 6, 95125, Catania, Italy

    Giovanni Russo

Authors
  1. Lorenzo Pareschi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Giovanni Russo
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Lorenzo Pareschi.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Pareschi, L., Russo, G. Implicit–Explicit Runge–Kutta Schemes and Applications to Hyperbolic Systems with Relaxation. J Sci Comput 25, 129–155 (2005). https://doi.org/10.1007/s10915-004-4636-4

Download citation

  • Received:

  • Accepted:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-004-4636-4

Keywords

AMS Subject Classification

Search

Navigation