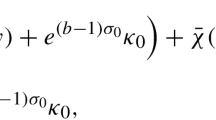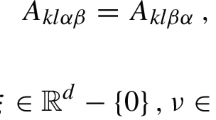Skip to main content
SpringerLink
Log in

Local Well-Posedness and Sensitivity Analysis for the Self-Organized Kinetic Model

  • Published:
Acta Applicandae Mathematicae Aims and scope Submit manuscript

Abstract

We consider the self-organized kinetic model (SOK), which was derived as the mean field limit of the Couzin-Vicsek algorithm. This model yields a singularity when the particle flux vanishes. By showing that the singularity does not happen in finite time, we obtain local existence and uniqueness of smooth solutions to SOK. Furthermore, considering uncertainties in the initial data and in the interaction kernel, we analyze the random SOK model (RSOK). We provide local sensitivity analysis to justify the regularity with respect to the random parameter and the stability of solutions to RSOK.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Aldana, M., Huepe, C.: Phase transitions in self-driven many-particle systems and related non-equilibrium models: a network approach. J. Stat. Phys. 112, 135–153 (2003)

    Article  Google Scholar 

  2. Bolley, F., Cañizo, J.A., Carrillo, J.A.: Mean-field limit for the stochastic Vicsek model. Appl. Math. Lett. 25(3), 339–343 (2012)

    Article  MathSciNet  Google Scholar 

  3. Briant, M., Merino-Aceituno, S.: Cauchy theory and mean-field limit for general Vicsek models in collective dynamics (2020). 2004.00883

  4. Couzin, I.D., Krause, J., James, R., Ruxton, G.D., Franks, N.R.: Collective memory and spatial sorting in animal groups. J. Theor. Biol. 218(1), 1–11 (2002)

    Article  MathSciNet  Google Scholar 

  5. Degond, P., Motsch, S.: Continuum limit of self-driven particles with orientation interaction. Math. Models Methods Appl. Sci. 18(suppl), 1193–1215 (2008)

    Article  MathSciNet  Google Scholar 

  6. Degond, P., Yang, T.: Diffusion in a continuum model of self-propelled particles with alignment interaction. Math. Models Methods Appl. Sci. 20(suppl. 1), 1459–1490 (2010)

    Article  MathSciNet  Google Scholar 

  7. Degond, P., Frouvelle, A., Liu, J.-G.: Macroscopic limits and phase transition in a system of self-propelled particles. J. Nonlinear Sci. 23(3), 427–456 (2013)

    Article  MathSciNet  Google Scholar 

  8. Degond, P., Liu, J.-G., Motsch, S., Panferov, V.: Hydrodynamic models of self-organized dynamics: derivation and existence theory. Methods Appl. Anal. 20(2), 89–114 (2013)

    Article  MathSciNet  Google Scholar 

  9. Degond, P., Frouvelle, A., Liu, J.-G.: Phase transitions, hysteresis, and hyperbolicity for self-organized alignment dynamics. Arch. Ration. Mech. Anal. 216(1), 63–115 (2015)

    Article  MathSciNet  Google Scholar 

  10. Figalli, A., Kang, M.-J., Morales, J.: Global well-posedness of the spatially homogeneous Kolmogorov-Vicsek model as a gradient flow. Arch. Ration. Mech. Anal. 227(3), 869–896 (2018)

    Article  MathSciNet  Google Scholar 

  11. Frouvelle, A.: A continuum model for alignment of self-propelled particles with anisotropy and density-dependent parameters. Math. Models Methods Appl. Sci. 22(7), 1250011 (2012)

    Article  MathSciNet  Google Scholar 

  12. Frouvelle, A., Liu, J.G.: Dynamics in a kinetic model of oriented particles with phase transition. SIAM J. Math. Anal. 44(2), 791–826 (2012)

    Article  MathSciNet  Google Scholar 

  13. Gamba, I.-M., Kang, M.-J.: Global weak solutions for Kolmogorov-Vicsek type equations with orientational interactions. Arch. Ration. Mech. Anal. 222(1), 317–342 (2016)

    Article  MathSciNet  Google Scholar 

  14. Grégoire, G., Chaté, C.: Onset of collective and cohesive motion. Phys. Rev. Lett. 92, 025702 (2004)

    Article  Google Scholar 

  15. Ha, S.-Y., Jin, S.: Local sensitivity analysis for the Cucker-Smale model with random inputs. Kinet. Relat. Models 11(4), 859–889 (2018)

    Article  MathSciNet  Google Scholar 

  16. Ha, S.-Y., Jin, S., Jung, J.: A local sensitivity analysis for the kinetic Cucker-Smale equation with random inputs. J. Differ. Equ. 265(8), 3618–3649 (2018)

    Article  MathSciNet  Google Scholar 

  17. Ha, S.-Y., Jin, S., Jung, J.: A local sensitivity analysis for the kinetic Kuramoto equation with random inputs. Netw. Heterog. Media 14(2), 317–340 (2019)

    Article  MathSciNet  Google Scholar 

  18. Ha, S.-Y., Jin, S., Jung, J.: Local sensitivity analysis for the Kuramoto-Daido model with random inputs in a large coupling regime. SIAM J. Math. Anal. 52(2), 2000–2040 (2020)

    Article  MathSciNet  Google Scholar 

  19. Ha, S.-Y., Jin, S., Jung, J., Shim, W.: A local sensitivity analysis for the hydrodynamic Cucker-Smale model with random inputs. J. Differ. Equ. 268(2), 636–679 (2020)

    Article  MathSciNet  Google Scholar 

  20. Jiang, N., Xiong, L., Zhang, T.-F.: Hydrodynamic limits of the kinetic self-organized models. SIAM J. Math. Anal. 48(5), 3383–3411 (2016)

    Article  MathSciNet  Google Scholar 

  21. Jiang, N., Luo, Y.-L., Zhang, T.-F.: Coupled self-organized hydrodynamics and Navier-Stokes models: local well-posedness and the limit from the self-organized kinetic-fluid models. Arch. Ration. Mech. Anal. 236(1), 329–387 (2020)

    Article  MathSciNet  Google Scholar 

  22. Jin, S., Liu, L.: An asymptotic-preserving stochastic Galerkin method for the semiconductor Boltzmann equation with random inputs and diffusive scalings. Multiscale Model. Simul. 15(1), 157–183 (2017)

    Article  MathSciNet  Google Scholar 

  23. Jin, S., Zhu, Y.: Hypocoercivity and uniform regularity for the Vlasov-Poisson-Fokker-Planck system with uncertainty and multiple scales. SIAM J. Math. Anal. 50(2), 1790–1816 (2018)

    Article  MathSciNet  Google Scholar 

  24. Jin, S., Liu, J.-G., Ma, Z.: Uniform spectral convergence of the stochastic Galerkin method for the linear transport equations with random inputs in diffusive regime and a micro-macro decomposition based asymptotic preserving method. Res. Math. Sci. 4, 15 (2017)

    Article  MathSciNet  Google Scholar 

  25. Otto, F., Tzavaras, A.E.: Continuity of velocity gradients in suspensions of rod-like molecules. Commun. Math. Phys. 277(3), 729–758 (2008)

    Article  MathSciNet  Google Scholar 

  26. Smith, R.C.: Uncertainty Quantification: Theory, Implementation, and Applications, vol. 12. SIAM, Philadelphia (2013)

    Google Scholar 

  27. Vicsek, T., Czirók, A., Ben-Jacob, E., Cohen, I., Shochet, O.: Novel type of phase transition in a system of self-driven particles. Phys. Rev. Lett. 75(6), 1226–1229 (1995)

    Article  MathSciNet  Google Scholar 

  28. Zhang, T.F., Jiang, N.: A local existence of viscous self-organized hydrodynamic model. Nonlinear Anal., Real World Appl. 34, 495–506 (2017)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

Ning Jiang was supported by, respectively, the National Natural Science Foundation of China (Grant Nos. 11971360 and 11731008), and the Strategic Priority Research Program of Chinese Academy of Sciences (Grant No. XDA25010404). The research of Z. Zhang was supported by the grant from the National Natural Science Foundation of China under contract No. 11801425 and by the Fundamental Research Funds for the Central Universities (WUT:2019IVB043,2020IB020). The authors thank the referees for their valuable comments and suggestions.

Author information

Authors and Affiliations

  1. School of Mathematics and Statistics, Wuhan University, Wuhan, 430072, P.R. China

    Ning Jiang

  2. School of Science, Wuhan University of Technology, Wuhan, 430070, P.R. China

    Zeng Zhang

Authors
  1. Ning Jiang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zeng Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Zeng Zhang.

Additional information

Publisher’s Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Jiang, N., Zhang, Z. Local Well-Posedness and Sensitivity Analysis for the Self-Organized Kinetic Model. Acta Appl Math 176, 12 (2021). https://doi.org/10.1007/s10440-021-00457-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10440-021-00457-8

Keywords

Mathematics Subject Classification (2010)

Search

Navigation