Abstract
This paper proposes an extension of a modular modeling approach, originally developed for lumped parameter systems, to the derivation of FEM-discretized equations of motion of one-dimensional distributed parameter systems. This methodology is characterized by the use of a recursive algorithm based on projection operators that allows any constraint condition to be enforced a posteriori. This leads to a modular approach in which a system can be conceived as the top member of a hierarchy in which the increase of complexity from one level to the parent one is associated to the enforcement of constraints. For lumped parameter systems this allows the implementation of modeling procedures starting from already known mathematical models of subsystems. In the case of distributed parameter systems, such a novel methodology not only allows to explore subsystem-based modeling strategies, but also makes it possible to propose formulations in which compatibility and boundary conditions can be enforced a posteriori. The benchmark chosen to explore these further possibilities is the classical problem of a cantilevered pipe conveying fluid. Taking a pipe made of a linear-elastic material, allowing geometric nonlinearities and assuming an internal plug-flow, a Hamiltonian derivation of FEM-discretized equations of motion is performed according to this novel approach. Numerical simulations are carried out to address the nonlinear model obtained.






Similar content being viewed by others
Notes
If both \(\mathbf {S}\) and \(\mathbf {f}\) are represented in matrix form, Eq. (3) can be rewritten as follows: \({\mathbf {S}}^{{\mathrm {T}}}{\mathbf {f}}= {\mathbf {0}}\). See notation remarks at the end of this section.
As a matter of fact, after [4] stated a more general extended form of Hamilton’s principle for non-material volumes, a question arouse if McIver’s form would suffice for the problem. In Casetta and Pesce [4], the extended Hamilton’s principle is obtained with an extra term in Eq. (13), related to flux of kinetic energy, what enables recovering the Lagrange’s equation for non material volumes derived by Irschik and Holl [8]. The question on McIver’s form sufficiency was answered by [9], for the usual case in which the pipe is already completely full of liquid, as in the present paper. However, in the authors’ opinion, in the transient cases of liquid filling, starting from an empty pipe state, or of two-phase plug-flows, such a sufficiency has to be discussed further.
Even though the inextensibility constraints are not yet enforced at this stage of the modeling procedure, it is known in advance that, as soon as this enforcement is done, the virtual work corresponding to the generalized forces associated to terms in \(\varepsilon\) in the expression of the potential energy will be identically zero. Therefore, there is no loss in generality in neglecting these terms from now on.
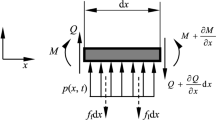
To compute these matrices from the integrals defined in the previous section, the interpolating polynomials must be extended to functions defined in the whole interval \([0, \ell ]\). This extension must be such that, outside the interval \([s_{k-1},s_k]\) for which the polynomial is originally defined, the extended function must be identically zero. Therefore, if these integrals are computed for a single generic element, the block diagonal matrices for the system can be assembled as conventionally done in FEM formulation.
It should be highlighted that once the generalized variables adopted for each node \((x_k, x'_k, z_k, z'_k)\) are assumed to be independent at level 0, a condition based on the inextensibility condition should be enforced among the \((x_{k-1}, z_{k-1})\) and \((x_{k}, z_{k})\) coordinates of two consecutive nodes. The conditions defined by Eq. (51) play such a role in the hierarchy proposed, which means that there is no over-rigidity in the constraint enforcement performed at level 3.
For long pipes, the effects of geometric stiffness (governed by the gravitational forces) are typically more relevant for determining the time scales of the dynamic response than those associated to bending stiffness. The proposed time scale based on the bending stiffness effects, however, can also be applied in the absence of axial tension [6] and is usually adopted in the literature [17].
References
Baumgarte J (1972) Stabilization of constraints and integrals of motion in dynamical systems. Comput Methods Appl Mech Eng 1(1):1–16
Benjamin TB (1961a) Dynamics of a system of articulated pipes conveying fluid. I. Theory. Proc R Soc Lond Ser A Math Phys Sci 261(1307):457LP–486LP
Benjamin TB (1961b) Dynamics of a system of articulated pipes conveying fluid. II. Experiments. Proc R Soc Lond Ser A Math Phys Sci 261(1307):487LP–499LP
Casetta L, Pesce CP (2013) The generalized Hamilton’s principle for a non-material volume. Acta Mech 224(4):919–924. https://doi.org/10.1007/s00707-012-0807-9
Gregory RW, Païdoussis MP (1966a) Unstable oscillation of tubular cantilevers conveying fluid. I. Theory. Proc R Soc Lond Ser A Math Phys Sci 293(1435):512LP–527LP
Gregory RW, Païdoussis MP (1966b) Unstable oscillation of tubular cantilevers conveying fluid. II. Experiments. Proc R Soc Lond Ser A Math Phys Sci 293(1435):528LP–542LP
Ibrahimbegovic A (2009) Nonlinear solid mechanics: theoretical formulations and finite element solution methods. Solid mechanics and its applications. Springer, Dordrecht
Irschik H, Holl HJ (2002) The equations of Lagrange written for a non-material volume. Acta Mech 153(3):231–248. https://doi.org/10.1007/BF01177454
Kheiri M, Païdoussis MP (2014) On the use of generalized Hamiltons principle for the derivation of the equation of motion of a pipe conveying fluid. J Fluids Struct 50:18–24. https://doi.org/10.1016/j.jfluidstructs.2014.06.007
McIver DB (1973) Hamilton’s principle for systems of changing mass. J Eng Math 7(3):249–261. https://doi.org/10.1007/BF01535286
Orsino RMM (2016) A contribution on modeling methodologies for multibody systems. Ph.D. thesis, Universidade de São Paulo
Orsino RMM (2017) Recursive modular modelling methodology for lumped-parameter dynamic systems. Proc R Soc A Math Phys Eng Sci 471(2183):1–16. https://doi.org/10.1098/rspa.2016.0891
Orsino RMM, Hess-Coelho TA (2015) A contribution on the modular modelling of multibody systems. Proc R Soc A Math Phys Eng Sci 471(2183):1–20. https://doi.org/10.1098/rspa.2015.0080
Orsino RMM, Pesce CP (2017a) Modular approach for the modeling and dynamic analysis of a pipe conveying fluid. In: 9th European nonlinear dynamics conference, ENOC 2017, Budapest, Hungary
Orsino RMM, Pesce CP (2017b) Novel modular modeling methodology applied to the problem of a pipe conveying fluid. In: XVII international symposium on dynamic problems of mechanics, DINAME 2017, São Sebastião, São Paulo, Brazil
Orsino RMM, Coutinho AG, Hess-Coelho TA (2016) Dynamic balancing of mechanisms and synthesizing of parallel robots SE-16. In: Zhang D, Wei B (eds) Dynamic modelling and control of balanced parallel mechanisms. Springer International Publishing, Switzerland. https://doi.org/10.1007/978-3-319-17683-3
Païdoussis MP (2014) Fluid–structure interactions: slender structures and axial flow, vol 1. Academic Press, Elsevier Science, London
Papastavridis JG (1988) On the nonlinear Appell’s equations and the determination of generalized reaction forces. Int J Eng Sci 26(6):609–625. https://doi.org/10.1016/0020-7225(88)90058-4
Udwadia FE, Kalaba RE (1992) A new perspective on constrained motion. Proc Math Phys Sci 439(1906):407–410
Udwadia FE, Kalaba RE (2002) On the foundations of analytical dynamics. Int J Nonlinear Mech 37(6):1079–1090
Yoshizawa M, Nao H, Hasegawa E, Tsujioka Y (1985) Buckling and postbuckling behavior of a flexible pipe conveying fluid. Bull JSME 28(240):1218–1225. https://doi.org/10.1299/jsme1958.28.1218
Yoshizawa M, Nao H, Hasegawa E, Tsujioka Y (1986) Lateral vibration of a flexible pipe conveying fluid with pulsating flow. Bull JSME 29(253):2243–2250. https://doi.org/10.1299/jsme1958.29.2243
Acknowledgements
Authors thank Dr. Guilherme Rosa Franzini and Prof. Luiz Bevilacqua for encouraging discussions. First author acknowledges the post-doctoral Grant #2016/09730-0, São Paulo Research Foundation (FAPESP). Second author acknowledges CNPq research Grant no. 308990/2014-5.
Author information
Authors and Affiliations
Corresponding author
Additional information
Technical Editor: Pedro Manuel Calas Lopes Pacheco.
This paper is an extended version of the work originally presented by Orsino and Pesce [15] in the XVII International Symposium on Dynamic Problems of Mechanics - DINAME 2017.
Appendix A: Routine used for numerical simulations
Appendix A: Routine used for numerical simulations
This appendix details the routine used for the numerical simulations presented in this paper. The constraint enforcement algorithm used is based on the application of the modular modeling methodology to produce a recursive form of Udwadia-Kalaba equation. In this case, the projection operator onto the kernel of \(\mathbf {B}_{r+1}\) (see Sect. 1) is obtained by \(\mathbf {C}_{r+1} = (\mathbf {I}- \mathbf {B}_{r+1}^{+} \mathbf {B}_{r+1})\), with \(\mathbf {I}\) representing an identity operator and \((\cdot )^{+}\) standing for the Moore–Penrose pseudo-inverse. [12] proved that an algorithm based on such a procedure can be put in the explicit form detailed below, which is analogous to the algorithm of a Kalman filter for estimating the state of a static process from a set of noiseless measurements.
Consider that the rows and columns of the coefficient matrices in Eq. (35) are reordered according to the order of the generalized coordinates in the column-matrix \(\mathbf{q}\) introduced in Eq. (37). Assuming U as a constant, the equations of motion of the system if neither compatibility nor boundary conditions are considered can be rewritten as a single matrix equation as follows:
Let \(\mathbf {N}= \mathbf {M}^{-1/2}\) and define \(\mathbf {z}_0\) as:
It should be noticed that the values of \(\ddot{\mathbf{q}}\) associated to the level 0 of the hierarchical representation of the problem shown in Fig. 1 are given by \(\ddot{\mathbf{q}}= \mathbf {N}\mathbf {z}_{0}\). As shown in Orsino [12], the variables \(\mathbf {z}_{r}\), from which the generalized accelerations at the level r of the hierarchy could be determined as \(\ddot{\mathbf{q}}= \mathbf {N}\mathbf {z}_{r}\), are computed according to the following algorithm (\(r=0,1,2\)):
In these equations \(\tilde{\mathbf {H}}_{r} = \tilde{\mathbf {A}}_{r} \mathbf {N}\), and \(\tilde{\mathbf {A}}_{r}\) and \(\tilde{\mathbf {b}}_{r}\) correspond to coefficient matrices associated the second time derivatives of the constraint equations specifically enforced at level r. Indeed, denote by \(\tilde{\mathbf {h}}_{r}(\mathbf{q}) = \mathbf {0}\) the aforementioned equations expressed in matrix form. Their first two time derivatives can be expressed as follows:
Therefore, the computational routine adopted basically consists of performing a numerical integration using, at each step, the values of generalized accelerations computed by \(\ddot{\mathbf{q}}= \mathbf {N}\mathbf {z}_{3}\). To apply Baumgarte’s technique [1] for stabilization of constraints, Eq. (68) can be replaced by:
For the simulations shown, the following values for the stabilization constants were chosen: \(\zeta = \sqrt{2}/2\) and \(\omega = 10\).
Rights and permissions
About this article
Cite this article
Orsino, R.M.M., Pesce, C.P. Modular methodology applied to the nonlinear modeling of a pipe conveying fluid. J Braz. Soc. Mech. Sci. Eng. 40, 67 (2018). https://doi.org/10.1007/s40430-018-0994-y
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s40430-018-0994-y