Type ${{\varvec{D}}}_{{\varvec{n}}}^\mathbf{(1)}$ rigged configuration bijection

Abstract

We establish a bijection between the set of rigged configurations and the set of tensor products of Kirillov–Reshetikhin crystals of type $D^{(1)}_n$ in full generality. We prove the invariance of rigged configurations under the action of the combinatorial R-matrix on tensor products and show that the bijection preserves certain statistics (cocharge and energy). As a result, we establish the fermionic formula for type $D_n^{(1)}$. In addition, we establish that the bijection is a classical crystal isomorphism.

Appendix: Example of the rigged configuration bijection

In this section, we provide an example of the algorithm $\Phi ^{-1}$. The reader may easily infer from the following example the meaning of the correspondence between the operators summarized in Table 1.

Example 7.1

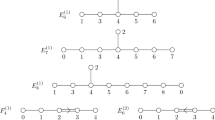
Consider the unrestricted rigged configuration $r_1 \in {\text {RC}}(L(B))$ for $B=B^{3,2}\otimes B^{3,3}\otimes B^{2,3}$ of type $D^{(1)}_5$:

In the above diagram, we show the partition $\mu ^{(a)}$ as defined in Sect. 3.1 over the corresponding rigged partition $(\nu ^{(a)},J^{(a)})$ in order to make it easier to see the operations $\gamma$ and $\beta$. In $\Phi ^{-1}$, let us remove the $B^{3,2}$ part first. We begin by applying $\gamma$ and obtain $r_2{:}{=}\gamma (r_1)$ which looks as follows:

The changes are the shape of $\mu ^{(3)}$ and the resulting change of the vacancy numbers for $P^{(3)}_1(\nu )$ which makes the length 1 strings of $(\nu ,J)^{(3)}$ non-singular.¹ This operation corresponds to

$$\begin{aligned} {\text {ls}}:B^{3,2}\longrightarrow B^{3,1}\otimes B^{3,1}. \end{aligned}$$

(7.1)

Then $r_3{:}{=}\beta (r_2)$ looks as follows:

This corresponds to

$$\begin{aligned} {\text {lb}} :B^{3,1}\longrightarrow B^{1,1}\otimes B^{2,1}. \end{aligned}$$

(7.2)

Note that the vacancy numbers for $\nu ^{(3)}$ do not change. Since $\beta$ adds length 1 singular strings to $(\nu ,J)^{(1)}$ and $(\nu ,J)^{(2)}$, applying $\delta$ removes the boxes with “$\times$” in the above diagram.² Then $\delta$ gives the following rigged configuration $r_4{:}{=}\delta (r_3)$ together with the output letter $\overline{5}$ which fills the bottom left corner of $B^{3,2}$ as

.

Since the above $\delta$ determines $B^{1,1}$ of (7.2), we start to apply $\gamma$, $\beta$, and $\delta$ corresponding to $B^{2,1}$ of (7.2). Since $\gamma (r_4) = r_4$, the unrestricted rigged configuration $r_5 {:}{=} \beta (r_4)$ looks as follows:

This corresponds to

$$\begin{aligned} {\text {lb}}:B^{2,1}\longrightarrow B^{1,1}\otimes B^{1,1}. \end{aligned}$$

(7.3)

Then $\delta$ removes the boxes with “$\times$” in the above diagram which determines one of $B^{1,1}$ in (7.3). As the result, we obtain the following unrestricted rigged configuration $r_6{:}{=}\delta (r_5)$ and the output letter within $B^{3,2}$ as

:

The next $\delta$ removes the box with “$\times$” in the above diagram and determines the remaining $B^{1,1}$ in (7.3). As the result, we obtain the following unrestricted rigged configuration $r_7 {:}{=} \delta (r_6)$ and the output letter within $B^{3,2}$ as

:

Next we determine the remaining $B^{3,1}$ in (7.1). $r_8 = (\delta \circ \beta )(r_7)$ is the following unrestricted rigged configuration with the output letter in $B^{2,3}$ as

:

$r_9 = (\delta \circ \beta )(r_8)$ is the following unrestricted rigged configuration with the output letter in $B^{2,3}$ as

:

$r_{10} = \delta (r_9)$ is the following unrestricted rigged configuration with the output letter in $B^{2,3}$ as

:

For the KN tableau representation of the rectangular tableau, we need to apply the inverse of the filling map of Definition 2.5 to obtain

. If we further determine $B^{3,3}$ and $B^{2,3}$ in this order, we obtain the empty rigged configuration and the following path

Summary As we see in the above example, the algorithm $\Phi ^{-1}$ is recursively defined as follows. Suppose that we consider the unrestricted rigged configuration $(\nu ,J)$ associated with the tensor product of type

$$\begin{aligned} B=B^{r,s}\otimes B'. \end{aligned}$$

Then we determine $b\in B^{r,s}$ by the following procedure. In correspondence with the operation

$$\begin{aligned} {\text {ls}}:B^{r,s} \longrightarrow B^{r,1}\otimes B^{r,s-1}, \end{aligned}$$

(7.4)

we apply $\gamma$ to the unrestricted rigged configuration. Then apply ${\text {lb}}$ on $B^{r,1}$ in (7.4)

$$\begin{aligned} {\text {lb}}:B^{r,1}\longrightarrow B^{1,1}\otimes B^{r-1,1}, \end{aligned}$$

(7.5)

which on the rigged configuration side corresponds to $\beta$. Finally we use ${\text {lh}}$ and $\delta$ to remove $B^{1,1}$ of (7.5). In order to process the remaining $B^{r-1,1}$ of (7.5), we apply $\beta$ and $\delta$ repeatedly for $(r-1)$-times. We fill the leftmost column of $B^{r,s}$ from bottom to top by the letters obtained by $\delta$ during this procedure. In order to determine the remaining $B^{r,s-1}$ of (7.4), we repeat the same procedure for the first column and fill the remaining columns of $B^{r,s}$ from left to right. Once $B^{r,s}$ is fully determined, we proceed to the leftmost rectangle of $B'$ and repeat the same procedure used for $B^{r,s}$. In this manner, we obtain the filling of shape B, which we denote by $b\in B$. Then we define

$$\begin{aligned} \Phi ^{-1}:(\nu ,J)\longmapsto b. \end{aligned}$$

The inverse procedure $\Phi$ is obtained by reversing all the steps of $\Phi ^{-1}$.