Abstract
After reading this chapter you know:
-
what the three main trigonometric ratios are and how they can be calculated,
-
how to express angles in degrees and radians,
-
how to sketch the three main trigonometric functions,
-
how to sketch functions of the form A sin(Bx + C) + D and how to interpret A, B, C and D,
-
what Fourier (or spectral or harmonic) analysis is and
-
how Fourier analysis is used in real-life examples.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
References
Online Sources of Information: Methods
http://galileo.rice.edu/sci/theories/ptolemaic_system.html (Epicycles)
Unbaking a cake by Carl Joseph (Alternative non-mathematical Fourier series explanation) https://www.youtube.com/watch?v=Qm84XIoTy0s&feature=youtu.be
http://math.stackexchange.com/questions/1002/fourier-transform-for-dummies (thread on relation between Epicycles and Fourier analysis)
Books
Otto Neugebauer – The exact sciences in antiquity. Courier Corporation, 1969. Available from books.google.com.
Papers
F.A. Farris, Wheels on wheels on wheels – surprising symmetry. Math. Mag. 69(3), 185–189 (1996)
D.B. Plewes, W. Kucharczyk, Physics of MRI: a primer. J. Magn. Res. Imaging 35, 1038–1054 (2012)
Author information
Authors and Affiliations
Corresponding author
Appendices
Symbols Used in This Chapter (in Order of Their Appearance)
sin | Sine |
cos | Cosine |
tan | Tangent |
\( \underset{x\to 0}{\lim \limits } \) | Limit for x approaching zero |
α,θ,ϕ | Angles |
r,R | Radius (of a circle) |
rad | Radian |
e | Exponential |
i | Imaginary unit |
∑ | Sum |
∫ | Integral |
f | Frequency |
t | Time |
ω | Angular frequency |
Overview of Equations, Rules and Theorems for Easy Reference
Mnemonic for memorizing trigonometric ratios
sine = O/H
cosine = A/H
tangent = O/A = sine/cosine
where O = opposite, A = adjacent, H = hypothenuse
Angles and values to remember
x in rad | π/6 | π/4 | π/3 | π/2 | π |
|---|---|---|---|---|---|
sin(x) | 0.5 | \( \frac{1}{2}\sqrt{2} \) | \( \frac{1}{2}\sqrt{3} \) | 1 | 0 |
Euler’s formula
Trigonometric relations
Answers to Exercises
-
3.1.
The ratios must be calculated for the angle θ, so the sine, e.g., is always the length of the edge opposite from θ divided by the length of the hypothenuse etcetera. Sometimes rotating the triangles in your mind to relate them to the triangles in Fig. 3.3 will help. Thus the answers are for the left triangle: sine = 4/5, cosine = 3/5, tangent = 4/3, middle triangle: sine = 4/7, cosine = √33/7, tangent = 4/√33, right triangle: sine = 3/5, cosine = 4/5, tangent = 3/4.
-
3.2
First the lengths of the edges of the two triangles that angles 1 to 4 belong to must be determined. For angle 1, the adjacent edge is 75 cm long; this is the opposite edge for angle 2. For angle 2, the adjacent edges is 45 cm long; this is the opposite edge for angle 1. Similarly, the adjacent edge for angle 3 (opposite edge angle 4) is 65 cm long and the adjacent edge for angle 4 (opposite edge angle 3) is 35 cm long. The angles can now be determined by using the trigonometric ratio for the tangent: tan = O/A. Thus, \( \tan \angle 1=\frac{45}{75} \), \( \tan \angle 2=\frac{75}{45} \), \( \tan \angle 3=\frac{35}{65} \) and \( \tan \angle 4=\frac{65}{35} \). A calculator can now be used to determine the angles using the inverse tangent (or atan) function.
-
3.3
-
(a)
30° = 30*2π/360 = π/6 rad
-
(b)
45° = 45*2π/360 = π/4 rad
-
(c)
60° = 60*2π/360 = π/3 rad
-
(d)
80° = 80*2π/360 = 4/9 π rad
-
(e)
90° = 80*2π/360 = π/2 rad
-
(f)
123° = 123*2π/360 = 123/180 π rad
-
(g)
(g) 260° = 260*2π/360 = 13/9 π rad
-
(h)
(h) -16° = −16*2π/360 = −4/45 π rad
-
(i)
-738° = −738 + 2*360° = −18° = −18*2π/360 = −1/10 π rad
-
(a)
-
3.4.
-
(a)
2π/3 rad = 2π/3*360/2π° = 120°
-
(b)
π/4 rad = π/4*360/2π° = 45°
-
(c)
9π/4 rad = 9π/4-2π rad = π/4*360/2π° = 45°
-
(d)
0.763π rad = 0.763 π *360/2π° = 137,34°
-
(e)
π rad = π*360/2π° = 180°
-
(f)
θπ rad = θπ*360/2π° = 180θ°
-
(g)
θ rad = θ*360/2π° = 180θ/π°
-
(a)
-
3.5.
-
(a)
false: cos 0° = cos (0 rad) = 1
-
(b)
false: sin 30° = sin (π/6 rad) = 0.5
-
(c)
false: sin 45° = sin (π/4 rad) = \( \frac{1}{2}\sqrt{2} \)
-
(d)
true
-
(e)
false: cos 60° = cos(π/3 rad) = 0.5
-
(a)
-
3.6.
For the angle of 60° the length of the opposite edge and the hypothenuse are x and 13, respectively. These two values can thus be used to calculate the sine of this angle by their ratio. But the sine of this angle is also equal to sin(60°) = sin(π/3 rad) = \( \frac{1}{2}\sqrt{3} \). Thus x/13 = \( \frac{1}{2}\sqrt{3} \), or x = \( \frac{13}{2}\sqrt{3} \).
-
3.7.
See Fig. 3.8 for the graphs of sin(x), cos(x) and tan(x). The exact values for the angles 0, 30, 45, 60 and 90 degrees (that help you make these sketches) are:
degrees
rad
sin
cos
tan
0
0
0
1
0
30
π/6
0.5
\( \frac{1}{2}\sqrt{3} \)
\( \frac{1}{3}\sqrt{3} \)
45
π/4
\( \frac{1}{2}\sqrt{2} \)
\( \frac{1}{2}\sqrt{2} \)
1
60
π/3
\( \frac{1}{2}\sqrt{3} \)
0.5
\( \sqrt{3} \)
90
π/2
1
0
∞
-
3.8.
-
(a)
cos(−2x) = cos(2x)
-
(b)
tan(−π/4) = −tan(π/4)
-
(c)
sin(−4π/3) = −sin(4π/3)
-
(a)
-
3.9.
sin(x): black, sin(2x): green, sin(x) + 2: red, sin(x + 2): magenta and 2 sin(x): blue.
-
3.10.
-
(a)
For sketching y = 4sin(x), first make a table, with easily obtained values (making use of Table 3.1 and the trigonometric symmetries), e.g.:
x
y
-π ≅ − 3.14
0
-π/2
−4
-π/3
-\( 2\sqrt{3} \) ≅ − 3.46
0
0
π/3
\( 2\sqrt{3} \) ≅ 3.46
π/2
4
π
0
Then sketch an x- and a y-axis to scale that are long enough to accommodate for the maximum (4) and minimum (−4) of the function and for at least one period (2π ≅ 6.28) of the function. Note that you are free to choose the scale of the axes. Put in the points you calculated in the table and sketch a smooth line through the points. Such a sketch could look like:

Note that a sketch of a trigonometric function does not need to be limited to the positive x-axis: I here sketched it for both negative and positive values of x and also for more than one period.
-
(b)
For sketching y = sin(4x), first make a table, with easily obtained values (making use of Table 3.1 and the trigonometric symmetries), e.g.:
x
y
-π/4
0
-π/8
−1
-π/12
-\( \frac{1}{2}\sqrt{3} \) ≅ − 0.87
0
0
π/12
\( \frac{1}{2}\sqrt{3} \) ≅ 0.87
π/8
1
π/4
0
Then sketch an x- and a y-axis to scale that are long enough to accommodate for the maximum (1) and minimum (−1) of the function and for at least one period (π/2 ≅ 1.57) of the function. Put in the points you calculated in the table and sketch a smooth line through the points. Such a sketch could look like:

-
(a)
-
3.11.
-
(a)
Following a similar procedure as in Exercise 3.10 a sketch of y = 2 + sin(x) could look like:
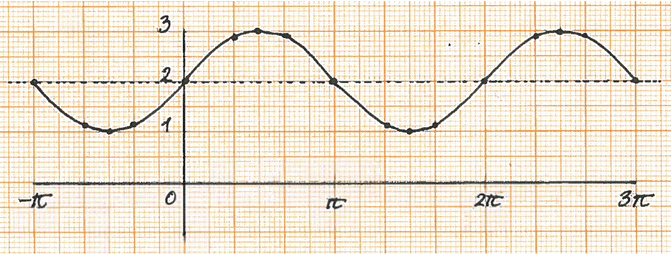
-
(b)
Similarly, a sketch of y = − cos(x) could look like:
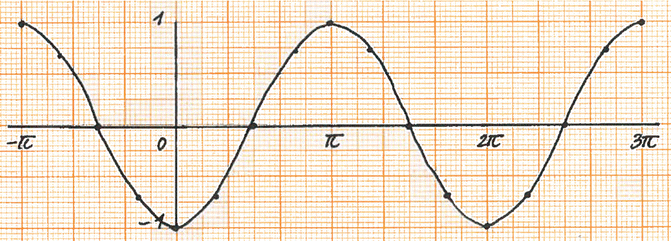
-
(a)
-
3.12.
-
(a)
y = − 3 sin(x) − 5 is obtained from the standard sine function by multiplying it by −3 and shifting it down by 5.
-
(b)
y = 2 sin(3x) is obtained by multiplying it by 2 and changing the period to 2π/3 (if this last part is hard to grasp: the period is that number that you fill in for x, making the total between the brackets equal to 2π).
-
(c)
y = 2 sin(x + π) + 2 is obtained by multiplying it by 2, shifting it up by 2 and shifting it to the left by π (again, it is to the left and not to the right because when I fill in -π, the value between brackets becomes 0).
-
(d)
\( y=3\sin \left(2x-\frac{\pi }{2}\right)-1 \) is obtained by multiplying it by 3, shifting it down by 1, changing the period to π and shifting it to the right by π/2.
-
(e)
\( y=-4\sin \left(\frac{\pi x}{5}\right) \) is obtained by multiplying it by −4 and changing the period to 10.
-
(f)
y = − x + 3 sin(2x − π) is obtained by multiplying it by 3, changing the period to π, shifting it to the right by π and finally by plotting this whole function around the line y = − x. It will look like:
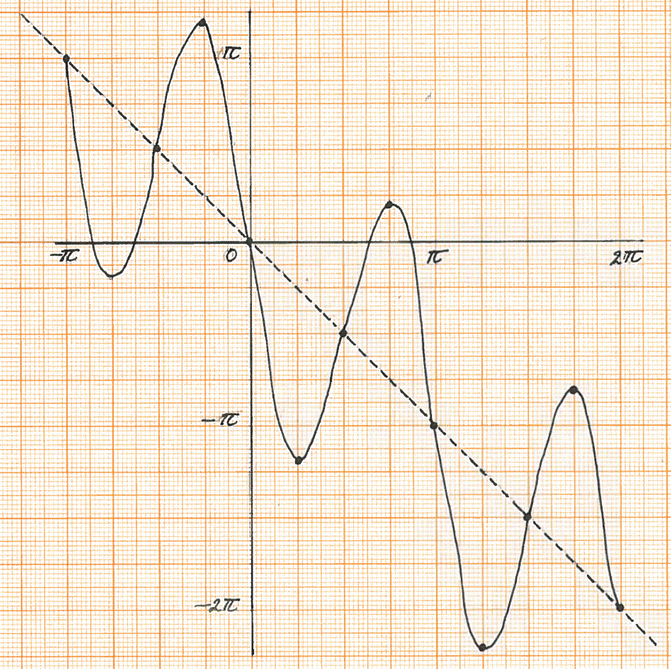
-
(a)
-
3.13.
The FFT of
-
(a)
a sine with frequency 3 Hz looks like:
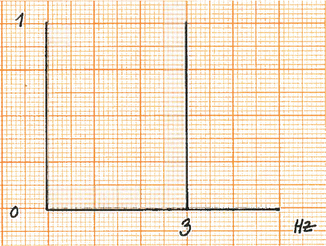
-
(b)
the sum of two sines (2 Hz and 5 Hz) looks like:
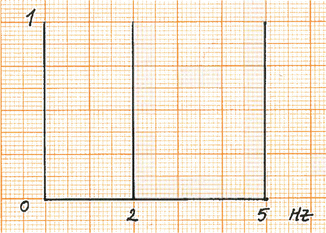
-
(c)
white noise looks like (random spectrum):
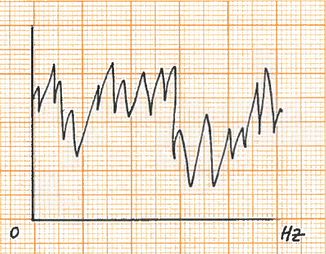
-
(d)
50 Hz noise looks like:
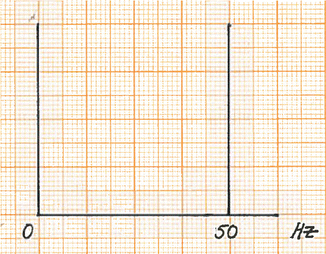
-
(a)
Glossary
- Accelerometry
-
Measurement of acceleration using accelerometers (sensor device).
- Antisymmetric
-
In this chapter: a function f for which f(−x) = − f(x).
- Cosine
-
(trigonometric) Ratio between the adjacent edge and the hypothenuse in a right-angled triangle.
- Data compression
-
To represent data such that it occupies less memory.
- Deferent
-
In specific movements described by epicycles and deferents the larger circle that an object moves along.
- Delta function
-
A function that is zero everywhere, except at zero, with an integral of 1 over the entire real domain.
- Diadochokinesis
-
Fast execution of opposite movements, e.g. rotating the hand from palm up to palm down and back.
- Enhanced physiological tremor
-
Tremor that occurs normally, e.g. when fatigued, but stronger in amplitude.
- Electroencephalography
-
Measurement of electrical brain activity using electrodes placed on the scalp.
- Electromyography
-
Measurement of electrical muscle activity using electrodes placed on the skin over a muscle (formally: surface electromyography).
- Epicycle
-
In specific movements described by epicycles and deferents the smaller circle that an object moves along.
- Essential tremor
-
Patients with this type of tremor typically present with action tremor (tremor during posture and/or movements), mostly in the arms, with tremor frequency in the range of 4–12 Hz.
- Filtering
-
Suppression of specific frequencies in a signal.
- Function
-
A mathematical relation, like a recipe, describing how to get from an input to an output.
- Functional tremor
-
Tremor for which no organic cause can be found.
- Hypothenuse
-
Edge opposite the right angle in a right-angled triangle.
- Modulo
-
Modulo(n) expresses equivalence up to a remainder n.
- Nyquist frequency
-
The highest frequency that is still adequately represented in a sampled signal (half the sampling frequency).
- Periodic
-
Repeating itself regularly.
- Right-angled (triangle)
-
A geometric object (triangle) with a right (90°) angle.
- Similar (triangles)
-
Triangles that have the same angles but differ in size.
- SI
-
Système international d’unités (international system of units).
- Sine
-
(trigonometric) Ratio between the opposite edge and the hypothenuse in a right-angled triangle.
- Spectrum
-
Here: representation of a function or signal as a function of frequency, typically resulting from a Fourier transform.
- Support
-
The support of a function is the set of points where the function values are non-zero.
- Symmetric
-
In this chapter: a function f for which f(−x) = f(x).
- Tangent
-
(trigonometric) Ratio between the opposite edge and the adjacent edge in a right-angled triangle.
- Transfer function
-
Here: expresses how strongly frequencies are preserved or suppressed by a filter, can vary between 0 and 1.
- Tremor
-
Oscillatory movement of a body part.
- Unit circle
-
Circle with radius one.
Rights and permissions
Copyright information
© 2017 Springer International Publishing AG
About this chapter
Cite this chapter
Maurits, N. (2017). Trigonometry. In: Math for Scientists. Springer, Cham. https://doi.org/10.1007/978-3-319-57354-0_3
Download citation
DOI: https://doi.org/10.1007/978-3-319-57354-0_3
Published:
Publisher Name: Springer, Cham
Print ISBN: 978-3-319-57353-3
Online ISBN: 978-3-319-57354-0
eBook Packages: Mathematics and StatisticsMathematics and Statistics (R0)










