Are Gatekeepers Important for the Renewal of the Local Knowledge Base? Evidence from U. S. Cities

Abstract

The authors offer an explorative perspective on the importance of gatekeepers for the expansion and renewal of U.S. cities’ knowledge base. They propose and test a number of indicators accounting for the role of gatekeepers in mediating knowledge flows across cities. Findings indicate that external direct relations are the key mechanism to inject fresh knowledge into a city and to amplify technological recombination opportunities; conversely, the greater the reliance on external relations governed by gatekeepers the lower the impact on a city knowledge base expansion and renewal.

The Role of Gatekeepers for Knowledge Renewal: Review of the Literature and Research Questions

Recent research on the role of social networks for the creation and the spatial diffusion of scientific and technological knowledge has increasingly emphasized the importance of connecting and combining spatially dispersed sources of knowledge (Boschma & Frenken, 2010). Openness to global sources of knowledge provides some shelter from the risk of over-embeddedness, of lock-in to obsolete sets of technologies, of decrease in the variety of technological approaches and solutions, and of redundancy of localized knowledge exchanges by favoring a continuous expansion, rejuvenation, and update of the existing knowledge base (Bathelt, Malmberg, & Maskell, 2004; Uzzi, 1996).

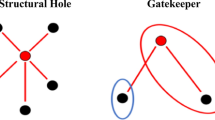
In this regard, recent research has concentrated on the role played by specific actors in local networks, the so-called gatekeepers, by investigating their characteristics, attributes, and performances. The literature on industrial clusters has been particularly successful in emphasizing the crucial interfacing functions gatekeepers perform between the local and the external knowledge systems, such as screening external sources, accessing them, and conveying new knowledge to local actors (Giuliani & Bell, 2005; Graf, 2011).

Following the original definition put forward by Gould and Fernandez (1989), gatekeeping is a specific form of brokerage that corresponds to structural position in transaction networks, in which “an actor can selectively grant outsiders access to members of his or her own group” (p. 92). By extension, in regional studies gatekeepers are generally understood to be individuals (and sometimes organizations) that enable knowledge transfer among different spatial units, e.g., industrial clusters, cities, or regions (Giuliani & Bell, 2005; Graf & Krüger, 2011; Morrison, 2008; Morrison, Rabellotti, & Zirulia, 2013). The uniqueness of this function rests on two specific features: (a) Gatekeepers establish exclusive linkages with outside actors and/or knowledge sources and (b) they guarantee knowledge transfer and absorption within their proximate working and social environments. In short, gatekeepers not only can search for and collect relevant information outside the professional and social contexts in which they are embedded, but they are also able to transcode this information and diffuse it within their organizations and geographical areas.

Several distinctive attributes enable gatekeepers to perform this fundamental activity: high productivity and performance (Burt, 1992); creativity; novel points of view that allow new solutions (Burt, 2004; Fleming, Mingo, & Chen, 2007; Hargadon & Sutton, 1997; Obstfeld, 2005); and maintaining influential positions in their respective social structures (Fernandez & Gould, 1994; Padgett & Ansell, 1993). These characteristics can give gatekeepers comparative advantages with respect to other network members, leading to higher (private) economic and innovative outcomes, as well as to greater control power over (and relative accruable rents from) the bridging ties and knowledge exchanges they enable between internal and external actors (Burt, 2008; Gould & Fernandez, 1989).

This bright side, however, may also entail a dark side. In particular, a gatekeeper can strategically choose whether to grant access or block information flows from outside. Albeit an individual gatekeeper’s impact may be slight, this possibility can have different and sizeable effects at the aggregate level of analysis, such as the regional one. In support of this line of argumentation, recent research has documented that gatekeepers can purposefully restrict the diffusion and circulation of valuable knowledge, as in the case of certain leading firms in industrial clusters (Giuliani & Bell, 2005; Hervas-Oliver & Albors-Garrigos, 2014; Morrison, 2008; Morrison et al., 2013).

In addition, gatekeepers can experience coordination costs and difficulties in managing and matching multiple external and internal connections, with a negative impact on the amount and efficiency of information flows (Whittington, Owen-Smith, & Powell, 2009). The increasing complexity of processing, coding, interpreting, and absorbing large amount of information from multiple sources (Dahlander & Frederiksen, 2012) can also hamper the efficiency of knowledge exchanges. In general, social structures in which gatekeepers dominate external linkages (and the related knowledge flows) are more exposed to disruptions of such links than social structures whose external ties are mostly direct, and possibly redundant. Finally, and more generally, direct linkages avoid leakages and noise in knowledge transmission , whereas longer chains of intermediaries imply slower knowledge transfer and a higher risk of distortion of the message content (Tushman & Scanlan, 1981).

The extant literature therefore suggests that openness and external ties are crucial for the renewal and expansion of the local knowledge base and, more generally, for innovation and creativity. However, it also suggests that, despite the importance often assigned to gatekeepers regarding the access and transfer of external knowledge (Graf, 2011; Munari, Sobrero, & Malipiero, 2012), their role is not unequivocally and unambiguously positive. Some research questions are thus still open. For example, is the open-mindedness, creativity, and innovativeness of gatekeepers sufficient to make the relations they mediate a more effective channel for knowledge transmission than direct external links? Are the disadvantages of the slower and noisier access to knowledge associated with gatekeepers compensated by the advantages of translation and transcoding of external information necessary for the successful transfer and application of externally sourced knowledge at the local level? In short, are all external relations alike with respect to expanding and renewing the existing local knowledge base?

We aim to offer an exploratory investigation of these issues and a preliminary assessment of the importance of gatekeepers for the renewal and expansion of the local knowledge base. We distinguish conceptually between direct external ties and external linkages mediated by gatekeepers and test their relative effectiveness as knowledge transfer channels. For this purpose, we propose a methodological approach to identifying and measuring the different types of external relations. In so doing, we also supply a methodological contribution to the modeling of the structure of external relations and the channels through which external knowledge flows into a city.

The empirical analysis was conducted on a large dataset of patents and their inventors in 196 U.S. Metropolitan Statistical Areas (MSAs) in the period 1990–2004. Urban settings particularly suit the study of the relationship between knowledge network properties and innovation, because invention in the United States has always been a predominantly metropolitan phenomenon (Carlino, Chatterjee, & Hunt, 2007).

In the next section, we discuss the construction of appropriate indicators to capture the intensity of a city’s external linkages, the identification of gatekeepers, and of their importance in mediating external knowledge flows. We then describe our data sources and the econometric framework. The results of the empirical analysis are presented in section results, followed by our conclusions.

Measuring the Contribution of Gatekeepers to External Linkages

In this chapter, we use patents as relational data and apply the tools of social network analysis in analyzing the impact of social networks on innovation, as recent literature suggests (Breschi & Lissoni, 2009; Ter Wal & Boschma, 2009). In particular, inventors are regarded as nodes of a network, with co-invention (namely, the designation of multiple inventors on the same patent) representing the link between nodes.

Specifically, in this chapter we use all patent applications made by U.S. organizations at the European Patent Office (EPO) from 1990 to 2004 recorded in the CRIOS-PATSTAT database. Names and addresses of inventors have been thoroughly cleaned and standardized, because the accurate identification of individual inventors is key to a correct application of social network analysis tools. Inventors’ addresses have been linked to one of the 370 U.S. Metropolitan Statistical Areas (MSAs), using the delineation files available on the U.S. Census Bureau website (specifically, the June 2003 delineations issued by U.S. Office of Management and Budget).

As customary in the literature, the co-invention network is constructed on the basis of a 5-year moving window, because the effectiveness of knowledge transmission through a network’s ties decay with its age.

The intensity of connection of metropolitan inventors with inventors external to the city is measured through the average distance-weighted external reach (ADWR) between inventors located in a given city and all other inventors located in all other cities. In particular, for an individual inventor i, it is defined as the sum of the reciprocal distances to all other inventors he/she can reach in the co-invention network.¹ Accordingly, the average distance-weighted external reach of city c ($ADW{R}_c$) is the distance-weighted external reach averaged across all inventors located in the city. Formally, this index is defined as follows:

$$ ADW{R}_c=\frac{{{\displaystyle \sum}}_{i=1}^{n_c}{{\displaystyle \sum}}_{j=1}^{n_h}\frac{1}{d_{ij}}}{n_c} $$

(14.1)

where ${n}_c$ is the number of inventors located in city c and ${n}_h$ is the number of inventors located in other cities (i.e., not located in city c), and ${d}_{ij}$ is the geodesic distance (i.e., shortest path) in the U.S. co-invention network between inventor $i$ (located in city c) and inventor $j$ (not located in city c).²

The index ranges from zero (i.e., all inventors in city $c$ do not collaborate with any external inventor) to ${n}_h$ (i.e., when every inventor directly collaborates with every other inventor in every other city).³ As the literature suggests, we expect that a higher $ADW{R}_c$ has a positive impact on the rate of expansion and renewal of a city’s knowledge base.

However, this aggregate indicator encompasses different types of external relations. In this paper, we make an effort to separate out different types of relationships between local and external inventors. In particular, we can classify external linkages as direct, whenever a local inventor is directly connected to an external inventor, and indirect, whenever a local inventor needs the intermediation of another co-localized inventor to reach external inventors.

As far the indirect linkages are concerned, these are defined as those shortest paths between inventors such that the geodesic distance ${d}_{ij}$between inventor $i$(located in focal city c) and inventor $j$ (not located in city c) goes through some other inventor also located in city c. Accordingly, the latter inventor can be defined as a gatekeeper, in the sense this inventor performs a bridging function between inventors located in a given city and other inventors located in other cities. This definition of gatekeeper is consistent with the original one proposed by Gould and Fernandez (1989), Allen (1977), and Tushman and Katz (1980). If we take all indirect linkages defined in this way and we aggregate them up to the city level, we can formally define the overall indirect reach of city $c$as:

$$ INDREAC{H}_c={\displaystyle \sum}_{i=1}^{n_c}{\displaystyle \sum}_{j=1}^{n_h}\frac{1}{d_{ij}^{ind}} $$

(14.2)

where ${d}_{ij}^{ind}$ is the distance in the co-invention network between inventor $i$ (in city c) and inventor j (not in city c) and the apex “$ind$” indicates that the shortest paths linking $i$ and $j$ involve the intermediation of at least one inventor (i.e., a gatekeeper) located in city c.⁴

As far as direct linkages are concerned, these are symmetrically defined as those paths between inventors such that the distance dij) between inventor i (located in focal city c) and inventor $j$ (not located in city c) does not pass through any other inventor located in city c. In other words, direct linkages, in the sense used in this paper, are those that do not involve the intermediation of other inventors located in the same city. In this respect, any type of actors, including gatekeepers, can undertake direct linkages. As before, if all direct linkages defined in this way are aggregated up to the city level, the overall direct reach of city $c$ can be formally defined as:

$$ DIRREAC{H}_c={\displaystyle \sum}_{\begin{array}{c}i=1\\ {}i\in GK\end{array}}^{n_c}{\displaystyle \sum}_{j=1}^{n_h}\frac{1}{d_{ij}}+{\displaystyle \sum}_{\begin{array}{c}i=1\\ {}i\notin GK\end{array}}^{n_c}{\displaystyle \sum}_{j=1}^{n_h}\frac{1}{d_{ij}} $$

(14.3)

where ${d}_{ij}$ is the distance in the co-invention network between inventor $i$ (in city c) and inventor $j$ (not in city c) ; the first term of the summation refers to the subset of inventors in city $c$ who perform the function of gatekeepers for some other co-located inventor, while the second term of the summation refers to the subset of inventors in city c who do not perform the function of gatekeepers for any other co-located inventor.

Importantly, the definitions above imply that the sum of (14.2) and (14.3) is equal to the numerator of (14.1), in other words, to the overall distance-weighted external reach of city c.

Armed with these definitions, we constructed several variables in order to test for the importance of gatekeepers. First, we computed (for each city c and each 5-year time window) the number of inventors who perform the function of gatekeepers. This variable tests whether the mere quantity of gatekeepers can be of relevance for a city’s capacity to recombine and expand its knowledge domains.

Second, we computed the share of the overall external reach, which is either directly or indirectly mediated by gatekeepers. Formally:

$$ SHREACH\_G{K}_c=\frac{{{\displaystyle \sum}}_{i=1}^{n_c}{{\displaystyle \sum}}_{j=1}^{n_h}\frac{1}{d_{ij}^{ind}}+{{\displaystyle \sum}}_{\begin{array}{c}i=1\\ {}i\in GK\end{array}}^{n_c}{{\displaystyle \sum}}_{j=1}^{n_h}\frac{1}{d_{ij}}}{{{\displaystyle \sum}}_{i=1}^{n_c}{{\displaystyle \sum}}_{j=1}^{n_h}\frac{1}{d_{ij}}} $$

(14.4)

This variable captures to what extent a city’s overall external reach would decrease when all gatekeepers are removed from the city (Borgatti, 2006; Valente & Fujimoto, 2010), in other words, how robust its external relations are to the removal of the links established by gatekeepers or, conversely, how much gatekeepers control the flows of knowledge across cities. Hence, it is suitable for testing whether gatekeepers are fundamental mediators of knowledge exchanges leading to higher technological recombination or whether direct relations (also not mediated by gatekeepers) are more effective. Higher values of the index correspond to cities in which external linkages mostly rely upon gatekeepers. On the other hand, lower values of the index imply that most of the linkages with externally located inventors are direct and do not need any intermediation (i.e., removing the gatekeepers would not diminish substantially the external reach).⁵

Third, we decomposed the overall distance weighted external reach mediated by gatekeepers (i.e., the numerator of (14.4)) into its two major components, i.e., the direct and the indirect and we computed the following shares:

$$ SHDIR\_G{K}_c=\frac{{{\displaystyle \sum}}_{\begin{array}{c}i=1\\ {}i\in GK\end{array}}^{n_c}{{\displaystyle \sum}}_{j=1}^{n_h}\frac{1}{d_{ij}}}{{{\displaystyle \sum}}_{i=1}^{n_c}{{\displaystyle \sum}}_{j=1}^{n_h}\frac{1}{d_{ij}}} $$

(14.5)

$$ SHINDIR\_G{K}_c=\frac{{{\displaystyle \sum}}_{i=1}^{n_c}{{\displaystyle \sum}}_{j=1}^{n_h}\frac{1}{d_{ij}^{ind}}}{{{\displaystyle \sum}}_{i=1}^{n_c}{{\displaystyle \sum}}_{j=1}^{n_h}\frac{1}{d_{ij}}} $$

(14.6)

Expression (14.5) is the share of the overall external reach that is due to direct external linkages made by gatekeepers, whereas expression (14.6) is the share of the overall external reach that is due to indirect external linkages mediated by gatekeepers. Such a distinction allows addressing two questions:

• (a) Whether indirect links mediated by gatekeepers are actually relevant for technological recombination in cities as claimed in the literature;

• (b) whether direct relations held by gatekeepers are superior to direct relations held by other actors because of the higher inventiveness, creativity, and power characterizing gatekeepers as proposed in the literature.

Fourth, we measured to what extent the indirect external reach mediated by gatekeepers (i.e., expression (14.2) above) is concentrated in the hands of few individuals. To this purpose, we proceeded as follows. For each individual gatekeeper we computed how much the indirect external reach of the city would decrease by removing this gatekeeper. Then, we took the top 10 % of gatekeepers in terms of impact and computed how much the indirect external reach would decrease by removing these individuals (GKREMOV). It is worth noting that this exercise is somehow related to the notion of network redundancy . To the extent that there are multiple shortest paths and thus gatekeepers between inventor $i$ (in city c) and inventor c, removing a specific gatekeeper does not have any effect on the overall external reach of the city. On the other hand, if there is only one shortest path and thus only one gatekeeper between them, then there is no redundancy, namely the gatekeeper has full control over any information flow between $i$ and j. In terms of the measure discussed above, the greater the impact of the top 10 % of gatekeepers on the overall external reach, the lower the redundancy in information paths and the more the access to external sources of knowledge is concentrated in the few key individuals. A priori, the impact of this variable is uncertain. On the one hand, dispersion of the gatekeeping function among many inventors, with a certain amount of redundancy resulting, could improve the reliability and continuity of access to external knowledge sources and mitigate the control power individual gatekeepers exercise on these sources of knowledge. On the other hand, as long as performing an effective gatekeeping function requires the possession of distinctive skills and attributes that are unlikely to be evenly distributed, concentrating that function among key individuals might result in a better outcome.

Finally, the role played by gatekeepers could also vary according to their concentration or dispersion across firms within a city. To this purpose, we computed the share of the indirect external reach mediated by gatekeepers (i.e., expression (14.2) above), which is accounted for by the top four patenting firms (CONCGK). Once again, the effect of this variable is a priori uncertain. On the one hand, if one believes that performing effectively as a gatekeeper requires special attributes that are not widely distributed in the population, then a higher concentration of that function in few (possibly large) firms might lead to a superior outcome. On the other hand, as long as access to external knowledge is concentrated in few local firms, any benefit that might derive from the activity of gatekeepers does not spread (or spreads more slowly) across all firms in the local economy.

A Hypothetical Example

Figure 14.1 below illustrates the measures derived above by using a highly simplified, hypothetical example of a city with seven inventors (from $a$ to g), who are connected either directly or indirectly to six inventors located outside the boundaries of the city (from $H$ to M).

Fig. 14.1

Hypothetical example of external relations and gatekeepers (Source: Design by authors)

By applying the expression (14.1) above, it is straightforward to check that

$$ \begin{array}{l}{\displaystyle \sum}_{i=1}^{n_c}{\displaystyle \sum}_{j=1}^{n_h}\frac{1}{d_{ij}}=\left(\frac{1}{d_{aH}}+\frac{1}{d_{aI}}+\frac{1}{d_{aJ}}\right)+\left(\frac{1}{d_{bH}}+\frac{1}{d_{bI}}+\frac{1}{d_{bJ}}\right)+\left(\frac{1}{d_{cH}}+\frac{1}{d_{cI}}+\frac{1}{d_{cJ}}\right)\\ {}\kern4em + \left(\frac{1}{d_{dK}}+\frac{1}{d_{dL}}\right)+\left(\frac{1}{d_{eK}}+\frac{1}{d_{eL}}\right)+\left(\frac{1}{d_{fK}}+\frac{1}{d_{fL}}\right)+\frac{1}{d_{gM}}\\ {}\kern3.25em =\left(1+\frac{1}{2}+\frac{1}{2}\right)+\left(\frac{1}{2}+\frac{1}{3}+\frac{1}{3}\right)+\left(\frac{1}{3}+\frac{1}{4}+\frac{1}{4}\right)+\left(1+\frac{1}{2}\right)\\ {}\kern4em + \left(1+1\right)+\left(\frac{1}{2}+1\right)+1=10\end{array} $$

From this, it follows that the average (distance-weighted) external reach of the city is $ADW{R}_c=\frac{10}{7}=1.43$. Of the seven inventors in the city, three perform the function of gatekeepers and are indicated by the octagon shape, in other words,a, $e$ and f.

As far as the overall indirect external reach mediated by gatekeepers is concerned (expression (14.2) above), this is equal to:

$$ \begin{array}{l}\left(\frac{1}{d_{bH}}+\frac{1}{d_{bI}}+\frac{1}{d_{bJ}}\right)+\left(\frac{1}{d_{cH}}+\frac{1}{d_{cI}}+\frac{1}{d_{cJ}}\right)+\left(\frac{1}{d_{dL}}\right)+\left(\frac{1}{d_{fK}}\right)=\left(\frac{1}{2}+\frac{1}{3}+\frac{1}{3}\right)\\ {}\kern1em + \left(\frac{1}{3}+\frac{1}{4}+\frac{1}{4}\right)+\left(\frac{1}{2}\right)+\left(\frac{1}{2}\right)=3\end{array} $$

The overall direct external reach (expression (14.3) above) is instead equal to:

$$ \underset{{\displaystyle \sum}_{\begin{array}{c}i=1\\ {}i\in GK\end{array}}^{n_c}{\displaystyle \sum}_{j=1}^{n_h}\frac{1}{d_{ij}}}{\underbrace{\left(\frac{1}{d_{aH}}+\frac{1}{d_{aI}}+\frac{1}{d_{aJ}}\right)+\left(\frac{1}{d_{eK}}+\frac{1}{d_{eL}}\right)+\left(\frac{1}{d_{fL}}\right)}}\underset{+{\displaystyle \sum}_{\begin{array}{c}i=1\\ {}i\notin GK\end{array}}^{n_c}{\displaystyle \sum}_{j=1}^{n_h}\frac{1}{d_{ij}}}{\underbrace{+\left(\frac{1}{d_{dK}}\right)+\left(\frac{1}{d_{gM}}\right)}}=5+2=7 $$

The share of the overall external reach, which is either directly or indirectly mediated by gatekeepers (expression (14.4) above), is thus given by:

$$ \frac{3+5}{10}=0.8 $$

Similarly, the share of the overall external reach, which is due to direct external linkages made by gatekeepers (expression (14.5) above), is given by:

$$ \frac{5}{10}=0.5 $$

The share of the overall external reach, which is due to indirect external linkages mediated by gatekeepers (expression (14.6) above), is given by:

$$ \frac{3}{10}=0.33 $$

As far as network redundancy is concerned, we observed that removing either gatekeeper e or gatekeeper $f$ has no impact on the overall indirect reach of the city. This is because there are two shortest paths (of length 2) linking inventor $d$ to inventor L. On the other hand, removing gatekeeper $a$ reduces the amount of indirect external reach by 2 (or 80 %). In this over-simplified context, we can say that the overall indirect reach of the city is concentrated among few (in this case, one) individuals.

Data and Estimation Framework

We used information on the technological content of patent documents to measure the ability of cities to renew and expand their knowledge base. Patents can be described as a bundle of different technologies, whereby each is identified by the technology codes classification used at the U.S. Patent and Trademark Office (Strumsky, Lobo, & van der Leeuw, 2012). The technology fields and their combinations found in locally produced patents can therefore provide a good description of a city’s knowledge base (Boschma, Balland, & Kogler, 2015; Kogler, Rigby, & Tucker, 2013). Importantly, this approach allows emphasis to be placed on the intrinsic recombinatorial nature of technical change and inventive processes (Fleming, 2001; Katila & Ahuja, 2002).

We defined our dependent variable as the number of new pairs of technology codes (i.e., combinations) introduced in a city at time t, in which both technology codes are new to the city, in other words, no local patent had been classified in those fields before time t. Differently from other studies (e.g., Fleming et al., 2007), new combinations of technology codes previously used in a city’s patents have been excluded, because they simply recombine existing knowledge, with no renewal or expansion of a city’s knowledge base resulting. Still, our estimates are robust to alternative (less restrictive) measuring of the dependent variable (not shown for the sake of brevity).

In order to compute the number of new combinations in each city, three filters have been applied. First, only cities showing persistent inventive activity (i.e., with a positive number of patents for each year in the period 1990–2004; i.e., 196 out of 370 cities) have been considered, so as to mitigate erratic patterns that may arise on a short time basis because of annual fluctuations and lumpiness in patent records.

Second, new combinations have been identified at the technology group level, as it corresponds to the lowest hierarchical level in the International Patent Classification (IPC, 2014) adopted at the EPO.⁶ Importantly, the number of technology groups per patent class exhibits an extremely skewed distribution: 20 % of patents are classified in only one IPC technology group and, therefore, cannot lead to any new recombination (i.e., they do not enter in the computation of the dependent variable); 95 % of all patents in the sample are classified in eight or less technology groups, and 99 % in fifteen or less groups, with the remaining 1 % of all patents being outliers classified in a number of technology groups ranging from 15 to 63. It is quite obvious that the higher the number of technology groups the greater the number of new combinations. In order to mitigate the bias in the computation of the dependent variable due to the presence of such extreme observations, its construction is based on patents classified in up to eight groups.⁷

Third, the construction of the dependent variable is based on patents in which only inventors located in the focal MSA are reported in the document. The exclusion of new combinations that are the outcome of cross-city collaborative patents provides a more restrictive measure of a city’s autonomous recombinatorial and inventive capabilities and should mitigate possible endogeneity concerns with reference to the main independent variables.

In addition to the variables described in the previous section, the empirical model includes other factors that may potentially affect the renewal and expansion of a city’s knowledge base.⁸ In order to account for the importance of agglomeration economies (see Duranton and Puga [2004] for a review), it includes two variables. First, the number of internal patents (INTPAT) in the city at time t (i.e., by excluding patents with inventors external to the city) captures both the scale effect associated with the agglomeration of inventive activities at the city level, as suggested by Bettencourt et al. (2007) and Lobo and Strumsky (2008), and the potential for technological recombination. Second, the degree of concentration of inventive activities among firms, computed as the Herfindahl index at the level of patent assignees (HFIRMS), accounts for (possible) effects of local market structure and competition (Beaudry & Schiffauerova, 2009).

A further set of variables controls for the nature of the local knowledge base. First, an index of absolute specialization, namely the Herfindahl index of the share of patents made in IPC four-digit (i.e., subclass) technology fields (HPATENTS), captures to what extent a city is specialized in a narrow set of fields, thereby controlling for the presence of externalities arising from technological specialization (Feldman & Audretsch, 1999).⁹ Second, the average number of citations of the non-patent literature made by a city’s internal patents (NPLCIT) measures the scientific orientation and generality of the local knowledge base. It is not a simple matter to anticipate the sign of this variable. More science-oriented knowledge can have more difficulties in finding its immediate technological application, leading to a negative effect of this variable on the recombinatorial capabilities of a city. On the other hand, more universal notions are more likely to find multiple applications at the junction of different technological domains (Fleming & Sorenson, 2004), meaning that a more general and science-based knowledge base could open greater possibilities of technological recombination. Third, because the Herfindahl index captures the absolute technological specialization of a city, the model also includes an index of relative technological specialization measuring the dissimilarity between the technological profile of city c and that of all the other cities with which inventors of city c have collaborative linkages, namely the so-called Krugman index (KI), at the level of technology groups in which a city’s patents are classified. For each city $c$ a time t, $KI$ is defined as follows:

$$ K{I}_c={\displaystyle \sum}_{i=1}^n\left|\frac{P_{ci}}{P_c}-\frac{P_i}{P}\right| $$

(14.7)

where ${P}_{ci}$ is the number of patents of city $c$ in technology field i; ${P}_c$ is the total number of patents of city c; and ${P}_i$ is the total number of patents obtained in all U.S. cities (excluding city c) in technology field i. This index has been adjusted in order to make cities with which inventors of city $c$ have more collaborative links (and thus knowledge exchange ) have more weight in the computation of the Krugman index of city c. Similarly, only cities with which inventors of city $c$ have external linkages enter into the computation of the index. In particular, ${P}_i$ has been weighted by the frequency of co-inventing links between inventors of city c and inventors of city d, and $P$ is the total number of patents obtained in all U.S. cities (except city c), weighted by the frequency of co-inventing links between inventors of city c and inventors of city d.¹⁰

The Krugman index ranges from 0 to 2, taking value 0 for cities whose technological profile is totally identical to the average technological profile of the cities with which it has external linkages, and taking value 2 for cities that are specialized in completely different fields. Dissimilarity from the average knowledge base of all other cities with which a city has linkages can make more fruitful (and necessary) communication, knowledge exchanges , and learning and, in turn, can stimulate the recombination of internal and external knowledge.

The last group of variables considered includes two further controls for other structural properties of the co-invention network within a city (i.e., based only on ties among inventors located in the same city). First, the largest connected component (LARGE) is the ratio between the number of inventors that are in the largest component of the network and the total number of metropolitan inventors. It ranges from zero (all inventors are isolates) to one (all inventors are directly or indirectly connected) and aims to capture the size and degree of internal connectivity in the co-invention network (Lobo & Strumsky, 2008). Second, the clustering coefficient (CLUST) captures the extent to which the partners of an inventor, within the city, are also partners with each other. This index ranges from zero to one, with higher values indicating that the internal city network is composed of dense cliques of collaboration.¹¹ Because cliquishness can cause isolation and localism; reduce exposure to alternative ideas; and limit the access, absorption, and recombination of externally sourced knowledge; it has to be expected to have a negative effect on the recombinatorial capacity of a city (Uzzi & Spiro, 2005).

All the explanatory variables (except the number of internal patents) are 1-year lagged over a 5-year moving window (i.e., computed over the period $[t-1,t-5$]) with respect to the dependent variable to mitigate endogeneity concerns. The definition of variables is summarized in Table 14.1. Table 14.2 reports summary statistics, while Table 14.3 reports the correlation matrix.¹²

Table 14.1 Definition of dependent and independent variables

Table 14.2 Summary statistics of dependent and independent variables

Table 14.3 Correlation matrix of dependent and independent variables

The empirical model estimated is based on a conditional negative binomial framework with fixed-effects by controlling for annual effects, which allows accounting for the integer and over-dispersed nature of the dependent variable, as was done in similar studies (Fleming, King, & Juda, 2007; Fleming et al., 2007 Schilling & Phelps, 2007).

Results

Table 14.4 reports the results of regression estimates. The first columns present the model with only the control variables. The following columns progressively include the variables accounting for external linkages and gatekeepers. All models report estimated coefficients transformed to incidence rate ratios (IRR), defined as $\exp \left(\hat{b}\right)$.

Table 14.4 Technological recombination and the role of gatekeepers

Concerning the control variables, the coefficient of the number of internal patents shows, as expected, that the scale of inventive inputs and experimentation matters. More specifically, estimates indicate that, all else being equal, a unit increase in the (log) number of internal patents is associated with a doubling in the number of new combinations of technology groups introduced in a city.

Concentration of inventive activities among relatively few firms is negatively associated with technological recombination, suggesting that competitive market structures are more conducive to a renewal of the local knowledge base. As far as the specialization variables are concerned, absolute technological specialization in a narrow set of technology fields has a negative impact on the ability to expand the knowledge base, whereas relative technological specialization (i.e., a dissimilarity of the technological profile to that of the other cities with which it exchanges knowledge) is positively related to the number of new combinations introduced in a city. A plausible interpretation of this result is that a lower cognitive overlap enhances complementarities and opportunities for learning.

Regarding the variables capturing the structure of the within-city co-invention network, the coefficients of the fraction of inventors in the largest component together with that of the clustering coefficient (albeit not significant) seem to suggest that in cities characterized by dense cliques of collaborators , knowledge may flow relatively quickly within cliques, but it may also be highly redundant, which hampers recombination.

Coming to the main variables of interest, external links prove to be an important mechanism to support the expansion and renewal of a city knowledge base (model 2). The IRR of $ADWR$ is larger than 1 and statistically significant, even though the magnitude of the effect is not extremely large: Keeping all other variables constant, a standard deviation increase in the average external reach brings around $7.2\%\left(=\left[ \exp \left(0.0068883\times 10.10\right)-1\right]\times 100\right)$ more new combinations in a city.

By decomposing the impact of external reach, it turns out that the sheer number of gatekeepers ($NUMGK$) is detrimental to recombination (model 3), even though the corresponding IRR is very close to 1. Nevertheless, this result is somewhat counterintuitive at a first glance, because gatekeepers are generally thought to be creative and imaginative actors. A possible interpretation, already anticipated in the previous sections of the paper, is that the control power these actors exert on the bridging ties and knowledge exchanges they govern can weaken indigenous capacity of recombination because they are in the position to grant, restrict, or even block the access to external knowledge flows. A further possible interpretation is that performing the gatekeeping function effectively requires skills and abilities that are not widely distributed in the population.

Both interpretations find further support in model (14.4). The share of the overall external reach mediated by gatekeepers (SHREACH_GK) shows a negative and statistically significant effect. All else equal, a standard deviation increase in this share is associated to a reduction of around 6 % in the number of new combinations introduced in a city.

To probe further into the impact of gatekeepers, model (14.5) splits the overall external reach mediated by gatekeepers into its direct and indirect components. Results show that the negative effect associated with gatekeepers is due precisely to the indirect external reach mediated by gatekeepers (i.e., SHINDIR_GK), rather than to the gatekeepers’ direct external ties (i.e., SHDIR_GK). Overall, these results suggest that, all else equal, direct links to external sources of knowledge outperform indirect ones, namely those mediated by gatekeepers, in sustaining expansion and renewal of a city’s knowledge base. In other words, the key message stemming from these results is that direct links, regardless whether developed by gatekeepers or other actors in the network, are far more reliable and fruitful in spurring technological recombination in cities.

Model (14.6) introduces into the regression the variable that captures the fraction of the indirect external reach accounted for by the top 10 % of gatekeepers (i.e., GKREMOV). The rather surprising result is that the IRR of this variable is larger than 1 and statistically significant, thereby indicating that a greater concentration of the gatekeeping function among few individuals is positively associated with technological recombination and may at least partially compensate for the generally negative effect of gatekeepers. Here, we can only offer a speculative interpretation of this finding. As already mentioned above and as pointed out in the organizational literature, effective gatekeepers are a relatively “rare breed and few networks have many of them” (Cross & Prusak, 2002, p. 109). Indeed, only few individuals are likely to have the intellectual expertise, social skills, and personality traits necessary to perform that role. Thus, one can advance the conjecture that the more diluted among many individuals the function of interfacing with the external environment is, the less effective, all else equal, the performance will be.

Model (14.7) further tests this conjecture by including in the regression the share of the indirect external reach accounted for by the top four firms in the city. The IRR of this variable is greater than 1 and statistically significant, thus suggesting that a greater concentration of the gatekeeping function in few firms is beneficial to exploration and technological recombination. Once again, advancing a definitive interpretation for this result is somewhat hazardous. At the same time, this result is consistent (or at least not in contrast) with several studies showing that leading large and technologically sophisticated firms are those more likely to act as gatekeepers and possibly generate externalities for other colocated entities (Agrawal & Cockburn, 2003). Still, we believe that this point represents an issue for further research.

Conclusions

We have offered an explorative perspective on the role of gatekeepers in the expansion and renewal of a city’s knowledge base. Quite interestingly, the results indicate that external relations and their structure play a pivotal role in renewing, expanding, and regenerating a city’s knowledge base, although only direct relations were particularly effective in this regard. Importantly, albeit considered imaginative, inspired, and open to new approaches and radical innovation, gatekeepers per se, and the indirect relations they mediate, do not necessarily contribute to enriching the knowledge base of the cities where they are located.

These results indeed challenge conventional wisdom that often invokes gatekeepers as the most important means of accessing and exploiting external knowledge as well as—more importantly— strategy and policy recommendations based on this assumption aimed at increasing the number and importance of gatekeepers in mediating knowledge flows across both organizational and geographical boundaries.

We contend that this conventional wisdom about the role and importance of gatekeepers is based on two misconceptions. First, external relations crucial to expanding and regenerating a city’s knowledge base encompass several types of relations, but direct relations perform this function most effectively, by allowing faster, more trusted, and less noisy knowledge exchanges . Second, at an aggregate level of analysis such as the urban level, the control power that gatekeepers can exert on the knowledge flows they govern can more than offset the benefits accruing from their superior inventive performance. An excessive reliance upon indirect flows mediated by gatekeepers can signal a situation of knowledge dependence that is at higher risk of linkage disruption. Moreover, the intellectual expertise and skills necessary to act as effective gatekeepers are likely to be rather rare and possibly only present in large and technologically sophisticated organizations.

Should one conclude from what our analysis that gatekeepers are not necessary—or even detrimental to the renewal of a city’s knowledge base? We believe that this conclusion is not warranted. In a companion article (Breschi & Lenzi, 2015), we show indeed that the role played by gatekeepers may indeed be important, but only in some specific circumstances. In particular, by confirming the longstanding intuition of Tushman and Katz (1980) and Tushman and Scanlan (1981), we show there that gatekeepers only play an important role when the knowledge base of a city is sufficiently different and specialized with respect to other cities to require the absorption of knowledge and the transcoding function of those actors.

In conclusion, the chapter is intended to provide a contribution to the literature on both conceptual and methodological grounds. First, we clarify and qualify the role and function of gatekeepers. Second, we propose an operational method to quantify the importance of gatekeepers in brokering knowledge flows across cities and a set of new indicators that allow measurement of the meso-level effects (i.e., at the city level) of individual behavior and interactions (i.e., of inventors, gatekeepers, and co-invention networks). We hope that they will be useful and deployed in future research.