Keywords

1 Introduction

Vibration is an inevitable phenomenon and can be harmful to structures in the domains of civil engineering or the machine industry. In recent decades, novel systems composed of a primary linear structure and a strongly nonlinear attachment have attracted attention because of their ability to absorb a wider range of vibration frequencies and their relatively small attached mass [1]. Because of the existence of a nonlinear component, which is always referred to as cubic stiffness, the system demonstrates localization and irreversible transient transfer of energy from the primary system to the attached mass [2]. This new concept is called a Nonlinear Energy Sink (NES). Several methods have been proposed for achieving a cubic stiffness. G. Kerschen used a straight air tract to support the primary system and NES. An essential cubic stiffness nonlinearity was obtained by means of a thin hat without tension [3]. Our idea is to use a variable pitch spring to produce the desired cubic stiffness by designing the distribution of the pitch.

The structure of the current paper is as follows. In Sect. 2, a NES system design process will be presented based on the variable pitch spring. In Sect. 3, the nonlinear constraint will be defined according to the various conditions. In Sect. 4, Multi Start, a global search method, will be applied and the optimization result will be compared. Section 5 contains some concluding remarks and discussion.

2 NES Design Process

The most significant component in a NES system is the cubic stiffness, which is obtained through the variable pitch spring in our study. A core characteristic of the variable pitch spring is its progressively increasing pitch, which results in a variable stiffness. Sequenzia G provides an iterative calculation method to define precisely the geometry of helix line [6]. Another design of a variable pitch spring coupled with a negative stiffness mechanism has been proposed by D. Qiu [5] and used in our study. The objective displacement-force relation of a variable pitch spring can be defined by the following equation, where k0 is the linear stiffness before the spring reaches its transition point st, a1 is the spring rate in the nonlinear part, and a3 is the cubic stiffness value.

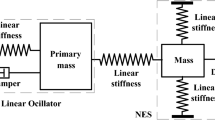
$$ F = \left\{ {\begin{array}{*{20}c} {k_{0} u} \\ {a_{3} \left( {u - s_{t} } \right)^{3} + a_{1} \left( {u - s_{t} } \right) + k_{0} s_{t} } \\ \end{array} \begin{array}{*{20}c} {\left( {u \le s_{t} } \right)} \\ {\left( {u > s_{t} } \right)} \\ \end{array} } \right. $$
(1)

However, when we apply the nonlinear spring, the force displacement characteristic inevitably includes a linear part [5]. To skip the linear part, a method of combining two pre-compressed springs at the transition point is adopted, as in part (b) of Fig. 1. So the composed stiffness curve \(f_{K} \, = \,\left( {a_{1} \, + \,k_{0} } \right)\,u + a_{3} u^{3}\) is obtained. To counterbalance the linear phase a1 + k0, a negative stiffness mechanism is introduced, as in part (a) of Fig. 1. When the two linear springs are subjected to horizontal pre-compression, they have a negative stiffness in the vertical direction. The force-displacement relation can be expressed as a Taylor expansion. It is important to note that the Taylor expansion is valid only when the displacement remains close to the initial balancing point. By adding the force of the pre-compressed variable pitch spring combined with a negative stiffness mechanism, a composed force displacement relation is expressed:

$$ F = \left( {a_{1} + k_{0} - 2k_{l} \frac{{l_{p} }}{{l_{0l} + 2l_{c} - l_{p} }}} \right)u + \left( {a_{3} + k_{l} \frac{{l_{0l} + 2l_{c} }}{{\left( {l_{0l} + 2l_{c} - l_{p} } \right)^{3} }}} \right)u^{3} $$
(2)

Here, l0l and lp are the free length of the linear spring and its length of pre-compression. lc is the length of the connectors, which are connected to the ground and the central mass by hinges. The connector is marked in Fig. 2. A schematic diagram of this NES system is proposed in Fig. 1. The linear component of Eq. (2) can be counterbalanced by defining the stiffness of cylindrical spring kl. To design a cylindrical spring with the target stiffness, the diameter of a linear cylindrical spring, Dl, and the diameter of its wire, dl, are necessary to fix its active number of coils through the following equation:

$$ n_{l} = \frac{{Gd_{l}^{4} }}{{8D_{l}^{3} k_{l} }} $$
(3)

A method using a special coordinate point is adopted to manufacture a variable pitch spring with the required stiffness curve. Groups of coils with different pitches are symmetrically distributed on both sides of the spring. The detailed process to determine the distribution of variable pitch can be found in Donghai’s work [4]. Through the above analysis, the whole NES system is determined by 11 independent variables (d, D, lf, dl, Dl, l0l, k0, a1, st, a3, pc). pc is a dimensionless coefficient of pre-compression \(l_{p} \, = \,p_{c} \, * \,l_{0l}\). The mass of the total of 4 springs is easily obtained:

$$ M = 2\left( {\left( {\sum\limits_{i = 1}^{{n_{a} }} {n_{i} + 1.5} } \right)D\frac{{d^{2} \pi^{2} }}{4} + \left( {n_{l} + 1.5} \right)D_{l} \frac{{d_{l}^{2} \pi^{2} }}{4}} \right)\rho $$
(4)

The sum of ni and nl are the total numbers of active coils in the variable pitch spring and the cylindrical spring, the extra 1.5 coils being added as end coils at both spring sides to support the active coils. The lower boundary (Lb) and upper boundary (Ub) of the parameters are presented in Table 1.

Table 1. Lower and upper boundaries for the parameters of the NES system.
Full size table
Fig. 1.
figure 1

Schematic of NES mechanism

Full size image

3 Nonlinear Constraint Analysis

In order to maintain reliable operation of the device in complex situations, the design of the spring and the parameter values should be strictly chosen to meet the different constraints, e.g. design constraints, buckling conditions, space between coils of the nonlinear spring, and static maximum constraint. These nonlinear constraints involve all the design parameters, and they can hardly be separated individually. For the sake of convenience, we sort the constraints in Table 2.

Table 2. Definition of the optimization constraint
Full size table

When the central mass moves to the upper maximal displacement, two potentially dangerous conditions appear. The separated position is indicated in the dotted red circle in Fig. 2, which corresponds to the 12th/13th/14th constraints.

Fig. 2.
figure 2

Detachment conditions (1) left: spring 2, 4 separation; (2) right: spring 1 or 3 released

Full size image

4 Optimization Result

The function fmincon of Matlab is used to obtain a local minimum value with respect to the nonlinear and linear constraint. This kind of nonlinear optimization problem is extremely sensitive to the initial starting point. To generate a global solution, one idea is to repeatedly run a local solver with the various starting points. The best solution is then picked among the multiple local minima. The Multi Start method was applied in our study.

Firstly, we should define the working course, Course = 80 mm, and the tolerance, toler = 5 mm, to prevent the center of mass falling outside the working course. The target cubic stiffness, A3 is 4e5 N/m3. The initial value is chosen with the parameters given in [5].

Fig. 3.
figure 3

Comparison of target stiffness and optimized design stiffness.

Full size image
Fig. 4.
figure 4

Symmetrical type of pitch distribution for different optimized designs (80/70/60 mm) and original design.

Full size image

When the number of local solvers is 1000, the lightest quality design parameter is obtained in Table 3 and a new distribution of pitch is found in Fig. 4. Our mass is reduced to 88.2 g. So, compared with the original mass of 182.98 g, there is a mass saving of 51.8%. At the same time, the error of the force-displacement relation of optimization (4.28%) is kept below the tolerance level (5%) presented in Fig. 3. In other side, the different design targets for example Course = 70 mm and Course = 60 mm are performed. The mass result reduce to 69.9 g and 60.3 g respectively. The detailed optimal parameters are presented in Table 3.

Table 3. Parameter of optimization result for Course = 80 mm/70 mm/60 mm
Full size table

5 Conclusions

This article has studied the realization and optimization of a NES system with a variable pitch spring. The main challenge is to construct a pure cubic stiffness system. The variable pitch springs are combined axially with pre-compression and negative stiffness mechanism are used to counterbalance the linear phase and leave a pure cubic stiffness. By using Multi Start method, it is possible to obtain global resolution with respect to the nonlinear constraint and minimize the mass of the system. The stiffness curve of optimized design basically coincides with the target stiffness curve, while the mass is reduced effectively. For the next stage of the study, we will analyze the optimization result and other constraints still further to reduce the number of parameters.