X-ray Focusing and Optics

Abstract

This chapter addresses fundamental concepts of X-ray optics and X-ray coherence, in view of the increasing number of X-ray applications requiring nano-focused X-ray beams. The chapter is meant as a tutorial to facilitate the understanding of later chapters of this book. After the introduction and an overview over focusing optics and recent benchmarks in X-ray focusing, we present refractive, reflective and diffractive X-ray optics in more detail. Particular emphasis is given to two kinds of X-ray optics which are particularly relevant for later chapters in this book, namely X-ray waveguides (XWG) and multilayer zone plates (MZP). Both are geared towards ultimate confinement and focusing, respectively, i.e. applications at the forefront of what is currently possible for multi-keV radiation. Since optics must be designed in view of coherence properties, we include a basic treatment of coherence theory and simulation for X-ray optics. Finally, the chapter closes with a brief outlook on compound (combined) optical schemes for hard X-ray microscopy.

Es ist aber leicht einzusehen, daß bei nahezu streifender Inzidenz der Röntgenstrahlen im Falle $n < 1$ eine nachweisbare Totalreflexion auftreten muß.

— Albert Einstein, 21st March, 1918

1 General Aspects of X-ray Optics and Focusing

X-ray optics can be considered as optics in the “vacuum limit”. In fact, the index of refraction $n=1-\delta +i\beta$ asymptotically approaches one for high photon energy E, as $\delta$ and $\beta$ decrease algebraically for $E\ge E_r$, where $E_r$ stands for an atomic resonance, i.e.  an absorption edge given by the corresponding electronic binding energy. For all materials, the X-ray regime is hence characterized by extremely small differences in the indices of refraction. This can be a blessing in terms of penetration power, or the validity of various approximations, such as kinematic scattering (neglect of multiple scattering) or the projection approximation, as addressed in Chap. 2. At the same time it can also be a curse, as one readily realizes the challenge of focusing radiation when the index difference between a lens and air or vaccuum goes to zero. More generally, not only focusing but any type of optical element and function is heavily constrained by the small differences in the index of refraction. For this reason, it is not yet possible to focus down to X-ray wavelength $\lambda$. In Abbe’s sense, the diffraction limit is not in $\lambda$ but in the achievable numerical aperture. In other words, the diffraction limit for X-rays is a limit of the diffraction structure. This has raised the question of a fundamental resolution limit for X-rays existing above the wavelength. In 2003, Bergemann and van der Veen had conjectured a fundamental length scale $\varLambda$ given by the decay length of evanescent waves, which should present a lower limit for any X-ray focus size well above the wavelength $\lambda$. This critical length prototypically appears in waveguide optics in terms of the minimum width to which a mode can be confined, as explained further below, and roughly ranges in between 8 and 15 nm depending on the density of the material used for focusing [1]. This limit was later rejected and successively disproved [2, 3]. Yet, the idea is correct that the small contrast in the index of refraction for X-rays and the correspondingly large decay length of evanescent waves indeed significantly constrain our focusing capabilities. More than fifteen years after the postulation of the “Bergemann limit” we must realize that we still do not know the minimum focus size nor the maximum local field enhancement (gain) in focusing X-ray radiation. Experimentally, however, 10 nm focal size has become a reality also for hard X-rays [4, 5]. Figure 3.1 illustrates the rapid development of hard X-ray focusing over the last two decades. This progress became possible after the advent of high brilliance (3rd generation) synchrotron radiation, which provide the necessary coherence for diffraction-limited or near-diffraction limited focusing. 

Fig. 3.1

Illustration of the rapid progress in X-ray nano-focusing, following the advent of 3rd generation synchrotron radiation. Major benchmarks are displayed as full symbols (2d focusing) or empty symbols (1d focusing), representing the following references (from left to right): FZP optics (brown circles) [6,7,8,9], CRL (black downwards-pointed triangles) [10,11,12,13,14,15], KB mirror optics [16,17,18,19,20,21,22], MLL/MZP (purple diamonds) [4, 23,24,25,26,27,28], and WG or KB/WG compound optics (orange triangles) [29,30,31,32,33], respectively. The arrows mark results obtained by the CRC ‘Nanoscale Photonic Imaging’ in Göttingen

The primary challenge for X-ray microscopy is hence to narrow down the gap between the theoretical resolution limit associated with the wavelength $\lambda$ and the actual resolution limited by the optical systems. Fresnel zone plates (FZP) have been developed as X-ray focusing and objective lenses by G. Schmahl and colleagues in Göttingen, initially for X-ray microscopy in the soft X-ray range (0.2–1.2 keV, $\lambda = 1$–7 nm). Spot sizes of soft X-ray microscopes of around 30 nm are common; “best values” are about two times smaller, in the range 10–15 nm [34]. Hard X-ray zone-plate optics was for a long time limited to above ${\simeq } 0.25\,\upmu$m, but over the last fifteen years, significant progress as been achieved by several advanced concepts which realize FZP optics based on multilayer deposition on planar solids as well as on thin wires. Such structures are denoted as multilayer Laue lense (MLL) and multilayer zone plates (MZP), respectively, and will be discussed in detail in Sect. 3.5. Progress within the present collaborative research center (CRC Nanoscale Photonic Imaging), in particular, has resulted in 5 nm point focusing by a MZP optics. Compared to diffractive optics, refractive optics as used for visible and UV light seem at the first glance, unsuitable due to the small X-ray refractive index, with $\delta$ ranging in the order of $10^{-5}$ for hard X-rays. To realize refraction comparable to that of lenses for visible light, a multitude of lenses must be lined up; this concept of compound refractive lenses (CRL) was invented in the 1990s by A. Snigirev and B. Lengeler at the European Synchrotron Radiation Facility (ESRF) in Grenoble [15], and has been thriving since. Today, CRLs made out of Beryllium are found almost at every synchrotron beamline. For nano-focusing, CRLs fabricated by electron beam (e-beam) lithography in silicon have been developed by C. Schroer, and reach spot sizes down to 50 nm [14]. Next to diffractive and refractive optics, reflective optics can be implemented for hard X-rays, taking advantage of grazing-incidence total reflection or multilayer-constructive reflection. Since long, curved mirrors have been appreciated as high efficiency and non-dispersive focusing elements for synchrotron radiation. In the 1990s, with advent of 3rd generation synchrotron sources, mirror-based optics reached spot sizes in the range of 1–5 $\upmu$m. With novel polishing tools for highly curved mirrors developed by the group of K. Yamauchi in Osaka [35], and alternatively of Kirkpatrick-Baez (KB) mirrors with adaptive bending as implemented by O. Hignette at ESRF [18], sub-100 nm focusing became available ten years ago. At the same time, first compound optics with two-stage focusing or collimation was implemented for hard X-rays. Using a combination of high gain KB mirrors and X-ray waveguide optics, a $25\times 47\,\mathrm {nm}^2$ exit beam with clean background and high degree of coherence was demonstrated in [30]. In the course of subsequent research within CRC Nanoscale Photonic Imaging, waveguide optics has been significantly improved, and point focusing down to 10 nm (in the exit plane of the waveguide) is now possible. At the same time efficiency has also been significantly improved. As a result, X-ray micro- and nanofocussing can be implemented today by either diffractive (example: Fresnel zone plates), reflective (examples: Kirckpatrick-Baez mirror, waveguides) and refractive optical elements (example: compound refractive lenses), and/or combinations thereof. X-ray optics and in particular nanofocusing has been an enabling tool to extend X-ray microscopy over the recent years, in spectral range, in resolution and in contrast mechanism. This is true not only for the classical full-field scheme of transmission X-ray microscopy (TXM) which is based on objective zone plates, or scanning X-ray transmission microscopy (STXM), but also for coherent diffraction imaging (CDI) and holography, which also take advantage of X-ray focusing, even if the resolution limits are no longer limited by the focal size. Figure 3.2 illustrates the rapid development of hard X-ray focusing over the last two decades, following the advent of high brilliance (3rd generation) synchrotron radiation, which had provided the necessary coherence for diffraction-limited or near-diffraction limited focusing.

Fig. 3.2

Number of scientific articles over the last 20 years, as retrieved by a Google Scholar search using the filter X-ray AND nano-focus. The increase nicely illustrates the progress and interest in this field

In this chapter, we give an introduction into reflective and diffractive X-ray optics, to provide basic knowledge for further chapters. For refractive optics, we refer to the excellent reviews in [36]. In Sect. 3.2, we first present the basics of X-ray reflectivity, followed by a section on mirrors (Sect. 3.3) and X-ray waveguides (Sect. 3.4). Section 3.5 then presents Fresnel zone plate (FZP) optics, and Sect. 3.6 an introduction to coherence. We close by briefly addressing compound optics and different variants of X-ray microscopes (Sect. 3.7).

2 X-ray Reflectivity and Reflective X-ray Optics

In Chap. 2 we have justified the use of scalar wave theory in the hard X-ray spectral range. Therefore, also Fresnel reflectivity can be accounted for simply by considering the boundary conditions of a scalar wave $\psi$ at interfaces of layered materials. More generally, one has to differentiate between the different polarisation states. The scalar approximation holds, since the decrements of the index of refraction $\delta$ and $\beta$ are much smaller than unity, and only small angles (much smaller than the Brewster angle) are relevant in X-ray reflectivity. In fact, small-angle approximation is also warranted in most cases. There are excellent treatments of X-ray reflectivity [37,38,39]. In this section, we follow the derivation presented in the textbook ‘Elements of modern X-ray physics’ by Als-Nielsen and McMorrow [37]. 

2.1 X-ray Reflectivity of an Ideal Single Interface

Consider a scalar wave with wave vector $\mathbf {k}_I$ and an amplitude $a_I$, impinging from vacuum onto a semi-infinite medium with a sharp interface. The reflected wave is denoted by $\mathbf {k}_R$ and $a_R$, and the transmitted wave by $\mathbf {k}_T$ and $a_T$. As boundary conditions we require the wave $\psi$ and its derivative $\nabla \psi$ to be continuous at the interface between the two media (Fig. 3.3)

$$\begin{aligned} a_I + a_R = a_T \end{aligned}$$

(3.1)

Fig. 3.3

An incoming wave $\psi _I = a_I \mathrm {e}^{i \mathbf {k}_I\cdot \mathbf {r}}$ at an incident angle $\alpha$ is partly reflected under the same angle $\alpha$ forming the wave $\psi _R = a_R \mathrm {e}^{i \mathbf {k}_R\cdot \mathbf {r}}$ and partly transmitted under an angle $\alpha '$ forming a transmitted wave $\psi _T = a_T \mathrm {e}^{i \mathbf {k}_T\cdot \mathbf {r}}$ following [37]

and

$$\begin{aligned} a_I \mathbf {k}_I+ a_R \mathbf {k}_R = a_T \mathbf {k}_T. \end{aligned}$$

(3.2)

The wave number is $k=|\mathbf {k}_{I,R}|$ in vacuum, and $nk=|\mathbf {k}_{T}|$ in the medium. Considering the components of the wave vector parallel and perpendicular to the surface yields

$$\begin{aligned} a_I k \cos \alpha + a_R k \cos \alpha = a_T\,nk \cos \alpha ' \end{aligned}$$

(3.3)

and

$$\begin{aligned} -(a_I -a_R) k \sin \alpha = -a_T\,nk \sin \alpha '. \end{aligned}$$

(3.4)

From the above equations, Snell’s law is obtained

$$\begin{aligned} \cos \alpha = n \cos \alpha '. \end{aligned}$$

(3.5)

Approximating the cosines for small angles using $\cos \alpha = 1-\alpha ^2/2$, and $n = 1-\delta + i\beta$, one finds

$$\begin{aligned} \alpha ^2= & {} \alpha '^2 + 2 \delta - 2 i\beta \nonumber \\= & {} \alpha '^2 + \alpha _\text {c}^2 - 2 i\beta . \end{aligned}$$

(3.6)

Here, $\alpha _\text {c}^2=\sqrt{2\delta }$ denotes the critical angle of total external reflection  from the optically thicker (here: vacuum) to the optically thinner medium. Using (3.1) and (3.4), we have

$$\begin{aligned} \frac{a_i -a_R}{a_I + a_R} = n \frac{\sin \,\alpha '}{\sin \,\alpha } \simeq \frac{\alpha '}{\alpha }. \end{aligned}$$

(3.7)

With

$$\begin{aligned} \frac{1- r}{1+ r} = \frac{\alpha '}{\alpha } \end{aligned}$$

(3.8)

and $1+r=t$, this leads to the Fresnel equations

$$\begin{aligned} r:= & {} \frac{a_R}{a_I} = \frac{\alpha - \alpha '}{\alpha + \alpha '},\end{aligned}$$

(3.9)

$$\begin{aligned} t:= & {} \frac{a_T}{a_I} = 1+r= 1+\frac{\alpha -\alpha '}{\alpha +\alpha '} = \frac{\alpha +\alpha '+\alpha -\alpha '}{\alpha +\alpha '}=\frac{2\alpha }{\alpha +\alpha '}, \end{aligned}$$

(3.10)

where r denotes the amplitude reflectivity and t the amplitude transmission function. The intensity reflectivity is expressed by

$$\begin{aligned} R_F = \left| \frac{\alpha -\alpha '}{\alpha +\alpha '} \right| ^2 = \left| \frac{q-q'}{q+q'} \right| ^2, \end{aligned}$$

(3.11)

where $q=2k\,\sin \,\alpha$ and $q'=2k\,\sin \,\alpha '$ denote the momentum transfer, which is always vertical to the interface. The reflectivity as a function of q is unity up to the critical wave vector $q_\text {c}=2k/\sqrt{2\delta }$ (discarding absorption) and then decreases algebraically with $q^{-4}$. This characteristic makes X-ray reflectometry a powerful tool for the study of surfaces and interfaces of materials, since weak signals of interface disturbances can interfere with this “carrier wave”, such that the signal of a single atomic layer becomes observable. The transmission function $T=|t|^2$ increases from zero to $q_\text {c}$, where it reaches a maximum of four (discarding absorption), and then decreases again to unity for $q \gg q_\text {c}$. The propagation angle in the medium $\alpha '$ is a complex number, which can hence be decomposed into

$$\begin{aligned} \alpha ' = \text {Re}\left( \alpha '\right) + i\,\text {Im}\left( \alpha '\right) . \end{aligned}$$

(3.12)

Accordingly, the transmitted wave can be expressed by

$$\begin{aligned} a_T \mathrm {e}^{ik \alpha ' z} = a_T\,\mathrm {e}^{ik\text {Re}(\alpha ') z}\,\mathrm {e}^{-k\text {Im}(\alpha ') z}. \end{aligned}$$

(3.13)

Hence, the intensity falls off with a 1/e penetration depth $\varLambda$ given by

$$\begin{aligned} \varLambda = \frac{1}{2k\,\text {Im}(\alpha ')} = \frac{1}{q_\text {c}\,\text {Im}(q')}. \end{aligned}$$

(3.14)

Below the critical angle, the real term is zero and the wave is purely evanescent with a decay length which goes to $1/q_\text {c}$ for $\alpha \ll \alpha _\text {c}$. This localisation of intensity to the immediate sub-surface region is exploited in grazing incidence diffraction (GID) [40], and grazing incidence small-angle scattering (GISAXS) [41] (Fig. 3.4).

Fig. 3.4

Fresnel reflectivity   R and transmission T as a function of momentum transfer q, expressed in natural (dimensionless) unit $q/q_\text {c}$

2.2 Multiple Interfaces and Multilayers

Let us first consider reflectivity in case of a sample with one layer above the substrate, still following [37]. In the following, $n_0$ is the index of refraction of vacuum, $n_1$ the index of refraction of the layer and $n_2$ the index of refraction of the substrate. In contrast to the case of reflection from a pure substrate, there is now a series of possible reflections:

• (i) Firstly, reflection at the interface 0 to 1 (interface vacuum/layer), amplitude reflectivity is $r_{01}$.

• (ii) Secondly, transmission at the interface 0 to 1, $t_{01}$, then reflection at the interface 1 to 2, $r_{12}$, followed by transmission at the interface 1 to 0, $t_{10}$. By adding this wave to the above, it is necessary to include the phase factor $p^2=\mathrm {e}^{iq\varDelta }$, where $\varDelta$ is the thickness of the layer.

• (iii) Thirdly, transmission at the interface 0 to 1, $t_{01}$, then reflection at the interface 1 to 2, $r_{12}$, followed by reflection at the interface 1 to 0, $r_{10}$, then another reflection at the interface 1 to 2, $r_{12}$, finally followed by transmission 1 to 0, $t_{10}$. The total phase factor for this wave is $p^4$.

Hence, the total amplitude reflectivity is

$$\begin{aligned} r_{\text {layer}}= & {} r_{01}+ t_{01}t_{10}r_{12} p^2+ t_{01}t_{10} r_{10} r_{12}^2 p^4+ t_{01}t_{01} r_{10}^2 r_{12}^3 p^6+ \dots \nonumber \\= & {} r_{01}+ t_{01}t_{10}r_{12} p^2 \,\left[ 1+ r_{10} r_{12} p^2 + r_{10}^2 r_{12}^2 p^4+ \dots \right] \nonumber \\= & {} r_{01}+ t_{01}t_{10}r_{12} p^2 \, \sum _{m =0}^{\infty } (r_{10} r_{12} p^2)^m \nonumber \\= & {} r_{01}+ t_{01}t_{10}r_{12} p^2 \, \frac{1}{1 - r_{10} r_{12} p^2}, \end{aligned}$$

(3.15)

where the geometrical series has been used in the last line. Using the definitions of r and t, as presented in the previous subsection, we obtain

$$\begin{aligned} r_{01}+ t_{01}t_{10} = \frac{(q_0 -q_1)^2}{(q_0 +q_1)^2} + \frac{2 q_0^2 2 q_1^2}{(q_0 +q_1)^2} = \frac{(q_0 +q_1)^2}{(q_0 +q_1)^2} = 1, \end{aligned}$$

(3.16)

with $r_{01}=-r_{10}$. Inserting this expression into the equation of $r_{\text {layer}}$ leads to

$$\begin{aligned} r_{\text {layer}} = \frac{r_{01} + r_{12} p^2}{1+r_{01}r_{12}p^2 }. \end{aligned}$$

(3.17)

The equation for the reflectivity of a thin layer (layer thickness $\varDelta$) can be further simplified for the case of identical materials on either side of the layer. In this case, $r_{01}=-r_{12}$ holds and (3.17) is simplified to

$$\begin{aligned} r_{\text {layer}} = \frac{r_{01}(1-p^2)}{1-r_{01}^2 p^2}. \end{aligned}$$

(3.18)

While the above equation is exact, further approximation can be performed when considering an angular range where refraction can be neglected (angle sufficiently large compared to critical angle). In this case $\left| r_{01}\right| \ll 1$ ($q\gg 1$), and the amplitude reflectivity r(q) can be written as

$$\begin{aligned} r(q) \approx \left( \frac{q_\text {c}}{2q}\right) ^2 \end{aligned}$$

(3.19)

Using these assumptions, the amplitude reflectivity of a thin layer becomes

$$\begin{aligned} r_{\text {layer}} = \frac{r_{01} (1-p^2)}{1-r_{01}^2 p^2} \approx r_{01}(1-p^2) \approx \left( \frac{q_\text {c}}{2q}\right) ^2 (1-\mathrm {e}^{iq\varDelta }) \end{aligned}$$

(3.20)

This can be rewritten as

$$\begin{aligned} r_{\text {layer}} = -\frac{16\pi \rho r_0}{4q^2}e^{iq\varDelta /2}(\mathrm {e}^{iq\varDelta /2}-\mathrm {e}^{-iq\varDelta /2}) \end{aligned}$$

(3.21)

$$\begin{aligned} = \left( \frac{16 \pi \rho r_o \varDelta }{2q}\right) \frac{\mathrm {e}^{iq\varDelta /2}}{2(q\varDelta /2)}-i\frac{e^{iq\varDelta /2}-e^{-iq\varDelta /2}}{2i} \end{aligned}$$

(3.22)

$$\begin{aligned} = -i\left( \frac{4\pi \rho r_0\varDelta }{q}\right) \left( \frac{\sin (q\varDelta /2)}{q\varDelta /2}\right) e^{iq\varDelta /2}. \end{aligned}$$

(3.23)

As the equation is supposed to describe the properties of a thin layer (layer thickness $\varDelta$), we assume $q\varDelta \ll 1$, which results in

$$\begin{aligned} r_\text {thin layer} \approx -i \frac{4\pi \rho r_0\varDelta }{q}=-i\frac{\lambda \rho r_0 \varDelta }{\sin (\alpha )}, \end{aligned}$$

(3.24)

using

$$\begin{aligned} q = \frac{4\pi \lambda }{\sin (\alpha )}. \end{aligned}$$

(3.25)

The expression for the reflectivity of a thin layer in (3.24) is known as the kinematical reflectivity. Note that this equation only holds for angles sufficiently above the critical angle.

Fig. 3.5

Illustration following [39]

Reflectivity of a stratified medium (e.g. multi-layerd thin film), with reflected and transmitted beams, as required for the Parratt algorithm. The reflectivity is calculated recursively from bottom to top. Each layer is parameterized with thickness $t_j$, roughness $\sigma _j$, and complex-valued index of refraction $n_j$. Profiles which are not step-wise constant can be approximated by a sequence of sufficiently thin slabs.

Next, we consider multiple layers (N layers) on top of an infinitely thick substrate, still following [37]. The reflectivity can be calculated using the Parratt algorithm [42], which is based on recursion. By definition, the Nth layer is on top of the substrate (see Fig. 3.5). The z-component of the wave vector, $k_{z,j}$ in the layer denoted j is determined by the wave vector $k_j$ and its x-component $k_{x,j}$, which is conserved through all layers $k_{x,j} = k_x$:

$$\begin{aligned} k_{z,j} = (n_j k)^2 -k_x^2 = (1-\delta _j + i\beta _j)^2 k^2 \simeq k_z^2 - 2\delta _j k^2 + 2i\beta _j k^2. \end{aligned}$$

(3.26)

The wave vector in the jth layer yields

$$\begin{aligned} q_j = \sqrt{q^2 -8k^2\delta _j +i8k^2\beta _j}. \end{aligned}$$

(3.27)

In a first step, the reflectivity is calculated for the interface of the Nth layer/substrate yielding

$$\begin{aligned} r'_{N,s} = \frac{q_N-q_{s}}{q_N+q_{s}}. \end{aligned}$$

(3.28)

Note that no multiple reflections have to be taken into account, since the substrate is assumed to be infinitely thick. Then, the reflectivity at the interface Nth layer/$N-1$th is considered, which can be written as

$$\begin{aligned} r_{N-1,N} = \frac{r'_{N-1,N} + r'_{N,s} p_N^2}{1 + r'_{N-1,N} r'_{N,s} p_N^2} , \end{aligned}$$

(3.29)

where the reflectivity expression for a single layer has been used. Here $r'_{j,j+1}$ denotes the reflectivity at the respective interface without considering multiple reflections, given by

$$\begin{aligned} r'_{j,j+1} = \frac{q_j-q_{j+1}}{q_j+q_{j+1}}. \end{aligned}$$

(3.30)

Next, the reflectivity at the interface of layers $(N-1)$ and $(N-2)$ is calculated using

$$\begin{aligned} r_{N-2,N} = \frac{r'_{N-2,N-1} + r'_{N,N-1}p_{N-1}^2}{1 + r'_{N-2,N-1} r'_{N-1,N} p_{N-1}^2 }. \end{aligned}$$

(3.31)

This procedure of determining the respective reflectivities can be repeated until the total reflectivity amplitude $r_{01}$ at the top interface 1st layer/vacuum is obtained. This iterative solution is the basis of reflectivity codes such as IMD by Windt [43]. Note that not only the intensity reflectivity as shown here, but the full fields inside the structure can be computed, by this or equivalent matrix methods with field vectors in each layer and boundary conditions at the interfaces taken into account. Typical reflectivity curves of periodic multilayers exhibit strong multilayer Bragg peaks, as well as total thickness oscillations known as Kiessig fringes. They reflect the interference of the reflected waves from the vacuum/layer and layer/substrate interfaces. From the period of these oscillations, the thickness of the layer can be determined.

2.3 Interfacial Roughness

Generalizing the results obtained for sharp or flat interface, where the density profile along z can be described by a step function, we now consider interfacial roughness, still following [37]. For real materials, we need to model a graded or rough interface. In this case, the density profile at the interface has to be modified. The density profile as a function of depth z can now be better described by an error function. Accordingly, the reflectivity of an ideal flat interface, which is given by (3.11), is modified in case of a rough interface by

$$\begin{aligned} R = R_F e^{-\sigma ^2 q_z^2}. \end{aligned}$$

(3.32)

We can derive this expression the following way (see [37]): First, we model the density profile of the interface by a function $\rho (z)$ which fulfills $\rho (z)\rightarrow 1$ for $z\rightarrow \infty$ and $\rho (z)\rightarrow 0$ for $z\rightarrow -\infty$ (see Fig. 3.6). Most commonly, $\rho (z)$ will be the error function (see below). Now we consider the contribution $\delta r(q_z)$ to the amplitude reflectivity r(q) from an infinitesimal thin slab at depth z

$$\begin{aligned} \delta r(q_z) = -i\frac{q_\text {c}^2}{4q}\rho (z)\,\text {d}z \end{aligned}$$

(3.33)

Fig. 3.6

Illustration following [39]

A vertically smeared out density profile $\rho (z)$ is used to model a rough interface.

and integrate over all infinitesimal thin layers to obtain the amplitude reflectivity r(q) as a superposition

$$\begin{aligned} r(q) = -i\frac{q_\text {c}^2}{4q}\int \limits _{-\infty }^{\infty }\rho (z)\,\text {e}^{iqz}\,\text {d}z~. \end{aligned}$$

(3.34)

Using partial integration, we find

$$\begin{aligned} r(q) = -i\frac{1}{iq}\frac{q_\text {c}^2}{4q}\int \limits _{-\infty }^{\infty }\frac{\text {d}\rho }{\text {d}z}\,{e}^{iqz}\,\text {d}z~. \end{aligned}$$

(3.35)

With 3.19 (limit of a perfect interface, $q\gg 1$), this yields

$$\begin{aligned} r(q) = r_F(q)\,\varPhi (q), \end{aligned}$$

(3.36)

using the definition

$$\begin{aligned} \varPhi (q)\equiv & {} \int \limits _{-\infty }^{\infty }\frac{\text {d}\rho }{\text {d}z}\,\text {e}^{iqz}\,\text {d}z. \end{aligned}$$

(3.37)

The function $\varPhi (q)$ describes the structure of the interface in reciprocal space (when modeling $\varPhi (r)$ with an error function in real space, as described below, its derivative ${\text {d}\rho }/{\text {d}z}$ will have the form of a Gaussian). The reflectivity (intensity) R(q), as measured in an experiment, is described by the so-called master formula [37]

$$\begin{aligned} \frac{R(q)}{R_F(q)} =\left| \frac{r(q)}{r_F(q)} \right| ^2 = \left| \int _{-\infty }^{\infty } \left( \frac{\text {d}\rho }{\text {d}z}\right) e^{iqz}\,\text {d}z\right| ^2 , \end{aligned}$$

(3.38)

which not only holds for a profile broadened by roughness, but more generally for any structured interface profile, within kinematic approximation. A common choice for the density profile of the interface $\rho (z)$ is the error function $\text {erf}(z)$ (see Fig. 3.6):

$$\begin{aligned} \rho (z) = \text {erf}\left( \frac{z}{\sqrt{2}\sigma }\right) . \end{aligned}$$

(3.39)

The parameter $\sigma$ gives a measure for the width of the graded region of the interface. This smeared out density profile can be regarded as an averaging of the rough surface. The derivative of the error function, ${\text {d}\rho }/{\text {d}z}$, is a Gaussian:

$$\begin{aligned} \frac{\text {d}\rho }{\text {d}z} = \frac{1}{\sqrt{2\pi \sigma ^2}}\text {e}^{-\frac{1}{2}\left( \frac{z}{\sigma }\right) ^2}. \end{aligned}$$

(3.40)

Hence we obtain

$$\begin{aligned} \frac{r(q)}{r_F(q)} = \int _{-\infty }^{\infty } \frac{\text {d}\rho }{\text {d}z}(z)\,e^{iqz}\,\text {d}z = \frac{1}{\sqrt{2\pi \sigma ^2}} \int _{-\infty }^{\infty } e^{-\frac{1}{2}\left( \frac{z}{\sigma }\right) ^2}e^{iqz}{\text {d}z} . \end{aligned}$$

(3.41)

By definition, the right hand side of (3.41) is the Fourier transform of ${\mathrm {d}\rho (z)}/{\mathrm {d}z}$. By computing the integral for the Gaussian case, we obtain

$$\begin{aligned} \frac{R(q)}{R_F(q)}=\left| \frac{r(q)}{r_F(q)}\right| ^2=\mathrm {e}^{-q^2\sigma ^2} ~. \end{aligned}$$

(3.42)

We can now discern two cases

• (i) $q_z \sigma \gg 1$, the surface is optically rough

• (ii) $q_z \sigma \ll 1$, the surface is optically flat.

Therefore, X-ray reflectivity can be used to quantify the roughness of a surface or interface. More importantly in the present context, mirror roughness severely affects the focusing intensity and field distribution.

3 X-ray Mirrors

Reflective optics in form of planar and curved mirrors are indispensable tools for synchrotron radiation science. Mirrors are encountered in almost every beamline for rejection of harmonics, which would also fullfill the Bragg condition of the monochromator. At fixed grazing angles of incidence $\alpha _i$, higher harmonics impinge above their critical angle $\alpha _c$, and are hence only very weakly reflected, while the fundamental is still below its critical angle and hence has a reflectivity r close to one. Mirror optics are also often preferred as the first optical element to take the white synchrotron beam, since a large surface area under grazing incidence can be used for cooling. In many beamlines, mirrors with moderate curvature are used to focus the beam to the desired position in the experimental hutch, in particular in the horizontal direction where the divergence is large. However, this type of focusing with large mirrors and large focal distances are designed for focal beam sizes of a few mm. Contrarily, micro- or nano-focusing for X-ray microscopy requires much shorter focal distances and much smaller radii of curvature. The most common arrangement of focusing mirrors for this purpose at synchrotron and FEL facilities is known as the Kirkpatrick-Baez (KB) mirror system, which we discuss in this section. Two major properties apply to KB focusing as to mirror optics in general: Firstly, it is non-dispersive, and hence well suited for broad bandpass or photon energy variation. Secondly, the efficiency is high since $r\simeq 1$ for $\alpha \le \alpha _c$.

Fig. 3.7

Geometry of KB focusing. A vertically (VFM) and horizontally focusing mirror (HFM), each with elliptical shape function, are aligned behind each other in orthogonal planes. Orthogonality and Bragg angles must be carefully aligned. Fixed curvature by polishing of the substrate and/or adaptively curved mirrors are both common

3.1 Kirkpatrick-Baez Geometry

A KB system consists of two crossed elliptically shaped mirrors [44], as sketched in Fig. 3.7. The mirror length is typically a factor of ten shorter than the large beamline mirrors, often around 10 cm. The mirror surface is polished to an elliptical shape. The ellipse is designed to have the first focal point at the radiation source, for example at the undulator exit, and the second at the focal plane of the experiment (sample position). Since ellipsoidal surfaces with two principle planes of curvature are difficult to fabricate, the two mirrors are elliptically curved only in one plane and are assembled perpendicular to each other. Rays are sequentially reflected off this orthogonal mirror pair, emulating a 3d ellipsoidal mirror surface.

In the design of a KB system, the following requirements must be considered. The mirrors must have

• a suitable reflectivity—so the grazing angle of incidence $\alpha$ is bounded by the critical angle $\alpha _\text {c}\sim \,\mathrm{mrad}$;

• a homogeneous phase of the reflected beam—so a well-shaped mirror with negligible figure errors to minimise aberrations;

• a well-polished surface—to reduce scattering which leads to artefacts for example in holographic imaging.

The first point limits the numerical aperture (NA) of reflective optics; since the critical angle scales linearly with X-ray wavelength, the achievable resolution $\lambda /\vartheta _\text {c}\sim {10}\,{\mathrm{nm}}$ is approximately constant with photon energy, and only depends on the material. This length scale would then be just one example of the more general limit postulated by Bergemann et al. for all kinds of X-ray focussing [1], as discussed in the introduction. The second and third points have been solved by technological progress. An important break-through has been achieved by the group of Yamauchi, by the development of the elastic emission machining (EEM) [35], which enabled the fabrication of elliptical surfaces with sub-nm figure errors and few ${\AA }$ roughness, even for mirror lengths of 100 mm and longer. As an example of this technology, Fig. 3.8 shows the height profile and deviations for the horizonally focusing mirror (HFM) of the GINIX instrument at the P10 beamline of the PETRAIII storage ring [45].

Fig. 3.8

Example of an elliptical KB mirror profile, with the surface (blue) polished to elliptical shape as a function of the position on the mirror (x-axis). The mirror is positioned at about 87 m behind the undulator at the P10 beamline of the PETRAIII storage ring [45], and has a focal length of about 300 mm. The deviations from the perfect height profile are also shown (red curve) and have a rms roughness of only $\sigma \simeq 0.1$ nm. The mirror is made of $\text {SiO}_2$ and coated with $\text {Rh}$

Geometrically, the elliptical shape yields a perfect point focus, providing a constant and real-valued reflectivity along the active surface. However, under total reflection, an angle-dependent phase-shift $\varphi (\alpha )$ occurs. From the Fresnel reflectivity formula $r=\frac{\alpha \,-\,\alpha '}{\alpha \,+\,\alpha '}$ with $\alpha '=\cos ^{-1}(\cos \alpha /n)\in i\,\mathbb {R}$ for $\alpha <\alpha _\text {c}$ and $n<1$, we obtain as phase shift $\varphi (\alpha )$

$$\begin{aligned} \varphi (\alpha ) = 2\tan ^{-1}\left( \frac{-\sqrt{2\delta -\sin ^2\alpha }}{\sin \alpha }\right) , \end{aligned}$$

(3.43)

where $\alpha$ varies along the mirror’s surface [46]. This phase gradient, $\nabla \varphi (\alpha )$, leads to a small shift of the beam. It is connected to the Goos-von Hänchen effect. Although totally reflected, an evanescent wave enters the medium to experience a small phase-lag. Numerically, this lateral shift of the focal spot is only on the order of a few nm. In addition, the index of refraction $n=1-\delta +i\beta$ has an imaginary part due to absorption. By ways of this imaginary component, the angle $\alpha '$ changes slightly, yielding a second phase contribution to $\varphi (\alpha )$. Again, the effect on the lateral position of the focal spot is in the nm range. The spot size, however, is unaffected. Hence, albeit the evanescent wave and absorption of the reflecting material, an elliptically shaped mirror operating under total external reflection provides efficient point-to-point focusing. However, since $\alpha _i\le \alpha _c$ must be fulfilled for all points on the reflecting surface, the numerical aperture is quite limited. To overcome this limitation without severe reduction in r, multilayer (ML) coatings are used.

3.2 Multilayer Mirrors

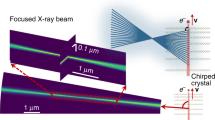
For “simple” mirrors based on total external reflection, the numerical aperture is limited by the critical angle $\vartheta _\text {c}\sim 4\,\text {mrad}$ for hard X-rays and typical coating materials, e.g. at 14 keV and Rh coating. Hence, also the focal spot size has a lower limit of about 50 nm, if we pose reasonable bounds on all other geometrical properties. To enhance the reflectivity at higher angles of incidence, multilayer coatings with alternating high and low density layers are applied. As known from planar multilayers, the first Bragg peak assures high reflectivity at angles of incidence which can be easily a factor of ten higher than $\alpha _c$, depending on the multilayer period $\varLambda$. Common materials for hard X-rays are e.g. W, Mo, Ta for the high density layers, and B$_4$C, C or Si for the low density layers. For a KB system, one expects that these layers and the substrate must follow the shape functions of conformal ellipses, with the X-ray source (undulator) and focal spot as the two focal points. However, due to refraction inside the multilayer structure, the layer shapes need to be slightly modified and varied across the mirror surface [47, 48]. Using such multilayer mirrors with a laterally graded layer period, it was for the first time possible to “Break the 10 nm barrier in hard X-ray focusing” [5].

In order to design optimal multilayer mirrors, e.g. for the upgraded beamline ID16a at the ESRF, an analytical treatment of dynamic X-ray diffraction inside such a graded multilayer structure in elliptical geometry has been developed in [49, 50]. Here we briefly describe this wave-optical theory of nano-focusing X-ray multilayer mirrors based on the Takagi-Taupin theory of strained crystals. The geometry and system of coordinates is shown in Fig. 3.9. As a natural choice, we use elliptical coordinates (t, s) given by

$$\begin{aligned} t := \frac{r_0+r_1}{2}, \qquad s := \frac{r_0-r_1}{2}, \end{aligned}$$

(3.44)

where $r_0$ is the distance of a point from the source S, and $r_1$ is the distance of this point to the focus F. We derive the Takagi-Taupin (TT) equations from the Helmholtz equation of a scalar field $\psi (s,t)$, here written not with the index of refraction n, but with the susceptibility $\chi =n^2-1$:

$$\begin{aligned} \nabla ^2\psi (s,t) + k^2\left[ 1+\chi (s,t)\right] \psi (s,t) = 0~. \end{aligned}$$

(3.45)

For a (quasi-)periodic structure, we write the susceptibility as a truncated Fourier series to first order

$$\begin{aligned} \chi (s,t) = \chi _0 + \chi _{\bar{1}}\exp \left( -2ikt\right) + \chi _1\exp \left( 2ikt\right) ~. \end{aligned}$$

(3.46)

Then, we decompose the field $\psi$ into two components: the incoming wave $\psi _0\exp \left( ikr_0\right)$ diverging from the source, and the reflected wave $\psi _1\exp \left( -ikr_1\right)$ converging to the focus. To re-write the Helmholtz equation, we need the folowing expressions:

$$\begin{aligned} \psi (s,t)= & {} \psi _0\exp \left( ik(s+t)\right] + \psi _1\exp \left[ ik(s-t)\right] \end{aligned}$$

(3.47)

$$\begin{aligned} \chi \psi\approx & {} \left( \chi _0\psi _0+\chi _1 \psi _1\right) \exp \left[ ik(s+t)\right] \end{aligned}$$

(3.48)

$$\begin{aligned}&\qquad&+\left( \chi _0\psi _1+\chi _{\bar{1}}\psi _0\right) \exp \left[ ik(s-t)\right] \end{aligned}$$

(3.49)

$$\begin{aligned} \nabla ^2\psi (s,t)= & {} \alpha ^2(\partial _s^2\psi ) + \beta ^2(\partial _t^2\psi ) + \frac{t(\partial _t\psi )-s(\partial _s\psi )}{t^2-s^2} \end{aligned}$$

(3.50)

$$\begin{aligned} \alpha ^2(s,t)= & {} \frac{c^2-s^2}{t^2-s^2} = \cos ^2\vartheta , \quad \beta ^2(s,t) = \sin ^2\vartheta \end{aligned}$$

(3.51)

Here, $\vartheta$ is the local angle of incidence, and 2c the distance between source and focus.

Fig. 3.9

a Multilayer coatings of KB mirrors approximately follow the shape functions of confocal ellipses. b Definition of the elliptical coordinates used for the Tagaki-Taupin theory

Assuming slowly varying envelopes, $\nabla ^2\psi _{0,1}(s,t)\approx 0$, and defining $u_h:=k\chi _h/2$, we obtain

$$\begin{aligned} (\alpha ^2\partial _s+\beta ^2\partial _t)\psi _0= & {} i(u_0\psi _0+u_1 \psi _1) - \psi _0/2(t+s), \end{aligned}$$

(3.52)

$$\begin{aligned} (\alpha ^2\partial _s-\beta ^2\partial _t)\psi _1= & {} i(u_0\psi _1+u_{\bar{1}}\psi _1) - \psi _1/2(t-s)~. \end{aligned}$$

(3.53)

With $\alpha ,\beta =\text {const}$, these Takagi-Taupin equations are valid in the flat case; here, these coefficients are dependent on coordinates as given above. When applied to curved multilayer mirrors, the bilayer period $\varLambda ^\text {B}$ of the stacked system following Bragg’s law is given by

$$\begin{aligned} \varLambda ^\text {B}= & {} \frac{\lambda }{2\sin \vartheta (s,t)} = \frac{\lambda }{2\beta }~. \end{aligned}$$

(3.54)

Now we take refraction of the X-ray beam due to the average index of refraction inside the ML structure into account. The modified Bragg condition then reads

$$\begin{aligned} \varLambda ^\text {mB}= & {} \frac{\lambda }{2\sqrt{n^2-\cos ^2\vartheta }} \approx \frac{\lambda }{2\sqrt{\beta ^2-2\delta }}, \end{aligned}$$

(3.55)

where $\delta =(\delta _1+\delta _2)/2$ is the average decrement, assuming equal thicknesses of the bilayers. For $\vartheta _\text {B}\ge 3\vartheta _\text {c}\approx 3\sqrt{2\delta }$, a good approximation is given by

$$\begin{aligned} \varLambda ^\text {mB}\approx & {} (1+\delta /\beta ^2)\,\varLambda ^\text {B}~. \end{aligned}$$

(3.56)

The increased layer thickness is accounted for by using a pseudo-Fourier series of $\chi (s,t)$, in which the exponentials are modified according to $\exp \left( \pm 2ikt\right) \mapsto \exp \left( \pm 2ikt(1-\delta /\beta ^2)\right]$. Replacing further $\psi _1$ by $\psi _1':=\psi _1\exp \left( -2ikt\delta /\beta ^2\right)$, the modified propagation constant $u_0$ in the second TT-equation is replaced by

$$\begin{aligned} u_0'\approx & {} u_0-2(\alpha ^2\partial _s\varphi -\beta ^2\partial _t\varphi )k, \qquad \varphi =(t-t_0)\delta /\beta ^2~. \end{aligned}$$

(3.57)

Assuming a constant $\vartheta$, it can be shown that $u_0'=-u_0^*$; in other words, while the first TT equation gives rise to a phase-lag due to refraction; the modified second equation now yields an anti-phase lag of the reflected wave, in fact correcting for refraction. In the curved case, the next-to-leading order term reads

$$\begin{aligned} u_0'\approx & {} u_0 + 2\delta k\left[ 1-2\frac{\alpha ^2\varDelta t}{\beta ^2(t+s)}\right] , \qquad \varDelta t:=t-t_0, \end{aligned}$$

(3.58)

with $t=t_0$ along the entrance surface. For realistic parameters, this curvature term leads to a small numerical correction on the required bi-layer spacing.

Reflectivity curves in dynamical diffraction are not symmetric; in particular, the peak intensity does not occur at the nominal Bragg angle. For further numerical optimisation, a scaling factor f interpolating between Bragg and modified Bragg layer spacing is now introduced; we define

$$\begin{aligned} \varLambda (f):= & {} \varLambda ^\text {B} + f\times (\varLambda ^\text {mB}-\varLambda ^\text {B}), \qquad f\in \mathbb {R}. \end{aligned}$$

(3.59)

The TT system of coupled differential equations is solved numerically and for different parameters f. Figure 3.10 summarises a simulation of a ML mirror for the ESRF beamline ID-16a. Both the focal spot size $\varDelta$ (red line), peak intensity in the focus (blue points), and the standard deviation of the reflected phase (green dashes) is shown as a function of optimisation parameter f. Based on the simulations, a value of $f=0.9$ yields the best results, and a theoretical focal spot size of about 5 nm (FWHM).

Fig. 3.10

From [51]

Simulated focus sizes for the geometry of the ESRF KB nano-focus upgrade beamline: a horizontal ML and b vertical ML, as a function of Bragg modification factor f. The focus sizes (red curve with reddish error bands) have been obtained from $\text {sinc}^2$-fits to the intensity in the focus. Standard deviation of reflected phase (along the ML surface) is shown in green on a logarithmic scale; peak intensity in the focus is shown in blue. Dotted line shows the diffraction limit. Simulation has been carried out for a point-source.

4 X-ray Waveguides

Compared to other spectral domains, notably that of visible and infrared light, waveguide optics is much less developed in the X-ray range. Total reflection in a thin film of low electron density surrounded by high electron density is the basis for guiding X-ray radiation. The first waveguiding effects for X-rays used propagation in planar (straight) thin film structures [33, 52,53,54,55,56], followed by the development of two-dimensional channel waveguides [30, 57], which have posed significant fabrication challenges up to recently [58,59,60]. Progress in waveguide fabrication has led to a usable exit flux outpassing in some case $10^9$ photons per second [58]. If optimized for small beam size, X-ray waveguides with beam confinement of sub-10 nm (FWHM) have also been demonstrated [32]. In the context of this volume, the use of X-ray waveguides to create a monochromatic and fully coherent secondary quasi-point source is of particular importance. This coherent point source is ideally suited for X-ray holography and coherent imaging techniques. This has resulted in (holographic) propagation imaging at unprecedented resolution and image quality [61]. Figure 3.11 illustrates the basic geometry of using X-ray waveguides to record in-line X-ray holograms. 

Fig. 3.11

Basic schematic of using a waveguide exit beam for holographic X-ray imaging. By selecting the waveguide to sample distance, objects can be imaged in a full field configuration and geometrically magnified holograms of nanoscale objects can be recorded. The exit field behind the sample is reconstructed by phase retrieval algorithms, which are often more robust in this regime, compared to far-field coherent diffractive imaging

4.1 Waveguide Modes: The Basics

X-ray waveguides can be treated as a special case of the general theory of electromagnetic waveguides, as presented in the classic textbook by Marcuse [62], which we follow here. The only particularities associated with waveguiding in the X-ray regime derive from the nature of the X-ray index of refraction and the short wavelength. Adapted to the X-ray case, notation and parameterisations used here, closely follow previous original work presented in [54, 57, 63]. We start from Maxwell’s equations, written down for an isotropic, linear, nonconducting, and nonmagnetic medium as

$$\begin{aligned} \nabla \times \mathbf {\mathcal {E}}= & {} -\frac{\partial {\mathbf {\mathcal {B}}}}{\partial t}, \end{aligned}$$

(3.60)

$$\begin{aligned} \nabla \times \mathbf {\mathcal {H}}= & {} \frac{\partial {\mathbf {\mathcal {D}}}}{\partial t}, \end{aligned}$$

(3.61)

$$\begin{aligned} \nabla \cdot \mathbf {\mathcal {D}}= & {} 0, \end{aligned}$$

(3.62)

$$\begin{aligned} \nabla \cdot \mathbf {\mathcal {B}}= & {} 0, \end{aligned}$$

(3.63)

where $\mathbf {\mathcal {E}}$ and $\mathbf {\mathcal {H}}$ denote the electric and magnetic field, $\mathbf {\mathcal {B}}=\mu _0 \mathbf {\mathcal {H}}$ the magnetic induction, and $\mathbf {\mathcal {D}}=\varepsilon _0\varepsilon \mathbf {\mathcal {E}}=\varepsilon _0n^2(r)\mathbf {\mathcal {E}}$ the electric displacement. We then take the curl of (3.60)

$$\begin{aligned} \nabla \times (\nabla \times \mathbf {\mathcal {E}}) = -\mu _0\partial _t(\nabla \times \mathbf {\mathcal {H}}) {\mathop {=}\limits ^{(3.61)}} -\mu _0\varepsilon _0 n^2 \partial _t^2 \mathbf {\mathcal {E}} ~\, \end{aligned}$$

(3.64)

and use $\nabla \times (\nabla \times \mathbf {\mathcal {E}}) = \nabla (\nabla \cdot \mathbf {\mathcal {E}}) - \nabla ^2 \mathbf {\mathcal {E}}$ to obtain the source-free (homogeneous) wave equation

$$\begin{aligned} \nabla ^2 \mathbf {\mathcal {E}} -\mu _0\varepsilon _0n^2\partial _t^2 \mathbf {\mathcal {E}} =0 , \end{aligned}$$

(3.65)

which holds for $\nabla \cdot \mathbf {\mathcal {E}} =0$. This is warranted for section-wise constant index n, or in the approximation of a weakly varying index, since in this case we can neglect $\nabla \cdot \mathbf {\mathcal {E}} \simeq 0$, as can be verified from

$$\begin{aligned} 0=\nabla \cdot \mathbf {\mathcal {D}} = \varepsilon _0 (\nabla n^2 ) \cdot \mathbf {\mathcal {E}} + \varepsilon _0 n^2 \nabla \cdot \mathbf {\mathcal {E}} \simeq \varepsilon _0 n^2 \nabla \cdot \mathbf {\mathcal {E}}~. \end{aligned}$$

(3.66)

Analogous to (3.65), we can also obtain the wave equation for the magnetic field

$$\begin{aligned} \nabla ^2 \mathbf {\mathcal {H}} = -\mu _0\varepsilon _0n^2\partial _t^2\mathbf {\mathcal {H}}~. \end{aligned}$$

(3.67)

Next we consider a solution of Maxwell’s equation which has the particular form of a guided wave, i.e.

$$\begin{aligned} \mathbf {\mathcal {E}}&= \mathbf {E}(\mathbf {r_\perp })\,e^{i(\omega t-\mathbf {k}\cdot \mathbf {r})}, \end{aligned}$$

(3.68)

$$\begin{aligned} \mathbf {\mathcal {H}}&= \mathbf {H}(\mathbf {r_\perp })\,e^{i(\omega t-\mathbf {k}\cdot \mathbf {r})}, \end{aligned}$$

(3.69)

where $\omega$ is the angular frequency, $\mathbf {k}=k \mathbf {e_k}$ the wave vector with magnitude (wave number) $2\pi /\lambda$, and $\mathbf {r_\perp }=\mathbf {r}-(\mathbf {e_k}\cdot \mathbf {r}) \mathbf {e_k}$ is a position vector perpendicular to $\mathbf {k}$. In other words, in a guided mode we require the field to be stationary with respect to the propagation axis, i.e. $\mathcal {E}$ and $\mathcal {H}$ are only functions of the coordinates perpendicular to the propagation axis. Unless they are constant (plane wave), this requires the presence of matter. More precisely, a distribution of the index of refraction $n(\mathbf {r_\perp })$ with the translational symmetry along $\mathbf {k}$, which is chosen such that the field is guided along the propagation axis while being confined in the orthogonal direction(s). A simple example is sketched in Fig. 3.12, with z as the propagation axis, and a stepwise constant index of refraction profile n(x)

$$\begin{aligned} n(x) = {\left\{ \begin{array}{ll} n_1 &{}\quad \text {if } \, -d/2<x<d/2, \\ n_2 &{}\quad \text {else ,} \end{array}\right. } \end{aligned}$$

(3.70)

Fig. 3.12

Sketch of a simple slab waveguide geometry. The guiding layer material with high index of refraction is sandwiched by a low index surrounding material (“cladding”). For X-rays, air/vacuum with $n_1\approx 1$ is an ideal guiding medium, and the index of refraction of the metal or semiconductor cladding is given by $n_2 = 1-\delta$

describing a simple planar waveguide geometry with guiding layer of refractive index $n_1$ and thickness d (guiding core) sandwiched between two semi-infinite cladding regions of refractive index $n_2<n_1$. The profile function n(x) parameterizes a planar waveguide with one-dimensional beam confinement (1DWG), while two-dimensional confinement would require a corresponding two-dimensional profile function n(x, y), describing for example a channel waveguide (2DWG) of cylindrical or rectangular cross section. For the given geometry of a planar waveguide, we hence have

$$\begin{aligned} \mathcal {E}_j = E_j(x)e^{i(\omega t-\beta z)}, \end{aligned}$$

(3.71)

$$\begin{aligned} \mathcal {H}_j = H_j(x)e^{i(\omega t-\beta z)}~. \end{aligned}$$

(3.72)

Inserting this ansatz in (3.60) and (3.61) yields six differential equations for the field components, out of which two sets are uncoupled, describing the transverse electric (TE) modes (modes without electric component in propagation direction)

$$\begin{aligned} i\beta E_y= & {} -i\omega \mu _0 H_x, \end{aligned}$$

(3.73)

$$\begin{aligned} \partial _x E_y= & {} -i\omega \mu _0 H_z, \end{aligned}$$

(3.74)

$$\begin{aligned} -i \beta H_x-\partial _x H_z= & {} i\omega \varepsilon _0n^2(x)E_y, \end{aligned}$$

(3.75)

and the transverse magnetic (TM) modes (modes without magnetic component in propagation direction)

$$\begin{aligned} -i\beta E_x -\partial _x E_z= & {} -i\omega \mu _0 H_y, \end{aligned}$$

(3.76)

$$\begin{aligned} i\beta H_y= & {} i \omega \varepsilon _0 n^2(x) E_x, \end{aligned}$$

(3.77)

$$\begin{aligned} \partial _x H_y= & {} i \omega \varepsilon _0 n^2(x) E_z~. \end{aligned}$$

(3.78)

For TE modes $E_x,E_z,H_y=0$ (for TM modes $E_y,H_x,H_z=0$). Expressing the field components along x and z by those in y, we obtain for the two sets of modes

$$\begin{aligned} \partial _x^2 E_y + \big (k^2n^2(x)-\beta ^2\big ) E_y= & {} 0, \end{aligned}$$

(3.79)

$$\begin{aligned} \partial _x^2 H_y + \big (k^2n^2(x)-\beta ^2\big ) H_y= & {} 0~. \end{aligned}$$

(3.80)

These equations are sometimes denoted as reduced wave equations. For X-rays, the propagation is extremely forward directed, i.e. the internal reflections angles are on the order of a few mrad, much smaller than the Brewster angle. For this reason, the TM and TE solutions degenerate, and scalar diffraction theory holds. Correspondingly, it is sufficient to consider a single scalar field $\psi$. In fact, we could also start directly from the scalar wave equation

$$\begin{aligned} \nabla ^2 \psi - \frac{n^2}{c^2} \partial _t^2 \psi = 0 \quad \quad \forall \,\psi \in \lbrace E_x,E_y,E_z,H_x,H_y,H_z \rbrace , \end{aligned}$$

(3.81)

with $c=\frac{1}{\sqrt{\varepsilon _0 \mu _0}}$ the speed of light, and $n=\sqrt{\varepsilon _r\mu _r}$ the refractive index, keeping in mind that the components are in general not independent. For forward directed propagation of X-rays, the scalar wave equation (3.81) is, an excellent approximation. The field $\psi$ can be written as a superposition of monochromatic fields $\varPsi _\omega$ (spectral decomposition)

$$\begin{aligned} \psi = \psi (\mathbf {r},t) = \frac{1}{2 \pi } \, \int _0^{\infty } \psi _{\omega }(\mathbf {r}) \,\mathrm {e}^{-i \omega t}\, d\omega , \end{aligned}$$

(3.82)

if stationary quasi-monochromatic waves are considered, i.e. $\psi \rightarrow \psi _{\omega }$ and $n \rightarrow n_{\omega }$. In this case time dependence is harmonic

$$\begin{aligned} \psi (\mathbf {r},t) = U(\mathbf {r}) e^{-i\omega t}, \quad u(\mathbf {r}) \in \mathbb {C} , \end{aligned}$$

(3.83)

and we can write down a differential equation only for the complex amplitude $U(\mathbf {r})$ by inserting (3.83) in (3.82)

$$\begin{aligned} \big (\nabla ^2 U(\mathbf {r})\big )\,e^{-i\omega t} + \frac{n^2}{c^2}\omega ^2 U(\mathbf {r})\,e^{-i\omega t} = 0 \end{aligned}$$

(3.84)

$$\begin{aligned} \Rightarrow \nabla ^2 U(\mathbf {r}) + n^2(\mathbf {r}) k_0^2 \,U(\mathbf {r}) = 0 ~. \end{aligned}$$

(3.85)

Equation (3.85) is the scalar Helmholtz equation (HE). Here, the wave number in vacuum is given by $k_0=\frac{\omega }{c}= \frac{2\pi }{\lambda }$. Another notation is $k(\mathbf {r})=n(\mathbf {r})k_0$ where $k(\mathbf {r})$ is the wave number in a medium. To simplify the notation, we will write k for $k_0$ in the following, and refer k to the absolute value of the wave number in vacuum. The component $k_z$ will be denoted as $k_z=\beta$. Again, we use a separation ansatz for guided modes

$$\begin{aligned} U(x,z) = u(x)\,e^{-i\beta z}, \end{aligned}$$

(3.86)

where $\beta$ is called the propagation constant. Insertion in (3.85) then yields the one-dimensional reduced wave equation for u(x),

$$\begin{aligned} \frac{d^2}{dx^2} u(x) + \big (n^2(x)k^2 - \beta ^2\big ) u(x) = 0 , \end{aligned}$$

(3.87)

which has the same form as (3.80). Hence we can either first work with Maxwell’s equation and then use scalar approximation later in the reduced Helmholz equation, or start from the scalar wave equation, and arrive at the same result. Note that in order to have $\beta$ real, we have to assume the refractive index to be real and thus at least initially ignore absorption. More generally, the modes will also be affected by the imaginary part of the index, but in practice it is sufficient to treat absorption a posteriori by an effective (weighted) absorption coefficient for each mode. Even though this does not matter in scalar approximation, u(x) could be taken to represent the horizontal component of the electric field, considering the so-called transverse electric (TE) modes of the waveguide. This requires u(x) to be continuous at the interfaces. Furthermore, for guided modes we require the field to vanish far inside the cladding, i.e., u(x) must approach zero in the limit $|x|\rightarrow \infty$. For symmetric potential functions (here: index of refraction profiles), the eigenfunctions (modes) have defined parity, i.e. are symmetric or anti-symmetric. A general form of a symmetric function which solves 3.87 and which does not diverge, is given by

$$\begin{aligned} u(x) = {\left\{ \begin{array}{ll} A\cos (\kappa x) \quad &{}\text {if } 0<|x|\le d/2 \\ C\,e^{\gamma \vert x\vert } \quad &{}\text {if } |x|>d/2 \end{array}\right. } \end{aligned}$$

(3.88)

where A and C are constants. Requiring u(x) and its derivative $u'(x)$ to be continuous functions at $x=\pm d/2$, we get

$$\begin{aligned} A\cos (\kappa d/2)= & {} C\,e^{-\gamma d/2},\end{aligned}$$

(3.89)

$$\begin{aligned} -A\kappa \sin (\kappa d/2)= & {} -\gamma C\,e^{-\gamma d/2} ~. \end{aligned}$$

(3.90)

Dividing (3.90) by (3.89), we obtain a transcendental equation

$$\begin{aligned} \kappa \tan \left( \kappa \frac{d}{2} \right) = \gamma ~. \end{aligned}$$

(3.91)

Symmetric modes are found by solving this transcendental equation. For anti-symmetric modes

$$\begin{aligned} u(x) = {\left\{ \begin{array}{ll} B\sin (\kappa x) \quad &{}\text {if } 0<|x|\le d/2, \\ C\,e^{\gamma \vert x\vert } \quad &{}\text {if } |x|>d/2, \end{array}\right. } \end{aligned}$$

(3.92)

we have correspondingly

$$\begin{aligned} -\kappa \cot \left( \kappa \frac{d}{2}\right) = \gamma . \end{aligned}$$

(3.93)

Using the definitions

$$\begin{aligned} \xi := \frac{\kappa d}{2} = \sqrt{k^2n_1^2-\beta ^2}\, \frac{d}{2} \end{aligned}$$

(3.94)

and

$$\begin{aligned} V := \sqrt{n_1^2 - n_2^2}\,\kappa d \approx \sqrt{2\delta _2-2\delta _1}\,\kappa d \end{aligned}$$

(3.95)

the transcendental equation can be rewritten as

$$\begin{aligned} \xi \tan (\xi ) = \sqrt{\frac{V^2}{4}-\xi ^2} \end{aligned}$$

(3.96)

for symmetric modes and

$$\begin{aligned} -\xi \cot (\xi ) = \sqrt{\frac{V^2}{4}-\xi ^2} \end{aligned}$$

(3.97)

for antisymmetric modes, respectively. The transcendental equation determines a discrete set of modes $\xi _m$, with $0\le m \le N-1$. The total number of guided modes N is given by

$$\begin{aligned} N = \left\lceil \frac{V}{\pi } \right\rceil , \end{aligned}$$

(3.98)

where $\lceil \rceil$ denotes the Gauss bracket (rounding to the next integer). The recipe to compute a mode, is then to solve the transcendental equation, and to compute the parameters in sequence $\xi _m \rightarrow \kappa _m \rightarrow \beta _m \rightarrow \gamma _m$, to obtain u(x). The smallest $\xi _0$ which solves (3.96) determines the fundamental mode

$$ u_0(x)={\left\{ \begin{array}{ll} \cos (\kappa _0 x) &{}\text {if }|x|<d/2\\ \cos (\kappa _0 d/2)e^{-\gamma _0 (|x|-d/2)} &{}\text {else.} \end{array}\right. } $$

Fig. 3.13

Graphical representation of the transcendental mode equation with the waveguide parameter V. Each intersection corresponds to a mode. Symmetric modes are indicated in blue, anti-symmetric modes by in orange. For given $V=8.44$, the waveguide supports $N = \lceil \frac{V}{\pi }\rceil =3$ modes

Fig. 3.14

Mode amplitudes (left) and intensities (right), corresponding to the solution of the transcendental equation shown in Fig. 3.13. The cladding is shaded in gray

In order to interpret a mode in a geometric optical picture it is helpful to consider the complete field in the guiding layer, e.g. of a symmetric mode, with mode envelope $u(x) = \cos (\kappa x)$ (Fig. 3.14)

$$\begin{aligned} U(x,z) = u(x)\,e^{i(\omega t-\beta _m z)} = \frac{A}{2}\left( e^{i(\omega t-\beta _m z-\kappa x)}+e^{i(\omega t-\beta _m z+\kappa x)}\right) ~. \end{aligned}$$

(3.99)

The right hand side corresponds to two internally reflected plane waves (guided by total reflection), or beams in the geometric optical model, with wave vectors $\mathbf {k}$

$$\begin{aligned} \mathbf {k}_{\pm }= \begin{pmatrix} \beta \\ 0\\ \pm \kappa \end{pmatrix}, \end{aligned}$$

(3.100)

as sketched in Fig. 3.17.

Fig. 3.15

Adapted from [32]

a Multi-modal propagation inside a planar waveguide with thin film sequence Ge/Mo [$d_i=30$ nm]/C [$d=35$ nm]/Mo[$d_i=30$ nm]/Ge, simulated for 17.5 keV and front coupling (plane wave). The intensity distribution is plotted in the range of 221–261$\,\upmu$m behind the waveguide entrance, showing a mode beating along the propagation direction z. b Intensity profiles corresponding to the dashed lines in a illustrating the interference due to multi-modal propagation. c A Fourier transformation with respect to z reveals both the shape of the guided modes (vertical profile), and the propagation constant (proportional to the horizontal offset). d FWHM of the simulated near-field distribution (top) and far-field distribution (bottom) as a function of z.

4.2 Coupling and Propagation

The modes $u_m(z)$ are eigenfunctions of the waveguide potential. For a rectangular profile they consist of a sine or cosine term with $m+1$ antinodes in the guiding layer, and an exponentially decaying evanescent wave in the cladding, as derived above. For more general potential shapes, the mode function can also be found numerically by integration via Numerov’s method (shooting method) [64]. For given geometry and boundary conditions, propagation can be calculated by finite difference (FD) calculations  as presented in Chap. 2, and the different modes can be dissected by means of Fourier transformation along z, see Fig. 3.15. Neglectingmodes and the corresponding interference effects is well described by linear combination of all N guided modes

$$\begin{aligned} \psi (x,z)=\sum \limits _{m=0}^{m_\text {max}} c_m u_m(x)\exp \left( -i\beta _m z\right) . \end{aligned}$$

(3.101)

In front-coupled waveguides, the coefficient $c_m$ is given by an overlap integral of the incident field $\psi _\text {in}$ and $u_m$ [29, 52]

$$ c_0=\frac{1}{||u_m||^2}\int \psi _\text {in}(x) u_m(x)\,dx~. $$

Absorption can be accounted for by a factor $\exp \left( -\mu _{\text {eff},m}x\right)$ in the right hand side of equation (3.101), with an “effective linear absorption coefficient” $\mu _{\text {eff},m}$ given by a mode-weighted average of the absorption coefficient profile $\mu (x)$ [65]

$$ \mu _{\text {eff},m}=\frac{1}{||u_m||^2}\int |u_m(x)|^2\mu (x)\,dx. $$

For a vacuum guide, only the intensity fraction in the cladding contributes to the absorption of the mode. The transition from multi-modal to mono-modal regime as a function of guiding layer thickness d is illustrated by Fig. 3.16a, b. Note that d can also be tapered along the optical axis as in (c) to concentrate the field. Instead of coupling from the side, a beam can also be coupled in through the cladding, via the so-called resonant beam coupler (RBC) geometry, see Fig. 3.17a. In this case, modes can be excited selectively, even if the waveguide support multiple modes. Figure 3.17 also shows a simulation depicting the position of a waveguide in the focal plane of a KB-mirror. By computing the propagation for different incoming realisations of the (stochastic) field, the guiding and filtering of a waveguide can be studied [66].

Fig. 3.16

Field intensity distribution (logarithmic color code) in a a nearly mono-modal, b a multi-modal air/silicon waveguide, simulated for plane wave incoming 8keV radiation with unit intensity. Due to higher absorption of the $m=1$ mode, only the fundamental mode $m=0$ persists in the $d=16$ nm guide, while $N=5$ modes propagate in the $d=96$ nm guide. c By tapering the exit intensity can be increased, and single mode radiation is achieved at the exit. The intensity gain between a and c for same exit width is directly visible

Fig. 3.17

adapted from [66]

Sketch of different coupling geometries. a Resonant beam coupling (RBC). The waveguide is illuminated by an incoming plane wave under grazing incidence with $\alpha _i$ tuned to a mode. Modes can be exited in an interval $\alpha _c^{\text {core}} \le \alpha _i \le \alpha _c^{\text {cladd}}$, where $\alpha _c^{\text {core}}$ and $\alpha _c^{\text {cladd}}$ denote the critical angles of total reflection for waveguide core and cladding, respectively. The mode is exited via an evanescent wave in the top cladding. b Front coupling scheme, illustrated in a ray-optical picture, with the mode formed by up and down reflected rays in the guiding core, according to (3.100). c Finite difference simulation in coupling a pre-focused beam (KB mirrors) into a silicon-air waveguide, propagation and out coupling,

4.3 Fabrication and Characterisation of X-ray Waveguides

To isolate a guided X-ray beam with a cross section down to about 10 nm close to the fundamental limit [1], long channels are needed with aspect ratios (length to width) in the range of $10^4{-}10^6$, depending on the photon energy E and cross section d. This is because the radiation entering at the sides of the over-illuminated channel entrance (radiative modes) has to be absorbed in the cladding material. Not only the small cross section, but also the high aspect ratios impose a significant challenge in fabrication. Waveguide structures for one-dimensional beam confinement by planar waveguides (1DWG) are easily obtained by thin film deposition techniques, but most applications require two-dimensional waveguides (2DWG). Using guiding channels of polymer structured by e-beam lithography and coated with metal or semiconductor cladding, 2DWGs were first realized in [57] and later improved by [30]. An alternative fabrication scheme based on dry etching of channels into silicon wafers and subsequent capping by wafer bonding makes it possible to employ an empty guiding core (air or vacuum) and hence to minimize absorption in particular for lower photon energies [60]. This has enabled a waveguide exit flux on the order of $10^8$ ph/s (P10 beamline of the PETRA III storage ring of DESY [67].

Fig. 3.18

From [60]

Fabrication of lithographic waveguides. a Sketch of waveguide processing sequence: resist deposition, e-beam exposure, reactive ion etching, mask removal, and finally wafer bonding. b Schematic of air-filled channel capped by a top wafer bonded to the substrate. c, d, e SEM micrographs of waveguide channel entrances. f Photograph of waveguide chip as cut by the wafer dicing machine.

Figure 3.18 illustrates the fabrication of waveguide channels in silicon by e-beam lithography and subsequent wafer bonding, according to [60]. A spin-coated poly-methyl-methacrylate (PMMA) is used as positive e-beam resist. The desired pattern of an array of waveguide channels is written by moving an interferometric laser stage below a stationary electron beam, in order to achieve the required channel length (of a few mm’s) without stitching errors. The developed resist then provides the etching mask for pattern transfer into the semiconductor substrate by reactive ion etching (RIE). Subsequently, the mask is removed and the channels a capped by a second wafer via hydrophilic wafer bonding [60]. An alternative fabrication scheme has been demonstrated in [31], where two planar waveguides (1DWG), which each confine the beam in an orthogonal direction, were combined in a crossed geometry to form an effective two-dimensional quasi-point source for holographic imaging. This crossed two-dimensional waveguide (c2DWG) scheme is compatible with fabrication by thin layer deposition. Hence, smaller guiding layers, a wider range of materials, and more complex layer sequences can be realized, including a two-component cladding optimized for high transmission [68]. Using for example an interlayer made of Mo, placed between the guiding core (C), and a high absorption cladding (Ge), this scheme provides excellent waveguides for the photon energy range between the Ge L-edges and the Mo K-edge, see Fig. 3.19. Figure 3.20 shows the measured far-field pattern of a Mo/C/Mo c2DWG system with guiding layer thickness $d=35$ nm. The far-field exhibits a relatively uniform intensity distribution in the center along with a characteristic arrangement of fringes in the tails. The large divergence reflects the small focal width of the waveguide as quantified reconstruction of the near-field intensity distribution by the error reduction (ER) algorithm [31]. The calculation of the field’s auto-correlation function by Fourier transformation of the far-field intensity can be used as a verification, since its width should give the value as the auto-correlation of the ER result.

Fig. 3.19

From [31]

Crossed planar waveguides. a Schematic. b Profiles $\text {Re}(n)$ and $\text {Im}(n)$ of the index of refraction $n=1-\delta +i\beta$, for photon energy $E=17.5$ keV. Transmission of the guided modes is increased by the high $\delta$ but relatively low $\beta$ of Mo. c Scanning electron microscopy (SEM) image (magnification 52.85 kx) showing the Mo/C/Mo layers in between Ge. The In52Sn48 alloy serves as bond material to an additional Ge cap wafer. d SEM micrograph 200 kx magnification.

Fig. 3.20

Adapted from [32]

X-ray waveguide beam with cross section at around 10 nm. a Fraunhofer far-field diffraction pattern of a beam exiting a crossed two-dimensional waveguide system (c2DWG) consisting of Mo/C/Mo layers, recorded with a pixel detector (Pilatus, Dectris Inc.) at a distance of about 5 m behind the waveguide exit ($E = 13.8$ keV, logarithmic scale, scale bar 0.02 Å$^{-1}$, 100 s dwell time). The two orthogonal slices had a guiding layer thickness of 35 nm, and a thickness of $l = 490\,\upmu$m (vertical slice: $l_1 = 270\,\upmu$m, horizontal slice: $l_2 = 220\,\upmu$m). A maximum (output) photon flux of $1.0 \times 10^8$ ph/s in the c2DWG beam was achieved by focusing a KB beam onto the waveguide entrance. b The near-field intensity distribution in the effective focal plane, obtained by inverting the diffraction pattern based on phase reconstruction by the error reduction (ER) algorithm (logarithmic scale, scale bar 20 nm). A high beam confinement in the effective confocal plane is achieved by multi-modal interference. c Line scans with corresponding Gaussian fits yield a FWHM of 10.7 and 11.4 nm in horizontal and vertical direction, respectively.

4.4 Advanced Waveguide Configurations

Waveguide optics enables a variety of optical functions, such as filtering, confining, guiding, coupling or splitting of beams. Advanced X-ray waveguides now begin to exploit such advanced functionalities, beyond simple filtering the mode structure of a synchrotron beam, which is already well established. Based on an array of waveguide channels, X-ray optics on a chip has been proposed in [69]. Beam concentration by tapering [58], guiding beams around a bent [69], and beam splitting for nano-interferometry [59], have also been demonstrated. In contrast to refractive or diffractive optics, X-ray waveguides are non-dispersive and can thus support broader bandpass. An advanced fabrication scheme with improved lithography, etching and wafer bonding steps has now paved the way to develop this field further [59]. Multiplexed beamlets can be particularly useful for coherent imaging [70], and possibly also X-ray quantum optical experiments [71]. As an example, the function of a waveguide beam splitter is illustrated in Fig. 3.21.

Fig. 3.21

From [59]

Minitaturized beam splitter based on X-ray waveguides. a Finite differences simulation of a beam splitter. b Top view SEM image of a splitting structure before wafer bonding. Scale bar denotes $1\,\upmu$m. c–f SEM images of the exit side of beam splitters with different spacings S. Scale bars denote 100 nm. g Schematic of the experimental geometry showing the coupling of the focused X-ray beam into the entrance of the beam splitter, the subsequent guiding in the two channels, the free space propagation behind the chip, and finally the far-field detector at a distance D. The far-field pattern shows the characteristic double slit interference pattern, modulated with features of the waveguide modal structure. Arrows mark bifurcations in the interference fringes (fork-shaped structures). Length and angles are not to scale. h Enlarged view of the interference pattern with a sinusodial fit to the intensity oscillations. i Scan in y-direction indicating the position of different beam splitters which have all been defined on the same chip with different geometric parameters, and which can be selected by translating the chip in the FZP focus. Detailed scan profile of a single channel with a width (FWHM) of 282.6 nm giving an upper limit for the beam size in the horizontal direction.

X-ray waveguides are also promising optical devices for the emerging field of ultra-fast X-ray optics at free electron laser (FEL) and higher harmonic generation (HHG) sources, since they support nearly dispersion-free pulse propagation down to ultra-short pulse width in the range of 0.1 fs [72]. FEL or HHG beam splitters with attosecond delay would be orders of magnitude smaller than macroscopic pulse delay stages. Spatial and temporal splitting of a pulse into two reflected beams, one displaced along the surface with respect to the other, can be also achieved by X-ray waveguides in resonant beam coupling geometry, based on a giant Goos-Hänchen effect [73]. As shown above for the stationary case, propagation is described by a finite number of guided modes, each with its own propagation constant and effective absorption index. The propagation of a short pulse is therefore governed by the effective dispersion and group velocity of the excited modes, which depend on the derivatives of the effective refractive indices for each mode. However, since these differ only very slightly, X-ray waveguides can be considered as nearly dispersion free optics down to femtosecond pulses, while dispersion effects start to become visible in form of mode separation only for attosecond pulses [72]. An example of pulse propagation in X-ray waveguides is shown in Fig. 3.22. A 12 keV pulse width of 5 attoseconds is simulated in a planar silicon (slab) waveguide with vacuum guiding layer of $d=100$ nm. The modes separate spatially by a few nm after several mm of propagation distances. Even if the pulse spectrum covers an absorption edge of the cladding material, modal dispersion could would manifest itself only for a pulse width of 0.3 fs, according to simulations by time-dependent finite difference propagation in [72].

Fig. 3.22

From [72]

a, b, c Intensity distribution (envelope) of a 5 attosecond beam propagating at different distances $z \approx 0.5\,\text {mm}$ (a), $z \approx 2\,\text {mm}$ (b), $z \approx 4 \, \text {mm}$ (c) in a silicon slab waveguide (air/vacuum guiding layer) of $100\,\text {nm}$ diameter. The waveguide’s edges are indicated by black lines.

5 Diffractive Optics and Zone Plates

In this section, we first recall the basic theory of Fresnel zone plate (FZP) optics, and then present different approaches of FZP fabrication. With the advent of improved fabrication techniques, smaller zones can now be achieved. However, this also required advanced optical design concepts and numerical methods for simulation, as presented in the last part of this section. Here we limit the discussion to the experimentally relevant case of binary zone plates, which are fabricated from two different materials; one of low and one of high density. The low density material can also be air or vacuum. 

5.1 Basic Theory of Fresnel Zone Plates

We assume a plane wave of wavelength $\lambda$ propagating along the optical axis and impinging on a circular aperture. The wave shall be focused to a point a distance f downstream the aperture. This focused wave is given as a sector of a spherical wave. The focus is formed by constructive interference of waves transmitted through rings around the optical axis, with radius $R=f+n\lambda /2$, $n\in 2\mathbb {N}_0$. Rings with $n\in 2\mathbb {N}_0+1$, on the other hand, would interfere destructively. The rings (or annuli) of different n form the so-called Fresnel Zones. These zones form concentric circles with radii

$$\begin{aligned} r_n = \sqrt{n\lambda f + \left( \frac{n\lambda }{2}\right) ^2}. \end{aligned}$$

(3.102)

For $n\ll f/\lambda = \mathcal {O}(10^7)$ for typical X-ray zone plates, the second term can be neglected. If now the “odd zones” with $n\in 2\mathbb {N}_0+1$ are blocked out in the aperture, the remaining waves interfere constructively in the focal spot. By Babinet’s principle, blocking the “even zones” will lead to the same intensity. An optical device which focuses light by absorbing light from the opaque rings is called an absorbing Fresnel Zone Plate. By blocking light in some areas, a bright spot appears on the optical axis. Jean-Auguste Fresnel was the first to obtain this result from calculation, as an extension of the optical phenomenon of Arago’s spot. As straightforward calculation shows, however, the focusing efficiency of such an absorbing FZP is limited to $1/\pi ^2\approx 10$% only (Fig. 3.23).

Fig. 3.23

From [74]

Schematic of a Fresnel zone plate (left) in the aperture plane, and (right) in a plane containing the optical axis. Different positive and negative diffraction orders m are obtained by positive interference.

Proposed by Lord Rayleigh in 1888, and first demonstrated by Wood ten years later, phase-reversing zones increase the efficiency to 40%. Instead of absorbing every other zone by a thick material, a relative phase-shift of $\pi$ is introduced. At hard X-ray energies of e.g. $E=12.4$ keV, it is challenging to achieve a full phase-shift of $\pi$. For example, for iridium with $n=1$–$2.19\times 10^{-5}$, an optical thickness of $2.28\,{\upmu }$m would be required. We discuss fabrication techniques and their advantages in the next subsection. The efficiency in the general case of a mixed absorbing/phase shifting zone plate follows further below.

Higher diffraction orders: Apart from the nominal focus at a distance f from the zone plate, higher orders at distances f/m, $m\in \mathbb {N}$, occur. Generalizing the zone plate law, the zone radii can be written as

$$\begin{aligned} r_n^2 \approx \frac{n}{m}\lambda f, \qquad n\in {m}\mathbb {N}_0. \end{aligned}$$

(3.103)

If now $m=2$, then $n/m\in \mathbb {N}_0$, and the neighbouring zones interfere destructively. So there is no focal spot at f/2 (in the thin zone plate approximation; the even spots can appear by volume effects, see later). This argument also holds for higher even numbers. For odd numbers, e.g. $m=3$, the condition for constructive interference is partly fulfilled for most of the zones. Hence, higher-order focal spots at f/3, f/5 etc. appear.

Negative diffraction orders: In the binary zone plates constructed as above, spherical waves converge onto the focal spot (and its higher order siblings). Applying the symmetry operations of time-reversal and inversion, however, also diverging waves are supported by the condition of constructive interference. Apart from the positive focal spots at f/m, also “negative orders” virtually emerging from spots located along the optical axis at $-f/m$ appear. These yield purely diverging waves. Usually, the negative and higher orders are blocked by a pinhole, the order sorting aperture (OSA).

Efficiency: In 1974, J. Kirz has presented a thorough treatment of Fresnel zone plates for soft X-rays, including the case of imaging at finite distances. Also, instead of purely absorbing or phase-shifting zones, the general case for a material with $n=1-\delta +i\beta$ was studied. Introducing the ratio $\eta :=\beta /\delta$, and using Fresnel integrals, the intensity of the first pair of zones can be written as

$$\begin{aligned} I&= |A_{0}+A_{1}|^2 = \frac{1}{2\pi } \int _0^\pi e^{is} \,\text {d}s + \frac{1}{2\pi } e^{-2\pi \beta t/\lambda } \int _\pi ^{2\pi } e^{i(s-2\pi t\delta /\lambda )}\,\text {d}s \end{aligned}$$

(3.104)

$$\begin{aligned}&= \frac{1}{\pi ^2} \left( 1+e^{-4\pi \beta t/\lambda } - 2e^{-2\pi \beta t/\lambda \cos (2\pi t\delta /\lambda )} \right) \end{aligned}$$

(3.105)

$$\begin{aligned}&= \frac{1}{\pi ^2} \left( 1+e^{-2\eta \varphi } - 2e^{-\eta \varphi } \cos \varphi \right) , \end{aligned}$$

(3.106)

with wavelength $\lambda$, optical thickness t and $\varphi :=2\pi t\delta /\lambda$. For all higher pairs of zones, the integrals evaluate to the same result, which can hence be regarded as the overall efficiency. We can deduce that even orders are not excited, and that higher (and negative) odd orders m are suppressed by a factor $1/m^2$. For optimal efficiency,

$$\begin{aligned} 0 = \frac{\partial I_1}{\partial \varphi ^*} \propto 1+e^{-2\eta \varphi ^*}-2e^{-\eta \varphi ^*}\cos \varphi ^*; \end{aligned}$$

(3.107)

for $\eta \rightarrow 0$, $\varphi ^*$ approaches $\pi$. The optimal optical thickness $t^*$ can be calculated as $t^*=\varphi ^*\,\lambda /(2\pi \delta )$.

Fig. 3.24

From [75]

Different steps in FZP fabrication by e-beam lithography. a A resist film is deposited by spin-coating. b The FZP pattern is written by the e-beam. c The illuminated resist is developed, leaving behind a pattern of circular trenches. d Metal (e.g. Ni) is grown by electrochemical methods. Electric conductivity is assured by the thin Au layer below. e The remaining resist is removed by solvent.

5.2 Fabrication Techniques

X-ray microscopy has for long been limited by the difficulties of fabricating high resolution and high quality X-ray lenses, notably Fresnel zone plates. In fact, soft X-ray microscopy started with FZP fabrication by holographic laser illumination, pioneered at Institut für Röntgenphysik by G. Schmahl and colleagues in the 1960s and 1970s [76,77,78]. Subsequently, this fabrication technique was replaced by e-beam lithography in the 1980ies, achieving a lateral resolution which was no longer limited by visible light. The different steps of FZP fabrication by e-beam lithography are illustrated in Fig. 3.24. Major challenges were both in the writing process, e.g. a suitable pattern generator, write-field limitations, and interferometric positioning minimizing stitching errors, as well as in the structure transfer by reactive ion etching (RIE). Continuous efforts have pushed the limits towards the 10 nm range for soft X-rays [34]. For hard X-rays, however, fabrication with larger aspect ratio (zone height to depth ratio) required to achieve the necessary phase shifts becomes much more demanding. Nevertheless, by seminal work of C. David and his group at the Paul-Scherrer-Institut diffractive optics is today also established in the hard X-ray regime. Special fabrication techniques such as zone-doubling have helped to increase the aspect ratio [79], and progress has cumulated in record focal spot sizes down to 17 nm (point focus) [80]. To push beyond these values, diffractive optics must be fabricated by thin film deposition and subsequent dicing. With magnetron sputtering (MS), for example, large thin films can be grown on a flat substrate. Two materials, one optically “thin” and one “thick”, can be deposited alternatively; this yields so-called multilayer Laue lenses (MLLs)  of virtually unlimited size [24, 25, 81]. Tens of thousands of bi-layer can be deposited with high accuracy. The final lens is then prepared by cutting out a slice of desired optical thickness using a focused ion beam (FIB) facility. Two such lamellae can then be used in series to form a two-dimensional focus. For a benchmark study with sub-10 nm point focus, see [23].

Contrarily, thin film deposition on a wire is called multilayer zone plate (MZPs). This goes back to an old idea [82, 83], which was also first implemented by magnetron sputtering (MS) and subsequent dicing [84]. This sputter-slice technique, however, was in most cases hampered by cumulative roughness, and the dicing also introduced severe artifacts. Only in recent years, these difficulties could be overcome by use of pulsed laser deposition (PLD). By this approach, the group of U. Krebs in Göttingen demonstrated cumulative smoothening of roughness [85] and was able to grow smooth multilayers with ultrathin layers. Using a FIB, the final lamella can be precisely cut to the desired optical thickness. Aspect ratios of one to several thousands can be achieved [86], and MZP optics has been implemented for hard X-ray energies in the broad range from $8\,\text {keV}$ up to above $100\,\text {keV}$ [87]. Figure 3.25a shows a sketch of MZP fabrication by PLD. An intense laser pulse is focused onto the target material (not shown), which then evaporates. A plasma plume forms, from which gas atoms are deposited on the substrate. Smoothing is favored by highly energetic particles with kinetic energies of up to $100\,\text {eV}$, resulting in high mobility and enhanced diffusion on the substrate surface. Advanced focused ion beam (FIB) cutting and manipulation protocols yield well positioned and mounted MZPs [86, 88]. For the MZP shown in Fig. 3.25b, a computer controlled KrF excimer laser (wavelength of $248\,\text {nm}$) was used with pulse duration of $30\,\text {ns}$ and repetition rate of $10\,\text {Hz}$. The laser beam was focused onto the different targets in ultrahigh vacuum of about $10^{-8}\,\text {mbar}$. The targets were moved constantly following an algorithm that allows uniform ablation from different directions. The films were grown at room temperature at a target-to-substrate distance of $6.5\,\text {cm}$ [88]. The latest generation of lenses are fabricated from Ta$_2$O$_5$ and ZrO$_2$. For more information, see the progress report in the second part of this book.

Fig. 3.25

a Schematic of thin film deposition on a rotating wire, e.g. by pulsed laser deposition. The zone plate is subsequently diced by a focused ion beam to the desired optical length. Adapted from [75]. b Transmission electron micrograph of a MZP lens, consisting of alternating thin layers of ZrO$_2$ and Ta$_2$O$_5$ fabricated by PLD and FIB on a rotating pulled glass wire with diameter $2r_0=1.2\,\upmu \text {m}$. The diameter is $D=3.2\,\upmu \text {m}$, the outer-most zone width is $dr_{81}=10.0$ nm, the focal length for the photon energy $E=18$ keV was $f=470\,\upmu$m [27]

5.3 Diffractive Optics Beyond the Projection Approximation

Above, we have described the working principle of optically thin zone plates. In the general case of a partially absorbing and phase-shifting zone plate, it is modelled as a complex-valued phase mask $\tau$ in two dimensions; the impinging wave-front $\psi$ is modulated by this phase mask. Numerically, this is calculated as a pixel-wise multiplication of two matrices:

$$\begin{aligned} \psi ' := \tau \psi , \qquad \psi '_{i,j} := \tau _{i,j} \cdot \psi _{i,j}. \end{aligned}$$

(3.108)

In the soft X-ray regime, where the optical thickness of FZPs is usually on the order of a few hundred nanometres, this model can usually be justified. We define the zone plate Fresnel number $F_\text {ZP}$ as

$$\begin{aligned} F_\text {ZP} := \frac{\left( \varDelta r_N\right) ^2}{\lambda \,t} \end{aligned}$$

(3.109)

with outermost zone width $\varDelta r_N=r_N-r_{N-1}$, wavelength $\lambda$, and thickness t. For $\varDelta r_N\ge {30}\,{\mathrm{nm}}$, $\lambda \approx {3}\,{\mathrm{nm}}$ and $t\le {300}\,{\mathrm{nm}}$ as an example of a soft X-ray FZP, $F_\text {ZP}\ge 1$; hence the treatment of a thin zone plate based on the projection approximation is completely adequate. For hard X-rays, however, we easily achieve $\varDelta r_N={5}\,{\mathrm{nm}}$, $\lambda ={0.1}\,{\mathrm{nm}}$ and $t={5}\,{\upmu \mathrm{m}}$, resulting in $F_\text {ZP}=0.05$. This gives a clear indication that diffraction effects within the FZP itself have to be accounted for. More specifically, the kinematic or Born approximation of single diffraction at the phase mask $\tau$ has to be replaced by dynamical diffraction theory. For such optically thick optics, volume effects have to be taken into account.

Fig. 3.26

From [27]

a Schematic of diffraction orders and multi-order focusing. Positive orders are shown in red, negative in green. The orders result from the binary nature of the zone plate. For FZP imaging, order sorting apertures can be used to select only the $m=+1$ diffraction order. Alternatively, algorithmic schemes to dissect different orders can be used, which is, however, still challenging [27]. The reconstruction of the focal field can be achieved by iterative projection algorithms [4, 27]. b Simulation of the focused intensity, on a logarithmic false-color representation along the optical axis; compare to (a).

A Takagi-Taupin based  theory for MLL optics has been derived by Yan et al. [89], extending previous dynamical treatments denoted as coupled wave theory [2, 90, 91]. Here we briefly summarise their model and findings of [89]. The derivation is similar to that presented above for multilayer mirrors (MLMs) and starts with the Helmholtz equation of a scalar or vector field amplitude that interacts with the pseudo-periodic susceptibility $\chi (\mathbf {r})$. For MLMs, the Fourier series can be truncated after one term, and only two beams (incoming and reflected) are considered. MZPs, on the other hand, show multiple diffractive orders, and hence multiple beams and more Fourier orders need to be taken into account. A further complication arises since $\chi (\mathbf {r})$ is not a simple periodic function, but changes according to the zone plate law. Nevertheless, Yan and co-workers argue that the zone plate can be considered as a “strained crystal” with a varying d-spacing of $d=2\varDelta r_n$. They use the coordinate transformation (Fig. 3.26)

$$\begin{aligned} x \mapsto \frac{T}{\lambda }\left( \sqrt{x^2+f^2}-f\right) , \end{aligned}$$

(3.110)

where T is the period of the new, unstrained and fully periodic lattice. The transformation yields a phase-factor

$$\begin{aligned} \exp \left( i\,\phi _h\right) := \exp \left( ikh(\sqrt{x^2+f^2}-f)\right) \end{aligned}$$

(3.111)

where h is the index of the diffractive order under consideration. Decomposing the field $\mathbf {E}$ into components $\mathbf {E}_h$, and using the a truncated series expansion for $\chi$, yields a set of coupled partial differential equations describing the system. Within the Takagi-Taupin formalism, the gradient of the phase $\phi _h$, is equal to the local reciprocal lattice vector:

$$\begin{aligned} \rho _h := \frac{2\pi h}{d}\hat{e}_x = \mathbf {\nabla }\phi _h. \end{aligned}$$

(3.112)

This shows that apart from the geometrical considerations for constructive interference discussed above, zone plates can also be considered as crystal optics where the local d-spacing is chosen such that all diffracted beams of a certain order h point to the same focal spot. When volume diffraction occurs, the diffracted X-ray beams are disturbed significantly within the structure. Geometrically speaking, a ray diffracted at the entrance of the zone plate at a specific zone would enter another zone while traversing the zone plate. Then, multiple diffraction will not follow Bragg’s law. To match the diffraction angles, the originally parallel zones have to be varied along the optical axis. To this end, Yan et al. discuss tilted, wedged, and curved zones. Based on their computations, a focusing efficiency of 67% at sub-1 nm spot sizes at a photon energy of 19.5 keV is possible using MLLs fabricated from Si and WSi$_2$ [89].

6 Basic Coherence Theory and Simulations for X-ray Optics

Coherence of light beams refers to their ability to exhibit interference effects. Already in the first interference experiment of light, the famous double slit-experiment of 1801, Thomas Young discussed the “visibility of fringes”, which today is referred to as the degree of coherence $\gamma (\mathbf {r}_1,t_1,\mathbf {r}_2,t_2)$ between two time-space-points $(\mathbf {r}_1, t_1)$ and $(\mathbf {r}_2, t_2)$. Whenever light waves emerging from these two points superimpose at a third point, the total intensity $I_{1,2}$ in general differs from the sum of the single intensities, $I_{1,2}\ne I_1+I_2$. This is immediately clear since Maxwells’ equations are linear in the amplitudes u, but not the intensity $|u|^2$

$$\begin{aligned} u_{1,2}= & {} u_1 + u_2 \\ I_{1,2}= & {} \left| u_1+u_2\right| ^2 = |u_1|^2 + |u_2|^2 + 2\gamma _{1,2}|u_1||u_2| \ne |u_1|^2+|u_2|^2. \end{aligned}$$

In the following, we first give the basic definitions of coherence functions from literature; afterwards, we will shortly discuss an analytical treatment for synchrotron radiation. Using a stochastic model suitable for the numerical treatment of partially coherent propagation of light through various optical elements and samples, we will show that X-ray waveguides can indeed be used as coherence filtering devices. 

6.1 Basic Definitions

Consider a scalar wave-field with complex amplitude $u(\mathbf {r}, t)$. Then the intensity $I(\mathbf {r}, t)$ at the space-time-point $(\mathbf {r}, t)$ can be defined as

$$\begin{aligned} I(\mathbf {r}, t):= & {} u^*(\mathbf {r}, t)\,u(\mathbf {r}, t), \end{aligned}$$

(3.113)

and the mutual intensity $\varGamma (\mathbf {r}_1, t_1, \mathbf {r}_2, t_2)$ between the given two space-time-points as

$$\begin{aligned} \varGamma (\mathbf {r}_1, t_1, \mathbf {r}_2, t_2):= & {} u^*(\mathbf {r}_1, t_1)\,u(\mathbf {r}_2, t_2). \end{aligned}$$

(3.114)

Note that higher-order correlations involving more than two space-time points can be defined, but are rather uncommon in practice. The temporal Fourier transform $W_{1,2} = \int ~d\tau ~\varGamma ~\exp \left( i \omega \tau \right)$ of the mutual intensity is known as the cross-spectral density. Note that the indices (1, 2) are often used for notational simplification to denote the two space-time-points. The normalized mutual intensity

$$\begin{aligned} \gamma (\mathbf {r}_1, t_1, \mathbf {r}_2, t_2):= & {} \frac{\varGamma (\mathbf {r}_1, t_1, \mathbf {r}_2, t_2)}{\sqrt{I(\mathbf {r}_1, t_1)\,I(\mathbf {r}_2, t_2)}} \end{aligned}$$

(3.115)

is a measure of the cross-correlation of the fields at two different points in space and time. For stationary signals, $\gamma$ depends only on the time difference $\tau =t_2-t_1$ and is also denoted as the complex degree of coherence. Further, for quasi-monochromatic waves, it is sufficient to consider the mutual intensity at the same-time $t_1=t_2$, since the time-harmonic variation of the fields is trivial. This same-time mutual intensity depends only on the spatial coordinates of two points

$$\begin{aligned} J(\mathbf {r}_1, \mathbf {r}_2):= & {} \langle u^*(\mathbf {r}_1)\,u(\mathbf {r}_2)\rangle _T, \end{aligned}$$

(3.116)

where $\langle \dots \rangle _T$ denotes the time-average over at least a period T, or for practical purposes the illumination time of the experiment. The mutual intensity J contains all information about measurable intensities. Finally, the normalized same-time mutual intensity is defined as

$$\begin{aligned} j(\mathbf {r}_1, \mathbf {r}_2):= & {} \frac{J(\mathbf {r}_1, \mathbf {r}_2)}{\sqrt{J(\mathbf {r}_1, \mathbf {r}_1)\,J(\mathbf {r}_2, \mathbf {r}_2)}} ~. \end{aligned}$$

(3.117)

We use the normalized quantity j, if we are interested in the visibility of interference fringes, for example, not the absolute intensity values. If one considers a Young’s double slit experiment with quasi-monochromatic light and two slits at points $\mathbf {r}_1$ and $\mathbf {r}_2$, the emitted spherical waves yield an interference pattern, with the fringe visibility (i.e. the Michelson contrast of the fringes) given by |j|. For $|j|<1$ we call the light field partially coherent, whereas $|j|=1$ and $|j|=0$ denote fully coherent and incoherent light, respectively. These limiting cases can in fact never be completely realized.

In many practical problems, we are furthermore primarily interested in evaluating j in a plane orthogonal to the optical axis, e.g. to study coherence in the plane of an optic, sample or detector. The coherence properties in any one of such planes, however, evolve as the beam propagates. For matter of concreteness, let z be the optical axis, and let y denote the lateral direction of interest. For simplicity, we drop the dependence on x, and consider z as a parameter. In view of interference, we are often interested in the field correlation between point $y_1$ and another point $y_2$ at a lateral distance $y_2=y_1+d$. For linear optical systems which are characterized by lateral shift invariance, the degree of coherence is homogeneous in planes perpendicular to the optical axis, and hence

$$\begin{aligned} j_z (d):= & {} j\big ((y,z), (y+d,z)\big ). \end{aligned}$$

(3.118)

For experiments it is then sufficient, for example, to fix one point to the optical axis and to measure $j(d) := j\big ((0,z), (d,z)\big )$. What can we say about the functional dependence of j(d)? We expect the correlation to decrease with distance d (must not always be the case!), and would like to associate a characteristic length $\xi$ to the decay of j(d). For the simplest case of an incoherent field in plane $z=0$ and paraxial propagation, it can be shown that the degree of coherence is given by a Fourier transform of the intensity in the source plane (van Cittert-Zernike theorem)  

$$\begin{aligned} j_z (d) = \int _{-\infty }^{\infty } ~dy'\, I(y') \,\exp \left( i \frac{2\pi }{\lambda z} {y'}d \right) , \end{aligned}$$

(3.119)

i.e. we can easily predict how the coherence evolves by propagation. By ways of this Fourier relationship, we see that the spatial coherence length scales as

$$\begin{aligned} \xi _{\perp } = \frac{\lambda z}{2s} , \end{aligned}$$

(3.120)

where s is the source size. Correspondingly, $\xi /z$ defines a “coherence angle”. Note that a precise definition of $\xi$ would require us to be more precise about the cut-off value, to which j would be allowed to decrease, as well as more information or assumption on the source distribution $I(y')$. However, this may all be incorporated into a prefactor. As an example, let us consider the 3rd generation synchrotron source PETRA III with a horizontal source size of $\sigma ={36}\,{\mathrm{nm}}$ ($1\sigma$) in the low $\beta$ sections. For $\lambda ={0.1}\,{\mathrm{nm}}$ the coherence angle is $\vartheta _\text {coh}\sim {2}\,{\upmu }\mathrm{rad}$. Typical optics, however, accept a beam angle of about ${5}\,{\upmu }\mathrm{rad}$, and the full beam has an opening angle of ${100}\,{\upmu }\mathrm{rad}$. One is thus facing the situation of rather reduced partial coherence, and small entrance slits or pinholes are required for coherent imaging or photon correlation spectroscopy experiments. For more information and the useful Gaussian shell model (GSM) to describe coherence properties of synchrotron beams, we refer to [92, 93]. Note that synchrotron sources are actually not fully incoherent; the radiation by the ultra-relativistic electron beam with $\gamma =\left( 1-(v/c)^2\right) ^{-1/2}\sim 10^4$ is confined to a small cone with opening $\vartheta \sim {10^{-4}}\,\mathrm{rad}$. This already yields a considerable correlation “already in the source plane”, which can be incorporated in the GSM by an additional parameter. Nevertheless, at the position of experiments, $\xi$ is dominated by propagation. While $\xi _{\perp }$ is the length scale characteristic for the correlations across a wavefront, coherence along the direction parallel to the optical axis is characterized by the longitudinal or temporal coherence length

$$\begin{aligned} \xi _z = \frac{\lambda ^2}{2\varDelta \lambda } , \end{aligned}$$

(3.121)

where $\varDelta \lambda$ is the spectral width. This relationship follows from the Wiener-Khinchin theorem.

6.2 Stochastic Model

How can we model the emission of “chaotic sources”? Such sources do not emit plane waves, but rather wave-trains of finite duration, and hence of finite bandwidth. In addition, each emitter acts on its own, independent of its neighbours. Say the temporal duration of a wave-train is $\tau _\text {train}$. But during the acquisition time $\tau _\text {acq}\gg \tau _\text {train}$, a detector registers light from many subsequent wave-trains, each of which has been emitted with a random phase. While a single wave-train may give rise to a fully coherent interference pattern, the patterns of different wave-trains are out of phase and hence shifted spatially. Over sufficiently long times, the patterns wash out, the interference fringes vanish. Usually, for monochromatized synchrotron radiation the relative bandwidth is on the order of $10^{-4}$ (common Si-111 monochromator). At X-ray frequencies of $\omega \sim {10^{18}}\mathrm{s}^{-1}$, this yields correlation times of $\tau _\text {c}\sim {10^{-14}}\mathrm{s}$ or shorter. This is of course well below the response time of typical detectors and shorter than the pulse duration. At X-ray free electron laser sources, however, extremely short pulses can be produced, and we can expect full temporal coherence. The finite temporal or longitudinal coherence time directly translates to the corresponding length $\xi _z=c\tau _c\sim {1}\,{\upmu }$m, and hence also the largest possible path length difference between two interfering waves is still much larger than molecular length scales.

Based on this simple picture of finite wave trains with stochastic phases varying in time and space, we can introduce a simple stochastic model to treat partial coherence numerically. We replace the continuous extended source of size D by a set of N independent point-sources. The field by each point-source can be propagated numerically through an optical system, e.g. by solving the Fresnel-Kirchhoff integral for focussing mirrors, or the paraxial wave-equation for waveguides. For each point-source, a complex-valued field in some region of interest is thus obtained, denoted by $u_n(x,y)$ for the nth point-source and given in a two-dimensional area (the generalisation to three dimensions is obvious). We define a single stochastic realisation as the random superposition

$$\begin{aligned} u_\text {speckle}:= & {} \sum _n w_n\,c_n\,u_n \end{aligned}$$

(3.122)

with random phase factors $c_n=\exp \left( i\varphi _n\right)$, $\varphi _n\in (0,2\pi ]$, and with weighting factors $w_n$ corresponding to the intensity envelope of the source. A single realisation corresponds to the interference pattern of a short wave-train with coherence time $\tau _\text {c}$; the pattern of a long exposure time $\tau \gg \tau _\text {c}$ is modelled for the time-average $\langle \dots \rangle _\tau$ over superpositions with random phase coefficients $c_n$. Numerically, a few thousand realisations should be taken into account. From such an ensemble, we calculate the degree of coherence j as

$$\begin{aligned} j_{1,2}:= & {} \frac{\langle u^*_1\,u_2 \rangle }{\sqrt{\langle u^*_1 u_1\rangle \,\langle u^*_2 u_2\rangle }} = \frac{\langle u^*_1\,u_2 \rangle }{\sqrt{I_1\,I_2}}. \end{aligned}$$

(3.123)

6.3 Coherence Propagation and Filtering

Using the stochastic model, the partially coherent  X-ray intensity and the degree of coherence can be propagated through an optical system. Here we briefly illustrate this with an example of mode propagation in X-ray waveguides, which can be used as coherence filters [66]. We also address nano-focusing of partially coherent radiation, using the example of the KB focus of the GINIX endstation at the P10 beamline of the PETRAIII storage ring at DESY [45, 51, 94]. Figure 3.27a shows the simulated intensity distribution $I(x,y)=J((x,y),(x,y))$ in the focal region (colour-coded) for X-ray photon energy $E={7.9}\,\mathrm{keV}$, and the geometric parameters of the horizontally focusing mirror (HFM). The green curves show iso-lines of the degree of coherence $|j(d,z)|=|j(x=0,z,x=d,z)|$ along the optical axis. As can be seen, only the central part of the intensity distribution is coherent. In fact, the spatial coherence drops to 0.5 for separations largerthan the predicted coherence length $\xi$, which in the focal plane is 74 nm, while the FWHM beam size is 220 nm. Using Talbot interferometry, a beam size of 203 nm (FWHM of a Gaussian fit) and a coherence length of $0.37\times {203}\,{\mathrm{nm}}={75}\,{\mathrm{nm}}$ have been measured [94]. To filter the coherent part of the illumination, an X-ray waveguide can be placed into the focal spot. In the simulation, a $D={50}\,{\mathrm{nm}}$ guiding layer (vacuum) in Si is illuminated by independently propagated point-sources, and the complex valued amplitudes are propagated using the paraxial methods described above. On this set of basic fields $u_n$, a stochastic ensemble is performed. The result is shown in Fig. 3.27b. Again, the colour-code shows the partially coherent intensity $I(x,z)=J(x,z,x,z)$; as can be seen, only the central part of the focused beam is coupled into the guiding layer. As the iso-lines of coherence along the optical axis show, the beam is now fully coherent. This effect has also been demonstrated experimentally [94].

Fig. 3.27

From [66]

Focus and coherence properties of KB focused (left) and WG filtered (right) X-ray beams: a shows the color-coded intensity distribution in the focal spot of the KB mirror, with isolines of the degree of coherence ($j=\lbrace 0.4,0.6,0.8\rbrace$); in b, the focused field is coupled into a WG. Plots c–f are cuts of intensity (red) and coherence (green) at the indicated positions (focal spot, after ${1}\,{\upmu }$m; at the center of the WG, after ${0.1}\,{\upmu }$m).

Using X-ray waveguides, it is also possible to directly measure the intensity distribution, by scanning them through the focal plane. This is of interest for characterisation of X-ray nano-focus instruments. Figure 3.28 shows results obtained in a characterisation of the KB optics at the NanoMAX beamline at MAX IV synchrotron in Lund. With a high dynamical range of larger than $1:10^4$, it is even possible to resolve interference patterns due to slit scattering of the KB entrance slit. From the visibility of these fringes, coherence properties can be quantified. This finite, or partial, coherence reduces the visibility of interference fringes. The resulting intensity pattern in the focal plane has been calculated based on an analytical model. Together with the measured profiles, the degree of coherence was measured as a function of (secondary) source size.

Fig. 3.28

Characterisation of focus and lateral coherence of a hard X-ray nanoprobe (NANOMAX beamline, MAXIV synchrotron). The focal intensity distribution is measured by scanning a waveguide through the focus. The coherence properties are quantified by analysis of the fringe visibili ty of the KB tails. a Two measured focus profiles of the KB mirror for partially coherent setting (secondary source size ${20}\,{\upmu }$m, blue dashed curve) and quasi-coherent setting (secondary source size ${5}\,{\upmu }$m, red line), shown on linear (left) and logarithmic (right) scale. b Intensity profiles of the three guided waveguide modes (left), and superposition of the three modes (right). c Simulated and experimental focus tails, for different coherence settings. The blue line shows the simulated intensity in a fully coherent setting; the red dashed line is convolved with the waveguide mode structure; the green pointed line is calculated for a secondary source size of ${15}\,{\upmu }$m (and convolved with the waveguide guiding channel). The black circles represent experimental data for a secondary source size of ${15}\,{\upmu }$m, with a sinusoidal fit shown as a black line. Experimental data has been shifted by a factor of 1 : 3.5 vertically for clarity. d Fringe contrast, quantifying the degree of coherence, as a function of the secondary source size. The blue thin line is simulated for ideal sampling, while the red thick line accounts for the convolution with the WG modes. The orange circles show contrast fits for experimental data; orange lines correspond to $2\sigma$ error bars of the fit. From [95]

7 Putting It All Together: Optics and X-ray Instrumentation

As a last note, we want to stress the importance of integrating X-ray optics into an instrument, be it an X-ray microscope, diffractometer or spectrometer. Notwithstanding the importance of its individual optical components, such as mirrors, waveguides, CRLs or Fresnel zone plates, the larger challenge is to put it all together into a fully working synchrotron instrument and beamline. The tasks are many: optical design and simulation, instrument control, radiation safety, precision, as well as data acquisition and management. Unlike other analytical techniques, instrumentation development is still largely in the hands of research groups, rather than commercial providers. The diversity of SR instruments is impressive. For a comprehensive view, we refer to the online documentation provided by almost all SR facilities, the beamline articles of IUCr’s Journal of Synchrotron Radiation, and the proceedings of the recent international conference on SR instrumentation. Instead of even trying to give an overview, we present an example: the Göttingen Instrument for Nano-Imaging with X-rays (GINIX) at the P10 coherence beamline of the PETRA III storage ring at DESY in Hamburg [45]. The instrument has been designed based for nanoscale focusing using compound optical systems. This can be either the combination of a focusing and a filtering step [30, 31, 67], or by two sequential focusing steps [4, 27, 81].

Fig. 3.29

The GINIX endstation, installed in the second experimental hutch (EH2) of the PETRAIII/P10 beamline at DESY. a Schematic of the beamline’s optical path, with (A) undulator, (B) primary slits, (C) secondary slits, (D) double crystal monochromator, (E) horizontal mirrors, (F) girder system with slits, (G) slits, (H) fast shutter, (I) monitor, (J) attenuators, (K) experimental setup in EH1, (L) slits, (M) monitor, (N) experimental setups in EH2 (GINIX or diffractometer), (O) rear detector bench. From [96]. b CAD drawing, showing (from right to left) the KB mirror tank (light grey), the hexpod with the waveguide optics (dark grey), the mounting for the cleaning apertures (dark grey top), online optical microscopes for alignment and inspection (yellow, brown), and the tomography sample stage (blue). c Photograph of the instrument

Fig. 3.30

From [45]

Ptychographic reconstruction of the KB probe at 13.8 keV with KB entrance slits $100\,\upmu \text {m} \times 100\,\upmu \text {m}$, reconstructed from data recorded by the Lambda detector and without pinhole (dataset 3 in [97]). a Amplitude and phase are drawn according to the colorbar next to the image. Vertical and horizontal line-cuts through the intensity in the focal plane yield $\text {FWHM} = 217$ nm and $\text {FWHM} = 136$ nm, respectively. b, c Intensity distribution as calculated from numerical propagation of the reconstructed field, in the b xy and c the xz planes. d Normalized sharpness as obtained from an area integral of the squared intensity, along with a Lorentzian fit, yielding a depth of focus (DOF) of 1.53 mm (FWHM). Scale bar in a 500 nm.

Figure 3.29 illustrates (a) the optical path of the P10 beamline, with its components and respective distances from the undulator source, as well as (b, c) the nano-focus optics and sample stage of the instrument. GINIX comprises a modular compound nano-focus optical system, composed of a high gain fixed curvature (KB) mirror and an X-ray waveguide module, which is used for holographic imaging and tomography [61]. For scanning SAXS recordings [98], a soft-edge aperture is used to clean the KB tails. Ultimate sub-10 nm focusing is possible with a high resolution scanning stage, equipped with MZP optics [27]. Three major imaging modalities are supported by the instrument: (i) near-field phase contrast imaging, also denoted as in-line holographic imaging, (ii) far-field coherent diffractive imaging (CDI) with ptychographic phase retrieval, and (iii) scanning nano-diffraction, in the small angle or wide-angle regime (scanning SAXS/WAXS). The KB mirrors are positioned at ${\sim } 85$ m behind the undulator source, and can be operated in the photon energy range between 6 and 14 keV [45]. The two orthogonal mirrors with Rh coating are polished to fixed elliptical curvature are each 100 mm long, and accept a maximum beam size of ${\simeq }0.4$ mm. The first mirror focuses in vertical direction (VFM) with focal length $f=302$ mm and incidence angle $\theta =3.954$ mrad (mirror center), the second mirror focuses in horizontal direction (HFM) with focal length $f=200$ mm and incidence angle $\theta =4.05$ mrad (mirror center). Optical metrology has confirmed a surface quality with height deviation of ${\le }0.5$ nm peak-to-valley. To reduce beam-induced degradation, the KB system is operated in a ultra high vacuum vessel. The beam size impinging onto the mirrors can be controlled by slits. When the slits are opened, the mirrors are operated under conditions of partial coherence, since the geometric acceptance exceeds the spatial coherence length. However, at the expense of flux density, one can select the coherent fraction by closing the slits in front of the KB [99]. The focus then becomes diffraction limited, i.e. fully coherent, which is important for coherent diffractive imaging (CDI) and ptychography. Depending on orbit parameters, slit settings and alignment status, focal spot sizes down to about ${\simeq } 200\,\mathrm{nm}\times 200\,\mathrm{nm}$ (FWHM, as measured by waveguide scans) can be achieved with a flux larger than $10^{11}\,\mathrm{ph/s}$ [94]. Coherent illumination of the mirrors by closing the slit in front of the KB results in focal spot sizes focus sizes in the range of 150–500 nm [97, 99,100,101]. A ptychographic probe reconstruction is shown in Fig. 3.30. Different ptychographic and multi-plane probe reconstruction methods have been compared in [102]. As one can see, quite a bit of effort is required to realize optics in the “vacuum limit”!